Hostname: page-component-78c5997874-m6dg7 Total loading time: 0 Render date: 2024-11-10T15:14:57.731Z Has data issue: false hasContentIssue false
  • English
  • Français

An optimal improvement for the Hardy inequality on the hyperbolic space and related manifolds

Part of: Other generalizations Linear function spaces and their duals Inequalities

Published online by Cambridge University Press:  01 February 2019

Elvise Berchio ,
Debdip Ganguly ,
Gabriele Grillo  and
Yehuda Pinchover
Elvise Berchio
Affiliation:
Dipartimento di Scienze Matematiche, Politecnico di Torino, Corso Duca degli Abruzzi 24, 10129Torino, Italy (elvise.berchio@polito.it)
Debdip Ganguly
Affiliation:
Department of Mathematics, Indian Institute of Science Education and Research, Dr. Homi Bhabha Road, Pashan Pune411008, India (debdipmath@gmail.com)
Gabriele Grillo
Affiliation:
Dipartimento di Matematica, Politecnico di Milano, Piazza Leonardo da Vinci 32, 20133 Milano, Italy (gabriele.grillo@polimi.it)
Yehuda Pinchover
Affiliation:
Department of Mathematics, Technion – Israel Institute of Technology, Haifa3200003, Israel (pincho@technion.ac.il)
Get access Rights & Permissions [Opens in a new window]

Abstract

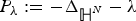We prove optimal improvements of the Hardy inequality on the hyperbolic space. Here, optimal means that the resulting operator is critical in the sense of Devyver, Fraas, and Pinchover (2014), namely the associated inequality cannot be further improved. Such inequalities arise from more general, optimal ones valid for the operator $ P_{\lambda }:= -\Delta _{{\open H}^{N}} - \lambda $ where 0 ⩽ λ ⩽ λ1(ℍN) and λ1(ℍN) is the bottom of the L2 spectrum of 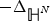$-\Delta _{{\open H}^{N}} $, a problem that had been studied in Berchio, Ganguly, and Grillo (2017) only for the operator 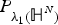$P_{\lambda _{1}({\open H}^{N})}$. A different, critical and new inequality on ℍN, locally of Hardy type is also shown. Such results have in fact greater generality since they are proved on general Cartan-Hadamard manifolds under curvature assumptions, possibly depending on the point. Existence/nonexistence of extremals for the related Hardy-Poincaré inequalities are also proved using concentration-compactness technique and a Liouville comparison theorem. As applications of our inequalities, we obtain an improved Rellich inequality and we derive a quantitative version of Heisenberg-Pauli-Weyl uncertainty principle for the operator $P_\lambda.$

Keywords

Hyperbolic spaceoptimal Hardy inequalityextremals

MSC classification

Primary: 46E35: Sobolev spaces and other spaces of “smooth” functions, embedding theorems, trace theorems
Secondary: 26D10: Inequalities involving derivatives and differential and integral operators 31C12: Potential theory on Riemannian manifolds
Type
Research Article
Information
Proceedings of the Royal Society of Edinburgh Section A: Mathematics , Volume 150 , Issue 4 , August 2020 , pp. 1699 - 1736
Check for updates
Copyright
Copyright © Royal Society of Edinburgh 2019

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

1Adimurthi. Hardy-Sobolev inequality in H 1(Ω) and its applications. Commun. Contemp. Math. 4 (2002), 409–434.CrossRefGoogle Scholar
2Akutagawa, K. and Kumura, H.. Geometric relative Hardy inequalities and the discrete spectrum of Schrödinger operators on manifolds. Calc. Var. Partial Differential Equations 48 (2013), 67–88.CrossRefGoogle Scholar
3Arapostathis, A., Biswas, A. and Ganguly, D.. Some Liouville-type results for eigenfunctions of elliptic operators. Trans. Amer. Math. Soc. 371 (2019), 4377–4409.CrossRefGoogle Scholar
4Baernstein, A. II. A unified approach to symmetrisation, Partial differential equations of elliptic type. In Sympos. Math., XXXV (Cortona, 1992), pp. 47–91, Cambridge University Press, Cambridge, 1994.Google Scholar
5Barbatis, G. and Tertikas, A.. On a class of Rellich inequalities. J. Comput. Appl. Math. 194 (2006), 156–172.CrossRefGoogle Scholar
6Barbatis, G., Filippas, S. and Tertikas, A.. Series expansion for L p Hardy inequalities. Indiana Univ. Math. J. 52 (2003), 171–190.Google Scholar
7Barbatis, G., Filippas, S. and Tertikas, A.. A unified approach to improved L p Hardy inequalities with best constants. Trans. Amer. Soc, 356 (2004), 2169–2196.CrossRefGoogle Scholar
8Berchio, E. and Ganguly, D.. Improved higher order Poincaré inequalities on the hyperbolic space via Hardy-type remainder terms. Commun. on Pure and Appl. Analysis 15 (2016), 1871–1892.Google Scholar
9Berchio, E., Ganguly, D. and Grillo, G.. Sharp Poincaré-Hardy and Poincaré-Rellich inequalities on the hyperbolic space. J. Funct. Anal. 272 (2017a), 1661–1703.CrossRefGoogle Scholar
10Berchio, E., D'Ambrosio, L., Ganguly, D. and Grillo, G.. Improved Lp-Poincaré inequalities on the hyperbolic space. Nonlinear Anal. 157 (2017b), 146–166.CrossRefGoogle Scholar
11Bianchini, B., Mari, L. and Rigoli, M.. Yamabe type equations with sign-changing nonlinearities on non-compact Riemannian manifolds. J. Funct. Anal. 268 (2015), 1–72.CrossRefGoogle Scholar
12Bianchini, B., Mari, L. and Rigoli, M.. Yamabe type equations with a sign-changing nonlinearity. and the prescribed curvature problem. J. Differential Equations 260 (2016), 7416–7497.CrossRefGoogle Scholar
13Brezis, H. and Marcus, M.. Hardy's inequalities revisited. Ann. Scuola Norm. Sup. Cl. Sci. (4) 25 (1997a), 217–237.Google Scholar
14Brezis, H. and Vazquez, J. L.. Blow-up solutions of some nonlinear elliptic problems. Rev. Mat. Univ. Complut. Madrid 10 (1997b), 443–469.Google Scholar
15Carron, G.. Inegalites de Hardy sur les varietes Riemanniennes non-compactes. J. Math. Pures Appl. (9) 76 (1997), 883–891.CrossRefGoogle Scholar
16Castorina, D. and Sanchon, M.. Regularity of stable solutions to semilinear elliptic equations on Riemannian models. Adv. Nonlinear Anal. 4 (2015), 295–309.CrossRefGoogle Scholar
17Cycon, H.L., Froese, R.G., Kirsch, W., Simon, B.. Schrödinger operators with application to quantum mechanics and global geometry, Springer Study edn. Texts and Monographs in Physics (Berlin: Springer-Verlag, 1987).CrossRefGoogle Scholar
18D'Ambrosio, L. and Dipierro, S.. Hardy inequalities on Riemannian manifolds and applications. Ann. Inst. H. Poinc. Anal. Non Lin. 31 (2014), 449–475.CrossRefGoogle Scholar
19Devyver, B., Fraas, M., Pinchover, Y.. Optimal Hardy weight for second-order elliptic operator: an answer to a problem of Agmon. J. Funct. Anal. 266 (2014), 4422– 4489.CrossRefGoogle Scholar
20Filippas, S. and Tertikas, A.. Optimizing improved Hardy inequalities. J. Funct. Anal. 192 (2002), 186–233.CrossRefGoogle Scholar
21Filippas, S., Tertikas, A. and Tidblom, J.. On the structure of Hardy-Sobolev-Maz'ya inequalities. J. Eur. Math. Soc. 11 (2009), 1165–1185.CrossRefGoogle Scholar
22Filippas, S., Moschini, L. and Tertikas, A.. Sharp trace Hardy-Sobolev-Maz'ya inequalities and the fractional Laplacian. Arch. Ration. Mech. Anal. 208 (2013), 109–161.CrossRefGoogle Scholar
23Gazzola, F., Grunau, H. and Mitidieri, E.. Hardy inequalities with optimal constants and remainder terms. Trans. Amer. Math. Soc. 356 (2004), 2149–2168.CrossRefGoogle Scholar
24Ghoussoub, N. and Moradifam, A.. Bessel pairs and optimal Hardy and Hardy–Rellich inequalities. Math. Ann. 349 (2011), 1–57.CrossRefGoogle Scholar
25Gilbarg, D. and Trudinger, N.. Elliptic partial differential equations of second order. Grundlehren der Mathematischen Wissenschaften, vol. 224 (Berlin-New York: Springer-Verlag, 1977).CrossRefGoogle Scholar
26Greene, R. and Wu, W.. Function theory of manifolds which possess a pole. Lecture Notes in Math., vol.699 (Berlin: Springer, 1979).CrossRefGoogle Scholar
27Grigoryan, A.. Analytic and geometric background of recurrence and non-explosion of the Brownian motion on Riemannian manifolds. Bull. Amer. Math. Soc. 36 (1999), 135–249.CrossRefGoogle Scholar
28Grillo, G., Muratori, M. and Vázquez, J. L.. The porous medium equation on Riemannian manifolds with negative curvature. The large-time behaviour. Adv. Math. 314 (2017), 328–377.CrossRefGoogle Scholar
29Kombe, I. and Ozaydin, M.. Improved Hardy and Rellich inequalities on Riemannian manifolds. Trans. Amer. Math. Soc. 361 (2009), 6191–6203.CrossRefGoogle Scholar
30Kombe, I. and Ozaydin, M.. Hardy-Poincaré, Rellich and uncertainty principle inequalities on Riemannian manifolds. Trans. Amer. Math. Soc. 365 (2013), 5035–5050.CrossRefGoogle Scholar
31Kristaly, A.. Sharp uncertainty principles on Riemannian manifolds: the influence of curvature, J. Math. Pures Appl. 119 (2018), 326–346.CrossRefGoogle Scholar
32Lions, P.L.. The concentration-compactness principle in the calculus of variations. The limit case II. Rev. Mat. Iberoamericana 1 (1985a), 45–121.CrossRefGoogle Scholar
33Lions, P.L.. The concentration-compactness principle in the calculus of variations. The limit case I. Rev. Mat. Iberoamericana 1 (1985b), 145–201.CrossRefGoogle Scholar
34Montefusco, E.. Lower semicontinuity of functionals via the concentration-compactness principle. J. Math. Anal. Application, 263 (2001), 264–276.CrossRefGoogle Scholar
35Ngô, Q.-A. and Nguyen, V.H.. Sharp constant for Poincaré-type inequalities in the hyperbolic space. Acta Math. Vietnam (2018). https://doi.org/10.1007/s40306-018-0269-9.CrossRefGoogle Scholar
36Petersen, P.. Riemannian geometry. Graduate texts in Mathematics, vol. 171 (NY: Springer, 1998).CrossRefGoogle Scholar
37Pinchover, Y.. On positive solutions of second order elliptic equations, stability results and classification. Duke Math J. 57 (1988), 955–980.CrossRefGoogle Scholar
38Pinchover, Y.. A Liouville-type theorem for Schrödinger operators. Comm. Math. Phys. 272 (2007), 75–84.CrossRefGoogle Scholar
39Pinchover, Y. and Tintarev, K.. A ground state alternative for singular Schrödinger operators. J. Funct. Anal. 230 (2006), 65–77.CrossRefGoogle Scholar
40Yang, Q., Su, D and Kong, Y.. Hardy inequalities on Riemannian manifolds with negative curvature. Commun. Contemp. Math. 16 (2014), 1350043, 24 pp.CrossRefGoogle Scholar