Hostname: page-component-745bb68f8f-hvd4g Total loading time: 0 Render date: 2025-01-30T05:43:19.077Z Has data issue: false hasContentIssue false
  • English
  • Français

Periodic solutions of p-Laplacian differential equations with jumping nonlinearity across half-eigenvalues

Part of: Qualitative theory Boundary value problems

Published online by Cambridge University Press:  07 January 2022

Tengfei Shen  and
Wenbin Liu
Tengfei Shen
Affiliation:
School of Mathematics, China University of Mining and Technology, Xuzhou Jiangsu, 221116, PR China (stfcool@126.com, liuwenbin-xz@163.com)
Wenbin Liu
Affiliation:
School of Mathematics, China University of Mining and Technology, Xuzhou Jiangsu, 221116, PR China (stfcool@126.com, liuwenbin-xz@163.com)
Get access Rights & Permissions [Opens in a new window]

Abstract

This paper aims to investigate the existence of periodic solutions for $p$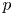-Laplacian differential equations with jumping nonlinearity under the frame of half-eigenvalue. Based on the continuity theorem, some new results are obtained, which enrich and generalize the previous results.

Keywords

Jumping nonlinearityTopological degreePeriodic solutionp-Laplacian equation

MSC classification

Primary: 34B15: Nonlinear boundary value problems 34C25: Periodic solutions
Type
Research Article
Information
Proceedings of the Royal Society of Edinburgh Section A: Mathematics , Volume 153 , Issue 1 , February 2023 , pp. 307 - 326
Check for updates
Copyright
Copyright © The Author(s), 2022. Published by Cambridge University Press on behalf of The Royal Society of Edinburgh

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Anane, A. and Dakkak, A.. Nonexistence of nontrivial solutions for an asymmetric problem with weights. Proyecciones Rev. De Matemática 19 (2000), 43–52.Google Scholar
Berestycki, H.. On some nonlinear Sturm-Liouville problems. J. Differ. Equ. 26 (1977), 375–390.CrossRefGoogle Scholar
Binding, P. and Rynne, B.. Oscillation and interlacing for various spectra of the $p$-Laplacian. Nonlinear Anal.: Theory, Methods Appl. 71 (2009), 2780–2791.CrossRefGoogle Scholar
Browne, J.. A Prüfer approach to half-linear Sturm-Liouville problems. Proc. Edinburgh Math. Soc. (Series 2) 41 (1998), 573–583.CrossRefGoogle Scholar
Chang, X. and Qiao, Y.. Existence of periodic solutions for a class of $p$-Laplacian equations. Boundary Value Prob. 2013 (2013), 96.CrossRefGoogle Scholar
Dancer, E. N.. Boundary-value problems for weakly nonlinear ordinary differential equations. Bull. Aust. Math. Soc. 15 (1976), 321–328.CrossRefGoogle Scholar
Del Pino, M., Manásevich, R. and Murúa, E.. Existence and multiplicity of solutions with prescribed period for a second order quasilinear ODE. Nonlinear Anal.: Theory, Methods Appl. 18 (1992), 79–92.CrossRefGoogle Scholar
Ding, T. and Zanolin, F.. Time-maps for the solvability of periodically perturbed nonlinear Duffing equations. Nonlinear Anal.: Theory, Methods Appl. 17 (1991), 635–653.CrossRefGoogle Scholar
Drábek, P. and Invernizzi, S.. On the periodic BVP for the forced Duffing equation with jumping nonlinearity. Nonlinear Anal.: Theory, Methods Appl. 10 (1986), 643–650.CrossRefGoogle Scholar
Fabry, C.. Landesman-Lazer conditions for periodic boundary value problems with asymmetric nonlinearities. J. Differ. Equ. 116 (1995), 405–418.CrossRefGoogle Scholar
Fučik, S.. Solvability of nonlinear equations and boundary value problems, Vol. 4 (Dordrecht: D. Reidel Publishing Company, 1980).Google Scholar
Genoud, F. and Rynne, B.. Landesman-Lazer conditions at half-eigenvalues of the $p$-Laplacian. J. Differ. Equ. 254 (2013), 3461–3475.CrossRefGoogle Scholar
Gossez, P. and Omari, P.. Nonresonance with respect to the Fučik spectrum for periodic solutions of second order ordinary differential equations. Nonlinear Anal.: Theory, Methods Appl. 14 (1990), 1079–1104.CrossRefGoogle Scholar
Jiang, M.. A Landesman-Lazer type theorem for periodic solutions of the resonant asymmetric $p$-Laplacian equation. Acta Math. Sinica 21 (2005), 1219–1228.CrossRefGoogle Scholar
Liu, B.. Boundedness of solutions for equations with $p$-Laplacian and an asymmetric nonlinear term. J. Differ. Equ. 207 (2004), 73–92.CrossRefGoogle Scholar
Liu, W. and Li, Y.. Existence of 2$\pi$-periodic solutions for the non-dissipative Duffing equation under asymptotic behaviors of potential function. Z. Angew. Math. Phys. 57 (2005), 1–11.CrossRefGoogle Scholar
Liu, W. and Li, Y.. Existence of periodic solutions for p-Laplacian equation under the frame of Fučik spectrum. Acta. Math. Sin. Engl. Ser. 27 (2011), 545–554.CrossRefGoogle Scholar
Li, W. and Yan, P.. Continuity and continuous differentiability of half-eigenvalues in potentials. Commun. Contemporary Math. 12 (2010), 977–996.CrossRefGoogle Scholar
Manásevich, R. and Mawhin, J.. Periodic solutions for nonlinear systems with $p$-Laplacian-like operators. J. Differ. Equ. 145 (1998), 367–393.CrossRefGoogle Scholar
Morris, A. and Robinson, B.. A Landesman-Lazer condition for the boundary-value problem $-u''= au^{+}-bu^{-}+ g(u)$ with periodic boundary conditions. Electron. J. Differ. Equ. Conf. 20 (2013), 103–117.Google Scholar
Nkashama, M. and Robinson, S.. Resonance and nonresonance in terms of average values. J. Differ. Equ. 132 (1996), 45–65.CrossRefGoogle Scholar
Rynne, B.. Landesman-Lazer conditions for resonant $p$-Laplacian problems with jumping nonlinearities. J. Differ. Equ. 261 (2016), 5829–5843.CrossRefGoogle Scholar
Rynne, B., Some recent results on periodic, jumping nonlinearity problems, in Spectral Theory and Nonlinear Analysis with Applications to Spatial Ecology, Universidad Complutense de Madrid (World Scientific, 2004).CrossRefGoogle Scholar
Rynne, B.. $p$-Laplacian problem with jumping nonlinearities. J. Differ. Equ. 226 (2006), 501–524.CrossRefGoogle Scholar
Rynne, B.. Non-resonance conditions for semilinear Sturm-Liouville problems with jumping non-linearities. J. Differ. Equ. 170 (2001), 215–227.10.1006/jdeq.2000.3817CrossRefGoogle Scholar
Yang, X.. Boundedness in nonlinear asymmetric oscillations. J. Differ. Equ. 183 (2002), 108–131.CrossRefGoogle Scholar
Zhang, M.. Nonresonance conditions for asymptotically positively homogeneous differential systems: the Fučik spectrum and its generalization. J. Differ. Equ. 145 (1998), 332–366.CrossRefGoogle Scholar