Published online by Cambridge University Press: 14 November 2011
We consider steady potential hydrodynamic-Poisson systems with a dissipation term (viscosity) proportional to a small parameter v in a two- or three-dimensional bounded domain. We show here that for any smooth solution of a boundary value problem which satisfies that the speed, denoted by |∇φv|, has an upper coarse bound  , uniform in the parameter v, then a sharper, correct uniform bound is obtained: the viscous speed |∇φv| is bounded pointwise, at points x0 in the interior of the flow domain, by cavitation speed (given by Bernoulli's Law at vacuum states) plus a term of
, uniform in the parameter v, then a sharper, correct uniform bound is obtained: the viscous speed |∇φv| is bounded pointwise, at points x0 in the interior of the flow domain, by cavitation speed (given by Bernoulli's Law at vacuum states) plus a term of 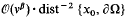 that depends on
that depends on  . The exponent is β = 1 for the standard isentropic gas flow model and β = 1/2 for the potential hydrodynamic Poisson system. Both cases are considered to have a γ-pressure law with 1<γ<2 in two space dimensions and 1 < γ< 3/2 in three space dimensions. These systems have cavitation speeds which take not necessarily constant values. In fact, for the potential hydrodynamic-Poisson systems, cavitation speed is a function that depends on the potential flow function and also on the electric potential.
. The exponent is β = 1 for the standard isentropic gas flow model and β = 1/2 for the potential hydrodynamic Poisson system. Both cases are considered to have a γ-pressure law with 1<γ<2 in two space dimensions and 1 < γ< 3/2 in three space dimensions. These systems have cavitation speeds which take not necessarily constant values. In fact, for the potential hydrodynamic-Poisson systems, cavitation speed is a function that depends on the potential flow function and also on the electric potential.
In addition, we consider a two-dimensional boundary value problem which has been proved to have a smooth solution whose speed is uniformly bounded. In this case, we show that the pointwise sharper bound can be extended to the section of the boundary ∂Ω\∂3Ω, where ∂3Ω is called the outflow boundary. The exponent β varies between 1 and 1/8 depending on the location of x0 at the boundary and on the curvature of the boundary at x0. In particular, our estimates apply to classical viscous approximation to transonic flow models.