Published online by Cambridge University Press: 14 November 2011
Ultradistributions of compact support are represented as the boundary values of Cauchy and Poisson integrals corresponding to tubular radial domains Tc' =ℝn + iC', C'⊂⊂C, where C is an open, connected, convex cone. The Cauchy integral of 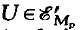 is shown to be an analytic function in TC' which satisfies a certain boundedness condition. Analytic functions which satisfy a specified growth condition in TC' have a distributional boundary value which can be used to determine an
is shown to be an analytic function in TC' which satisfies a certain boundedness condition. Analytic functions which satisfy a specified growth condition in TC' have a distributional boundary value which can be used to determine an 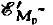 distribution.
distribution.