Hostname: page-component-6bb9c88b65-fsdjw Total loading time: 0 Render date: 2025-07-22T01:55:45.398Z Has data issue: false hasContentIssue false
  • English
  • Français

The equation utt − Δu = |u|p for the critical value of p

Published online by Cambridge University Press:  14 November 2011

Jack Schaeffer
Jack Schaeffer
Affiliation:
Department of Mathematics, Carnegie-Mellon University, Pittsburgh, PA 15213, U.S.A.
Get access Rights & Permissions [Opens in a new window]

Synopsis

The equation utt − Δu = |u|p is considered in two and three space dimensions. Smooth Cauchy data of compact support are given at t = 0. For the case of three space dimensions, John has shown that solutions with sufficiently small data exist globally in time if but that small data solutions blow up in finite time if Glassey has shown the two dimensional case is similar. This paper shows that small data solutions blow up in finite time when p is the critical value,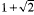 in three dimensions and in two.

Information

Type
Research Article
Information
Proceedings of the Royal Society of Edinburgh Section A: Mathematics , Volume 101 , Issue 1-2 , 1985 , pp. 31 - 44
Copyright
Copyright © Royal Society of Edinburgh 1985

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Article purchase

Temporarily unavailable

References

1Fujita, H.. On the blowing-up of solutions of the Cauchy problem for ut = Δu + u1+α J. Fac. Sci. Univ. Tokyo Sect. IA Math. 13 (1966), 109–124.Google Scholar
2Glassey, R.. Finite-time blow-up for solutions of nonlinear wave equations. Math. Z. 177 (1981), 323–340.CrossRefGoogle Scholar
3Glassey, R.. Existence in the large for u = F (u) in two space dimensions. Math. Z. 178 (1981), 233–261.CrossRefGoogle Scholar
4John, F.. Blow-up of solutions of nonlinear wave equations in three space dimensions. Manuscripta Math. 28 (1979), 235–268.CrossRefGoogle Scholar
5Sideris, T.. Nonexistence of global solutions to semilinear wave equations in high dimensions. Brown University L.C.D.S. Report #81–23 (October 1981).Google Scholar
6Weissler, F. B.. Existence and nonexistence of global solutions for a semilinear heat equation. Israel J. Math. 38 (1981), 29–40.CrossRefGoogle Scholar