Hostname: page-component-cd9895bd7-jkksz Total loading time: 0 Render date: 2024-12-27T05:08:05.291Z Has data issue: false hasContentIssue false
  • English
  • Français

Many synchronized vector solutions for a Bose–Einstein system

Part of: Elliptic equations and systems

Published online by Cambridge University Press:  13 January 2020

Wei Long ,
Zhongwei Tang  and
Sudan Yang
Wei Long
Affiliation:
College of Mathematics and Information Science, Jiangxi Normal University, Nanchang, Jiangxi 330022, P. R. China (lwhope@jxnu.edu.cn; 2353692672@qq.com)
Zhongwei Tang
Affiliation:
School of Mathematical Sciences, Beijing Normal University, Beijing100875, P. R. China (tangzw@bnu.edu.cn)
Sudan Yang
Affiliation:
College of Mathematics and Information Science, Jiangxi Normal University, Nanchang, Jiangxi 330022, P. R. China (lwhope@jxnu.edu.cn; 2353692672@qq.com)
Get access Rights & Permissions [Opens in a new window]

Abstract

This paper is concerned with the following nonlinear Schrödinger system in 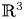${\mathbb R}^3$

$$\left\{ {\beging{matrix}{ {-\Delta u + (1 + \alpha P(x))u = \mu u^3 + \beta uv^2,} \hfill & {x\in {\open R}^3,} \hfill \cr {-\Delta v + (1 + \alpha Q(x))u = \nu v^3 + \beta u^2v,} \hfill & {x\in {\open R}^3,} \hfill \cr {u,v > 0,} \hfill & {x\in {\open R}^3,} \hfill \cr } } \right.$$
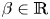where $\beta \in {\mathbb R}$ is a coupling constant, 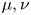$\mu ,\nu $ are positive constants, P,Q are weight functions decaying exponentially to zero at infinity, α can be regarded as a parameter. This type of system arises, in particular, in models in Bose–Einstein condensates theory and Kerr-like photo refractive media.

We prove that, for any positive integer k > 1, there exists a suitable range of α such that the above problem has a non-radial positive solution with exactly k maximum points which tend to infinity as 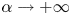$\alpha \to +\infty $ (or 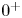$0^+$). Moreover, we also construct prescribed number of sign-changing solutions.

Keywords

multiple positive solutionsnonlinear Bose–Einstein system

MSC classification

Primary: 35J20: Variational methods for second-order elliptic equations
Secondary: 35J60: Nonlinear elliptic equations
Type
Research Article
Information
Proceedings of the Royal Society of Edinburgh Section A: Mathematics , Volume 150 , Issue 6 , December 2020 , pp. 3293 - 3320
Check for updates
Copyright
Copyright © The Author(s), 2020. Published by The Royal Society of Edinburgh

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

1Ambrosetti, A. and Colorado, E.. Bound and ground states of coupled nonlinear Schrödinger equations. C. R. Math. Acad. Sci. Paris 342 (2006), 453–458.CrossRefGoogle Scholar
2Ambrosetti, A. and Colorado, E.. Standing waves of some coupled nonlinear Schrödinger equations. J. London Math. Soc. 75 (2007), 67–82.CrossRefGoogle Scholar
3Ambrosetti, A., Colorado, E. and Ruiz, D.. Multi-bump solitons to linearly coupled systems of nonlinear Schrödinger equations. Calc. Var. Partial Differential Equations 30 (2007), 85–112.CrossRefGoogle Scholar
4Bahri, A. and Li, Y. Y.. On a min-max procedure for the existence of a positive solution for certain scalar field equations in ${\mathbb R}^N$. Rev. Mat. Iberoamericana 6 (1990), 1–15.CrossRefGoogle Scholar
5Bahri, A. and Lions, P. L.. On the existence of a positive solution of semilinear elliptic equations in unbounded domains. Ann. Inst. H. Poincaré Anal. Non Linéaire 14 (1997), 365–413.CrossRefGoogle Scholar
6Bartsch, T. and Wang, Z. Q.. Multiple positive solutions for a nonlinear schrödinger equation. Z. Angew. Math. Phsy. 51 (2000), 336–384.Google Scholar
7Bartsch, T. and Wang, Z.-Q.. Note on ground states of nonlinear Schrödinger systems. J. Partial Differential Equations 19 (2006), 200–207.Google Scholar
8Chang, S., Lin, C. S., Lin, T. C. and Lin, W.. Segregated nodal domains of two-dimensional multispecies Bose–Einstein condensates. Phys. D 196 (2004), 341–361.CrossRefGoogle Scholar
9Chen, Z. and Zou, W.. Standing waves for coupled nonlinear Schrödinger equations with decaying potentials. J. Math. Phys. 54 (2013), 111505.CrossRefGoogle Scholar
10Conti, M., Terracini, S. and Verzini, G.. Neharis problem and competing species systems. Ann. Inst. H. Poincaré Anal. Non Linéaire 19 (2002), 871–888.CrossRefGoogle Scholar
11Dancer, E. N., Wei, J. and Weth, T.. A priori bounds versus multiple existence of positive solutions for a nonlinear Schrödinger system. Ann. Inst. H. Poincaré Anal. Non Linéaire 27 (2010), 953–969.CrossRefGoogle Scholar
12D'Aprile, T. and Pistoia, A.. Existence, multiplicity and profile of sign-changing clustered solutions of a semiclassical nonlinear Schödinger equation. Ann. Inst. H. Poincaré Anal. Non Linéaire 26 (2009), 1423–1451.CrossRefGoogle Scholar
13Ding, W. Y. and Ni, W. M.. On the existence of positive entire solutions of a semilinear elliptic equation. Arch. Ration. Mech. Anal. 91 (1986), 283–308.CrossRefGoogle Scholar
14Esry, B. D., Greene, C. H., Burke, J. P. and Bohn, J. L.. Hartree–Fock theory for double condensates. Phys. Rev. Lett. 78 (1997), 3594–3597.CrossRefGoogle Scholar
15de Figueiredo, D. G. and Lopes, O.. Solitary waves for some nonlinear Schrödinger systems. Ann. Inst. H. Poincaré Anal. Non Linéaire 25 (2008), 149–161.Google Scholar
16Guo, Y., Luo, S. and Zou, W.. The existence, uniqueness and nonexistence of the ground state to the N-coupled Schrödinger systems in ${\mathbb R}^n(n\leq 4)$.Google Scholar
17Ikoma, N. and Tanaka, K.. A local mountain pass type result for a system of nonlinear Schrödinger equations. Calc. Var. Partial Differential Equations 40 (2011), 449–480.CrossRefGoogle Scholar
18Kang, X. and Wei, J.. On interacting bumps of semi-classical states of nonlinear Schrödinger equations. Adv. Differential Equations 5 (2000), 899–928.Google Scholar
19Lin, T. and Wei, J.. Ground state of N coupled nonlinear Schrödinger equations in ${\mathbb R}^n, n\geq 3$. Comm. Math. Phys. 255 (2005), 629–653.CrossRefGoogle Scholar
20Lin, T. and Wei, J.. Spikes in two coupled nonlinear Schrödinger equations. Ann. Inst. H. Poincaré Anal. Non Linéaire 22 (2005), 403–439.CrossRefGoogle Scholar
21Lin, F., Ni, W. M. and Wei, J.. On the number of interior peak solutions for a singularly perturbed Neumann problem. Comm. Pure Appl. Math. 60 (2007), 252–281.CrossRefGoogle Scholar
22Lin, L., Liu, Z. and Chen, S.. Multi-bump solutions for a semilinear Schrödinger equation. Indiana Univ. Math. J. 58 (2009), 1659–1689.CrossRefGoogle Scholar
23Lions, P. L.. The concentration-compactness principle in the calculus of variations. The locally compact case. I. Ann. Inst. H. Poincaré Anal. Non Linéaire 1 (1984), 109–145.CrossRefGoogle Scholar
24Lions, P. L.. The concentration-compactness principle in the calculus of variations. The locally compact case. II. Ann. Inst. H. Poincaré Anal. Non Linéaire 1 (1984), 223–283.CrossRefGoogle Scholar
25Liu, Z. and Wang, Z-Q.. Multiple bound states of nonlinear Schrödinger systems. Commun. Math. Phys. 282 (2008), 721–731.CrossRefGoogle Scholar
26Long, W. and Peng, S.. Multiple positive or sign-changing solutions for a type of nonlinear Schrödinger equation. Ann. Sc. Norm. Super. Pisa Cl. Sci. (5) 16 (2016), 603–623.Google Scholar
27Lucia, M. and Tang, Z.. Multi-bump bound states for a system of nonlinear Schrödinger equations. J. Differential Equations 252 (2012), 3630–3657.CrossRefGoogle Scholar
28Lucia, M. and Tang, Z.. Multi-bump bound states for a Schrödinger system via Lyapunov–Schmidt reduction. NoDEA Nonlinear Differential Equations Appl. 24 (2017), Art. 65, 22 pp.CrossRefGoogle Scholar
29Mitchell, M. and Segev, M.. Self-trapping of incoherent white light. Nature 387 (1997), 880–882.CrossRefGoogle Scholar
30Noris, B., Tavares, H., Terracini, S. and Verzini, G.. Uniform Hölder bounds for nonlinear Schrödinger systems with strong competition. Comm. Pure Appl. Math. 63 (2010), 267–302.Google Scholar
31Peng, S. and Wang, Z.-Q.. Segregated and synchronized vector solutions for nonlinear Schrödinger systems. Arch. Ration. Mech. Anal. 208 (2013), 305–339.CrossRefGoogle Scholar
32Rüegg, Ch., Cavadini, N., Furrer, A., Güdel, H.-U., Krämer, K., Mutka, H., Wildes, A., Habicht, K. and Vorderwisch, P.. Bose–Einstein condensation of the triple states in the magnetic insulator TlCuCl3. Nature 423 (2003), 62–65.CrossRefGoogle Scholar
33Sirakov, B.. Least energy solitary waves for a system of nonlinear Schrödinger equations in ${\mathbb R}^n$. Comm. Math. Phys. 271 (2007), 199–221.CrossRefGoogle Scholar
34Terracini, S. and Verzini, G.. Multipulse phase in k-mixtures of Bose–Einstein condenstates. Arch. Rat. Mech. Anal. 194 (2009), 717–741.CrossRefGoogle Scholar
35Timmermans, E.. Phase separation of Bose–Einstein condensates. Phys. Rev. Lett. 81 (1998), 5718–5721.CrossRefGoogle Scholar
36Wei, J. and Weth, T.. Nonradial symmetric bound states for a system of two coupled Schrödinger equations. Rend. Lincei Mat. Appl. 18 (2007), 279–293.Google Scholar
37Wei, J. and Weth, T.. Radial solutions and phase separation in a system of two coupled Schrödinger equations. Arch. Rat. Mech. Anal. 190 (2008), 83–106.CrossRefGoogle Scholar
38Wei, J. and Yan, S.. New solutions for nonlinear Schrödinger equations with critical nonlinearity. J. Differential Equations 237 (2007), 446–472.CrossRefGoogle Scholar
39Wei, J. and Yan, S.. Infinite many positive solutions for the nonlinear Schrödinger equation in ${\mathbb R}^n$. Calc. Var. Partial Differential Equations 37 (2010), 423–439.CrossRefGoogle Scholar