Published online by Cambridge University Press: 27 December 2018
In this paper, we study under what boundary conditions the inequality
holds true. It is known that such an estimate holds if either the tangential or normal component of ω vanishes on the boundary ∂Ω. We show that the vanishing tangential component condition is a special case of a more general one. In two dimensions, we give an interpolation result between these two classical boundary conditions.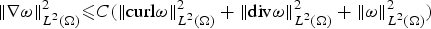 $${\rm \Vert }\nabla \omega {\rm \Vert }_{L^2(\Omega )}^2 \les C({\rm \Vert }{\rm curl}\omega {\rm \Vert }_{L^2(\Omega )}^2 + {\rm \Vert }{\rm div}\omega {\rm \Vert }_{L^2(\Omega )}^2 + {\rm \Vert }\omega {\rm \Vert }_{L^2(\Omega )}^2 )$$
$${\rm \Vert }\nabla \omega {\rm \Vert }_{L^2(\Omega )}^2 \les C({\rm \Vert }{\rm curl}\omega {\rm \Vert }_{L^2(\Omega )}^2 + {\rm \Vert }{\rm div}\omega {\rm \Vert }_{L^2(\Omega )}^2 + {\rm \Vert }\omega {\rm \Vert }_{L^2(\Omega )}^2 )$$