Sharp decay estimates in a bioconvection model with quadratic degradation in bounded domains
Published online by Cambridge University Press: 25 April 2018
Abstract
The paper studies large time behaviour of solutions to the Keller–Segel system with quadratic degradation in a liquid environment, as given by
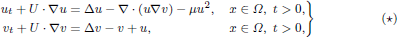
under Neumann boundary conditions in a bounded domain Ω ⊂ ℝn, where n ≥ 1 is arbitrary. It is shown that whenever U : Ω × (0,∞) → ℝn is a bounded and sufficiently regular solenoidal vector field any non-trivial global bounded solution of (⋆) approaches the trivial equilibrium at a rate that, with respect to the norm in either of the spaces L1(Ω) and L∞(Ω), can be controlled from above and below by appropriate multiples of 1/(t + 1). This underlines that, even up to this quantitative level of accuracy, the large time behaviour in (⋆) is essentially independent not only of the particular fluid flow, but also of any effect originating from chemotactic cross-diffusion. The latter is in contrast to the corresponding Cauchy problem, for which known results show that in the n = 2 case the presence of chemotaxis can significantly enhance biomixing by reducing the respective spatial L1 norms of solutions.
Information
- Type
- Research Article
- Information
- Proceedings of the Royal Society of Edinburgh Section A: Mathematics , Volume 148 , Issue 5 , October 2018 , pp. 939 - 955
- Copyright
- Copyright © Royal Society of Edinburgh 2018
- 12
- Cited by

