No CrossRef data available.
Article contents
GENERALIZING BOOLOS’ THEOREM
Published online by Cambridge University Press: 17 October 2016
Abstract
It’s well known that it’s possible to extract, from Frege’s Grudgesetze, an interpretation of second-order Peano Arithmetic in the theory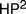 
$H{P^2}$, whose sole axiom is Hume’s principle. What’s less well known is that, in Die Grundlagen Der Arithmetic §82–83 Boolos (2011), George Boolos provided a converse interpretation of 
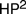$H{P^2}$ in 
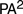$P{A^2}$. Boolos’ interpretation can be used to show that the Frege’s construction allows for any model of 
$P{A^2}$ to be recovered from some model of 
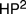$H{P^2}$. So the space of possible arithmetical universes is precisely characterized by Hume’s principle.
In this paper, I show that a large class of second-order theories admit characterization by an abstraction principle in this sense. The proof makes use of structural abstraction principles, a class of abstraction principles of considerable intrinsic interest, and categories of interpretations in the sense of Visser (2003).
- Type
- Research Article
- Information
- Copyright
- Copyright © Association for Symbolic Logic 2016
References
BIBLIOGRAPHY
Boolos, G. S. (2011). Die Grundlagen der Arithmetik §82–83. In Burgess, J. P., editor. Logic, Logic, and Logic. Cambridge: Harvard University Press, pp. 315–333.Google Scholar
Craig, W. & Vaught, R. L. (1958). Finite axiomatizability using additional predicates. The Journal of Symbolic Logic, 23(3), 289–308.Google Scholar
Väänänen, J. (2012). Second order logic or set theory?
The Bulletin of Symbolic Logic, 18(1), 91–121.Google Scholar
Visser, A. (2006). Logic in Tehran. In Enyat, A., Kalantari, I., and Moniri, M., editors. Proceedings of the Workshop and Conference on Logic, Algebra and Arithmetic. La Jolla, CA: Association of Symbolic Logic, pp. 284–341.Google Scholar
Walsh, S., Cook, R. T., & Visser, A. (2013). ESSLLI Notes on Abstraction Principles. Unpublished, available on request.Google Scholar