Article contents
A MATHEMATICAL COMMITMENT WITHOUT COMPUTATIONAL STRENGTH
Published online by Cambridge University Press: 02 July 2021
Abstract
We present a new manifestation of Gödel’s second incompleteness theorem and discuss its foundational significance, in particular with respect to Hilbert’s program. Specifically, we consider a proper extension of Peano arithmetic (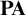 $\mathbf {PA}$) by a mathematically meaningful axiom scheme that consists of
$\mathbf {PA}$) by a mathematically meaningful axiom scheme that consists of 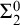 $\Sigma ^0_2$-sentences. These sentences assert that each computably enumerable (
$\Sigma ^0_2$-sentences. These sentences assert that each computably enumerable (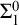 $\Sigma ^0_1$-definable without parameters) property of finite binary trees has a finite basis. Since this fact entails the existence of polynomial time algorithms, it is relevant for computer science. On a technical level, our axiom scheme is a variant of an independence result due to Harvey Friedman. At the same time, the meta-mathematical properties of our axiom scheme distinguish it from most known independence results: Due to its logical complexity, our axiom scheme does not add computational strength. The only known method to establish its independence relies on Gödel’s second incompleteness theorem. In contrast, Gödel’s theorem is not needed for typical examples of
$\Sigma ^0_1$-definable without parameters) property of finite binary trees has a finite basis. Since this fact entails the existence of polynomial time algorithms, it is relevant for computer science. On a technical level, our axiom scheme is a variant of an independence result due to Harvey Friedman. At the same time, the meta-mathematical properties of our axiom scheme distinguish it from most known independence results: Due to its logical complexity, our axiom scheme does not add computational strength. The only known method to establish its independence relies on Gödel’s second incompleteness theorem. In contrast, Gödel’s theorem is not needed for typical examples of 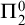 $\Pi ^0_2$-independence (such as the Paris–Harrington principle), since computational strength provides an extensional invariant on the level of
$\Pi ^0_2$-independence (such as the Paris–Harrington principle), since computational strength provides an extensional invariant on the level of 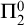 $\Pi ^0_2$-sentences.
$\Pi ^0_2$-sentences.
Keywords
Information
- Type
- Research Article
- Information
- Copyright
- © The Author(s), 2021. Published by Cambridge University Press on behalf of The Association for Symbolic Logic
References
BIBLIOGRAPHY
- 1
- Cited by


