Hostname: page-component-78c5997874-4rdpn Total loading time: 0 Render date: 2024-11-13T05:32:12.979Z Has data issue: false hasContentIssue false
  • English
  • Français

THE MODAL LOGIC OF SET-THEORETIC POTENTIALISM AND THE POTENTIALIST MAXIMALITY PRINCIPLES

Part of: General logic Model theory

Published online by Cambridge University Press:  04 October 2019

JOEL DAVID HAMKINS  and
ØYSTEIN LINNEBO
JOEL DAVID HAMKINS
Affiliation:
FACULTY OF PHILOSOPHY UNIVERSITY OF OXFORDOXFORD, UK and UNIVERSITY COLLEGEOXFORD, UKE-mail: jhamkins@gc.cuny.eduURL: http://jdh.hamkins.org
ØYSTEIN LINNEBO
Affiliation:
DEPARTMENT OF PHILOSOPHY, IFIKK UNIVERSITY OF OSLO, POSTBOKS 1020 BLINDERN 0315OSLO, NORWAYE-mail: oystein.linnebo@ifikk.uio.noURL: http://oysteinlinnebo.org
Get access Rights & Permissions [Opens in a new window]

Abstract

We analyze the precise modal commitments of several natural varieties of set-theoretic potentialism, using tools we develop for a general model-theoretic account of potentialism, building on those of Hamkins, Leibman and Löwe [14], including the use of buttons, switches, dials and ratchets. Among the potentialist conceptions we consider are: rank potentialism (true in all larger 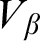$V_\beta $ ), Grothendieck–Zermelo potentialism (true in all larger 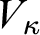$V_\kappa $ for inaccessible cardinals 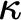$\kappa $ ), transitive-set potentialism (true in all larger transitive sets), forcing potentialism (true in all forcing extensions), countable-transitive-model potentialism (true in all larger countable transitive models of ZFC), countable-model potentialism (true in all larger countable models of ZFC), and others. In each case, we identify lower bounds for the modal validities, which are generally either S4.2 or S4.3, and an upper bound of S5, proving in each case that these bounds are optimal. The validity of S5 in a world is a potentialist maximality principle, an interesting set-theoretic principle of its own. The results can be viewed as providing an analysis of the modal commitments of the various set-theoretic multiverse conceptions corresponding to each potentialist account.

Keywords

potentialismmodal logicmodels of set theory

MSC classification

Secondary: 03C62: Models of arithmetic and set theory 03B45: Modal logic (including the logic of norms)
Type
Research Article
Information
The Review of Symbolic Logic , Volume 15 , Issue 1 , March 2022 , pp. 1 - 35
Check for updates
Copyright
© Association for Symbolic Logic, 2022

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

BIBLIOGRAPHY

Boolos, G. (1993). The Logic of Provability. Cambridge: Cambridge University Press.Google Scholar
Ewald, W. (1996). From Kant to Hilbert: A Source Book in the Foundations of Mathematics , Vol. 2. Oxford: Oxford University Press.Google Scholar
Fuchs, G., Hamkins, J. D., and Reitz, J. (2015). Set-theoretic geology. Annals of Pure and Applied Logic, 166(4), 464–501. Available from: http://jdh.hamkins.org/set-theoreticgeology.CrossRefGoogle Scholar
Garson, J. W. (2001). Quantification in modal logic. In Gabbay, D. and Guenthner, F., editors. Handbook of Philosophical Logic, Vol. 3. Dordrecht: Kluwer Academic Publishers, pp. 267–323.CrossRefGoogle Scholar
Gitman, V. and Hamkins, J. D. (2010). A natural model of the multiverse axioms. Notre Dame Journal of Formal Logic, 51(4), 475–484. Available from: http://wp.me/p5M0LV-3I.CrossRefGoogle Scholar
Hamkins, J. D. (2003). A simple maximality principle. Journal of Symbolic Logic, 68(2), 527–550. Available from: http://wp.me/p5M0LV-2v.CrossRefGoogle Scholar
Hamkins, J. D. (2011). The set-theoretic multiverse: A natural context for set theory. Annals of the Japan Association for Philosophy of Science, 19, 37–55. Available from: http://jdh.hamkins.org/themultiverseanaturalcontext.CrossRefGoogle Scholar
Hamkins, J. D. (2012). The set-theoretic multiverse. Review of Symbolic Logic, 5, 416–449. Available from http://jdh.hamkins.org/themultiverse.CrossRefGoogle Scholar
Hamkins, J. D. (2013). Every countable model of set theory embeds into its own constructible universe. Journal of Mathematical Logic, 13(2), 1350006. Available from: http://wp.me/p5M0LV-jn.CrossRefGoogle Scholar
Hamkins, J. D. (2014). A multiverse perspective on the axiom of constructibility. In C. Chong et al., editors. Infinity and Truth, Vol. 25. Hackensack: World Scientific Publishing, pp. 25–45. Available from: http://wp.me/p5M0LV-qE.CrossRefGoogle Scholar
Hamkins, J. D. (2014). Local properties in set theory. Mathematics and Philosophy of the Infinite. Available from: http://jdh.hamkins.org/local-properties-in-set-theory/.Google Scholar
Hamkins, J. D. (2016). Upward closure and amalgamation in the generic multiverse of a countable model of set theory. RIMS Kyôkyûroku, 17–31. Available from: http://wp.me/p5M0LV-1cv.Google Scholar
Hamkins, J. D. (2018). The modal logic of arithmetic potentialism and the universal algorithm. Preprint, arXiv:1801.04599. Available from: http://wp.me/p5M0LV-1zz.Google Scholar
Hamkins, J. D., Leibman, G., and Löwe, B. (2015). Structural connections between a forcing class and its modal logic. Israel Journal of Mathematics, 207(2), 617–651. Available from: http://wp.me/p5M0LV-kf.CrossRefGoogle Scholar
Hamkins, J. D. and Löwe, B. (2008). The modal logic of forcing. Transaction of the American Mathematical Society, 360(4), 1793–1817. Available from: http://wp.me/p5M0LV-3h.CrossRefGoogle Scholar
Hamkins, J. D. and Löwe, B. (2013). Moving up and down in the generic multiverse. In Lodaya, K., editor. Logic and Its Applications, ICLA 2013, Lecture Notes in Computer Science, Vol. 7750. Berlin–Heidelberg: Springer, pp. 139–147. Available from: http://wp.me/p5M0LV-od.Google Scholar
Hamkins, J. D. and Woodin, W. H. (2018). The universal finite set. Preprint, arXiv:1711.07952. Available from: http://jdh.hamkins.org/the-universal-finite-set.Google Scholar
Hellman, G. (1989). Mathematics Without Numbers. Oxford: Clarendon.Google Scholar
Lear, J. (1977). Sets and semantics. Journal of Philosophy, 74(2), 86–102.CrossRefGoogle Scholar
Linnebo, Ø. (2013). The potential hierarchy of sets. Review of Symbolic Logic, 6(2), 205–228.CrossRefGoogle Scholar
Linnebo, Ø. and Shapiro, S. (2019). Actual and potential infinity. Noûs, 53(1), 160–191.CrossRefGoogle Scholar
Parsons, C. (1983). Sets and modality. In Mathematics in Philosophy. Cornell: Cornell University Press, pp. 298–341.Google Scholar
Piribauer, J. (2017). The Modal Logic of Generic Multiverses. Master’s Thesis, Universiteit van Amsterdam.Google Scholar
Putnam, H. (1967). Mathematics without foundations. Journal of Philosophy, LXIV(1), 5–22.CrossRefGoogle Scholar
Studd, J. (2013). The iterative conception of set: A (bi-)modal axiomatisation. Journal of Philosophical Logic, 42(5), 697–725.CrossRefGoogle Scholar
Tait, W. W. (1998). Zermelo’s conception of set theory and reflection principles. In Schirn, M., editor. The Philosophy of Mathematics Today. Oxford: Clarendon Press.Google Scholar
Usuba, T. (2018). The downward directed grounds hypothesis and very large cardinals. Preprint, Journal of Mathematical Logic, 17(2), 1750009.CrossRefGoogle Scholar
Zermelo, E. (1930). Über Grenzzahlen und Mengenbereiche. Fundamenta Mathematicae, 16, 29–47, Translated in [2].CrossRefGoogle Scholar