Hostname: page-component-78c5997874-mlc7c Total loading time: 0 Render date: 2024-11-11T02:15:13.112Z Has data issue: false hasContentIssue false
  • English
  • Français

WHAT IS A RULE OF INFERENCE?

Part of: Proof theory and constructive mathematics General logic

Published online by Cambridge University Press:  21 December 2020

NEIL TENNANT
NEIL TENNANT*
Affiliation:
DEPARTMENT OF PHILOSOPHY THE OHIO STATE UNIVERSITYCOLUMBUS, OH43210, USAE-mail: tennant9@osu.edu
Get access Rights & Permissions [Opens in a new window]

Abstract

We explore the problems that confront any attempt to explain or explicate exactly what a primitive logical rule of inference is, or consists in. We arrive at a proposed solution that places a surprisingly heavy load on the prospect of being able to understand and deal with specifications of rules that are essentially self-referring. That is, any rule $\rho $ is to be understood via a specification that involves, embedded within it, reference to rule 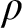$\rho $ itself. Just how we arrive at this position is explained by reference to familiar rules as well as less familiar ones with unusual features. An inquiry of this kind is surprisingly absent from the foundations of inferentialism—the view that meanings of expressions (especially logical ones) are to be characterized by the rules of inference that govern them.

Keywords

Classical LogicIntuitionistic LogicMinimal LogicCore Logicproof systemsrules of inferenceself-referenceimpredicative definitionsinductive definition of proofHorn clausesModus Ponensdischarge of assumptionsparallelized elimination rulesclassical rules of negation

MSC classification

Primary: 03F03: Proof theory, general
Secondary: 03B47: Substructural logics (including relevance, entailment, linear logic, Lambek calculus, BCK and BCI logics) 03B20: Subsystems of classical logic (including intuitionistic logic)
Type
Research Article
Information
The Review of Symbolic Logic , Volume 14 , Issue 2 , June 2021 , pp. 307 - 346
Check for updates
Copyright
© Association for Symbolic Logic, 2020

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

BIBLIOGRAPHY

Anderson, A. R. & Belnap, N. D. Jnr. (1975). Entailment: The Logic of Relevance and Necessity. Vol. 1. Princeton and London: Princeton University Press.Google Scholar
Belnap, N. (1962). Tonk, Plonk and Plink. Analysis, 22, 130–134.CrossRefGoogle Scholar
Boghossian, P. (2014). What is inference? Philosophical Studies, 1690(1), 1–18.CrossRefGoogle Scholar
Brauer, E. & Tennant, N. (2020). Transmission of verification. The Review of Symbolic Logic, 1–23. http://dx.doi.org/10.1017/S1755020320000234.CrossRefGoogle Scholar
Cook, R. T. & Cogburn, J. (2000). What negation is not: Intuitionism and ‘0 = 1’. Analysis, 600(1), 5–12.CrossRefGoogle Scholar
Dean, J. & Kurokawa, H. (2016). Kreisel’s theory of constructions, the Kreisel-Goodman paradox, and the second clause. In Piecha, T. and Schroeder-Heister, P., editors. Advances in Proof-Theoretic Semantics. Berlin: Springer, pp. 27–63.CrossRefGoogle Scholar
Dummett, M. (1973). The justification of deduction. Proceedings of the British Academy, LIX, 201–232.Google Scholar
Dummett, M. (1991). The Logical Basis of Metaphysics. Cambridge, MA: Harvard University Press.Google Scholar
Dummett, M. (2000). Elements of Intuitionism (second edition). Oxford, UK: Clarendon Press.Google Scholar
Gentzen, G. (1936). Die Widerspruchsfreiheit der reinen Zahlentheorie. Mathematische Annalen, 112(1), 493–565.CrossRefGoogle Scholar
Gentzen, G. (1934, 1935). Untersuchungen über das logische Schliessen I. Math. Zeitschrift, 39(176–210), 405–431. Translated as ‘Investigations into logical deduction’. In Szabo, M. E., editor. The Collected Papers of Gerhard Gentzen. North-Holland, Amsterdam, pp. 68–131.CrossRefGoogle Scholar
Glivenko, V. (1929). Sur quelques points de la logique de M. Brouwer. Bull. Soc. Math. Belg., 15(5), 183–188.Google Scholar
Gödel, K. (1930). Die Vollständigkeit der Axiome des logischen Funktionenkalküls. Monatshefte für Mathematik und Physik, 37(1), 349–360.CrossRefGoogle Scholar
Gödel, K. (1933). Zur intuitionistischen Arithmetik und Zahlentheorie. Ergebnisse eines mathematischen Kolloquiums, 4(1933), 34–38.Google Scholar
Hilbert, D. & Ackermann, W. (1938). Grundzüge der Theoretischen Logik, 2. Auflage. Berlin: Springer.CrossRefGoogle Scholar
Hilbert, D. & Ackermann, W. (1950). Principles of Mathematical Logic. New York, NY: Chelsea Publishing Co.Google Scholar
Jaśkowski, S. (1934). On the rules of suppositions in formal logic. Studia Logica, 10(1), 5–32.Google Scholar
Jeffrey, R. C. (1967). Formal Logic: Its Scope and Limits. New York, NY: McGraw-Hill, Inc.Google Scholar
Kahle, R. & Schroeder-Heister, P. (2006). Introduction: Proof-theoretic semantics. Synthese (Special issue: Kahle R., and Schroeder-Heister, P., editors. Proof-Theoretic Semantics), 148(3), 503–506.CrossRefGoogle Scholar
Kreisel, G. (1962). Foundations of intuitionistic logic. In Nagel, E., Suppes, P., and Tarski, A., editors. Logic, Methodology and Philosophy of Science. Stanford: Stanford University Press, pp. 198–210.Google Scholar
Martin-Löf, P. (1994). Analytic and synthetic judgments in type theory. In Parrini, P., editor. Kant and Contemporary Epistemology. Dordrecht: Kluwer Academic Publishers, pp. 87–99.CrossRefGoogle Scholar
Martin-Löf, P. (1996). On the meanings of the logical constants and the justifications of the logical laws. Nordic Journal of Philosophical Logic, 10(1), 11–60.Google Scholar
Prawitz, D. (1965). Natural Deduction: A Proof-Theoretical Study. Stockholm: Almqvist & Wiksell.Google Scholar
Prawitz, D. (1971). Ideas and results in proof theory. In Fenstad, J. E., editor. Proceedings of the Second Scandinavian Logic Symposium. Amsterdam: North-Holland, pp. 235–307.CrossRefGoogle Scholar
Prawitz, D. (1974). On the idea of a general proof theory. Synthese, 27(0) 63–77.CrossRefGoogle Scholar
Prawitz, D. (1987). Dummett on a theory of meaning and its impact on logic. In Taylor, B., editor. Michael Dummett: Contributions to Philosophy. Nijhoff International Philosophy Series, Vol. 25. Dordrecht: Martinus Nijhoff Publishers, pp. 117–165.CrossRefGoogle Scholar
Prawitz, D. (1998). Comments on the papers. Theoria, 640(2–3), 283–337.Google Scholar
Prawitz, D. (2019). The seeming interdependence between the concepts of valid inference and proof. Topoi, 38(3), 493–503.CrossRefGoogle Scholar
Schroeder-Heister, P. (1984). A natural extension of natural deduction. Journal of Symbolic Logic, 49(4), 1284–1300.CrossRefGoogle Scholar
Schroeder-Heister, P. (1991). Uniform proof-theoretic semantics for logical constants. Journal of Symbolic Logic, 56(0), 1142.Google Scholar
Schroeder-Heister, P. (2006). Validity concepts in proof-theoretic semantics. Synthese (Special issue: Kahle R., and Schroeder-Heister, P., editors. Proof-Theoretic Semantics), 148(3), 525–571.CrossRefGoogle Scholar
Schroeder-Heister, P. (2018). Proof-theoretic semantics. In Zalta, E. N., editor. The Stanford Encyclopedia of Philosophy (spring 2018 edition). Metaphysics Research Lab, Stanford University.Google Scholar
Schroeder-Heister, P. & Piecha, T., editors. (2016) Advances in Proof-Theoretic Semantics. Berlin: Springer.Google Scholar
Scott, D. (1974). Rules and derived rules. In Stenlund, S., editor. Logical Theory and Semantic Analysis. Dordrecht: Reidel, pp. 147–161.CrossRefGoogle Scholar
Shoesmith, D. J. & Smiley, T. J. (1978). Multiple-Conclusion Logic. Cambridge and New York: Cambridge University Press.CrossRefGoogle Scholar
Smiley, T. (1962). The independence of connectives. The Journal of Symbolic Logic, 27(4), 426–436.CrossRefGoogle Scholar
Tennant, N. (1978). Natural Logic. Edinburgh: Edinburgh University Press.Google Scholar
Tennant, N. (1992). Autologic. Edinburgh: Edinburgh University Press.Google Scholar
Tennant, N. (1996). The law of excluded middle is synthetic a priori, if valid. Philosophical Topics, 24(1), 205–229.CrossRefGoogle Scholar
Tennant, N. (1999). Negation, absurdity and contrariety. In Gabbay, D. and Wansing, H., editors. What is Negation? Dordrecht: Kluwer, pp. 199–222.CrossRefGoogle Scholar
Tennant, N. (2010). Inferential semantics. In Lear, J. and Oliver, A., editors, The Force of Argument: Essays in Honor of Timothy Smiley. London: Routledge, pp. 223–257.Google Scholar
Tennant, N. (2012). Cut for core logic. Review of Symbolic Logic, 50(3), 450–479.CrossRefGoogle Scholar
Tennant, N. (2015a). Cut for classical core logic. Review of Symbolic Logic, 80(2), 236–256.CrossRefGoogle Scholar
Tennant, N. (2015b). The relevance of premises to conclusions of core proofs. Review of Symbolic Logic, 80(4), 743–784.CrossRefGoogle Scholar
Tennant, N. (2017). Core Logic. Oxford: Oxford University Press.CrossRefGoogle Scholar
Tennant, N. (2018). Logical theory of truthmakers and falsitymakers. In Glanzberg, M., editor. Handbook on Truth. Oxford, UK: Oxford University Press, pp. 355–393.Google Scholar
Troelstra, A. S. and Schwichtenberg, H. (2000). Basic Proof Theory (second edition). Cambridge, UK: Cambridge University Press.CrossRefGoogle Scholar
van Atten, M. (2018). Predicativity and parametric polymorphism of Brouwerian implication. Available from https://arxiv.org/pdf/1710.07704.pdf. p. 41.Google Scholar
Weinstein, S. (1993). The intended interpretation of intuitionistic logic. Journal of Philosophical Logic, 120(2), 261–270.Google Scholar
Wright, C. (2014). Comment on Paul Boghossian, “What is inference”. Philosophical Studies, 169(1), 27–37.CrossRefGoogle Scholar