Hostname: page-component-cd9895bd7-jn8rn Total loading time: 0 Render date: 2024-12-26T03:38:28.589Z Has data issue: false hasContentIssue false
  • English
  • Français

LOGICS ABOVE S4 AND THE LEBESGUE MEASURE ALGEBRA

Published online by Cambridge University Press:  28 October 2016

TAMAR LANDO
TAMAR LANDO*
Affiliation:
Department of Philosophy, Columbia University
*
*DEPARTMENT OF PHILOSOPHY COLUMBIA UNIVERSITY 708 PHILOSOPHY HALL 1150 AMSTERDAM AVENUE MAIL CODE: 4971 NEW YORK, NY 10027, USA E-mail: tal2108@columbia.edu
Get access Rights & Permissions [Opens in a new window]

Abstract

We study the measure semantics for propositional modal logics, in which formulas are interpreted in the Lebesgue measure algebra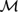${\cal M}$, or algebra of Borel subsets of the real interval [0,1] modulo sets of measure zero. It was shown in Lando (2012) and Fernández-Duque (2010) that the propositional modal logic S4 is complete for the Lebesgue measure algebra. The main result of the present paper is that every logic L aboveS4 is complete for some subalgebra of 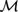${\cal M}$. Indeed, there is a single model over a subalgebra of 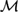${\cal M}$ in which all nontheorems of L are refuted. This work builds on recent work by Bezhanishvili, Gabelaia, & Lucero-Bryan (2015) on the topological semantics for logics above S4. In Bezhanishvili et al., (2015), it is shown that there are logics above that are not the logic of any subalgebra of the interior algebra over the real line, 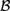${\cal B}$(ℝ), but that every logic above is the logic of some subalgebra of the interior algebra over the rationals, 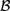${\cal B}$(ℚ), and the interior algebra over Cantor space, 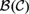${\cal B}\left( {\cal C} \right)$.

Type
Research Article
Information
The Review of Symbolic Logic , Volume 10 , Issue 1 , March 2017 , pp. 51 - 64
Copyright
Copyright © Association for Symbolic Logic 2016 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

BIBLIOGRAPHY

Aiello, M., van Benthem, J., & Bezhanishvili, G. (2003). Reasoning about space the modal way. Journal of Logic and Computation, 13(6), 889–920.CrossRefGoogle Scholar
Bezhanishvili, G., & Gabelaia, D. (2011). Connected modal logics. Archive for Mathematical Logic, 50, 287–317.CrossRefGoogle Scholar
Bezhanishvili, G., Gabelaia, D., & Lucero-Bryan, J. (2015). Topological completeness of logics above s4. Journal of Symbolic Logic, 80(2), 520–566.CrossRefGoogle Scholar
Blackburn, P., de Rijke, M., & Venema, Y. (2001). Modal Logic. Cambridge Tracts in Theoretical Computer Science, vol. 53. Cambridge: Cambridge University Press.CrossRefGoogle Scholar
Blok, W. J. (1976). Varieties of Interior Algebras. Ph.D. Thesis, University of Amsterdam.Google Scholar
Blok, W. J., & Dwinger, P. H. (1975). Equational classes of closure algebras. Archive for Mathematical Logic, 37, 189–198.Google Scholar
Chagrov, A., & Zakharyaschev, M. (1997). Modal Logic. Oxford Logic Guides, vol. 35. Oxford: Oxford University Press.CrossRefGoogle Scholar
Fernández-Duque, D. (2010). Absolute completeness of s4u for its measure-theoretic semantics. Advances in Modal Logic, 8, 100–119.Google Scholar
Givant, S., & Halmos, P. (2009). Introduction to Boolean Algebras. New York: Springer-Verlag.Google Scholar
Lando, T. (2012). Completeness of s4 for the Lebesgue measure algebra. Journal of Philosophical Logic, 41(2), 287–316.CrossRefGoogle Scholar
Lando, T. (2015). First order s4 and its measure-theoretic semantics. Annals of Pure and Applied Logic, 166(2), 187–218.CrossRefGoogle Scholar
McKinsey, J. C. C., & Tarski, A. (1944). The algebra of topology. Annals of Mathematics, 45(1), 141–191.CrossRefGoogle Scholar
Rasiowa, H., & Sikorski, R. (1963). The Mathematics of Metamathematics. Warsaw: Państwowe Wydawnictwo Naukowe.Google Scholar
Royden, H. L., & Fitzpatrick, P. M. (2010). Real Analysis (fourth edition). New Jersey: Prentice Hall.Google Scholar
Scott, D. (2009) Mixing Modality and Probability. Lecture Notes, UC Berkeley, Logic Colloquium, September.Google Scholar