No CrossRef data available.
Article contents
The Theory of Selection: its History and Development
Published online by Cambridge University Press: 07 November 2014
Synopsis of paper
The Paper is a review of the theory of selection as developed by British actuaries.
The fundamental ideas underlying the theory were present in the minds of actuaries from a very early date. Apparently the earliest allusion to the subject was that of Joshua Milne in his treatise on Annuities and Assurances; but its importance was clearly recognised by Arthur Morgan in his Introduction to the Experience of the Equitable Society, and by Thomas Galloway in the Preface to the Experience of the Amicable Society.
- Type
- Research Article
- Information
- Copyright
- Copyright © Institute and Faculty of Actuaries 1926
References
page 44 note * Of 21,398 lives included in this experience, 9324 or just under 44 percent. discontinued their policies. A. H. Bailey said (J.I.A. xix., 408):–‘When 'life insurance was first practised in this country, in the early days of the 'Royal Exchange and the London Assurance, from 1720 for several years 'onward, the whole of the policies were term policies. In the early days 'of the Equitable, the great bulk of the policies were the same; and 'formerly the premiums quoted in prospectuses generally, were premiums 'for one and seven years and the whole term of life, and no others.’. This no doubt accounts in large measure for the high rate of withdrawal which characterised several of these old experiences.
page 45 note * Tables of Mortality deduced from the Experience of the Amicable Society, by Thomas Galloway, Registrar of the Society. Published 1841.
page 45 note † Life Contingency Tables, Part I. : The Ohances of Premature Death and the Value of Selection among Assured Lives. E. J. Farren, London, 1850.
page 55 note * Simultaneously and independently G. W. Berridge prepared a table of annuity values, which vary with the duration of assurance. J.I.A. xix., 351.
page 60 note * It was intended to examine some of the more important American experiences of assured lives, but the discussion has already run to a considerable length. It has therefore been restricted to a review of the theory as put forward by British actuaries. Reference may, however, be made to Mr. Chatham's paper quoted above, and to the diagrams appended to that paper.
page 64 note * It has been pointed out to the writer that the doctrine of Spurious Selection seems to have been anticipated by T. B. Sprague (J.I.A. xxxii., 199) in replying to a letter by Mr. T. B. Macaulay (J.I.A. xxxii., 117) on ‘Maximum Mortality Percentages’. Sprague said : ‘On treating the ‘Annuitant experience [i.e. the Government Annuitants experience 1882] ‘in the same way as I treated the experience of assured lives in 1870, I ‘found a very similar law prevailed in the results; that is to say, taking ‘quinquennial groups of ages attained, I found that, in almost all of these ‘groups, as the time elapst since the date of purchase continually increast, ‘the ratio of the actual to the expected claims, first inoreast to a maximum, ‘and then diminisht. In this case, the law cannot be a result of withdrawals, ‘for there are none ; and some other explanation of the law must ‘be sought for. The only possible explanation seems to be a peculiar ‘composition of the body of lives on which annuities are purchast. Suppose, ‘for example, that the nominees of any age on whose lives annuities ‘are purchast, comprise (1) a majority, who are subject to a low rate of ‘mortality at first, gradually increasing in the course of, say, 10 years, to ‘the normal rate corresponding to the age; and (2) a minority, ‘who are also subject to a low rate of mortality at first, but, after the lapse of a few ‘years, are subject to such heavy rates of mortality that they are all dead ‘within, say, 25 years from the date of purchase; then, confining our ‘attention to nominees of a given age at entry, it is clear that during the ‘10 years after purchase, the ratio of the actual to the expected deaths, as ‘calculated according to the normal rate of mortality, will continually ‘increase : that, after the lapse of the 10 years, it will still increase, in ‘consequence of the heavy mortality among the minority lives, and will for ‘several years exceed unity; but, that, after the lapse of the 25 years, ‘when all the minority lives are dead, the ratio will become equal to unity ; ‘in other words, the ratio will attain a maximum and then diminish’. Sprague was here measuring the operation of Spurious Selection by means of reference to a standard table ; but the modern doctrine seems to be clearly though briefly stated. It appears that the statistical process, which produces the effect of spurious selection, is illustrated in the example given by Mr. Macaulay in the letter above referred to (J.I.A. xxxii., 121). There it is shown that the effect of amalgamating several series of percentages (or rates), each of which increases continuously, is to produce a series which first increases and then decreases, and the result seems to be due simply to the varying proportions in which the higher percentages (or rates) are represented in the mixture.
page 66 note * In this connection referenoe may be made to Mr. G. W. Richmond's paper on The Mortality Experience of the Gotha Life Office, 1829–1896 (T.F.A. v.,96). There he gives a table showing the percentages of Actual Deaths in each of the four successive periods, in which the experience had been analysed, to the deaths expected on the basis of the mortality experience of the whole period 1829–1896. If we represent the mortality of the earliest period by 100, the comparative measures of the mortality of the three following periods are as follows :–
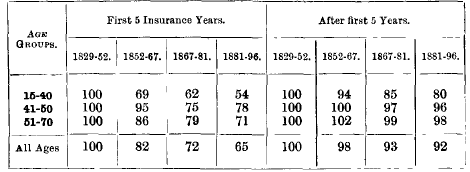
He states that Dr. Karup attributed the rapid fall in the mortality values during the early years of duration to the keener selection of new risks rather than to the general decline in the death-rate, and it seems difficult to explain the figures in any other way. For the ‘after 5 years’ experience the comparatively small decrease, especially in the later years, seems at first sight diffioult to explain; for population data show that Germanmortality has been improving over a long period in a way not dissimilar from that in this country. If, however, the effects of selection persist for more than five years, the ‘ultimate’ exposed to risk in the earliest period would probably include a considerable proportion of lives who had recently passed out of the ‘select’ period, and who might be regarded as partially select lives. As the Office became older, this proportion would gradually decrease with a resulting increase in the average rate, and this would tend to obscure the effect due to general improvement in vitality.
page 80 note * These expectations are added for purposes of comparison. They relate to exact ages, whereas the unadjusted expectations based on the new data are for ages last birthday. Allowance for this was, however, made in the calculation of the expected deaths in the comparison following.
page 97 note * J.I.A., Iv., p.l.
page 99 note * Is the lighter mortality of the general population at age 60 (say) in 1925 compared with 1890 due only to “1925”? May it not be that 1865 was a “healthier” year to be born in than 1830, that 1875 to 1885 were healthier years for school and adolescence than 1840 to 1850, and that 1885 to 1915 were healthier years for manhood or for the bearing of children than 1850 to 1880?
page 99 note † Thus (5) was not assumed to be non-existent by our exterpolation ; but the change due to it was assumed to be allowed for, automatically, by the use of (4).
page 102 note * Cf. G. F. Hardy, J.I.A., xxiii., pp. 2–3 (1881) ; Lectures, pp. 17–18.
page 103 note * T.F.A., xi., 13 and 14.


