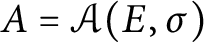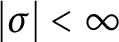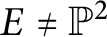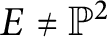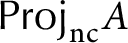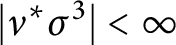Quantum projective planes finite over their centers

Abstract

For a three-dimensional quantum polynomial algebra $A=\mathcal {A}(E,\sigma )$ , Artin, Tate, and Van den Bergh showed that A is finite over its center if and only if $|\sigma |<\infty $ . Moreover, Artin showed that if A is finite over its center and $E\neq \mathbb P^{2}$ , then A has a fat point module, which plays an important role in noncommutative algebraic geometry; however, the converse is not true in general. In this paper, we will show that if $E\neq \mathbb P^{2}$ , then A has a fat point module if and only if the quantum projective plane ${\sf Proj}_{\text {nc}} A$ is finite over its center in the sense of this paper if and only if $|\nu ^{*}\sigma ^{3}|<\infty $ where $\nu $ is the Nakayama automorphism of A. In particular, we will show that if the second Hessian of E is zero, then A has no fat point module.

1 Introduction

A quantum polynomial algebra is a noncommutative analogue of a commutative polynomial algebra, and a quantum projective space is the noncommutative projective scheme associated to a quantum polynomial algebra, so they are the most basic objects to study in noncommutative algebraic geometry. In fact, the starting point of the subject noncommutative algebraic geometry is the paper [3] by Artin, Tate, and Van den Bergh, showing that there exists a nice correspondence between three-dimensional quantum polynomial algebras A and geometric pairs $(E, \sigma )$ where $E=\mathbb P^{2}$ or a cubic divisor in $\mathbb P^{2}$ , and $\sigma \in \text {Aut} E$ , so the classification of three-dimensional quantum polynomial algebras reduces to the classification of “regular” geometric pairs. Write $A=\mathcal A(E, \sigma )$ for a three-dimensional quantum polynomial algebra corresponding to the geometric pair $(E, \sigma )$ . The geometric properties of the geometric pair $(E, \sigma )$ provide some algebraic properties of $A=\mathcal A(E, \sigma )$ . One of the most striking results of such is in the companion paper [4].

Theorem 1.1 [4, Theorem 7.1]

Let $A=\mathcal A(E, \sigma )$ be a three-dimensional quantum polynomial algebra. Then $|\sigma |<\infty $ if and only if A is finite over its center.

Let $A=\mathcal A(E, \sigma )$ be a three-dimensional quantum polynomial algebra. To prove the above theorem, fat points of the quantum projective plane ${\sf Proj}_{\text {nc}} A$ play an essential role. By Artin [2], if A is finite over its center and $E\neq \mathbb {P}^{2}$ , then ${\sf Proj}_{\text {nc}} A$ has a fat point; however, the converse is not true. To check the existence of a fat point, there is a more important notion than $|\sigma |$ , namely,

$$ \begin{align*}\|\sigma \|:=\text{inf}\{i\in \mathbb{N}^{+} \mid \sigma^{i}=\phi|_{E} \text{ for some } \phi\in \text{Aut}\mathbb{P}^{2}\}.\end{align*} $$

In fact, ${\sf Proj}_{\text {nc}} A$ has a fat point if and only if $1<\|\sigma \|<\infty $ by [2].

In [13], the notion that ${\sf Proj}_{\text {nc}} A$ is finite over its center was introduced, and the following result was proved.

Theorem 1.2 [13, Theorem 4.17]

Let $A=\mathcal A(E, \sigma )$ be a three-dimensional quantum polynomial algebra such that $E\subset \mathbb P^{2}$ is a triangle. Then $\|\sigma \|<\infty $ if and only if ${\sf Proj}_{\text {nc}} A$ is finite over its center.

The purpose of this paper is to extend the above theorem to all three-dimensional quantum polynomial algebras. In fact, the following is our main result.

Theorem 1.3 (Theorem 3.6 and Corollary 4.1)

Let $A=\mathcal A(E, \sigma )$ be a three-dimensional quantum polynomial algebra such that $E\neq \mathbb P^{2}$ , and $\nu \in \text {Aut} A$ the Nakayama automorphism of A. Then the following are equivalent:

1. (1) $|\nu ^{*}\sigma ^{3}|<\infty $ .

2. (2) $\|\sigma \|<\infty $ .

3. (3) ${\sf Proj}_{\text {nc}} A$ is finite over its center.

4. (4) ${\sf Proj}_{\text {nc}} A$ has a fat point.

Note that if $E=\mathbb P^{2}$ , then $||\sigma ||=1$ , but $\text {Proj}_{\text {nc}}A$ has no fat point (see Lemma 2.14).

As a biproduct, we have the following corollary.

Corollary 1.4 Let $A=\mathcal A(E, \sigma )$ be a three-dimensional quantum polynomial algebra. If the second Hessian of E is zero, then A is never finite over its center.

These results are important to study representation theory of the Beilinson algebra $\nabla A$ , which is a typical example of a $2$ -representation infinite algebra defined in [6]. This was the original motivation of the paper [13].

2 Preliminaries

Throughout this paper, we fix an algebraically closed field k of characteristic $0$ . All algebras and (noncommutative) schemes are defined over k. We further assume that all (graded) algebras are finitely generated (in degree $1$ ) over k, that is, algebras of the form $k\langle x_{1}, \dots , x_{n}\rangle /I$ for some (homogeneous) ideal $I\lhd k\langle x_{1}, \dots , x_{n}\rangle $ (where $\deg x_{i}=1$ for every $i=1, \dots , n$ ).

2.1 Geometric quantum polynomial algebras

In this subsection, we define geometric algebras and quantum polynomial algebras.

Definition 2.1 [12, Definition 4.3]

A geometric pair $(E,\sigma )$ consists of a projective scheme $E \subset \mathbb P^{n-1}$ and $\sigma \in \text {Aut}_{k}\,E$ . For a quadratic algebra $A=k\langle x_{1}, \dots , x_{n}\rangle /I$ where $I\lhd k\langle x_{1}, \dots , x_{n}\rangle $ is a homogeneous ideal generated by elements of degree $2$ , we define

$$ \begin{align*} \mathcal{V}(I_{2}):=\{ (p,q)\in\mathbb{P}^{n-1}\times \mathbb P^{n-1} \,|\,f(p,q)=0\,\,\text{for any } f \in I_{2} \}. \end{align*} $$

1. (1) We say that A satisfies (G $1$ ) if there exists a geometric pair $(E,\sigma )$ such that

   $$ \begin{align*} \mathcal{V}(I_{2})=\{ (p,\sigma(p)) \in \mathbb{P}^{n-1}\times \mathbb P^{n-1} \,|\,p \in E \}. \end{align*} $$
   In this case, we write $\mathcal {P}(A)=(E,\sigma )$ , and call E the point scheme of A.

2. (2) We say that A satisfies (G $2$ ) if there exists a geometric pair $(E,\sigma )$ such that

   $$ \begin{align*} I_{2}=\{ f \in k\langle x_{1}, \dots, x_{n}\rangle_{2} \,|\,f(p,\sigma(p))=0\,\,\text{for any } p \in E \}. \end{align*} $$
   In this case, we write $A=\mathcal {A}(E,\sigma )$ .

3. (3) A quadratic algebra A is called geometric if A satisfies both (G1) and (G2) with $A=\mathcal {A}(\mathcal {P}(A))$ .

Definition 2.2 A right Noetherian graded algebra A is called a d-dimensional quantum polynomial algebra if

1. (1) $\operatorname {gldim} A=d$ ,

2. (2) $\text {Ext}^{i}_{A}(k, A)\cong \begin {cases} k & \text { if } i=d, \\ 0 & \text { if } i\neq d, \end {cases}$ and

3. (3) $H_{A}(t):=\sum _{i=0}^{\infty }(\dim _{k}A_{i})t^{i}=(1-t)^{-d}$ .

Note that a three-dimensional quantum polynomial algebra is exactly the same as a three-dimensional quadratic AS-regular algebra, so we have the following result.

Theorem 2.1 [3]

Every three-dimensional quantum polynomial algebra is a geometric algebra where the point scheme is either $\mathbb P^{2}$ or a cubic divisor in $\mathbb P^{2}$ .

Remark 2.2 There exists a four-dimensional quantum polynomial algebra which is not a geometric algebra; however, as far as we know, there exists no example of a quantum polynomial algebra which does not satisfy (G1).

We define the type of a three-dimensional quantum polynomial algebra $A=\mathcal A(E, \sigma )$ in terms of the point scheme $E\subset \mathbb P^{2}$ .

1. Type P E is $\mathbb {P}^{2}$ .

2. Type S E is a triangle.

3. Type S’ E is a union of a line and a conic meeting at two points.

4. Type T E is a union of three lines meeting at one point.

5. Type T’ E is a union of a line and a conic meeting at one point.

6. Type NC E is a nodal cubic curve.

7. Type CC E is a cuspidal cubic curve.

8. Type TL E is a triple line.

9. Type WL E is a union of a double line and a line.

10. Type EC E is an elliptic curve.

2.2 Quantum projective spaces finite over their centers

Definition 2.3 A noncommutative scheme (over k) is a pair $X=({\sf mod} X, \mathcal O_{X})$ consisting of a k-linear abelian category ${\sf mod} X$ and an object $\mathcal O_{X}\in {\sf mod} X$ . We say that two noncommutative schemes $X=(\textsf{mod} X, \mathcal O_{X})$ and $Y=(\textsf{mod} Y, \mathcal O_{Y})$ are isomorphic, denoted by $X\cong Y$ , if there exists an equivalence functor $F:{\sf mod} X\to {\sf mod} Y$ such that $F(\mathcal O_{X})\cong \mathcal O_{Y}$ .

If X is a commutative Noetherian scheme, then we view X as a noncommutative scheme by $({\sf mod} X, \mathcal O_{X})$ where ${\sf mod} X$ is the category of coherent sheaves on X and $\mathcal O_{X}$ is the structure sheaf on X.

Noncommutative affine and projective schemes are defined in [5].

Definition 2.4 If R is a right Noetherian algebra, then we define the noncommutative affine scheme associated to R by ${\sf Spec}_{\text {nc}} R=({\sf mod} R, R)$ where ${\sf mod} R$ is the category of finitely generated right R-modules and $R\in {\sf mod} R$ is the regular right module.

Note that if R is commutative, then ${\sf Spec}_{\text {nc}} R\cong {\sf Spec} R$ .

Definition 2.5 If A is a right Noetherian graded algebra, ${\sf grmod} A$ is the category of finitely generated graded right A-modules, and ${\sf tors} A$ is the full subcategory of ${\sf grmod} A$ consisting of finite-dimensional modules over k, then we define the noncommutative projective scheme associated to A by ${\sf Proj}_{\text {nc}} A=({\sf tails} A, \pi A)$ where ${\sf tails} A:={\sf grmod} A/{\sf tors} A$ is the quotient category, $\pi :{\sf grmod} A\to {\sf tails} A$ is the quotient functor, and $A\in {\sf grmod} A$ is the regular graded right module. If A is a d-dimensional quantum polynomial algebra, then we call ${\sf Proj}_{\text {nc}} A$ a quantum $\mathbb P^{d-1}$ . In particular, if $d=3$ , then we call ${\sf Proj}_{\text {nc}} A$ a quantum projective plane.

Note that if A is commutative, then ${\sf Proj}_{\text {nc}} A\cong {\sf Proj} A$ . It is known that if A is a two-dimensional quantum polynomial algebra, then ${\sf Proj}_{\text {nc}} A\cong \mathbb P^{1}$ .

For a three-dimensional quantum polynomial algebra $A=\mathcal A(E, \sigma )$ , we have the following geometric characterization when A is finite over its center.

Theorem 2.3 [4, Theorem 7.1]

Let $A=\mathcal {A}(E,\sigma )$ be a three-dimensional quantum polynomial algebra. Then the following are equivalent:

1. (1) $|\sigma |<\infty $ .

2. (2) A is finite over its center.

Since the property that A is finite over its center is not preserved under isomorphisms of noncommutative projective schemes ${\sf Proj}_{\text {nc}} A$ , we will make the following rather ad hoc definition.

Definition 2.6 Let A be a d-dimensional quantum polynomial algebra. We say that ${\sf Proj}_{\text {nc}}A$ is finite over its center if there exists a d-dimensional quantum polynomial algebra $A^{\prime }$ finite over its center such that ${\sf Proj}_{\text {nc}} A\cong {\sf Proj}_{\text {nc}} A^{\prime }$ .

For a three-dimensional quantum polynomial algebra, the above definition coincides with [13, Definition 4.14] by the following result.

Lemma 2.4 [1, Corollary A.10]

Let A and $A^{\prime }$ be three-dimensional quantum polynomial algebras. Then ${\sf grmod} A\cong {\sf grmod} A^{\prime }$ if and only if ${\sf Proj}_{\text {nc}} A\cong {\sf Proj}_{\text {nc}} A^{\prime }$ .

To characterize “geometric” quantum projective spaces finite over their centers, we will introduce the following notion.

Definition 2.7 [13, Definition 4.6]

For a geometric pair $(E, \sigma )$ where $E\subset \mathbb {P}^{n-1}$ and $\sigma \in \text {Aut}_{k}E$ , we define

$$ \begin{align*}\text{Aut}_{k}(\mathbb P^{n-1}, E):=\{\phi|_{E}\in \text{Aut}_{k} E\mid \phi\in \text{Aut}_{k} \mathbb P^{n-1}\}, \text{ and} \end{align*} $$

$$ \begin{align*} \|\sigma \|:=\text{inf}\{i\in \mathbb{N}^{+} \mid \sigma^{i}\in \text{Aut}_{k} (\mathbb P^{n-1}, E)\}. \end{align*} $$

For a geometric pair $(E, \sigma )$ , clearly $\|\sigma \| \leq |\sigma |$ . The following are the basic properties of $\|\sigma \|$ .

Lemma 2.5 [13, Lemma 4.16(1)], [14, Lemma 2.5]

Let A and $A^{\prime }$ be d-dimensional quantum polynomial algebras satisfying (G1) with $\mathcal {P}(A)=(E,\sigma )$ and $\mathcal {P}(A^{\prime })=(E^{\prime },\sigma ^{\prime })$ .

1. (1) If $A\cong A^{\prime }$ , then $E\cong E^{\prime }$ and $|\sigma |=|\sigma ^{\prime }|$ .

2. (2) If $\mathsf{grmod}\,A\cong \mathsf{grmod}\,A^{\prime }$ , then $E\cong E^{\prime }$ and $||\sigma ||=||\sigma ^{\prime }||$ .

In particular, if A and $A^{\prime }$ are three-dimensional quantum polynomial algebras such that ${\sf Proj}_{\text {nc}} A\cong {\sf Proj}_{\text {nc}} A^{\prime }$ , then $E\cong E^{\prime }$ (that is, A and $A^{\prime }$ are of the same type) and $||\sigma ||=||\sigma ^{\prime }||$ .

For a three-dimensional quantum polynomial algebra $A=\mathcal A(E, \sigma )$ of Type S, we have the following geometric characterization when a quantum projective plane ${\sf Proj}_{\text {nc}}A$ is finite over its center.

Theorem 2.6 [13, Theorem 4.17]

Let $A=\mathcal {A}(E,\sigma )$ be a three-dimensional quantum polynomial algebra of Type S. Then the following are equivalent:

1. (1) $\|\sigma \|<\infty $ .

2. (2) ${\sf Proj}_{\text {nc}}A$ is finite over its center.

The purpose of this paper is to extend the above theorem to all types.

2.3 Points of a noncommutative scheme

Definition 2.8 Let R be an algebra. A point of ${\sf Spec}_{\text {nc}} R$ is an isomorphism class of a simple right R-module $M\in {\sf mod} R$ such that $\dim _{k}M<\infty $ . A point M is called fat if $\dim _{k}M>1$ .

Remark 2.7 If R is a commutative algebra and $p\in {\sf Spec} A$ is a closed point, then $A/\mathfrak{m}_{p}\in {\sf mod} R$ is a point where $\mathfrak{m}_{p}$ is the maximal ideal of R corresponding to p. In fact, this gives a bijection between the set of closed points of ${\sf Spec} R$ and the set of points of ${\sf Spec}_{\text {nc}} R$ . In this commutative case, there exists no fat point.

Remark 2.8 Fat points are not preserved under Morita equivalences. For example, ${\sf mod} k\cong {\sf mod} M_{2}(k)$ , but it is easy to see that ${\sf Spec}_{\text {nc}} k$ has no fat point while ${\sf Spec}_{\text {nc}} M_{2}(k)$ has a fat point. However, since ${\sf Spec}_{\text {nc}} R\cong {\sf Spec}_{\text {nc}} R^{\prime }$ if and only if $R\cong R^{\prime }$ , fat points are preserved under isomorphisms of ${\sf Spec}_{\text {nc}} R$ .

Example 2.9 If $R=k\langle u, v\rangle /(uv-vu-1)$ is the first Weyl algebra, then it is well known that there exists no finite-dimensional right R-module, so ${\sf Spec}_{\text {nc}} R$ has no point at all.

Example 2.10 (cf. [15])

If $R=k\langle u, v\rangle /(vu-uv-u)$ is the enveloping algebra of a two-dimensional nonabelian Lie algebra, then the set of points of ${\sf Spec}_{\text {nc}} R$ is given by $\{R/uR+(v-\mu )R\}_{\mu \in k}$ , so ${\sf Spec}_{\text {nc}} R$ has no fat point. In fact, the linear map $\delta :k[u]\to k[u]$ defined by $\delta (f(u))=uf^{\prime }(u)$ is a derivation of $k[u]$ such that $R=k[u][v; \delta ]$ is the Ore extension, so that $vf(u)=f(u)v+uf^{\prime }(u)$ . If M is a finite-dimensional right R-module, then there exists $f(u)=a_{d}u^{d}+\cdots +a_{1}u+a_{0}\in k[u]\subset R$ of the minimal degree $\deg f(u)=d\geq 1$ such that $Mf(u)=0$ . Since $uf^{\prime }(u)=vf(u)-f(u)v$ , $M(df(u)-uf^{\prime }(u))=0$ such that $\deg (df(u)-uf^{\prime }(u))<\deg f(u)$ , $df(u)=uf^{\prime }(u)$ by minimality of $\deg f(u)=d\geq 1$ , but this is possible only if $f(u)=a_{1}u$ , so $Mu=0$ . It follows that M can be viewed as an $R/(u)$ -module, a point of ${\sf Spec}_{\text {nc}} (R/(u))\cong {\sf Spec}_{\text {nc}} k[v]$ , so $M\cong R/uR+(v-\mu )R$ for some $\mu \in k$ . Since ${\sf Spec}_{\text {nc}} (R/(u))\cong {\sf Spec}_{\text {nc}} k[v]$ is a commutative scheme, ${\sf Spec}_{\text {nc}} R$ has no fat point.

Example 2.11 [13, Lemma 4.19]

If $R=k\langle u, v\rangle /(uv+vu)$ is a two-dimensional (ungraded) quantum polynomial algebra, then the set of points of ${\sf Spec}_{\text {nc}} R$ is given by

$$ \begin{align*} \{R/(u-\lambda)R+vR\}_{\lambda\in k}&\cup\{R/uR+(v-\mu)R\}_{\mu\in k}\\ &\cup\{R/(x^{2}-\lambda)R+(\sqrt{\mu}x+\sqrt{-\lambda}y)R+(y^{2}-\mu)R\}_{0\neq \lambda, \, \mu\in k}. \end{align*} $$

Among them, $\{R/(x^{2}-\lambda )R+(\sqrt {\mu }x+\sqrt {-\lambda }y)R+(y^{2}-\mu )R\}_{0\neq \lambda , \, \mu \in k}$ is the set of fat points of ${\sf Spec}_{\text {nc}} R$ .

Definition 2.9 Let A be a graded algebra. A point of ${\sf Proj}_{\text {nc}} A$ is an isomorphism class of a simple object of the form $\pi M\in {\sf tails} A$ where $M\in {\sf grmod} A$ is a graded right A-module such that $\lim _{i\to \infty }\dim _{k}M_{i}<\infty $ . A point $\pi M$ is called fat if $\lim _{i\to \infty } \dim _{k}M_{i}>1$ , and, in this case, M is called a fat point module over A.

Remark 2.12 If A is a graded commutative algebra and $p\in {\sf Proj} A$ is a closed point, then $\pi (A/\mathfrak m_{p})\in {\sf tails} A$ is a point where $\mathfrak m_{p}$ is the homogeneous maximal ideal of A corresponding to p. In fact, this gives a bijection between the set of closed points of ${\sf Proj} A$ and the set of points of ${\sf Proj}_{\text {nc}} A$ . In this commutative case, there exists no fat point.

Remark 2.13 It is unclear that fat points are preserved under isomorphisms of ${\sf Proj}_{\text {nc}} A$ in general. However, fat point modules are preserved under graded Morita equivalences, so if A and $A^{\prime }$ are both three-dimensional quantum polynomial algebras such that ${\sf Proj}_{\text {nc}} A\cong {\sf Proj}_{\text {nc}} A^{\prime }$ , then there exists a natural bijection between the set of fat points of ${\sf Proj}_{\text {nc}} A$ and that of ${\sf Proj}_{\text {nc}} A^{\prime }$ by Lemma 2.4.

The following facts will be used to prove our main results.

Lemma 2.14 [2, 13]

Let $A=\mathcal A(E, \sigma )$ be a three-dimensional quantum polynomial algebra.

1. (1) $\|\sigma \|=1$ if and only if $E=\mathbb {P}^{2}$ .

2. (2) $1<\|\sigma \|<\infty $ if and only if ${\sf Proj}_{\text {nc}} A$ has a fat point.

Theorem 2.15 [13, Theorem 4.20]

If A is a quantum polynomial algebra and $x\in A$ is a homogeneous normal element of positive degree, then there exists a bijection between the set of points of ${\sf Proj}_{\text {nc}} A$ and the disjoint union of the set of points of ${\sf Proj}_{\text {nc}} A/(x)$ and the set of points of ${\sf Spec}_{\text {nc}} A[x^{-1}]_{0}$ . In this bijection, fat points correspond to fat points.

3 Main results

In this section, we will state and prove our main results.

Let A be a graded algebra and $\nu \in \text {Aut} A$ a graded algebra automorphism. For a graded A–A-bimodule M, we define a new graded A–A bimodule $M_{\nu }=M$ as a graded vector space with the new actions $a*m*b:=am\nu (b)$ for $a, b\in A, m\in M$ . Let A be a d-dimensional quantum polynomial algebra. The canonical module of A is defined by $\omega _{A}:=\lim _{i\to \infty }\text {Ext}^{d}_{A}(A/A_{\geq i}, A)$ , which has a natural graded A–A bimodule structure. It is known that there exists $\nu \in \text {Aut} A$ such that $\omega _{A}\cong A_{\nu ^{-1}}(-d)$ as graded A–A bimodules. We call $\nu $ the Nakayama automorphism of A. Since $A_{0}=k$ , the Nakayama automorphism $\nu $ is uniquely determined by A. Among quantum polynomial algebras, Calabi–Yau quantum polynomial algebras defined below are easier to handle.

Definition 3.1 A quantum polynomial algebra A is called Calabi–Yau if the Nakayama automorphism of A is the identity.

The following theorem plays an essential role to prove our main results, claiming that every quantum projective plane has a three-dimensional Calabi–Yau quantum polynomial algebra as a homogeneous coordinate ring.

Theorem 3.1 [8, Theorem 4.4]

For every three-dimensional quantum polynomial algebra A, there exists a three-dimensional Calabi–Yau quantum polynomial algebra $A^{\prime }$ such that ${\sf grmod} A\cong {\sf grmod} A^{\prime }$ , so that ${\sf Proj}_{\text {nc}} A\cong {\sf Proj}_{\text {nc}} A^{\prime }$ .

By the above theorem, the proofs of our main results reduce to the Calabi–Yau case.

3.1 Calabi–Yau case

Let $E=\mathcal V(x^{3}+y^{3}+z^{3}-\lambda xyz)\subset \mathbb P^{2}, \; \lambda \in k, \lambda ^{3}\neq 27$ be an elliptic curve in the Hesse form. We fix a group structure with the identity element $o:=(1,-1,0)\in E$ , and write $E[n]:=\{p\in E\mid np=o\}$ the set of n-torsion points. We also denote by $\sigma _{p}\in \text {Aut}_{k} E$ the translation automorphism by a point $p\in E$ . It is known that $\sigma _{p}\in \text {Aut}_{k}(\mathbb P^{2}, E)$ if and only if $p\in E[3]$ (cf. [12, Lemma 5.3]).

Lemma 3.2 Denote a three-dimensional Calabi–Yau quantum polynomial algebra as

$$ \begin{align*}A=k\langle x,y,z \rangle/(f_{1}, f_{2}, f_{3})=\mathcal{A}(E,\sigma).\end{align*} $$

Then Table 1 gives a list of defining relations $f_{1}, f_{2}, f_{3}$ and the corresponding geometric pairs $(E,\sigma )$ for such algebras up to isomorphism. In Table 1, we remark that:

1. (1) Type S and Type T are further divided into Type S $_{1}$ and Type S $_{3}$ , and Type T $_{1}$ and Type T $_{3}$ , respectively, in terms of the form of $\sigma $ .

2. (2) The point scheme E may consist of several irreducible components, and, in this case, $\sigma $ is described on each component.

3. (3) For Type NC and Type CC, $\sigma $ in Table 1 is defined except for the unique singular point $(0, 0, 1)\in E$ , which is preserved by $\sigma $ .

4. (4) For Type TL and Type WL, E is nonreduced, and the description of $\sigma $ is omitted.

Proof The list of the defining relations $f_{1}, f_{2}, f_{3}$ is given in [7, Theorem 3.3] and [9, Corollary 4.3]. It is not difficult to calculate their corresponding geometric pairs $(E, \sigma )$ using the condition (G1) (see, for example, [16, proof of Theorem 3.1] for Type P, S $_{1}$ , S $_{3}$ , S’, and [14, proof of Theorem 3.6] for Type T $_{1}$ , T’). We only give some calculations to check that $(E, \sigma )$ in Table 1 is correct for Type CC.

Table 1 List of defining relations and the corresponding geometric pairs.

Let $A=k\langle x,y,z \rangle /(f_{1}, f_{2}, f_{3})$ be a three-dimensional Calabi–Yau quantum polynomial algebra of Type CC where

$$ \begin{align*}f_{1}=yz-zy+y^{2}+3x^{2}, \; f_{2}=zx-xz+yx+xy-yz-zy, \; f_{3}=xy-yx-y^{2},\end{align*} $$

and let $E=\mathcal {V}(x^{3}-y^{2}z)$ , and

$$ \begin{align*}\sigma(a,b,c)=\begin{cases} \left(a-b,b, -3\dfrac{a^{2}}{b}+3a-b+c\right) & \text{ if } (a, b, c)\neq (0, 0, 1), \\ (0, 0, 1) & \text{ if } (a, b, c)=(0, 0, 1), \end{cases}\end{align*} $$

as in Table 1. If $p=(a, b, c)\in E$ , then $a^{3}-b^{2}c=0$ , so

$$ \begin{align*} f_{1}(p, \sigma(p)) &= f_{1}\left((a, b, c), \left(a-b,b, -3\dfrac{a^{2}}{b}+3a-b+c\right)\right) \\ &= \; b\left(-3\dfrac{a^{2}}{b}+3a-b+c\right)-cb+b^{2}+3a(a-b) \\ &= \; -3a^{2}+3ab-b^{2}+bc-bc+b^{2}+3a^{2}-3ab =0, \\ f_{2}(p, \sigma(p)) &= f_{2}\left((a, b, c), \left(a-b,b, -3\dfrac{a^{2}}{b}+3a-b+c\right)\right)\\ &= \; c(a-b)-a\left(-3\dfrac{a^{2}}{b}+3a-b+c\right)+b(a-b)\\ &\quad +ab-b\left(-3\dfrac{a^{2}}{b}+3a-b+c\right)-cb \\ &= \; ac-bc+3\dfrac{a^{3}}{b}-3a^{2}+ab-ac+ab-b^{2}\\ &\quad+ab+3a^{2}-3ab+b^{2}-bc-bc \\ &= \; \dfrac{3}{b}(a^{3}-b^{2}c)=0, \\ f_{3}(p, \sigma(p)) &= f_{3}\left((a, b, c), \left(a-b,b, -3\dfrac{a^{2}}{b}+3a-b+c\right)\right) \\ &= \; ab-b(a-b)-b^{2} = ab-ab+b^{2}-b^{2} = 0, \end{align*} $$

hence $\{(p, \sigma (p))\in \mathbb P^{2}\times \mathbb P^{2}\mid p\in E\}\subset \mathcal V(f_{1}, f_{2}, f_{3})$ . Since $E\subset \mathbb P^{2}$ is a cuspidal cubic curve (and we know that the point scheme of A is not $\mathbb P^{2}$ ), E is the point scheme of A, so ${\mathcal P}(A)=(E, \sigma )$ . ▪

Theorem 3.3 If $A=\mathcal A(E, \sigma )$ is a three-dimensional Calabi–Yau quantum polynomial algebra, then $||\sigma ||=|\sigma ^{3}|$ , so the following are equivalent:

1. (1) $|\sigma |<\infty $ .

2. (2) $||\sigma ||<\infty $ .

3. (3) A is finite over its center.

4. (4) ${\sf Proj}_{\text {nc}} A$ is finite over its center.

Proof First, we will show that $||\sigma ||=|\sigma ^{3}|$ for each type using the defining relations $f_{1}, f_{2}, f_{3}$ and geometric pairs $(E, \sigma )$ given in Lemma 3.2. Recall that $\sigma ^{i}\in \text {Aut}_{k}(\mathbb P^{2}, E)$ if and only if it is represented by a matrix in $\text {PGL}_{3}(k)\cong \text {Aut}_{k} \mathbb P^{2}$ .

Type P Since $\sigma ^{3}=\text {id}$ , $||\sigma ||=1=|\sigma ^{3}|$ .

$\underline {\text {Type S}_{1}}$ Since

$$ \begin{align*} \begin{cases} \sigma^{i}(0,b,c)=(0,b,\alpha^{i} c), \\ \sigma^{i}(a,0,c)=(\alpha^{i} a,0,c)=(\alpha^{2i}a, 0, \alpha^{i}c), \\ \sigma^{i}(a,b,0)=(a,\alpha^{i} b,0)=(\alpha^{2i}a, \alpha^{3i}b, 0), \end{cases} \end{align*} $$

$\sigma ^{i}\in \text {Aut}_{k}(\mathbb P^{2}, E)$ if and only if $\alpha ^{3i}=1$ , so $||\sigma ||=|\alpha ^{3}|=|\sigma ^{3}|$ .

$\underline {\text {Type S}_{3}}$ Since

$$ \begin{align*} \begin{cases} \sigma^{i}(0,b,c)=(0,b,\alpha^{i} c)\begin{pmatrix} 0 & 1 & 0 \\ 0 & 0 & 1 \\ 1 & 0 & 0 \end{pmatrix}^{i}, \\ \sigma^{i}(a,0,c)=(\alpha^{i} a,0,c)\begin{pmatrix} 0 & 1 & 0 \\ 0 & 0 & 1 \\ 1 & 0 & 0 \end{pmatrix}^{i}, \\ \sigma^{i}(a,b,0)=(a,\alpha^{i} b,0)\begin{pmatrix} 0 & 1 & 0 \\ 0 & 0 & 1 \\ 1 & 0 & 0 \end{pmatrix}^{i}, \end{cases} \end{align*} $$

and $\begin {pmatrix} 0 & 1 & 0 \\ 0 & 0 & 1 \\ 1 & 0 & 0 \end {pmatrix}\in \text {Aut}_{k} (\mathbb P^{2}, E)$ , $\sigma ^{i}\in \text {Aut}_{k}(\mathbb P^{2}, E)$ if and only if $\alpha ^{3i}=1$ , so $||\sigma ||=|\alpha ^{3}|=|\sigma ^{3}|$ .

Type S’ Since

$$ \begin{align*} \begin{cases} \sigma^{i}(0,b,c)=(0,b, \alpha^{i} c), \\ \sigma^{i}(a,b,c)=(a,\alpha^{i} b,\alpha^{-i} c)=(\alpha^{-i}a, b, \alpha^{-2i}c), \\ \end{cases} \end{align*} $$

$\sigma ^{i}\in \text {Aut}_{k}(\mathbb P^{2}, E)$ if and only if $\alpha ^{3i}=1$ , so $||\sigma ||=|\alpha ^{3}|=|\sigma ^{3}|$ .

$\underline {\text {Type T}_{1}}$ Since

$$ \begin{align*} \begin{cases} \sigma^{i}(0,b,c)=(0,b,ib+c), \\ \sigma^{i}(a,0,c)=(a,0,ia+c), \\ \sigma^{i}(a,a,c)=(a,a,-ia+c), \end{cases} \end{align*} $$

$\sigma ^{i}\not \in \text {Aut}_{k} (\mathbb P^{2}, E)$ for every $i\geq 1$ , so $||\sigma ||=\infty =|\sigma ^{3}|$ .

$\underline {\text {Type T}_{3}}$ Since

$$ \begin{align*} \begin{cases} \sigma^{3i}(0,b,c)=(0,b,ib+c), \\ \sigma^{3i}(a,0,c)=(a,0, ia+c), \\ \sigma^{3i}(a,a,c)=(a,a,-ia+c), \end{cases} \end{align*} $$

$\sigma ^{3i}\not \in \text {Aut}_{k} (\mathbb P^{2}, E)$ for every $i\geq 1$ , so $||\sigma ||=\infty =|\sigma ^{3}|$ .

Type T’ Since

$$ \begin{align*} \begin{cases} \sigma^{i}(a,0,c)=(a,0,ia+c), \\ \sigma^{i}(a,b,c)=(a-ib,b,-2ia+i^{2}b+c), \end{cases} \end{align*} $$

$\sigma ^{i}\not \in \text {Aut}_{k} (\mathbb P^{2}, E)$ for every $i\geq 1$ , so $||\sigma ||=\infty =|\sigma ^{3}|$ .

Type NC Since

$$ \begin{align*}\sigma^{i}(a, b, c)=\left(a, \alpha^{i} b, -\frac{\alpha^{3i}-1}{\alpha^{i-1}(\alpha^{3}-1)}\frac{a^{2}}{b}+\alpha^{2i} c\right),\end{align*} $$

$\sigma ^{i}\in \text {Aut}_{k}(\mathbb P^{2}, E)$ if and only if $\alpha ^{3i}=1$ , so $||\sigma ||=|\alpha ^{3}|=|\sigma ^{3}|$ .

Type CC Since

$$ \begin{align*}\sigma^{i}(a, b, c)=\left(a-ib, b, -3i\frac{a^{2}}{b}+3i^{2}a-i^{3}b+c\right),\end{align*} $$

$\sigma ^{i}\not \in \text {Aut} (\mathbb P^{2}, E)$ for every $i\geq 1$ , so $||\sigma ||=\infty =|\sigma ^{3}|$ .

Type TL Since $A=k\langle x, y, z\rangle /(yz-\alpha zy-x^{2}, zx-\alpha xz, xy-\alpha yx), \; \alpha ^{3}=1$ , we see that $x\in A_{1}$ is a regular normal element. Since $A/(x)\cong k\langle y, z\rangle /(yz-\alpha zy)$ is a two-dimensional quantum polynomial algebra, ${\sf Proj}_{\text {nc}} A/(x)\cong \mathbb P^{1}$ has no fat point. Since $A[x^{-1}]_{0}\cong k\langle u, v\rangle /(uv-vu-\alpha )$ where $u=yx^{-1}, v=zx^{-1}$ is isomorphic to the first Weyl algebra, ${\sf Spec}_{\text {nc}} A[x^{-1}]_{0}$ has no (fat) point by Example 2.9. By Theorem 2.15, ${\sf Proj}_{\text {nc}} A$ has no fat point. Since $E\neq \mathbb P^{2}$ , $||\sigma ||=\infty =|\sigma ^{3}|$ by Lemma 2.14.

Type WL Since $A=k\langle x, y, z\rangle /(yz-zy-(1/3)y^{2}, zx-xz-(1/3)(yx+xy), xy-yx)$ , we see that $y\in A_{1}$ is a regular normal element. Since $A/(y)\cong k[x, z]$ is a two-dimensional (quantum) polynomial algebra, ${\sf Proj}_{\text {nc}} A/(y)= \mathbb P^{1}$ has no fat point. Since $A[y^{-1}]_{0}\cong k\langle u, v\rangle /(vu-uv-u)$ where $u=xy^{-1}, v=zy^{-1}$ is isomorphic to the enveloping algebra of a two-dimensional nonabelian Lie algebra, ${\sf Spec}_{\text {nc}} A[y^{-1}]_{0}$ has no fat point by Example 2.10. By Theorem 2.15, ${\sf Proj}_{\text {nc}} A$ has no fat point. Since $E\neq \mathbb P^{2}$ , $||\sigma ||=\infty =|\sigma ^{3}|$ by Lemma 2.14.

Type EC Since $\sigma _{p}^{i}=\sigma _{ip}\in \text {Aut}_{k} (\mathbb P^{2}, E)$ if and only if $ip\in E[3]$ if and only if $3ip=o$ , $||\sigma _{p}||=|3p|=|\sigma ^{3}_{p}|$ .

Next, we will show the equivalences (1) $\Leftrightarrow $ (2) $\Leftrightarrow $ (3) $\Leftrightarrow $ (4). Since $||\sigma ||=|\sigma ^{3}|$ for every type, (1) $\Leftrightarrow $ (2). By Theorem 2.3, (1) $\Leftrightarrow $ (3). By definition, (3) $\Rightarrow $ (4), so it is enough to show that (4) $\Rightarrow $ (2). Indeed, if ${\sf Proj}_{\text {nc}}A$ is finite over its center, then there exists a three-dimensional quantum polynomial algebra $A^{\prime }=\mathcal {A}(E^{\prime },\sigma ^{\prime })$ which is finite over its center such that ${\sf Proj}_{\text {nc}} A\cong {\sf Proj}_{\text {nc}} A^{\prime }$ by Definition 2.6, so $\|\sigma \|=\|\sigma ^{\prime }\| \leq $ $|\sigma ^{\prime }|<\infty $ by Lemma 2.5 and Theorem 2.3.▪

3.2 General case

Definition 3.2 [14, Definition 3.2]

For a d-dimensional geometric quantum polynomial algebra $A=\mathcal A(E, \sigma )$ with the Nakayama automorphism $\nu \in \text {Aut} A$ , we define a new graded algebra $\overline A:=\mathcal A(E, \nu ^{*}\sigma ^{d})$ satisfying (G2).

Lemma 3.4 [14, Theorem 3.5]

Let A and $A^{\prime }$ be geometric quantum polynomial algebras. If ${\sf grmod} A\cong {\sf grmod} A^{\prime }$ , then $\overline A\cong \overline {A^{\prime }}$ .

Remark 3.5 If A and $A^{\prime }$ are both three-dimensional quantum polynomial algebras of the same Type P, S $_{1}$ , S’ $_{1}$ , T $_{1}$ , T’ $_{1}$ , then the converse of the above lemma was proved in [14, Theorem 3.6].

Theorem 3.6 If $A=\mathcal A(E, \sigma )$ is a three-dimensional quantum polynomial algebra with the Nakayama automorphism $\nu \in \text {Aut} A$ , then $||\sigma ||=|\nu ^{*}\sigma ^{3}|$ , so the following are equivalent:

1. (1) $|\nu ^{*}\sigma ^{3}|<\infty $ .

2. (2) $||\sigma ||<\infty $ .

3. (3) ${\sf Proj}_{\text {nc}} A$ is finite over its center.

Moreover, if A is of Type T, T’, CC, TL, WL, then A is never finite over its center.

Proof For every three-dimensional quantum polynomial algebra $A=\mathcal A(E, \sigma )$ , there exists a three-dimensional Calabi–Yau quantum polynomial algebra $A^{\prime }=\mathcal A(E^{\prime }, \sigma ^{\prime })$ such that ${\sf grmod} A\cong {\sf grmod} A^{\prime }$ by Theorem 3.1. Since the Nakayama automorphism of $A^{\prime }$ is the identity, $\mathcal A(E, \nu ^{*}\sigma ^{3})\ =\ \overline A \ \cong\ \overline {A^{\prime }}\ =\ \mathcal A(E^{\prime }, {\sigma ^{\prime }}^{3})$ by Lemma 3.4, so

$$ \begin{align*}||\sigma||=||\sigma^{\prime}||=|{\sigma^{\prime}}^{3}|=|\nu^{*}\sigma^{3}|\end{align*} $$

by Lemma 2.5 and Theorem 3.3. Since ${\sf Proj}_{\text {nc}} A$ is finite over its center if and only if ${\sf Proj}_{\text {nc}} A^{\prime }$ is finite over its center if and only if $||\sigma ^{\prime }||<\infty $ by Theorem 3.3, we have the equivalences (1) $\Leftrightarrow $ (2) $\Leftrightarrow $ (3).

If A is a three-dimensional quantum polynomial algebra of Type T, T’, CC, TL, WL, then $A^{\prime }$ is of the same type by Lemma 2.5, so $||\sigma ||=||\sigma ^{\prime }||=\infty $ by the proof of Theorem 3.3. It follows that $|\sigma |=\infty $ , so A is not finite over its center by Theorem 2.3.▪

4 An application to Beilinson algebras

We finally apply our results to representation theory of finite-dimensional algebras.

Definition 4.1 [6, Definition 2.7]

Let R be a finite-dimensional algebra of $\text {gldim} R=d<\infty $ . We define an autoequivalence $\nu _{d}\in \text {Aut} D^{b}({\sf mod} R)$ by $\nu _{d}(M):=M\otimes _{R}^{\text {L}}DR[-d]$ where $D^{b}({\sf mod} R)$ is the bounded derived category of ${\sf mod} R$ and $DR:=\text {Hom}_{k}(R, k)$ . We say that R is d-representation infinite if $\nu _{d}^{-i}(R)\in {\sf mod} R$ for all $i\in \mathbb N$ . In this case, we say that a module $M\in {\sf mod} R$ is d-regular if $\nu _{d}^{i}(M)\in {\sf mod} R$ for all $i\in \mathbb Z$ .

By [10], a $1$ -representation infinite algebra is exactly the same as a finite-dimensional hereditary algebra of infinite representation type. For representation theory of such an algebra, regular modules play an essential role.

For a d-dimensional quantum polynomial algebra A, we define the Beilinson algebra of A by

$$ \begin{align*}\nabla A:=\begin{pmatrix} A_{0} & A_{1} & \cdots & A_{d-1} \\ 0 & A_{0} & \cdots & A_{d-2} \\ \vdots & \ddots & \vdots & \vdots \\ 0 & 0 & \cdots & A_{0} \end{pmatrix}.\end{align*} $$

The Beilinson algebra is a typical example of a $(d-1)$ -representation infinite algebra by [11, Theorem 4.12]. To investigate representation theory of such an algebra, it is important to classify simple $(d-1)$ -regular modules.

Corollary 4.1 Let $A=\mathcal A(E, \sigma )$ be a three-dimensional quantum polynomial algebra with the Nakayama automorphism $\nu \in \text {Aut} A$ . Then the following are equivalent:

1. (1) $|\nu ^{*}\sigma ^{3}|=1$ or $\infty $ .

2. (2) ${\sf Proj}_{\text {nc}} A$ has no fat point.

3. (3) The isomorphism classes of simple $2$ -regular modules over $\nabla A$ are parameterized by the set of closed points of $E\subset \mathbb P^{2}$ .

In particular, if A is of P, T, T’, CC, TL, WL, then A satisfies all of the above conditions.

Proof (1) $\Leftrightarrow $ (2): This follow from Theorem 3.6 and Lemma 2.14.

(2) $\Leftrightarrow $ (3): By [13, Theorem 3.6], isomorphism classes of simple $2$ -regular modules over $\nabla A$ are parameterized by the set of points of ${\sf Proj}_{\text {nc}} A$ . On the other hand, it is well known that the points of ${\sf Proj}_{\text {nc}} A$ which are not fat (called ordinary points in [13]) are parameterized by the set of closed points of E (see [13, Proposition 4.4]); hence, the result holds.▪

Remark 4.2 We have the following characterization of Type P, T, T’, CC, TL, WL. Let $A=\mathcal A(E, \sigma )$ be a three-dimensional quantum polynomial algebra. Write $E=\mathcal V(f)\subset \mathbb P^{2}$ where $f\in k[x, y, z]_{3}$ . Recall that the Hessian of f is defined by $H(f):=\det \begin {pmatrix} f_{xx} & f_{xy} & f_{xz} \\ f_{yx} & f_{yy} & f_{yz} \\ f_{zx} & f_{zy} & f_{zz} \end {pmatrix}\in k[x, y, z]_{3}$ . Then A is of Type P, T, T’, CC, TL, WL if and only if $H^{2}(f):=H(H(f))=0$ .

Remark 4.3 If A is a two-dimensional quantum polynomial algebra, then , so $\nabla A$ is a finite-dimensional hereditary algebra of tame representation type. It is known that the isomorphism classes of simple regular modules over $\nabla A$ are parameterized by $\mathbb P^{1}$ (cf. [13, Theorem 3.19]). For a three-dimensional quantum polynomial algebra A, we expect that the following are equivalent:

1. (1) ${\sf Proj}_{\text {nc}} A$ is finite over its center.

2. (2) $\nabla A$ is $2$ -representation tame in the sense of [6].

3. (3) The isomorphism classes of simple $2$ -regular modules over $\nabla A$ are parameterized by $\mathbb P^{2}$ .

These equivalences are shown for Type S in [13, Theorems 4.17 and 4.21].