1. Introduction and motivation
Wall-bounded flows are ubiquitous in various applications, such as over aircraft wings and around submarines, where they affect vessel performance through the imposition of wall-shear-stress (![]() ${\tau }_{w}$) and wall-pressure (
${\tau }_{w}$) and wall-pressure (![]() $\,p_{w}$) fluctuations on the bounding surface. The former,
$\,p_{w}$) fluctuations on the bounding surface. The former, ![]() ${\tau }_w$, are associated with the skin-friction drag which limits vehicle speed, while the latter,
${\tau }_w$, are associated with the skin-friction drag which limits vehicle speed, while the latter, ![]() $p_w$, are responsible for flow-induced vibrations that affect structural stability and generate noise. Nevertheless, accurately modelling the fluctuations in
$p_w$, are responsible for flow-induced vibrations that affect structural stability and generate noise. Nevertheless, accurately modelling the fluctuations in ![]() ${\tau }_w$ and
${\tau }_w$ and ![]() $p_w$ remains a challenge due to the limited understanding of the dependence of their generation mechanisms on the Reynolds number.
$p_w$ remains a challenge due to the limited understanding of the dependence of their generation mechanisms on the Reynolds number.
Figure 1 shows a compilation of premultiplied frequency spectra of ![]() ${\tau }_{w}$ and
${\tau }_{w}$ and ![]() $p_{w}$ for a wide range of Reynolds numbers. Here, the viscous-scaled time is defined as
$p_{w}$ for a wide range of Reynolds numbers. Here, the viscous-scaled time is defined as ![]() $T^+ = {{{U}^2_{\tau }}}/(\,{f}{\nu })$, where
$T^+ = {{{U}^2_{\tau }}}/(\,{f}{\nu })$, where ![]() $f$ is the frequency of turbulence scales,
$f$ is the frequency of turbulence scales, ![]() $\nu$ is the kinematic viscosity and
$\nu$ is the kinematic viscosity and ![]() ${U}_{\tau }$ is the mean friction velocity, with superscript ‘+’ indicating viscous scaling. All these spectra have been computed from previously published high-fidelity simulations (
${U}_{\tau }$ is the mean friction velocity, with superscript ‘+’ indicating viscous scaling. All these spectra have been computed from previously published high-fidelity simulations (![]() $500 \lesssim Re_{\tau } \lesssim 2000$) and experimental data sets (
$500 \lesssim Re_{\tau } \lesssim 2000$) and experimental data sets (![]() $ {O}(10^4) \lesssim Re_{\tau } \lesssim {O}(10^6)$) of a turbulent boundary layer (TBL), where
$ {O}(10^4) \lesssim Re_{\tau } \lesssim {O}(10^6)$) of a turbulent boundary layer (TBL), where ![]() $Re_{\tau } = {U}_{\tau }{\delta }/{\nu }$ is the friction Reynolds number and
$Re_{\tau } = {U}_{\tau }{\delta }/{\nu }$ is the friction Reynolds number and ![]() $\delta$ is the TBL thickness. Although the experimental spectra have certain limitations at
$\delta$ is the TBL thickness. Although the experimental spectra have certain limitations at ![]() $T^+ \lesssim 100$ owing to spatial and temporal resolution, they will not influence this discussion (see § 3).
$T^+ \lesssim 100$ owing to spatial and temporal resolution, they will not influence this discussion (see § 3).

Figure 1. Premultiplied energy spectra of (a) ![]() ${\tau }_w$, (b)
${\tau }_w$, (b) ![]() $p_w$ and (c) premultiplied spectrogram of the bulk turbulence production (
$p_w$ and (c) premultiplied spectrogram of the bulk turbulence production (![]() $\,f{z^+}{P^+}$) as a function of
$\,f{z^+}{P^+}$) as a function of ![]() $T^+ (= 1/{f^+})$. Data for
$T^+ (= 1/{f^+})$. Data for ![]() $500 \lesssim Re_{\tau } \lesssim 2000$ are from Eitel-Amor, Örlü & Schlatter (Reference Eitel-Amor, Örlü and Schlatter2014), for
$500 \lesssim Re_{\tau } \lesssim 2000$ are from Eitel-Amor, Örlü & Schlatter (Reference Eitel-Amor, Örlü and Schlatter2014), for ![]() $Re_{\tau } \sim {O}(10^4)$ are from Marusic et al. (Reference Marusic, Chandran, Rouhi, Fu, Wine, Holloway, Chung and Smits2021) and for
$Re_{\tau } \sim {O}(10^4)$ are from Marusic et al. (Reference Marusic, Chandran, Rouhi, Fu, Wine, Holloway, Chung and Smits2021) and for ![]() $Re_{\tau } \sim {O}(10^6)$ are from the Surface Layer Turbulence and Environmental Science Test (SLTEST) facility data sets acquired by (a) Marusic & Heuer (Reference Marusic and Heuer2007) and (b) Klewicki, Priyadarshana & Metzger (Reference Klewicki, Priyadarshana and Metzger2008). Grey and yellow coloured backgrounds in (a–c) indicate the nominal intermediate (
$Re_{\tau } \sim {O}(10^6)$ are from the Surface Layer Turbulence and Environmental Science Test (SLTEST) facility data sets acquired by (a) Marusic & Heuer (Reference Marusic and Heuer2007) and (b) Klewicki, Priyadarshana & Metzger (Reference Klewicki, Priyadarshana and Metzger2008). Grey and yellow coloured backgrounds in (a–c) indicate the nominal intermediate (![]() $ {O}(10^2) \lesssim T^+ \lesssim {O}(10^3)$) and large-scale ranges (
$ {O}(10^2) \lesssim T^+ \lesssim {O}(10^3)$) and large-scale ranges (![]() $T^+ \gtrsim {O}(10^3)$), respectively.
$T^+ \gtrsim {O}(10^3)$), respectively.
A noteworthy observation from figure 1(a,b) is the increasing energy contribution to the ![]() ${\tau }_w- (T^+ \gtrsim {O}(10^3))$ and
${\tau }_w- (T^+ \gtrsim {O}(10^3))$ and ![]() $p_w$-spectra (
$p_w$-spectra (![]() $T^+ > {O}(10^2)$) with increasing
$T^+ > {O}(10^2)$) with increasing ![]() $Re_{\tau }$, which has been noted previously in all canonical wall flows (Tsuji et al. Reference Tsuji, Fransson, Alfredsson and Johansson2007; Örlü & Schlatter Reference Örlü and Schlatter2011; Mathis et al. Reference Mathis, Marusic, Chernyshenko and Hutchins2013; Panton, Lee & Moser Reference Panton, Lee and Moser2017; Yu, Ceci & Pirozzoli Reference Yu, Ceci and Pirozzoli2022; Baars, Dacome & Lee Reference Baars, Dacome and Lee2024). While these viscous-scaled spectra exhibit
$Re_{\tau }$, which has been noted previously in all canonical wall flows (Tsuji et al. Reference Tsuji, Fransson, Alfredsson and Johansson2007; Örlü & Schlatter Reference Örlü and Schlatter2011; Mathis et al. Reference Mathis, Marusic, Chernyshenko and Hutchins2013; Panton, Lee & Moser Reference Panton, Lee and Moser2017; Yu, Ceci & Pirozzoli Reference Yu, Ceci and Pirozzoli2022; Baars, Dacome & Lee Reference Baars, Dacome and Lee2024). While these viscous-scaled spectra exhibit ![]() $Re_{\tau }$-invariance in the small-scale range (
$Re_{\tau }$-invariance in the small-scale range (![]() $T^+ \lesssim 100$), they exhibit distinct
$T^+ \lesssim 100$), they exhibit distinct ![]() $Re_{\tau }$-variations in the intermediate (
$Re_{\tau }$-variations in the intermediate (![]() $O(10^2) \lesssim T^+ \lesssim O(10^3)$) and large-scale ranges (
$O(10^2) \lesssim T^+ \lesssim O(10^3)$) and large-scale ranges (![]() $T^+ \gtrsim O(10^3)$), with these nominal bounds considered based on the largest possible
$T^+ \gtrsim O(10^3)$), with these nominal bounds considered based on the largest possible ![]() $Re_{\tau }$-range (figure 1a,b). Although past studies have broadly linked these
$Re_{\tau }$-range (figure 1a,b). Although past studies have broadly linked these ![]() $Re_{\tau }$ trends to the energization of large inertial motions, the present study addresses two fundamental questions that have remained unanswered: (i) Which motions are responsible for transporting the large-scale energy (i.e. energy of large-scale motions), residing in the outer region, towards the wall to influence the wall-shear stress (Lee & Moser Reference Lee and Moser2019; Lee & Hwang Reference Lee and Hwang2024)? (ii) Which motions contribute to the rapid
$Re_{\tau }$ trends to the energization of large inertial motions, the present study addresses two fundamental questions that have remained unanswered: (i) Which motions are responsible for transporting the large-scale energy (i.e. energy of large-scale motions), residing in the outer region, towards the wall to influence the wall-shear stress (Lee & Moser Reference Lee and Moser2019; Lee & Hwang Reference Lee and Hwang2024)? (ii) Which motions contribute to the rapid ![]() $Re_{\tau }$-growth of
$Re_{\tau }$-growth of ![]() $p_w$ in the intermediate scales (
$p_w$ in the intermediate scales (![]() $O(10^2) \lesssim T^+ \lesssim O(10^3)$), and why is this growth relatively weaker in the case of
$O(10^2) \lesssim T^+ \lesssim O(10^3)$), and why is this growth relatively weaker in the case of ![]() ${\tau }_w$?
${\tau }_w$?
The origin of the large-scale inertia-dominated energy in the outer region has now been well established in the literature (Lee & Moser Reference Lee and Moser2019), based on the turbulent kinetic energy (TKE) production term, ![]() $P^+ = -2{{\overline {uw}}^+}({{\partial }{{{U}}^+}}/{{\partial }{z^+}})$. Here,
$P^+ = -2{{\overline {uw}}^+}({{\partial }{{{U}}^+}}/{{\partial }{z^+}})$. Here, ![]() $u$,
$u$, ![]() $v$ and
$v$ and ![]() $w$ represent instantaneous velocity fluctuations along the streamwise (
$w$ represent instantaneous velocity fluctuations along the streamwise (![]() $x$), spanwise (
$x$), spanwise (![]() $\kern0.06em y$) and wall-normal (
$\kern0.06em y$) and wall-normal (![]() $z$) directions, respectively, while capital letters and overbar indicate time averaging. Figure 1(c) shows the premultiplied spectra of the bulk turbulence production (
$z$) directions, respectively, while capital letters and overbar indicate time averaging. Figure 1(c) shows the premultiplied spectra of the bulk turbulence production (![]() $\,f{z^+}{P^+}(z^+; T^+)$) from the same simulation data sets as in figure 1(a,b). Note here that the premultiplication with
$\,f{z^+}{P^+}(z^+; T^+)$) from the same simulation data sets as in figure 1(a,b). Note here that the premultiplication with ![]() $z^+$ artificially amplifies the spectral content in the outer region compared with the inner region, but the majority of the energy production essentially occurs near the wall when
$z^+$ artificially amplifies the spectral content in the outer region compared with the inner region, but the majority of the energy production essentially occurs near the wall when ![]() $Re_{\tau } \lesssim {O}(10^3)$. Figure 1(c) confirms the weak energy production in the near-wall large scales (
$Re_{\tau } \lesssim {O}(10^3)$. Figure 1(c) confirms the weak energy production in the near-wall large scales (![]() $T^+ > 1000$) compared with the small scales, thereby suggesting association of the large-scale
$T^+ > 1000$) compared with the small scales, thereby suggesting association of the large-scale ![]() ${\tau _w}-$signatures with wall-ward energy transport from the outer region (Lee & Hwang Reference Lee and Hwang2024). While several past studies have quantified and predicted the superposition of large-scale signatures on
${\tau _w}-$signatures with wall-ward energy transport from the outer region (Lee & Hwang Reference Lee and Hwang2024). While several past studies have quantified and predicted the superposition of large-scale signatures on ![]() ${\tau }_w$ (Metzger & Klewicki Reference Metzger and Klewicki2001; Örlü & Schlatter Reference Örlü and Schlatter2011; Mathis et al. Reference Mathis, Marusic, Chernyshenko and Hutchins2013), fundamental understanding of their energy-transfer mechanisms is still lacking, starting from the motions governing the transport of large-scale energy from outer region towards the wall.
${\tau }_w$ (Metzger & Klewicki Reference Metzger and Klewicki2001; Örlü & Schlatter Reference Örlü and Schlatter2011; Mathis et al. Reference Mathis, Marusic, Chernyshenko and Hutchins2013), fundamental understanding of their energy-transfer mechanisms is still lacking, starting from the motions governing the transport of large-scale energy from outer region towards the wall.
With regards to the differences between ![]() ${\tau }_w$ and
${\tau }_w$ and ![]() $p_w$ over
$p_w$ over ![]() $ {O}(10^2) \lesssim T^+ \lesssim {O}(10^3)$, although considerable TKE is produced in this scale range in the inner region, the contour levels of the TKE production spectra in figure 1(c) exhibit a convincing collapse/invariance across different
$ {O}(10^2) \lesssim T^+ \lesssim {O}(10^3)$, although considerable TKE is produced in this scale range in the inner region, the contour levels of the TKE production spectra in figure 1(c) exhibit a convincing collapse/invariance across different ![]() $Re_{\tau }$. This suggests a negligible
$Re_{\tau }$. This suggests a negligible ![]() $Re_{\tau }$-increment in the near-wall TKE production in the intermediate-scale range (
$Re_{\tau }$-increment in the near-wall TKE production in the intermediate-scale range (![]() $ {O}(10^2) \lesssim T^+ \lesssim {O}(10^3)$), consistent with results presented by Lee & Moser (Reference Lee and Moser2019). It means that the
$ {O}(10^2) \lesssim T^+ \lesssim {O}(10^3)$), consistent with results presented by Lee & Moser (Reference Lee and Moser2019). It means that the ![]() $Re_{\tau }$ increase of
$Re_{\tau }$ increase of ![]() $f{{\phi }^{+}_{{{p}_w}{{p}_w}}}$ in the intermediate-scale range is also associated with the energization of turbulence motions farther away from the wall, which, however, seem to influence
$f{{\phi }^{+}_{{{p}_w}{{p}_w}}}$ in the intermediate-scale range is also associated with the energization of turbulence motions farther away from the wall, which, however, seem to influence ![]() $f{{\phi }^{+}_{{{\tau }_w}{{\tau }_w}}}$ relatively weakly. The present study attempts to advance our fundamental understanding of the turbulence motions governing these trends, and their roles in the underlying energy-transfer mechanisms. This information would be valuable for designing high
$f{{\phi }^{+}_{{{\tau }_w}{{\tau }_w}}}$ relatively weakly. The present study attempts to advance our fundamental understanding of the turbulence motions governing these trends, and their roles in the underlying energy-transfer mechanisms. This information would be valuable for designing high![]() $-Re_{\tau }$ drag and noise reduction strategies in the future.
$-Re_{\tau }$ drag and noise reduction strategies in the future.
2. Active and inactive components and their roles in the energy-transfer mechanisms
This study explores the ability of the attached-eddy (i.e. wall-scaled eddy; Townsend Reference Townsend1976) framework to provide a phenomenological explanation for the questions raised above. This is motivated by the fact that the same framework has been previously successful in predicting the ![]() ${\tau }_w$- and
${\tau }_w$- and ![]() $p_w$-spectra, associated with the inertial scales, across a broad
$p_w$-spectra, associated with the inertial scales, across a broad ![]() $Re_{\tau }$-range (Ahn, Graham & Rizzi Reference Ahn, Graham and Rizzi2010; Marusic et al. Reference Marusic, Chandran, Rouhi, Fu, Wine, Holloway, Chung and Smits2021). Per Townsend's hypothesis, the outer region (
$Re_{\tau }$-range (Ahn, Graham & Rizzi Reference Ahn, Graham and Rizzi2010; Marusic et al. Reference Marusic, Chandran, Rouhi, Fu, Wine, Holloway, Chung and Smits2021). Per Townsend's hypothesis, the outer region (![]() $z \gtrsim z_{min}$) of a high
$z \gtrsim z_{min}$) of a high![]() $-Re_{\tau }$ wall-bounded flow can be statistically represented by a hierarchy (
$-Re_{\tau }$ wall-bounded flow can be statistically represented by a hierarchy (![]() $ {O}(z_{min}) \lesssim \mathcal {H} \lesssim {O}(\delta )$) of geometrically self-similar, inviscid, wall-scaled eddies dominated by inertia (figure 2), where
$ {O}(z_{min}) \lesssim \mathcal {H} \lesssim {O}(\delta )$) of geometrically self-similar, inviscid, wall-scaled eddies dominated by inertia (figure 2), where ![]() $\mathcal {H}$ is the eddy height and
$\mathcal {H}$ is the eddy height and ![]() $z_{min}$ is the nominal lower bound of the log region. These wall-scaled eddies have been classically referred to as attached eddies in the literature, in reference to their scaling with
$z_{min}$ is the nominal lower bound of the log region. These wall-scaled eddies have been classically referred to as attached eddies in the literature, in reference to their scaling with ![]() $z$ and
$z$ and ![]() ${U}_{\tau }$, and not necessarily implying their physical extension to the wall. Townsend (Reference Townsend1976) proposed that these eddies have a population density varying inversely to their height
${U}_{\tau }$, and not necessarily implying their physical extension to the wall. Townsend (Reference Townsend1976) proposed that these eddies have a population density varying inversely to their height ![]() $\mathcal {H}$, leading their cumulative velocity contributions at
$\mathcal {H}$, leading their cumulative velocity contributions at ![]() $z \gtrsim z_{min}$ to follow (at asymptotically high
$z \gtrsim z_{min}$ to follow (at asymptotically high ![]() $Re_\tau$)
$Re_\tau$)
 \begin{equation} \left. \begin{aligned} {{\overline{u^2}}^+} & = {B_1} - {A_1} \ln({z}/{\delta}), \\ {{\overline{v^2}}^+} & = {B_2} - {A_2} \ln({z}/{\delta}), \\ {{\overline{w^2}}^+} & = {B_3}\quad \text{and} \\ {{\overline{uw}}^+} & = {B_4}, \end{aligned} \right\} \end{equation}
\begin{equation} \left. \begin{aligned} {{\overline{u^2}}^+} & = {B_1} - {A_1} \ln({z}/{\delta}), \\ {{\overline{v^2}}^+} & = {B_2} - {A_2} \ln({z}/{\delta}), \\ {{\overline{w^2}}^+} & = {B_3}\quad \text{and} \\ {{\overline{uw}}^+} & = {B_4}, \end{aligned} \right\} \end{equation}
where ![]() $B_{1-4}$ and
$B_{1-4}$ and ![]() $A_{1-2}$ are constants. The increased availability of high-
$A_{1-2}$ are constants. The increased availability of high-![]() $Re_{\tau }$ data over the past two decades has provided considerable empirical support to these expressions (Jimenez & Hoyas Reference Jimenez and Hoyas2008; Hultmark et al. Reference Hultmark, Vallikivi, Bailey and Smits2012; Lee & Moser Reference Lee and Moser2015; Orlandi, Bernardini & Pirozzoli Reference Orlandi, Bernardini and Pirozzoli2015) in the nominal log region of the TBL (the exact definition of which may vary in the literature). The disagreement beyond the log region is owing to the statistical significance of the non-wall-scaled eddy-type motions coexisting in boundary layers, particularly in the boundary layer wake region (Marusic & Perry Reference Marusic and Perry1995).
$Re_{\tau }$ data over the past two decades has provided considerable empirical support to these expressions (Jimenez & Hoyas Reference Jimenez and Hoyas2008; Hultmark et al. Reference Hultmark, Vallikivi, Bailey and Smits2012; Lee & Moser Reference Lee and Moser2015; Orlandi, Bernardini & Pirozzoli Reference Orlandi, Bernardini and Pirozzoli2015) in the nominal log region of the TBL (the exact definition of which may vary in the literature). The disagreement beyond the log region is owing to the statistical significance of the non-wall-scaled eddy-type motions coexisting in boundary layers, particularly in the boundary layer wake region (Marusic & Perry Reference Marusic and Perry1995).

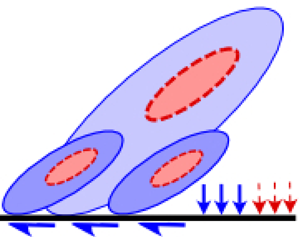
Figure 2. Conceptual sketches of wall-scaled eddies in a TBL at relatively (a) low (![]() $Re_{{\tau }_1}$) and (b) high (
$Re_{{\tau }_1}$) and (b) high (![]() $Re_{{\tau }_2}$) Reynolds numbers. (c–e) Depict the roles of the active and inactive parts of wall-scaled eddies in the energy-transfer mechanisms per the hypotheses of Bradshaw (Reference Bradshaw1967) and de Giovanetti, Hwang & Choi (Reference de Giovanetti, Hwang and Choi2016). Blue and red sections, respectively, correspond to the inactive and active portions of the wall-scaled eddies (grey scaled), which are defined based on the point of observation,
$Re_{{\tau }_2}$) Reynolds numbers. (c–e) Depict the roles of the active and inactive parts of wall-scaled eddies in the energy-transfer mechanisms per the hypotheses of Bradshaw (Reference Bradshaw1967) and de Giovanetti, Hwang & Choi (Reference de Giovanetti, Hwang and Choi2016). Blue and red sections, respectively, correspond to the inactive and active portions of the wall-scaled eddies (grey scaled), which are defined based on the point of observation, ![]() $z$: (c)
$z$: (c) ![]() $z_1$ or (d,e)
$z_1$ or (d,e) ![]() $z_2$. In (d,e), white eddies with dashed contours are centred lower than
$z_2$. In (d,e), white eddies with dashed contours are centred lower than ![]() $z$, and hence are not involved in either local TKE production at
$z$, and hence are not involved in either local TKE production at ![]() $z$ (red region), or non-local energy transport from
$z$ (red region), or non-local energy transport from ![]() $z$ to the wall (cyan arrows). Greater thickness of the arrows in (e) indicates larger transport magnitude at higher
$z$ to the wall (cyan arrows). Greater thickness of the arrows in (e) indicates larger transport magnitude at higher ![]() $Re_{\tau }$. Panels show (a)
$Re_{\tau }$. Panels show (a) ![]() $Re_{{\tau }_1}$; (b)
$Re_{{\tau }_1}$; (b) ![]() $Re_{{\tau }_2}>Re_{{\tau }_1}$; (c)
$Re_{{\tau }_2}>Re_{{\tau }_1}$; (c) ![]() $Re_{{\tau }_1}$, point of observation:
$Re_{{\tau }_1}$, point of observation: ![]() $z_1$; (d)
$z_1$; (d) ![]() $Re_{{\tau }_1}$, point of observation:
$Re_{{\tau }_1}$, point of observation: ![]() $z_2$; (e)
$z_2$; (e) ![]() $Re_{{\tau }_2}$, point of observation:
$Re_{{\tau }_2}$, point of observation: ![]() $z_2$.
$z_2$.
While the expressions for ![]() ${\overline {u^2}}^+$ and
${\overline {u^2}}^+$ and ![]() ${\overline {v^2}}^+$ in (2.1) suggest their dependence on
${\overline {v^2}}^+$ in (2.1) suggest their dependence on ![]() $Re_{\tau }$,
$Re_{\tau }$, ![]() ${\overline {w^2}}^+$ and
${\overline {w^2}}^+$ and ![]() ${\overline {uw}}^+$ are postulated to be
${\overline {uw}}^+$ are postulated to be ![]() $Re_{\tau }$-independent in the large
$Re_{\tau }$-independent in the large ![]() $Re_{\tau }$ limit. Townsend (Reference Townsend1976) explained this contradiction by proposing that the flow at any point of observation (
$Re_{\tau }$ limit. Townsend (Reference Townsend1976) explained this contradiction by proposing that the flow at any point of observation (![]() $z \gtrsim z_{min}$) comprises two components of wall-scaled eddies (figure 2) – an ‘active’ component of the wall-scaled eddy that is responsible for local turbulent transfer and accounts for the instantaneous Reynolds shear stresses (
$z \gtrsim z_{min}$) comprises two components of wall-scaled eddies (figure 2) – an ‘active’ component of the wall-scaled eddy that is responsible for local turbulent transfer and accounts for the instantaneous Reynolds shear stresses (![]() $uw$) at
$uw$) at ![]() $z$, and an ‘inactive’ component that does not contribute to
$z$, and an ‘inactive’ component that does not contribute to ![]() $uw$ at
$uw$ at ![]() $z$. Here, the inactive component predominantly comes from wall-scaled eddies much taller than
$z$. Here, the inactive component predominantly comes from wall-scaled eddies much taller than ![]() $z$, which contribute to
$z$, which contribute to ![]() $uw$ (i.e. behave as active motions) at wall-normal locations larger than
$uw$ (i.e. behave as active motions) at wall-normal locations larger than ![]() $z$. Hence, while the active component is solely responsible for the local TKE production, Townsend (Reference Townsend1976) described inactive motions as non-local ‘swirling motions’ whose ‘effect on that part of the layer between the point of observation and the wall is one of slow random variation of ‘mean velocity’ which cause corresponding variation of wall stress’. These statements were supported by Bradshaw (Reference Bradshaw1967) and de Giovanetti et al. (Reference de Giovanetti, Hwang and Choi2016), who hypothesized that the inactive parts of the wall-scaled eddies are responsible for transporting large-scale energy produced in the outer layer (by active parts), to the wall. As per Bradshaw (Reference Bradshaw1967), this process is necessary to maintain the near-wall energy balance and is sketched in figure 2(c–e).
$z$. Hence, while the active component is solely responsible for the local TKE production, Townsend (Reference Townsend1976) described inactive motions as non-local ‘swirling motions’ whose ‘effect on that part of the layer between the point of observation and the wall is one of slow random variation of ‘mean velocity’ which cause corresponding variation of wall stress’. These statements were supported by Bradshaw (Reference Bradshaw1967) and de Giovanetti et al. (Reference de Giovanetti, Hwang and Choi2016), who hypothesized that the inactive parts of the wall-scaled eddies are responsible for transporting large-scale energy produced in the outer layer (by active parts), to the wall. As per Bradshaw (Reference Bradshaw1967), this process is necessary to maintain the near-wall energy balance and is sketched in figure 2(c–e).
Although the non-local wall-normal transport of large-scale energy has been previously observed through correlation/spectral analysis of the TKE budget equations (Cho, Hwang & Choi Reference Cho, Hwang and Choi2018; Lee & Moser Reference Lee and Moser2019; Yin, Hwang & Vassilicos Reference Yin, Hwang and Vassilicos2024), its connection with the inactive part of the wall-scaled eddies has never been definitively established, primarily due to the lack of a reliable flow-decomposition methodology. Along the same lines, while the association of ![]() $p_w$-signatures with intense
$p_w$-signatures with intense ![]() $uw$ events is well known (Gibeau & Ghaemi Reference Gibeau and Ghaemi2021; Baars et al. Reference Baars, Dacome and Lee2024), their distinct correlations with
$uw$ events is well known (Gibeau & Ghaemi Reference Gibeau and Ghaemi2021; Baars et al. Reference Baars, Dacome and Lee2024), their distinct correlations with ![]() $uw$-contributing (active) and non-contributing (inactive) components has never been explicitly established. Recently, Deshpande, Monty & Marusic (Reference Deshpande, Monty and Marusic2021) proposed a spectral linear stochastic estimation (SLSE)-based methodology that enables data-driven decomposition of the log-region flow into its corresponding active and inactive components. This provides an opportunity to establish the association of active/inactive components with
$uw$-contributing (active) and non-contributing (inactive) components has never been explicitly established. Recently, Deshpande, Monty & Marusic (Reference Deshpande, Monty and Marusic2021) proposed a spectral linear stochastic estimation (SLSE)-based methodology that enables data-driven decomposition of the log-region flow into its corresponding active and inactive components. This provides an opportunity to establish the association of active/inactive components with ![]() ${\tau }_w$ and
${\tau }_w$ and ![]() $p_w$, and thereby explain their
$p_w$, and thereby explain their ![]() $Re_{\tau }$-variation based on the
$Re_{\tau }$-variation based on the ![]() $Re_{\tau }$ growth of the wall-scaled eddy hierarchy (figure 2).
$Re_{\tau }$ growth of the wall-scaled eddy hierarchy (figure 2).
2.1. Revisiting the concept of active and inactive motions
Before proceeding with the new analysis, we briefly review the concept of active and inactive motions by means of a simplified mathematical description, which is inspired by Townsend's original ideas and adapted from our previous work (Deshpande, Monty & Marusic Reference Deshpande, Monty and Marusic2020; Deshpande et al. Reference Deshpande, Monty and Marusic2021). We consider the simplest model of a wall-bounded flow comprising wall-scaled eddies as the only eddying motions, which are represented by hairpin-type vortical structures. It is important to note here, however, that the concept of active and inactive motions does not depend on the shape of the vortical structures, but is rather associated with the spatial distribution of velocity signatures generated by these vortical structures, as depicted in figure 3(a) (estimated by simple Biot–Savart calculations). The inviscid nature of the wall-scaled eddy model permits slip at the wall (i.e. finite ![]() $u$ and
$u$ and ![]() $v$), while
$v$), while ![]() $w = 0$ is enforced at
$w = 0$ is enforced at ![]() $z = 0$ to remain consistent with wall impermeability. These differences in the boundary conditions result in spatially localized
$z = 0$ to remain consistent with wall impermeability. These differences in the boundary conditions result in spatially localized ![]() $w$-signatures (and consequently
$w$-signatures (and consequently ![]() $uw$-signatures) at
$uw$-signatures) at ![]() $z$ from any wall-scaled eddy of
$z$ from any wall-scaled eddy of ![]() $\mathcal {H} \sim {O}(z)$, while the
$\mathcal {H} \sim {O}(z)$, while the ![]() $u$- and
$u$- and ![]() $v$-signatures extend across 0
$v$-signatures extend across 0 ![]() $\lesssim z < \mathcal {H}$ (i.e. non-local; refer to figure 3a). It is these characteristics that distinguish the active and inactive contributions in the velocity flow field, despite both of them originating from the same wall-scaled vortical structures.
$\lesssim z < \mathcal {H}$ (i.e. non-local; refer to figure 3a). It is these characteristics that distinguish the active and inactive contributions in the velocity flow field, despite both of them originating from the same wall-scaled vortical structures.

Figure 3. (a) Spatial signatures of the three velocity components (![]() $u,v,w$) from a hairpin-type vortex structure of height
$u,v,w$) from a hairpin-type vortex structure of height ![]() $\mathcal {H}$, representative of a wall-scaled eddy (Marusic & Perry Reference Marusic and Perry1995). Regions in magenta and indigo, respectively, denote high- and low-momentum regions for the corresponding velocity fluctuations. (b) Eddy-intensity functions (
$\mathcal {H}$, representative of a wall-scaled eddy (Marusic & Perry Reference Marusic and Perry1995). Regions in magenta and indigo, respectively, denote high- and low-momentum regions for the corresponding velocity fluctuations. (b) Eddy-intensity functions (![]() $I_{ij}$) for wall-scaled eddies of three different heights
$I_{ij}$) for wall-scaled eddies of three different heights ![]() ${\mathcal {H}}_i$, with
${\mathcal {H}}_i$, with ![]() ${\mathcal {H}}_1 < {\mathcal {H}}_2 < {\mathcal {H}}_3$. Here,
${\mathcal {H}}_1 < {\mathcal {H}}_2 < {\mathcal {H}}_3$. Here, ![]() $u_i,v_i,w_i$ respectively denote velocity signatures generated from these wall-scaled eddies that are sensed by a probe, depending on their
$u_i,v_i,w_i$ respectively denote velocity signatures generated from these wall-scaled eddies that are sensed by a probe, depending on their ![]() $z$-location. Red and blue background shadings of
$z$-location. Red and blue background shadings of ![]() $u_i,v_i,w_i$, respectively, indicate active and inactive contributions at that location. The figure has been adapted from Deshpande et al. (Reference Deshpande, Monty and Marusic2020).
$u_i,v_i,w_i$, respectively, indicate active and inactive contributions at that location. The figure has been adapted from Deshpande et al. (Reference Deshpande, Monty and Marusic2020).
Differences in the active/inactive contributions to the Reynolds stresses can be understood by invoking Townsend's (Reference Townsend1976) eddy-intensity function ![]() $I_{ij}$ (where
$I_{ij}$ (where ![]() $i,j = u$,
$i,j = u$, ![]() $v$ or
$v$ or ![]() $w$), depicted schematically in figure 3(b) for the four non-zero combinations of
$w$), depicted schematically in figure 3(b) for the four non-zero combinations of ![]() $i,j$ (refer to (2.1)). Here,
$i,j$ (refer to (2.1)). Here, ![]() $I_{ij}$ is representative of the contribution from each eddy to the normal and Reynolds shear stresses as a function of
$I_{ij}$ is representative of the contribution from each eddy to the normal and Reynolds shear stresses as a function of ![]() $z$, and this is sketched for wall-scaled eddies of three different heights (
$z$, and this is sketched for wall-scaled eddies of three different heights (![]() $\mathcal {H}_{1}$–
$\mathcal {H}_{1}$–![]() $\mathcal {H}_{3}$) in figure 3(b), as an example. Effects of increasing
$\mathcal {H}_{3}$) in figure 3(b), as an example. Effects of increasing ![]() $Re_{\tau }$ can be accounted for by including taller eddies of height
$Re_{\tau }$ can be accounted for by including taller eddies of height ![]() ${\mathcal {H}}_4 > {\mathcal {H}}_3$ and so on. The wall-normal distributions of
${\mathcal {H}}_4 > {\mathcal {H}}_3$ and so on. The wall-normal distributions of ![]() $I_{ij}$ are inspired by the individual velocity distributions in figure 3(a), and they are used to explain the cumulative contributions to the Reynolds stresses (from the three eddies) at three different probe locations in figure 3(b). For instance, when the probe is positioned at
$I_{ij}$ are inspired by the individual velocity distributions in figure 3(a), and they are used to explain the cumulative contributions to the Reynolds stresses (from the three eddies) at three different probe locations in figure 3(b). For instance, when the probe is positioned at ![]() $z \sim \mathcal {H}_{1}$, all three eddies will contribute to
$z \sim \mathcal {H}_{1}$, all three eddies will contribute to ![]() $\overline {u^2}(z)$ and
$\overline {u^2}(z)$ and ![]() $\overline {v^2}(z)$, while the contribution to
$\overline {v^2}(z)$, while the contribution to ![]() $\overline {w^2}(z)$ and
$\overline {w^2}(z)$ and ![]() $\overline {uw}(z)$ would come solely from the eddy of height
$\overline {uw}(z)$ would come solely from the eddy of height ![]() $\mathcal {H}_{1}$. However, upon increasing the probe location to
$\mathcal {H}_{1}$. However, upon increasing the probe location to ![]() $z \sim \mathcal {H}_{2}$, contributions to the wall-parallel stresses are only made by the tallest two eddies, while those to
$z \sim \mathcal {H}_{2}$, contributions to the wall-parallel stresses are only made by the tallest two eddies, while those to ![]() $\overline {w^2}(z)$ and
$\overline {w^2}(z)$ and ![]() $\overline {uw}(z)$ only come from the eddy of height
$\overline {uw}(z)$ only come from the eddy of height ![]() $\mathcal {H}_{2}$, and so on. Connecting these observations with Townsend's definitions in § 2, the active contributions at any
$\mathcal {H}_{2}$, and so on. Connecting these observations with Townsend's definitions in § 2, the active contributions at any ![]() $z$ can be solely associated with eddies of height,
$z$ can be solely associated with eddies of height, ![]() $\mathcal {H} \sim {O}(z)$, which will contribute to
$\mathcal {H} \sim {O}(z)$, which will contribute to ![]() $u$,
$u$, ![]() $v$,
$v$, ![]() $w$ and hence
$w$ and hence ![]() $uw$, at
$uw$, at ![]() $z$ (indicated by red background shading in figure 3b). On the other hand, the inactive contributions are associated with relatively tall wall-scaled eddies:
$z$ (indicated by red background shading in figure 3b). On the other hand, the inactive contributions are associated with relatively tall wall-scaled eddies: ![]() $ {O}(z) \ll \mathcal {H} \lesssim {O}(\delta )$. These contribute to
$ {O}(z) \ll \mathcal {H} \lesssim {O}(\delta )$. These contribute to ![]() $u(z)$ and
$u(z)$ and ![]() $v(z)$, but not
$v(z)$, but not ![]() $w(z)$ and
$w(z)$ and ![]() $uw(z)$ (indicated by blue background shading).
$uw(z)$ (indicated by blue background shading).
Considering that increasing ![]() $Re_{\tau }$ introduces new eddies that are much taller than
$Re_{\tau }$ introduces new eddies that are much taller than ![]() $z$, only the inactive contributions to
$z$, only the inactive contributions to ![]() $u(z)$ and
$u(z)$ and ![]() $v(z)$ will increase for all
$v(z)$ will increase for all ![]() $z \ll \mathcal {H}$, while the active contributions will exhibit
$z \ll \mathcal {H}$, while the active contributions will exhibit ![]() $Re_{\tau }$-invariance when scaled in wall units (i.e.
$Re_{\tau }$-invariance when scaled in wall units (i.e. ![]() $z$ and
$z$ and ![]() $U_{\tau }$). This explains the contrasting trends exhibited by the Reynolds stresses in (2.1), where
$U_{\tau }$). This explains the contrasting trends exhibited by the Reynolds stresses in (2.1), where ![]() ${\overline {u^2}}^+(z)$ and
${\overline {u^2}}^+(z)$ and ![]() ${\overline {v^2}}^+(z)$ are
${\overline {v^2}}^+(z)$ are ![]() $Re_{\tau }$-dependent while
$Re_{\tau }$-dependent while ![]() ${\overline {w^2}}^+(z)$ and
${\overline {w^2}}^+(z)$ and ![]() ${\overline {uw}}^+(z)$ exhibit
${\overline {uw}}^+(z)$ exhibit ![]() $Re_{\tau }$-invariance in the log region. In summary, both active and inactive motions contribute to the wall-parallel velocity fluctuations, but only the former contribute to the Reynolds shear stresses and
$Re_{\tau }$-invariance in the log region. In summary, both active and inactive motions contribute to the wall-parallel velocity fluctuations, but only the former contribute to the Reynolds shear stresses and ![]() $w$-fluctuations. This is expressed mathematically by the following (Panton Reference Panton2007; Deshpande & Marusic Reference Deshpande and Marusic2021):
$w$-fluctuations. This is expressed mathematically by the following (Panton Reference Panton2007; Deshpande & Marusic Reference Deshpande and Marusic2021):
 \begin{equation} \left. \begin{aligned} {u}(z) & = {u_{a}}(z) + {u_{ia}}(z),\\ {v}(z) & = {v_{a}}(z) + {v_{ia}}(z)\quad \text{and} \\ {w}(z) & = {w_{a}}(z), \end{aligned} \right\} \end{equation}
\begin{equation} \left. \begin{aligned} {u}(z) & = {u_{a}}(z) + {u_{ia}}(z),\\ {v}(z) & = {v_{a}}(z) + {v_{ia}}(z)\quad \text{and} \\ {w}(z) & = {w_{a}}(z), \end{aligned} \right\} \end{equation}
where subscripts ‘![]() ${a}$’ and ‘
${a}$’ and ‘![]() ${ia}$’, respectively, denote active and inactive components. The variances can be decomposed according to the following (Panton Reference Panton2007; Deshpande & Marusic Reference Deshpande and Marusic2021):
${ia}$’, respectively, denote active and inactive components. The variances can be decomposed according to the following (Panton Reference Panton2007; Deshpande & Marusic Reference Deshpande and Marusic2021):
 \begin{equation} \left. \begin{aligned} {{\overline{u^2}}^+} & = {{\overline{u^2_{ia}}}^+} + {{\overline{u^2_{a}}}^+} + 2\overline{{u_{ia}}{u_{a}}},\\ {{\overline{v^2}}^+} & = {{\overline{v^2_{ia}}}^+} + {{\overline{v^2_{a}}}^+} + 2\overline{{v_{ia}}{v_{a}}},\\ {{\overline{w^2}}^+} & = {{\overline{w^2_{a}}}^+} \quad \text{and} \\ {{\overline{uw}}^+} & = {{\overline{{u_{a}}{w}}}^+} + {{\overline{{u_{ia}}{w}}}^+}. \end{aligned} \right\} \end{equation}
\begin{equation} \left. \begin{aligned} {{\overline{u^2}}^+} & = {{\overline{u^2_{ia}}}^+} + {{\overline{u^2_{a}}}^+} + 2\overline{{u_{ia}}{u_{a}}},\\ {{\overline{v^2}}^+} & = {{\overline{v^2_{ia}}}^+} + {{\overline{v^2_{a}}}^+} + 2\overline{{v_{ia}}{v_{a}}},\\ {{\overline{w^2}}^+} & = {{\overline{w^2_{a}}}^+} \quad \text{and} \\ {{\overline{uw}}^+} & = {{\overline{{u_{a}}{w}}}^+} + {{\overline{{u_{ia}}{w}}}^+}. \end{aligned} \right\} \end{equation}
While ![]() $\overline {{u_{ia}}{u_{a}}}$,
$\overline {{u_{ia}}{u_{a}}}$, ![]() $\overline {{v_{ia}}{v_{a}}}$ and
$\overline {{v_{ia}}{v_{a}}}$ and ![]() $\overline {{u_{ia}}{w}} = 0$ for a traditionally conceptualized wall-scaled eddy field (i.e. considering only linear superposition of velocity fluctuations originating from various hierarchies of wall-scaled eddies), that is not true for an actual/real wall-bounded flow (Deshpande et al. Reference Deshpande, Monty and Marusic2021; Deshpande & Marusic Reference Deshpande and Marusic2021). A real TBL also comprises very-large-scale inertial motions/superstructures that are inherently inactive per the definition of Townsend (Deshpande et al. Reference Deshpande, Monty and Marusic2021) and interact nonlinearly with/modulate the eddies local to
$\overline {{u_{ia}}{w}} = 0$ for a traditionally conceptualized wall-scaled eddy field (i.e. considering only linear superposition of velocity fluctuations originating from various hierarchies of wall-scaled eddies), that is not true for an actual/real wall-bounded flow (Deshpande et al. Reference Deshpande, Monty and Marusic2021; Deshpande & Marusic Reference Deshpande and Marusic2021). A real TBL also comprises very-large-scale inertial motions/superstructures that are inherently inactive per the definition of Townsend (Deshpande et al. Reference Deshpande, Monty and Marusic2021) and interact nonlinearly with/modulate the eddies local to ![]() $z$ (Metzger & Klewicki Reference Metzger and Klewicki2001; Mathis et al. Reference Mathis, Marusic, Chernyshenko and Hutchins2013; Baars, Hutchins & Marusic Reference Baars, Hutchins and Marusic2016). The causality between superstructures and nonlinear interactions, however, is a topic of ongoing debate (Andreolli et al. Reference Andreolli, Gatti, Vinuesa, Örlü and Schlatter2023). This, combined with the fact that these nonlinear interactions are smaller in magnitude than individual
$z$ (Metzger & Klewicki Reference Metzger and Klewicki2001; Mathis et al. Reference Mathis, Marusic, Chernyshenko and Hutchins2013; Baars, Hutchins & Marusic Reference Baars, Hutchins and Marusic2016). The causality between superstructures and nonlinear interactions, however, is a topic of ongoing debate (Andreolli et al. Reference Andreolli, Gatti, Vinuesa, Örlü and Schlatter2023). This, combined with the fact that these nonlinear interactions are smaller in magnitude than individual ![]() $\overline {u^2_{ia}}$ and
$\overline {u^2_{ia}}$ and ![]() $\overline {u^2_{a}}$ components (Deshpande & Marusic Reference Deshpande and Marusic2021), makes the investigation of nonlinear interactions beyond the scope of this study. The present study aims to deploy the decomposition methodology facilitating (2.2) on instantaneous flow fields of published data sets, to analyse for the first time the active and inactive contributions to
$\overline {u^2_{a}}$ components (Deshpande & Marusic Reference Deshpande and Marusic2021), makes the investigation of nonlinear interactions beyond the scope of this study. The present study aims to deploy the decomposition methodology facilitating (2.2) on instantaneous flow fields of published data sets, to analyse for the first time the active and inactive contributions to ![]() ${\tau }_w$ and
${\tau }_w$ and ![]() $p_w$. This work builds on the past successes of the decomposition methodology (Deshpande et al. Reference Deshpande, Monty and Marusic2021) that has yielded empirical support for the scaling characteristics of the active and inactive components, hypothesized by Townsend (Reference Townsend1976). This includes the
$p_w$. This work builds on the past successes of the decomposition methodology (Deshpande et al. Reference Deshpande, Monty and Marusic2021) that has yielded empirical support for the scaling characteristics of the active and inactive components, hypothesized by Townsend (Reference Townsend1976). This includes the ![]() $z$-scaling behaviour of
$z$-scaling behaviour of ![]() ${{\overline {u^2_{a}}}^+}$, and the inverse-logarithmic variation of
${{\overline {u^2_{a}}}^+}$, and the inverse-logarithmic variation of ![]() ${{\overline {u^2_{ia}}}^+}$ associated with the
${{\overline {u^2_{ia}}}^+}$ associated with the ![]() $k^{-1}$-scaling (where k represents the streamwise/spanwise wavenumber), to list a few.
$k^{-1}$-scaling (where k represents the streamwise/spanwise wavenumber), to list a few.
3. Data sets and methodology
The present study considers two previously published multi-point data sets across a large ![]() $Re_{\tau }$ range:
$Re_{\tau }$ range: ![]() $ {O}(10^3) \lesssim Re_{\tau } \lesssim {O}(10^6)$, for the SLSE analysis. The low
$ {O}(10^3) \lesssim Re_{\tau } \lesssim {O}(10^6)$, for the SLSE analysis. The low![]() $-Re_{\tau }$ data set is from the high-resolution large-eddy simulation (LES) of a zero-pressure-gradient TBL by Eitel-Amor et al. (Reference Eitel-Amor, Örlü and Schlatter2014), which was computed over a numerical domain large enough for the TBL to evolve up to
$-Re_{\tau }$ data set is from the high-resolution large-eddy simulation (LES) of a zero-pressure-gradient TBL by Eitel-Amor et al. (Reference Eitel-Amor, Örlü and Schlatter2014), which was computed over a numerical domain large enough for the TBL to evolve up to ![]() $Re_{\tau } \approx 2000$. In comparison with a fully resolved direct numerical simulation (DNS), the resolution employed in the LES data is just a factor of 2 coarser in the wall-parallel directions, and approximately 1.5 times coarser along the wall-normal one. Hence, instead of using a conventional subgrid-scale model in the simulation, a small forcing was implemented on the very small scales, essentially to add some extra dissipation. As a consequence, only two minor statistical differences emerge compared with a fully resolved DNS: a slight attenuation of the near-wall peak of
$Re_{\tau } \approx 2000$. In comparison with a fully resolved direct numerical simulation (DNS), the resolution employed in the LES data is just a factor of 2 coarser in the wall-parallel directions, and approximately 1.5 times coarser along the wall-normal one. Hence, instead of using a conventional subgrid-scale model in the simulation, a small forcing was implemented on the very small scales, essentially to add some extra dissipation. As a consequence, only two minor statistical differences emerge compared with a fully resolved DNS: a slight attenuation of the near-wall peak of ![]() ${\overline {u^2}}^+$, and a resolved TKE dissipation 87.2 % of that in a conventional DNS. However, adding the dissipation associated with the forcing recovers 99.8 % of the TKE dissipation. Since the present study predominantly focuses on the correlation between synchronously sampled time series of the desired wall properties (
${\overline {u^2}}^+$, and a resolved TKE dissipation 87.2 % of that in a conventional DNS. However, adding the dissipation associated with the forcing recovers 99.8 % of the TKE dissipation. Since the present study predominantly focuses on the correlation between synchronously sampled time series of the desired wall properties (![]() ${\tau }^+_w$,
${\tau }^+_w$, ![]() ${p}^+_w$) and the overlying flow field (
${p}^+_w$) and the overlying flow field (![]() $u^+$,
$u^+$, ![]() $w^+$), the use of a high-resolution LES instead of DNS does not influence the present conclusions. These correlations are investigated at designated streamwise locations of the numerical domain, corresponding to
$w^+$), the use of a high-resolution LES instead of DNS does not influence the present conclusions. These correlations are investigated at designated streamwise locations of the numerical domain, corresponding to ![]() $Re_{\tau } \approx 500, 1000, 1500$ and 2000. For this, the time series of the LES were sampled across the entire domain cross-section (
$Re_{\tau } \approx 500, 1000, 1500$ and 2000. For this, the time series of the LES were sampled across the entire domain cross-section (![]() $\kern0.06em y-z$), with a resolution of
$\kern0.06em y-z$), with a resolution of ![]() ${\Delta }t^+ \lesssim 0.5$ and for a total eddy-turnover time (
${\Delta }t^+ \lesssim 0.5$ and for a total eddy-turnover time (![]() ${t_{samp}}{U_{\infty }}/{\delta }) \approx 243$, where
${t_{samp}}{U_{\infty }}/{\delta }) \approx 243$, where ![]() $U_\infty$ is the free-stream speed. Combined with the option to ensemble average across the span, these time series are long enough to obtain a sufficiently converged frequency spectrum for capturing the inertial phenomena (demonstrated in Eitel-Amor et al. Reference Eitel-Amor, Örlü and Schlatter2014).
$U_\infty$ is the free-stream speed. Combined with the option to ensemble average across the span, these time series are long enough to obtain a sufficiently converged frequency spectrum for capturing the inertial phenomena (demonstrated in Eitel-Amor et al. Reference Eitel-Amor, Örlü and Schlatter2014).
The high-![]() $Re_{\tau } (\sim {O}(10^6))$ data set is from the neutrally buoyant surface layer at the SLTEST facility in Utah (Marusic & Heuer Reference Marusic and Heuer2007). The data comprise synchronously acquired time series of (
$Re_{\tau } (\sim {O}(10^6))$ data set is from the neutrally buoyant surface layer at the SLTEST facility in Utah (Marusic & Heuer Reference Marusic and Heuer2007). The data comprise synchronously acquired time series of (![]() $u^+$,
$u^+$, ![]() $w^+$) from five sonic anemometers positioned on a vertical tower, within the log region (
$w^+$) from five sonic anemometers positioned on a vertical tower, within the log region (![]() $0.0025 < z/{\delta } < 0.0293$), and wall-shear-stress signals (
$0.0025 < z/{\delta } < 0.0293$), and wall-shear-stress signals (![]() ${\tau }^+_w$) measured using a custom-designed sensor placed vertically below the sonics. These data were acquired at a time resolution of
${\tau }^+_w$) measured using a custom-designed sensor placed vertically below the sonics. These data were acquired at a time resolution of ![]() ${\Delta }t^+ \lesssim 78.4$ and for a total of 175 eddy-turnover times, across a viscous-scaled measuring volume of 1400 (of sonics).
${\Delta }t^+ \lesssim 78.4$ and for a total of 175 eddy-turnover times, across a viscous-scaled measuring volume of 1400 (of sonics).
While the sampling intervals for both LES and SLTEST data sets are not sufficient for fully converging/resolving the very-large-scale phenomena (quantitatively), they have been analysed previously by Deshpande, Vinuesa & Marusic (Reference Deshpande, Vinuesa and Marusic2024) and found to be sufficient to attain an accurate qualitative understanding. This was demonstrated by repeating the same statistical analysis (as presented ahead in § 3.1) on the simultaneous ![]() $u^+,w^+$ and
$u^+,w^+$ and ![]() ${\tau }^+_w$ measurements conducted in the large Melbourne wind tunnel (Deshpande & Marusic Reference Deshpande and Marusic2021), time series for which were sampled for a much longer time interval and at greater frequency. The results and trends derived from the wind-tunnel data were qualitatively consistent with those obtained from the LES and SLTEST data sets, which will serve as the basis for the present study. Both these data sets provide rare access to velocity-fluctuation time series across the log region synchronously with
${\tau }^+_w$ measurements conducted in the large Melbourne wind tunnel (Deshpande & Marusic Reference Deshpande and Marusic2021), time series for which were sampled for a much longer time interval and at greater frequency. The results and trends derived from the wind-tunnel data were qualitatively consistent with those obtained from the LES and SLTEST data sets, which will serve as the basis for the present study. Both these data sets provide rare access to velocity-fluctuation time series across the log region synchronously with ![]() ${\tau }_w$, for TBLs spanning a broad
${\tau }_w$, for TBLs spanning a broad ![]() $Re_{\tau }$ range (
$Re_{\tau }$ range (![]() $10^3$–
$10^3$–![]() $10^6$). This offers a unique opportunity to directly test the hypotheses of Bradshaw (Reference Bradshaw1967) and de Giovanetti et al. (Reference de Giovanetti, Hwang and Choi2016), regarding TKE production and transport mechanisms from the log region to the wall, and to understand how they affect
$10^6$). This offers a unique opportunity to directly test the hypotheses of Bradshaw (Reference Bradshaw1967) and de Giovanetti et al. (Reference de Giovanetti, Hwang and Choi2016), regarding TKE production and transport mechanisms from the log region to the wall, and to understand how they affect ![]() ${\tau }_w$ and
${\tau }_w$ and ![]() $p_w$. Our present conclusions will only depend on the qualitative energy variation across
$p_w$. Our present conclusions will only depend on the qualitative energy variation across ![]() $z$, and not focus on its quantification/scaling (requiring convergence). We note that, although the SLTEST flows are transitionally rough TBLs, the roughness effects are insignificant beyond the roughness sublayer (Klewicki et al. Reference Klewicki, Priyadarshana and Metzger2008; Marusic & Heuer Reference Marusic and Heuer2007). Hence, the roughness will not influence the inertial eddies, which are our primary focus.
$z$, and not focus on its quantification/scaling (requiring convergence). We note that, although the SLTEST flows are transitionally rough TBLs, the roughness effects are insignificant beyond the roughness sublayer (Klewicki et al. Reference Klewicki, Priyadarshana and Metzger2008; Marusic & Heuer Reference Marusic and Heuer2007). Hence, the roughness will not influence the inertial eddies, which are our primary focus.
3.1. Data-driven flow-decomposition methodology
The multi-point nature of both the LES and SLTEST data sets permits theoretical estimation of ![]() $u_{a}$ and
$u_{a}$ and ![]() $u_{ia}$ by following the SLSE-based methodology proposed previously in Deshpande et al. (Reference Deshpande, Monty and Marusic2021) and Deshpande & Marusic (Reference Deshpande and Marusic2021). Throughout this paper, we limit our SLSE analysis to the log region where the concept of active and inactive motions, as well as expressions in (2.1), have received considerable empirical support (Deshpande et al. Reference Deshpande, Monty and Marusic2021). Per the SLSE methodology (Baars et al. Reference Baars, Hutchins and Marusic2016), the instantaneous component
$u_{ia}$ by following the SLSE-based methodology proposed previously in Deshpande et al. (Reference Deshpande, Monty and Marusic2021) and Deshpande & Marusic (Reference Deshpande and Marusic2021). Throughout this paper, we limit our SLSE analysis to the log region where the concept of active and inactive motions, as well as expressions in (2.1), have received considerable empirical support (Deshpande et al. Reference Deshpande, Monty and Marusic2021). Per the SLSE methodology (Baars et al. Reference Baars, Hutchins and Marusic2016), the instantaneous component ![]() $u_{ia}(z)$ in the log region can be obtained by
$u_{ia}(z)$ in the log region can be obtained by
Here, ![]() ${\widetilde {u_{\tau }}}(T^+) = \sqrt {{\widetilde {{\tau }_{w}}}/{\rho }} = \mathcal {F}(u_{\tau }(t^+))$ is essentially the Fourier transform of the friction velocity fluctuations,
${\widetilde {u_{\tau }}}(T^+) = \sqrt {{\widetilde {{\tau }_{w}}}/{\rho }} = \mathcal {F}(u_{\tau }(t^+))$ is essentially the Fourier transform of the friction velocity fluctuations, ![]() $u_{\tau }(t^+)$, in time
$u_{\tau }(t^+)$, in time ![]() $t$, where
$t$, where ![]() $\rho$ is density. The parameter
$\rho$ is density. The parameter ![]() ${\widetilde {u_{\tau }}}$ acts as the scale-specific unconditional input required to obtain the scale-specific conditional output,
${\widetilde {u_{\tau }}}$ acts as the scale-specific unconditional input required to obtain the scale-specific conditional output, ![]() ${\widetilde {u_{ia}}}(z^{+};{T^{+}})$. The linear relationship between
${\widetilde {u_{ia}}}(z^{+};{T^{+}})$. The linear relationship between ![]() $\widetilde {u_{ia}}$ and
$\widetilde {u_{ia}}$ and ![]() $\widetilde {u_{\tau }}$ is inspired by the original definitions of active and inactive motions derived from the wall-scaled eddy model (refer § 2), which assumes a linear superposition of the velocity signatures generated by individual eddies. Further,
$\widetilde {u_{\tau }}$ is inspired by the original definitions of active and inactive motions derived from the wall-scaled eddy model (refer § 2), which assumes a linear superposition of the velocity signatures generated by individual eddies. Further, ![]() $H_{L}$ is the complex-valued linear transfer kernel reconstructed by cross-correlating the synchronously acquired
$H_{L}$ is the complex-valued linear transfer kernel reconstructed by cross-correlating the synchronously acquired ![]() $\tilde {u}(z^+)$ and
$\tilde {u}(z^+)$ and ![]() $\widetilde {u_{\tau }}$ according to the following:
$\widetilde {u_{\tau }}$ according to the following:
where the curly brackets (![]() $\{{\cdot } \}$) and asterisk (
$\{{\cdot } \}$) and asterisk (![]() $\ast$) denote ensemble averaging and complex conjugate, respectively. Here, the definition of
$\ast$) denote ensemble averaging and complex conjugate, respectively. Here, the definition of ![]() $H_{L}$ is underpinned by the discussion presented in § 2 and figure 3 that the inactive components coexisting at
$H_{L}$ is underpinned by the discussion presented in § 2 and figure 3 that the inactive components coexisting at ![]() $z^+$ extend down to the wall (i.e. influence
$z^+$ extend down to the wall (i.e. influence ![]() $u_{\tau }$), while the active components are localized to
$u_{\tau }$), while the active components are localized to ![]() $z$. Hence, (3.1) and (3.2) essentially classify
$z$. Hence, (3.1) and (3.2) essentially classify ![]() $\widetilde {u_{ia}}$ as a subset of the total momentum
$\widetilde {u_{ia}}$ as a subset of the total momentum ![]() $\tilde {u}$ that is coherent with
$\tilde {u}$ that is coherent with ![]() $\widetilde {u_{\tau }}$. Figure 4(a,b) depicts
$\widetilde {u_{\tau }}$. Figure 4(a,b) depicts ![]() $|H_{L}|({z^+}, T^+)$ computed from the LES and SLTEST data sets at
$|H_{L}|({z^+}, T^+)$ computed from the LES and SLTEST data sets at ![]() $z^+$ within the log region, alongside the premultiplied spectra of
$z^+$ within the log region, alongside the premultiplied spectra of ![]() $u_{\tau }$ (
$u_{\tau }$ (![]() $\,{f}{{\phi }^+_{{u_{\tau }}{u_{\tau }}}}$), where
$\,{f}{{\phi }^+_{{u_{\tau }}{u_{\tau }}}}$), where ![]() $|{{\cdot }}|$ represents the modulus. Before plotting,
$|{{\cdot }}|$ represents the modulus. Before plotting, ![]() $|H_{L}|$ has been smoothed based on a 25 % bandwidth moving filter (Baars et al. Reference Baars, Hutchins and Marusic2016). This is done to remove the noise emerging from the mathematical operations in (3.2), which are conducted on a per-scale basis. It is evident that
$|H_{L}|$ has been smoothed based on a 25 % bandwidth moving filter (Baars et al. Reference Baars, Hutchins and Marusic2016). This is done to remove the noise emerging from the mathematical operations in (3.2), which are conducted on a per-scale basis. It is evident that ![]() $|H_L|$ is non-zero across a broadband range of
$|H_L|$ is non-zero across a broadband range of ![]() $T^+$ when
$T^+$ when ![]() $z$ is close to the lower bound of the log region. However,
$z$ is close to the lower bound of the log region. However, ![]() $|H_L|$ gets restricted to relatively large scales with an increase in
$|H_L|$ gets restricted to relatively large scales with an increase in ![]() $z^+$, essentially acting as a
$z^+$, essentially acting as a ![]() $z$-dependent low-pass filter conforming to larger scales with increasing
$z$-dependent low-pass filter conforming to larger scales with increasing ![]() $z$, in a manner consistent with Townsend's wall-scaled eddy hypothesis (refer § 2). These trends also align with the
$z$, in a manner consistent with Townsend's wall-scaled eddy hypothesis (refer § 2). These trends also align with the ![]() $|H_L|$ computed previously using long time-series signals from the Melbourne wind-tunnel data (Deshpande & Marusic Reference Deshpande and Marusic2021; Deshpande et al. Reference Deshpande, Vinuesa and Marusic2024), confirming they are physical and not artefacts of insufficient convergence.
$|H_L|$ computed previously using long time-series signals from the Melbourne wind-tunnel data (Deshpande & Marusic Reference Deshpande and Marusic2021; Deshpande et al. Reference Deshpande, Vinuesa and Marusic2024), confirming they are physical and not artefacts of insufficient convergence.

Figure 4. Absolute values of the linear transfer kernel (![]() $|H_L|(z^+;T^+$); (3.2)) computed for the (a) LES and (b) SLTEST data with grey shading representing changing
$|H_L|(z^+;T^+$); (3.2)) computed for the (a) LES and (b) SLTEST data with grey shading representing changing ![]() $z^+$. Here,
$z^+$. Here, ![]() $|H_L|$ is plotted for
$|H_L|$ is plotted for ![]() $z^+$ limited to the log region and its ordinate is considered on the primary vertical axis (left; in black) while the solid golden line represents the premultiplied spectra of the friction velocity (
$z^+$ limited to the log region and its ordinate is considered on the primary vertical axis (left; in black) while the solid golden line represents the premultiplied spectra of the friction velocity (![]() $\,f{{\phi }^+_{{u_{\tau }}{u_{\tau }}}}$) and its ordinate is considered on the secondary vertical axis (right; in golden yellow). (c,d) Premultiplied spectra of the active (
$\,f{{\phi }^+_{{u_{\tau }}{u_{\tau }}}}$) and its ordinate is considered on the secondary vertical axis (right; in golden yellow). (c,d) Premultiplied spectra of the active (![]() $u_{a}$) and inactive
$u_{a}$) and inactive ![]() $u$-components (
$u$-components (![]() $u_{ia}$) estimated for various
$u_{ia}$) estimated for various ![]() $z$-locations in the log region for both the LES and SLTEST data. Note that the horizontal axis is chosen to test for
$z$-locations in the log region for both the LES and SLTEST data. Note that the horizontal axis is chosen to test for ![]() $z$-scaling of the spectra by invoking Taylor's hypothesis. Panels show data for (a)
$z$-scaling of the spectra by invoking Taylor's hypothesis. Panels show data for (a) ![]() $Re_{\tau } \approx 2000$, (b)
$Re_{\tau } \approx 2000$, (b) ![]() $Re_{\tau } \approx {O}(10^6)$, (c,d) both
$Re_{\tau } \approx {O}(10^6)$, (c,d) both ![]() $Re_{\tau}\approx 2000$ and
$Re_{\tau}\approx 2000$ and ![]() $Re_{\tau}\approx O(10^6)$.
$Re_{\tau}\approx O(10^6)$.
Significantly, for both the LES and SLTEST data, ![]() $|H_{L}|$ extends to sufficiently large
$|H_{L}|$ extends to sufficiently large ![]() $T^+$ values where the corresponding
$T^+$ values where the corresponding ![]() $f{{\phi }^+_{{u_{\tau }}{u_{\tau }}}}$ is negligible beyond the considered range. This ensures proper estimation of the very-large-scale
$f{{\phi }^+_{{u_{\tau }}{u_{\tau }}}}$ is negligible beyond the considered range. This ensures proper estimation of the very-large-scale ![]() ${\widetilde {u_{ia}}}$ signal. Similarly, the time resolution and frequency response of the wall-shear-stress sensor at high
${\widetilde {u_{ia}}}$ signal. Similarly, the time resolution and frequency response of the wall-shear-stress sensor at high ![]() $Re_{\tau }$, despite limiting the measurement of the wall-shear-stress spectrum to
$Re_{\tau }$, despite limiting the measurement of the wall-shear-stress spectrum to ![]() $T^+ \gtrsim$ 1000 (figures 1a and 4b), is sufficient to resolve
$T^+ \gtrsim$ 1000 (figures 1a and 4b), is sufficient to resolve ![]() ${\widetilde {u_{ia}}}$ considering
${\widetilde {u_{ia}}}$ considering ![]() $|H_{L}| \sim 0$ at
$|H_{L}| \sim 0$ at ![]() $T^+ \lesssim 1000$. The efficacy of extracting the inactive contributions is demonstrated by examining the scaling characteristics of the premultiplied
$T^+ \lesssim 1000$. The efficacy of extracting the inactive contributions is demonstrated by examining the scaling characteristics of the premultiplied ![]() $u_{ia}$-spectra (
$u_{ia}$-spectra (![]() $\,f{{\phi }^+_{{u_{ia}}{u_{ia}}}}$; also see Deshpande et al. Reference Deshpande, Monty and Marusic2021). Figure 4(d) depicts
$\,f{{\phi }^+_{{u_{ia}}{u_{ia}}}}$; also see Deshpande et al. Reference Deshpande, Monty and Marusic2021). Figure 4(d) depicts ![]() $f{{\phi }^+_{{u_{ia}}{u_{ia}}}}$ estimated for various
$f{{\phi }^+_{{u_{ia}}{u_{ia}}}}$ estimated for various ![]() $z/{\delta }$ in the log region for the two data sets, and it is plotted against
$z/{\delta }$ in the log region for the two data sets, and it is plotted against ![]() $z$-scaled streamwise wavelengths (
$z$-scaled streamwise wavelengths (![]() ${T^+}{U^+}(z^+)/z^+$) computed based on Taylor's hypothesis (well accepted for inertial scales below 6
${T^+}{U^+}(z^+)/z^+$) computed based on Taylor's hypothesis (well accepted for inertial scales below 6![]() $\delta$; Dennis & Nickels Reference Dennis and Nickels2008). Considering that inactive contributions at any
$\delta$; Dennis & Nickels Reference Dennis and Nickels2008). Considering that inactive contributions at any ![]() $z$ are associated with wall-scaled eddies across
$z$ are associated with wall-scaled eddies across ![]() $ {O}(z) \lesssim \mathcal {H} \lesssim {O}(\delta )$,
$ {O}(z) \lesssim \mathcal {H} \lesssim {O}(\delta )$, ![]() $f{{\phi }^+_{{u_{ia}}{u_{ia}}}}$ is expected to exhibit
$f{{\phi }^+_{{u_{ia}}{u_{ia}}}}$ is expected to exhibit ![]() $z$-scaling only in the relatively small-scale range, consistent with the trends in figure 4(d). Further, it is evident that the relatively large-scale contributions to
$z$-scaling only in the relatively small-scale range, consistent with the trends in figure 4(d). Further, it is evident that the relatively large-scale contributions to ![]() $f{{\phi }^+_{{u_{ia}}{u_{ia}}}}$ decrease with
$f{{\phi }^+_{{u_{ia}}{u_{ia}}}}$ decrease with ![]() $z/{\delta }$ across both data sets, which is also consistent with the inverse-logarithmic variation of
$z/{\delta }$ across both data sets, which is also consistent with the inverse-logarithmic variation of ![]() $\overline {u^2_{ia}}$ expected based on the wall-scaled eddy hypothesis (refer to § 2 and Deshpande et al. Reference Deshpande, Monty and Marusic2021).
$\overline {u^2_{ia}}$ expected based on the wall-scaled eddy hypothesis (refer to § 2 and Deshpande et al. Reference Deshpande, Monty and Marusic2021).
Once ![]() ${\widetilde {u_{ia}}}$ is obtained via (3.1), its time-domain equivalent can be calculated simply by taking the inverse Fourier transform (Deshpande & Marusic Reference Deshpande and Marusic2021)
${\widetilde {u_{ia}}}$ is obtained via (3.1), its time-domain equivalent can be calculated simply by taking the inverse Fourier transform (Deshpande & Marusic Reference Deshpande and Marusic2021) ![]() ${{{u}_{ia}}(z^{+}; {t^{+}})} = {\mathcal {F}^{-1}}({{\widetilde {{u}_{ia}}}(z^{+};{T^{+}})})$. Considering the discussion on the past hypotheses in § 2, the novel analysis here is not to establish the correlation of
${{{u}_{ia}}(z^{+}; {t^{+}})} = {\mathcal {F}^{-1}}({{\widetilde {{u}_{ia}}}(z^{+};{T^{+}})})$. Considering the discussion on the past hypotheses in § 2, the novel analysis here is not to establish the correlation of ![]() $u_{ia}$ with
$u_{ia}$ with ![]() ${\tau }_w$ (which is imposed by definition in (3.1)), but to investigate the variation of simultaneously acquired
${\tau }_w$ (which is imposed by definition in (3.1)), but to investigate the variation of simultaneously acquired ![]() $u_{ia}$-signals across various
$u_{ia}$-signals across various ![]() $z$-locations in the log region (see § 4.1). Estimation of
$z$-locations in the log region (see § 4.1). Estimation of ![]() $u_{ia}$ also permits calculation of the
$u_{ia}$ also permits calculation of the ![]() $u_{a}$-time series by simple subtraction,
$u_{a}$-time series by simple subtraction, ![]() ${{{u}_{a}}(z^{+};{t^{+}})} = {{{u}}(z^{+};{t^{+}})}$ –
${{{u}_{a}}(z^{+};{t^{+}})} = {{{u}}(z^{+};{t^{+}})}$ – ![]() ${{{u}_{ia}}(z^{+};{t^{+}})}$, thereby associating
${{{u}_{ia}}(z^{+};{t^{+}})}$, thereby associating ![]() $u_{a}$ with the
$u_{a}$ with the ![]() $u$-subset that is incoherent with the wall (i.e.
$u$-subset that is incoherent with the wall (i.e. ![]() $u_{\tau }$). Similar to the investigation of
$u_{\tau }$). Similar to the investigation of ![]() $f{{\phi }^+_{{u_{ia}}{u_{ia}}}}$, we can examine the premultiplied
$f{{\phi }^+_{{u_{ia}}{u_{ia}}}}$, we can examine the premultiplied ![]() $u_{a}$-spectrum (
$u_{a}$-spectrum (![]() $\,f{{\phi }^+_{{u_{a}}{u_{a}}}}$) for its expected scaling arguments. Per Townsend's (Reference Townsend1976) hypothesis, active contributions at
$\,f{{\phi }^+_{{u_{a}}{u_{a}}}}$) for its expected scaling arguments. Per Townsend's (Reference Townsend1976) hypothesis, active contributions at ![]() $z$ are solely associated with wall-scaled eddies of
$z$ are solely associated with wall-scaled eddies of ![]() $\mathcal {H} \sim {O}(z)$, suggesting
$\mathcal {H} \sim {O}(z)$, suggesting ![]() $f{{\phi }^+_{{u_{a}}{u_{a}}}}$ should exhibit
$f{{\phi }^+_{{u_{a}}{u_{a}}}}$ should exhibit ![]() $z$-scaling irrespective of the flow
$z$-scaling irrespective of the flow ![]() $Re_{\tau }$. Figure 4(c) depicts reasonably good
$Re_{\tau }$. Figure 4(c) depicts reasonably good ![]() $z$-scaling of the premultiplied
$z$-scaling of the premultiplied ![]() $u_{a}$-spectra across
$u_{a}$-spectra across ![]() $ {O}(10^3) \lesssim Re_{\tau } \lesssim {O}(10^6)$, consistent with the previous findings in Deshpande et al. (Reference Deshpande, Monty and Marusic2021). This ability to estimate
$ {O}(10^3) \lesssim Re_{\tau } \lesssim {O}(10^6)$, consistent with the previous findings in Deshpande et al. (Reference Deshpande, Monty and Marusic2021). This ability to estimate ![]() $u_{a}$ enables computation of the Reynolds shear stresses associated exclusively with the active motions (
$u_{a}$ enables computation of the Reynolds shear stresses associated exclusively with the active motions (![]() ${u_{a}}w(z^+; t^+)$), which should correspond closely with the net Reynolds shear stresses (
${u_{a}}w(z^+; t^+)$), which should correspond closely with the net Reynolds shear stresses (![]() $uw(z^+; t^+)$) per Townsend's (Reference Townsend1961) hypothesis. These hypotheses are tested in this study using simultaneously acquired time-series signals, across the log region, for the first time (see § 4.1).
$uw(z^+; t^+)$) per Townsend's (Reference Townsend1961) hypothesis. These hypotheses are tested in this study using simultaneously acquired time-series signals, across the log region, for the first time (see § 4.1).
4. Results and discussions
4.1. Active and inactive contributions to the wall-shear stress
This section focuses on responding to research question (i) raised in § 1, regarding the role played by inactive motions in transporting large-scale energy from the outer region to the wall (to explain ![]() ${\tau }_w$-signatures at large
${\tau }_w$-signatures at large ![]() $T^+$). Figure 5(a, f) plots a small subset of the full
$T^+$). Figure 5(a, f) plots a small subset of the full ![]() $u^+$-time series sampled in the log region, synchronously with that of
$u^+$-time series sampled in the log region, synchronously with that of ![]() ${\tau }^+_w$, from the LES and SLTEST data sets, respectively. These data are used to obtain the corresponding active (figure 5c,h) and inactive components (figure 5e,j) of
${\tau }^+_w$, from the LES and SLTEST data sets, respectively. These data are used to obtain the corresponding active (figure 5c,h) and inactive components (figure 5e,j) of ![]() $u^+$ by following the procedure described in § 3.1. As expected based on (3.1),
$u^+$ by following the procedure described in § 3.1. As expected based on (3.1), ![]() $u_{ia}$-signals correspond predominantly to low-frequency features (i.e. large
$u_{ia}$-signals correspond predominantly to low-frequency features (i.e. large ![]() $T^+$) that are highly correlated with
$T^+$) that are highly correlated with ![]() ${\tau }_w$, while
${\tau }_w$, while ![]() $u_{a}$-signals are representative of the intermediate-frequency phenomena that are uncorrelated with
$u_{a}$-signals are representative of the intermediate-frequency phenomena that are uncorrelated with ![]() ${\tau }_w$. This, however, should not be misinterpreted to imply negligible contributions of intermediate-scaled coherent structures to
${\tau }_w$. This, however, should not be misinterpreted to imply negligible contributions of intermediate-scaled coherent structures to ![]() ${\tau }_w$. Considering that the active–inactive classification is relative to
${\tau }_w$. Considering that the active–inactive classification is relative to ![]() $z$ (refer § 2), subsets of the structures that are active at
$z$ (refer § 2), subsets of the structures that are active at ![]() $z \sim z_1$ would be associated with inactive contributions much closer to the wall (
$z \sim z_1$ would be associated with inactive contributions much closer to the wall (![]() $z \ll z_1$), and consequently influence
$z \ll z_1$), and consequently influence ![]() ${\tau }_w$ from that location. Also plotted in figure 5(b,g) are the
${\tau }_w$ from that location. Also plotted in figure 5(b,g) are the ![]() $w^+$-signals corresponding to the same instants as those considered for the
$w^+$-signals corresponding to the same instants as those considered for the ![]() $u^+$-signals. Notably, the
$u^+$-signals. Notably, the ![]() $w$-signals exhibit the same characteristics as those of
$w$-signals exhibit the same characteristics as those of ![]() $u_{a}$ across the log region. This is consistent with Townsend's description of
$u_{a}$ across the log region. This is consistent with Townsend's description of ![]() $u_{a}$ and
$u_{a}$ and ![]() $w$ being associated with wall-scaled eddies local to
$w$ being associated with wall-scaled eddies local to ![]() $z$ (i.e.
$z$ (i.e. ![]() $\mathcal {H} \sim z$), while
$\mathcal {H} \sim z$), while ![]() $u_{ia}$ corresponds to relatively taller and larger wall-scaled eddies (
$u_{ia}$ corresponds to relatively taller and larger wall-scaled eddies (![]() $ {O}(z) \ll \mathcal {H} \lesssim {O}(\delta )$) whose contributions extend to the wall (refer § 2). It is worth noting here that active motions are deemed ‘local’ relative to their distance from the wall (i.e.
$ {O}(z) \ll \mathcal {H} \lesssim {O}(\delta )$) whose contributions extend to the wall (refer § 2). It is worth noting here that active motions are deemed ‘local’ relative to their distance from the wall (i.e. ![]() $z \gg 0$). This should not be confused with the well-known localized behaviour of the Kolmogorov scales, which are spatially much smaller than the active–inactive motions (the latter being subsets of inertia-dominated motions). Hence, the active motions are bound to have a notable wall-normal coherence despite their ‘localization’, which is evident through the simultaneously acquired
$z \gg 0$). This should not be confused with the well-known localized behaviour of the Kolmogorov scales, which are spatially much smaller than the active–inactive motions (the latter being subsets of inertia-dominated motions). Hence, the active motions are bound to have a notable wall-normal coherence despite their ‘localization’, which is evident through the simultaneously acquired ![]() $u_a$- and
$u_a$- and ![]() $w$-signals across the log region in figure 5.
$w$-signals across the log region in figure 5.

Figure 5. Instantaneous (a, f) streamwise and (b,g) amplified wall-normal velocity fluctuations (in black) at various ![]() $z^+$ decomposed into their corresponding (c,h) active (
$z^+$ decomposed into their corresponding (c,h) active (![]() $u^+_{a}$; in red) and (e,j) inactive (
$u^+_{a}$; in red) and (e,j) inactive (![]() $u^+_{ia}$; in blue) components via the SLSE-based methodology discussed in § 3.1. Note the different vertical offsets for various time series as well as different ordinate ranges. (d,i) Compare the time series of the full momentum flux (
$u^+_{ia}$; in blue) components via the SLSE-based methodology discussed in § 3.1. Note the different vertical offsets for various time series as well as different ordinate ranges. (d,i) Compare the time series of the full momentum flux (![]() ${u^+}{w^+}$; in black) and that associated with the active motions (
${u^+}{w^+}$; in black) and that associated with the active motions (![]() ${u^+_{a}}{w^+}$; in red). Dark to light shading indicates increase in
${u^+_{a}}{w^+}$; in red). Dark to light shading indicates increase in ![]() $z/{\delta }$ across all plots. The synchronously acquired time series of the wall-shear-stress fluctuations (
$z/{\delta }$ across all plots. The synchronously acquired time series of the wall-shear-stress fluctuations (![]() ${\tau }^{+}_{w}$; in golden) have been plotted for: (a–e)
${\tau }^{+}_{w}$; in golden) have been plotted for: (a–e) ![]() $Re_{\tau } \approx 2000$ (LES) and ( f–j)
$Re_{\tau } \approx 2000$ (LES) and ( f–j) ![]() $Re_{\tau } \approx {O}(10^6)$ (SLTEST) data sets and has been intentionally amplified for clarity. Background green shading in (d,e,i,j) highlights portions of the time series associated with relatively high magnitudes of
$Re_{\tau } \approx {O}(10^6)$ (SLTEST) data sets and has been intentionally amplified for clarity. Background green shading in (d,e,i,j) highlights portions of the time series associated with relatively high magnitudes of ![]() $u^+_{ia}$, which increase with decreasing
$u^+_{ia}$, which increase with decreasing ![]() $z/{\delta }$ indicating downward momentum transfer.
$z/{\delta }$ indicating downward momentum transfer.
Townsend's (Reference Townsend1976) hypothesis is mainly centred on the fact that the active components are solely responsible for the shear stresses and TKE production across the log region – this is clearly evident from figure 5(d,i) comparing ![]() ${u_{a}}w$ (in red) with
${u_{a}}w$ (in red) with ![]() $uw$ (in thicker black lines). The good overlap of the two signals, across
$uw$ (in thicker black lines). The good overlap of the two signals, across ![]() $10^3 \lesssim Re_{\tau } \lesssim 10^6$, is a testament to the capability of the SLSE-based methodology of extracting the active components from simultaneously acquired signals across the log region. The observations from figure 5(i) have been reaffirmed statistically in figure 6(a), where we compare the premultiplied co-spectra of the full Reynolds shear stress (
$10^3 \lesssim Re_{\tau } \lesssim 10^6$, is a testament to the capability of the SLSE-based methodology of extracting the active components from simultaneously acquired signals across the log region. The observations from figure 5(i) have been reaffirmed statistically in figure 6(a), where we compare the premultiplied co-spectra of the full Reynolds shear stress (![]() $\,{f}{{\phi }^+_{uw}}$) with that associated solely with the active component (
$\,{f}{{\phi }^+_{uw}}$) with that associated solely with the active component (![]() $\,{f}{{\phi }^+_{{u_{a}}w}}$), across various
$\,{f}{{\phi }^+_{{u_{a}}w}}$), across various ![]() $z/{\delta }$ for the
$z/{\delta }$ for the ![]() $Re_{\tau } \sim {O}(10^6)$ data set. The horizontal axis in this figure has been chosen to test for
$Re_{\tau } \sim {O}(10^6)$ data set. The horizontal axis in this figure has been chosen to test for ![]() $z$-scaling of the co-spectra, by invoking Taylor's hypothesis (Dennis & Nickels Reference Dennis and Nickels2008) similar to figure 4(c,d). In figure 6(a),
$z$-scaling of the co-spectra, by invoking Taylor's hypothesis (Dennis & Nickels Reference Dennis and Nickels2008) similar to figure 4(c,d). In figure 6(a), ![]() ${f}{{\phi }^+_{uw}}$ and
${f}{{\phi }^+_{uw}}$ and ![]() ${f}{{\phi }^+_{{u_{a}}w}}$ agree very well in the intermediate-scale range for all
${f}{{\phi }^+_{{u_{a}}w}}$ agree very well in the intermediate-scale range for all ![]() $z/{\delta }$, with the co-spectrum peak exhibiting wall scaling at
$z/{\delta }$, with the co-spectrum peak exhibiting wall scaling at ![]() ${T^+}{U^+}(z^+) \approx 15z^+$. This reinforces the association of
${T^+}{U^+}(z^+) \approx 15z^+$. This reinforces the association of ![]() $u_{a}$ with the active components per the definition of Townsend (Reference Townsend1976).
$u_{a}$ with the active components per the definition of Townsend (Reference Townsend1976).

Figure 6. (a) Premultiplied co-spectra of the full Reynolds shear stress (![]() $\,{f}{{\phi }^+_{uw}}$; in black) compared against those associated with the active component (
$\,{f}{{\phi }^+_{uw}}$; in black) compared against those associated with the active component (![]() $\,{f}{{\phi }^+_{{u_{a}}w}}$; in red) at various
$\,{f}{{\phi }^+_{{u_{a}}w}}$; in red) at various ![]() $z/{\delta }$. Spectrum profiles for increasing
$z/{\delta }$. Spectrum profiles for increasing ![]() $z/{\delta }$ are vertically offset by 0.2 for convenience of comparison. Dashed green line indicates
$z/{\delta }$ are vertically offset by 0.2 for convenience of comparison. Dashed green line indicates ![]() $z$-scaling of the co-spectrum peak at
$z$-scaling of the co-spectrum peak at ![]() ${T^+}{U^+}(z^+) \approx 15z$. (b) Cross-correlation between
${T^+}{U^+}(z^+) \approx 15z$. (b) Cross-correlation between ![]() $u_{ia}$ at
$u_{ia}$ at ![]() $z_{ref} = 0.0025\delta$ and
$z_{ref} = 0.0025\delta$ and ![]() $z > z_{ref}$, with the maximum
$z > z_{ref}$, with the maximum ![]() $R_{{u_{ia}}{u_{ia}}}$ highlighted by green circles.
$R_{{u_{ia}}{u_{ia}}}$ highlighted by green circles.
There are, however, slight disagreements between ![]() ${f}{{\phi }^+_{uw}}$ and
${f}{{\phi }^+_{uw}}$ and ![]() ${f}{{\phi }^+_{{u_{a}}w}}$ at the larger scales. This can be associated with the differences observed between the
${f}{{\phi }^+_{{u_{a}}w}}$ at the larger scales. This can be associated with the differences observed between the ![]() ${u_{a}}w$- and
${u_{a}}w$- and ![]() $uw$-time series in figure 5(d,i), at instants indicated by a green background. These are representative of the nonlinear interactions between the active motions and inactive superstructures (i.e.
$uw$-time series in figure 5(d,i), at instants indicated by a green background. These are representative of the nonlinear interactions between the active motions and inactive superstructures (i.e. ![]() ${u_{ia}}w$), which become non-negligible only at time instants corresponding to high-amplitude signatures in
${u_{ia}}w$), which become non-negligible only at time instants corresponding to high-amplitude signatures in ![]() $u_{ia}$ (also highlighted by the green background in figure 5e,j). Notably, the magnitudes of these nonlinear interactions (i.e.
$u_{ia}$ (also highlighted by the green background in figure 5e,j). Notably, the magnitudes of these nonlinear interactions (i.e. ![]() ${u_{ia}}w$) are nominally of the same magnitude at
${u_{ia}}w$) are nominally of the same magnitude at ![]() $Re_{\tau } \approx 2000$ and
$Re_{\tau } \approx 2000$ and ![]() $ {O}(10^6)$, across various
$ {O}(10^6)$, across various ![]() $z/{\delta }$, which is consistent with the weak
$z/{\delta }$, which is consistent with the weak ![]() $Re_{\tau }$-dependence of the inter-scale/nonlinear interactions reported by Mathis, Hutchins & Marusic (Reference Mathis, Hutchins and Marusic2009) across the log region. Hence, figure 6(a) reaffirms that the nonlinear interactions between the active and inactive components contribute towards a minor subset of the Reynolds shear stresses (and consequently, the
$Re_{\tau }$-dependence of the inter-scale/nonlinear interactions reported by Mathis, Hutchins & Marusic (Reference Mathis, Hutchins and Marusic2009) across the log region. Hence, figure 6(a) reaffirms that the nonlinear interactions between the active and inactive components contribute towards a minor subset of the Reynolds shear stresses (and consequently, the ![]() $\overline {u^2}$ production), associated with the very large scales. These contributions, however, cannot be explained based on Townsend's wall-scaled eddy framework and is a shortcoming, requiring consideration in future upgrades of the model/framework.
$\overline {u^2}$ production), associated with the very large scales. These contributions, however, cannot be explained based on Townsend's wall-scaled eddy framework and is a shortcoming, requiring consideration in future upgrades of the model/framework.
Another notable observation from figure 5(e,j) is the discernible increase in ![]() $u_{ia}$-fluctuation magnitudes with decreasing
$u_{ia}$-fluctuation magnitudes with decreasing ![]() $z/{\delta }$, which can be compared based on the dashed grey lines representing zero magnitude (for signals at each
$z/{\delta }$, which can be compared based on the dashed grey lines representing zero magnitude (for signals at each ![]() $z$ location). This suggests a wall-ward transport of streamwise momentum by the inactive components (indicated by the dashed black arrows) that is locally produced at each
$z$ location). This suggests a wall-ward transport of streamwise momentum by the inactive components (indicated by the dashed black arrows) that is locally produced at each ![]() $z$ by the active component (conceptualized in figure 2c–e). Since the correlation between
$z$ by the active component (conceptualized in figure 2c–e). Since the correlation between ![]() $u_{ia}$ and
$u_{ia}$ and ![]() ${\tau }_w$ has already been quantified by
${\tau }_w$ has already been quantified by ![]() $|H_{L}|$ via (3.2), we know that this transport occurs all the way down to the wall. The novel result here is the time-synchronized increment in
$|H_{L}|$ via (3.2), we know that this transport occurs all the way down to the wall. The novel result here is the time-synchronized increment in ![]() $u_{ia}$ magnitude with decreasing
$u_{ia}$ magnitude with decreasing ![]() $z$, which is revealed only after the flow decomposition. It presents direct evidence of the non-local transfer of momentum/energy in the wall-normal direction across a broad
$z$, which is revealed only after the flow decomposition. It presents direct evidence of the non-local transfer of momentum/energy in the wall-normal direction across a broad ![]() $Re_{\tau }$-range, which was previously noted only via spectral distributions at low
$Re_{\tau }$-range, which was previously noted only via spectral distributions at low ![]() $Re_{\tau }$ (Cho et al. Reference Cho, Hwang and Choi2018; Lee & Moser Reference Lee and Moser2019). The average time delay/offset for the increase in
$Re_{\tau }$ (Cho et al. Reference Cho, Hwang and Choi2018; Lee & Moser Reference Lee and Moser2019). The average time delay/offset for the increase in ![]() $u_{ia}$ magnitude, with decreasing
$u_{ia}$ magnitude, with decreasing ![]() $z$, can be estimated by computing the two-point correlation (
$z$, can be estimated by computing the two-point correlation (![]() $R_{{u_{ia}}{u_{ia}}}$) defined as follows:
$R_{{u_{ia}}{u_{ia}}}$) defined as follows:
 \begin{equation} R_{{u_{ia}}{u_{ia}}}({z},{z_{ref}};{\Delta}t) = \frac{ \overline{ {{u_{ia}}({z_{ref}} \approx 0.0025{\delta}, {t})}{{u_{ia}}({z}, {t} + {\Delta}t)} } } { \sqrt{ \overline{{u^2_{ia}}({z_{ref}} \approx 0.0025{\delta}) }} \sqrt{ \overline{{u^2_{ia}}({z}) }} }. \end{equation}
\begin{equation} R_{{u_{ia}}{u_{ia}}}({z},{z_{ref}};{\Delta}t) = \frac{ \overline{ {{u_{ia}}({z_{ref}} \approx 0.0025{\delta}, {t})}{{u_{ia}}({z}, {t} + {\Delta}t)} } } { \sqrt{ \overline{{u^2_{ia}}({z_{ref}} \approx 0.0025{\delta}) }} \sqrt{ \overline{{u^2_{ia}}({z}) }} }. \end{equation}
Figure 6(b) depicts ![]() $R_{{u_{ia}}{u_{ia}}}$ for the
$R_{{u_{ia}}{u_{ia}}}$ for the ![]() $Re_{\tau } \sim {O}(10^6)$ data at various
$Re_{\tau } \sim {O}(10^6)$ data at various ![]() $z > z_{ref}$. It is evident that the maxima of
$z > z_{ref}$. It is evident that the maxima of ![]() $R_{{u_{ia}}{u_{ia}}}$, which are highlighted by green circles, are found for a non-zero
$R_{{u_{ia}}{u_{ia}}}$, which are highlighted by green circles, are found for a non-zero ![]() $|{\Delta }t|$ that increases with increasing
$|{\Delta }t|$ that increases with increasing ![]() $z/{\delta }$. This can be interpreted as the average increase in time needed for the wall-normal energy transport, from
$z/{\delta }$. This can be interpreted as the average increase in time needed for the wall-normal energy transport, from ![]() $z$ to
$z$ to ![]() $z_{ref}$.
$z_{ref}$.
The significance of this empirical evidence is highlighted by quoting text directly from de Giovanetti et al. (Reference de Giovanetti, Hwang and Choi2016), who hypothesized that: ‘the energy-containing motions, which essentially reside in the logarithmic and outer regions, transport the streamwise momentum to the near-wall region through their inactive part, while generating Reynolds shear stress with their wall-detached wall-normal velocity component in the region much further from the wall’. Here, de Giovanetti et al. (Reference de Giovanetti, Hwang and Choi2016) use ‘wall detached’ to essentially refer to the active component responsible for the ![]() $w$-fluctuations, given that it does not physically extend down to the wall. Hence, figure 5(d,e,i,j) cumulatively provides strong empirical evidence in support of the hypotheses proposed by Bradshaw (Reference Bradshaw1967) and de Giovanetti et al. (Reference de Giovanetti, Hwang and Choi2016). They also align with the recent conjecture by Lee & Hwang (Reference Lee and Hwang2024), regarding the ‘origin’ of the large-scale
$w$-fluctuations, given that it does not physically extend down to the wall. Hence, figure 5(d,e,i,j) cumulatively provides strong empirical evidence in support of the hypotheses proposed by Bradshaw (Reference Bradshaw1967) and de Giovanetti et al. (Reference de Giovanetti, Hwang and Choi2016). They also align with the recent conjecture by Lee & Hwang (Reference Lee and Hwang2024), regarding the ‘origin’ of the large-scale ![]() ${\tau _w}$-signatures based on outer energy transported to the wall.
${\tau _w}$-signatures based on outer energy transported to the wall.
Unfortunately, the data considered in this study do not permit a direct comparison of ![]() $\overline {u^2_{ia}}$ with
$\overline {u^2_{ia}}$ with ![]() $\overline {{\tau }^2_w}$ for increasing
$\overline {{\tau }^2_w}$ for increasing ![]() $Re_{\tau }$, owing to mismatched
$Re_{\tau }$, owing to mismatched ![]() $z^+$ and
$z^+$ and ![]() $z/{\delta }$ for the two data sets, alongside the under-resolved measurement of
$z/{\delta }$ for the two data sets, alongside the under-resolved measurement of ![]() $\tau _w$ (both spatially and temporally). Interested readers may refer to discussions and analyses presented in Deshpande et al. (Reference Deshpande, Monty and Marusic2021), wherein it is suggested that
$\tau _w$ (both spatially and temporally). Interested readers may refer to discussions and analyses presented in Deshpande et al. (Reference Deshpande, Monty and Marusic2021), wherein it is suggested that ![]() $\overline {u^2_{ia}}$ at a fixed
$\overline {u^2_{ia}}$ at a fixed ![]() $z/{\delta }$ would be expected to increase with
$z/{\delta }$ would be expected to increase with ![]() $Re_{\tau }$ due to the broadening of the wall-scaled eddy hierarchy (see also figures 2b,e and 3). This insight potentially explains the Reynolds-number dependency of the low-frequency/large-scale signatures in the
$Re_{\tau }$ due to the broadening of the wall-scaled eddy hierarchy (see also figures 2b,e and 3). This insight potentially explains the Reynolds-number dependency of the low-frequency/large-scale signatures in the ![]() ${\tau }_w$-spectra (
${\tau }_w$-spectra (![]() $T^+ >$ 1000), as noted in figure 1(a) and discussed in the literature (Örlü & Schlatter Reference Örlü and Schlatter2011; Mathis et al. Reference Mathis, Marusic, Chernyshenko and Hutchins2013). These large-scale contributions, however, only correspond to a subset of the total inertial velocity signatures, i.e. the ones that are physically coherent with the wall. This, combined with the statistically dominant contribution from the near-wall streaks (
$T^+ >$ 1000), as noted in figure 1(a) and discussed in the literature (Örlü & Schlatter Reference Örlü and Schlatter2011; Mathis et al. Reference Mathis, Marusic, Chernyshenko and Hutchins2013). These large-scale contributions, however, only correspond to a subset of the total inertial velocity signatures, i.e. the ones that are physically coherent with the wall. This, combined with the statistically dominant contribution from the near-wall streaks (![]() $T^+ \lesssim {O}(10^3)$) is likely responsible for the weak
$T^+ \lesssim {O}(10^3)$) is likely responsible for the weak ![]() $Re_{\tau }$-growth of the
$Re_{\tau }$-growth of the ![]() ${\tau }_w$-spectra in the intermediate scales (
${\tau }_w$-spectra in the intermediate scales (![]() $ {O}(10^2) \lesssim T^+ \lesssim {O}(10^3)$).
$ {O}(10^2) \lesssim T^+ \lesssim {O}(10^3)$).
Besides testing past hypotheses, analysis in this section also demonstrates the unique capability to segregate instantaneous flow components associated with two key energy-transfer mechanisms in high ![]() $Re_{\tau }$ wall flows – TKE production and its wall-normal transport. This is a promising development for design of real-time control strategies targeting either of these mechanisms. However, more work is required with respect to identifying three-dimensional flow features/motions responsible for these mechanisms before such strategies can be actualized. As is evident from figure 5(b,g), the wall-normal transport cannot be explained purely based on the
$Re_{\tau }$ wall flows – TKE production and its wall-normal transport. This is a promising development for design of real-time control strategies targeting either of these mechanisms. However, more work is required with respect to identifying three-dimensional flow features/motions responsible for these mechanisms before such strategies can be actualized. As is evident from figure 5(b,g), the wall-normal transport cannot be explained purely based on the ![]() $w$-time series sampled across limited (and large) wall-normal offsets. But it could be possible by analysing velocity fluctuations/gradients acquired with good wall-normal resolution. This, however, is beyond the scope of the present study.
$w$-time series sampled across limited (and large) wall-normal offsets. But it could be possible by analysing velocity fluctuations/gradients acquired with good wall-normal resolution. This, however, is beyond the scope of the present study.
4.2. Active and inactive contributions to the wall pressure
This section focuses on addressing research question (ii) raised in § 1, regarding the inertial motions responsible for the ![]() $Re_{\tau }$-growth of the
$Re_{\tau }$-growth of the ![]() $p_w$-spectra. For this, we compute the linear coherence spectrum (Gibeau & Ghaemi Reference Gibeau and Ghaemi2021; Baars et al. Reference Baars, Dacome and Lee2024) between the fluctuating wall-pressure and velocity fluctuations according to the following:
$p_w$-spectra. For this, we compute the linear coherence spectrum (Gibeau & Ghaemi Reference Gibeau and Ghaemi2021; Baars et al. Reference Baars, Dacome and Lee2024) between the fluctuating wall-pressure and velocity fluctuations according to the following:
where ![]() $u_i$ can be either
$u_i$ can be either ![]() $u$,
$u$, ![]() $w$,
$w$, ![]() $u_{ia}$ or
$u_{ia}$ or ![]() $u_{a}$, (
$u_{a}$, (![]() $|\cdot |$) represents absolute values, while all other notations are the same as in (3.2). Note that
$|\cdot |$) represents absolute values, while all other notations are the same as in (3.2). Note that ![]() ${\gamma ^2_{{u_i}{p_w}}}$ can be interpreted as the spectral equivalent of a physical two-point correlation between
${\gamma ^2_{{u_i}{p_w}}}$ can be interpreted as the spectral equivalent of a physical two-point correlation between ![]() $p_w$ and
$p_w$ and ![]() $u_i$, which varies in the range 0
$u_i$, which varies in the range 0 ![]() $\le {\gamma ^2_{{u_i}{p_w}}} \le$ 1 by definition. Furthermore,
$\le {\gamma ^2_{{u_i}{p_w}}} \le$ 1 by definition. Furthermore, ![]() ${\gamma ^2_{{u}{p_w}}}$ and
${\gamma ^2_{{u}{p_w}}}$ and ![]() ${\gamma ^2_{{w}{p_w}}}$ have been analysed previously for a canonical TBL at
${\gamma ^2_{{w}{p_w}}}$ have been analysed previously for a canonical TBL at ![]() $Re_{\tau } \approx 2000$ by Gibeau & Ghaemi (Reference Gibeau and Ghaemi2021), to establish the scale-based coupling between the inertial motions and the large-scale pressure (LSP;
$Re_{\tau } \approx 2000$ by Gibeau & Ghaemi (Reference Gibeau and Ghaemi2021), to establish the scale-based coupling between the inertial motions and the large-scale pressure (LSP; ![]() $0.8 < T{U_{\infty }}/{\delta } < 7$), as well as the very-large-scale pressure (VLSP;
$0.8 < T{U_{\infty }}/{\delta } < 7$), as well as the very-large-scale pressure (VLSP; ![]() $T{U_{\infty }}/{\delta } > 7$) regions of the
$T{U_{\infty }}/{\delta } > 7$) regions of the ![]() $p_w$-spectrum. To validate our analysis, we recompute
$p_w$-spectrum. To validate our analysis, we recompute ![]() ${\gamma ^2_{{u}{p_w}}}$ and
${\gamma ^2_{{u}{p_w}}}$ and ![]() ${\gamma ^2_{{w}{p_w}}}$ (figure 7a,b) from the LES data set at
${\gamma ^2_{{w}{p_w}}}$ (figure 7a,b) from the LES data set at ![]() $Re_{\tau } \approx 2000$ and compare it with the outer-scaled
$Re_{\tau } \approx 2000$ and compare it with the outer-scaled ![]() $p_w$-spectrum (figure 7c). The coherence spectrum depicts characteristics consistent with those reported by Gibeau & Ghaemi (Reference Gibeau and Ghaemi2021): the VLSP region is associated with relatively strong coherence between
$p_w$-spectrum (figure 7c). The coherence spectrum depicts characteristics consistent with those reported by Gibeau & Ghaemi (Reference Gibeau and Ghaemi2021): the VLSP region is associated with relatively strong coherence between ![]() $u$ and
$u$ and ![]() $p_w$, but not between
$p_w$, but not between ![]() $w$ and
$w$ and ![]() $p_w$. While, in case of LSP, reasonable coherence can be noted between both
$p_w$. While, in case of LSP, reasonable coherence can be noted between both ![]() $u$ and
$u$ and ![]() $p_w$ and
$p_w$ and ![]() $w$ and
$w$ and ![]() $p_w$. If the same spectrograms were plotted as a function of streamwise wavelengths based on Taylor's hypothesis (i.e.
$p_w$. If the same spectrograms were plotted as a function of streamwise wavelengths based on Taylor's hypothesis (i.e. ![]() ${\lambda }^+_x = {T^+}{{{U}}^+}(z^+)$), the energetic ridges of
${\lambda }^+_x = {T^+}{{{U}}^+}(z^+)$), the energetic ridges of ![]() ${\gamma ^2_{{u}{p_w}}}$ and
${\gamma ^2_{{u}{p_w}}}$ and ![]() ${\gamma ^2_{{w}{p_w}}}$ would respectively exhibit distance-from-the-wall scalings:
${\gamma ^2_{{w}{p_w}}}$ would respectively exhibit distance-from-the-wall scalings: ![]() ${{\lambda }_x}/z$ = 14 and
${{\lambda }_x}/z$ = 14 and ![]() ${{\lambda }_x}/z = 8.5$ (not shown here), consistent with Baars et al. (Reference Baars, Dacome and Lee2024). Exhibition of this scaling confirms the association of the
${{\lambda }_x}/z = 8.5$ (not shown here), consistent with Baars et al. (Reference Baars, Dacome and Lee2024). Exhibition of this scaling confirms the association of the ![]() $p_w$-spectrum with the wall-scaled eddy hierarchy coexisting in the inertial/large-scale range.
$p_w$-spectrum with the wall-scaled eddy hierarchy coexisting in the inertial/large-scale range.

Figure 7. Linear coherence spectrum (![]() ${\gamma }^2_{{u_i}{p_w}}$; (4.2)) computed between
${\gamma }^2_{{u_i}{p_w}}$; (4.2)) computed between ![]() $u_i(z^+)$ and
$u_i(z^+)$ and ![]() $p_w$ as a function of outer- or inner-scaled
$p_w$ as a function of outer- or inner-scaled ![]() $T$: (a)
$T$: (a) ![]() ${\gamma }^2_{{u}{p_w}}$, (b)
${\gamma }^2_{{u}{p_w}}$, (b) ![]() ${\gamma }^2_{{w}{p_w}}$, (d,g)
${\gamma }^2_{{w}{p_w}}$, (d,g) ![]() ${\gamma }^2_{{u_{ia}}{p_w}}$ and (e,h)
${\gamma }^2_{{u_{ia}}{p_w}}$ and (e,h) ![]() ${\gamma }^2_{{u_{a}}{p_w}}$. (a,b,d,e) Depict
${\gamma }^2_{{u_{a}}{p_w}}$. (a,b,d,e) Depict ![]() ${\gamma }^2_{{u_i}{p_w}}$ computed from
${\gamma }^2_{{u_i}{p_w}}$ computed from ![]() $Re_{\tau } \approx 2000$ LES data compared against the (c, f) outer-scaled
$Re_{\tau } \approx 2000$ LES data compared against the (c, f) outer-scaled ![]() $p_w$-spectrum plotted vs outer-scaled
$p_w$-spectrum plotted vs outer-scaled ![]() $T$. Panels (g,h), respectively, depict
$T$. Panels (g,h), respectively, depict ![]() ${\gamma }^2_{{u_{ia}}{p_w}}$ and
${\gamma }^2_{{u_{ia}}{p_w}}$ and ![]() ${\gamma }^2_{{u_{a}}{p_w}}$ contours for data across various
${\gamma }^2_{{u_{a}}{p_w}}$ contours for data across various ![]() $Re_{\tau }$, which are compared against (i)
$Re_{\tau }$, which are compared against (i) ![]() $f{{\phi }^+_{{p_w}{p_w}}}$ plotted vs
$f{{\phi }^+_{{p_w}{p_w}}}$ plotted vs ![]() $T^+$. Panels show (a)
$T^+$. Panels show (a) ![]() ${\gamma }^2_{{u}{p_w}}$, (b)
${\gamma }^2_{{u}{p_w}}$, (b) ![]() ${\gamma }^2_{{w}{p_w}}$, (d)
${\gamma }^2_{{w}{p_w}}$, (d) ![]() ${\gamma }^2_{{u_{ia}}{p_w}}$, (e)
${\gamma }^2_{{u_{ia}}{p_w}}$, (e) ![]() ${\gamma }^2_{{u_{a}}{p_w}}$, (g)
${\gamma }^2_{{u_{a}}{p_w}}$, (g) ![]() ${\gamma }^2_{{u_{ia}}{p_w}}=0.02, 0.09$, (h)
${\gamma }^2_{{u_{ia}}{p_w}}=0.02, 0.09$, (h) ![]() ${\gamma }^2_{{u_{a}}{p_w}}=0.07$.
${\gamma }^2_{{u_{a}}{p_w}}=0.07$.
Connecting the differences between ![]() ${{\gamma }^2_{{u}{p_w}}}$ and
${{\gamma }^2_{{u}{p_w}}}$ and ![]() ${{\gamma }^2_{{w}{p_w}}}$ with the characteristics of active and inactive components discussed in § 2, it can be hypothesized that the
${{\gamma }^2_{{w}{p_w}}}$ with the characteristics of active and inactive components discussed in § 2, it can be hypothesized that the ![]() $p_w$-spectrum in the LSP and VLSP ranges, is, respectively, correlated to the active and inactive motions. The availability of decomposed
$p_w$-spectrum in the LSP and VLSP ranges, is, respectively, correlated to the active and inactive motions. The availability of decomposed ![]() $u_{a}$ and
$u_{a}$ and ![]() $u_{ia}$ components, for the same data as in figure 7(a,b), permits us to directly test this hypothesis by analysing the
$u_{ia}$ components, for the same data as in figure 7(a,b), permits us to directly test this hypothesis by analysing the ![]() ${{\gamma }^2_{{u_{ia}}{p_w}}}$ and
${{\gamma }^2_{{u_{ia}}{p_w}}}$ and ![]() ${{\gamma }^2_{{u_{a}}{p_w}}}$ results of figure 7(d,e), respectively. It is evident that the coherence between
${{\gamma }^2_{{u_{a}}{p_w}}}$ results of figure 7(d,e), respectively. It is evident that the coherence between ![]() $u_{ia}$ and
$u_{ia}$ and ![]() $p_w$ is limited to the VLSP range, while that between
$p_w$ is limited to the VLSP range, while that between ![]() $u_{a}$ and
$u_{a}$ and ![]() $p_w$ (in the inertial region) is predominantly in the LSP range, similar to
$p_w$ (in the inertial region) is predominantly in the LSP range, similar to ![]() ${{\gamma }^2_{{w}{p_w}}}$. All the observations are consistent with our hypothesis above, with the similarity between
${{\gamma }^2_{{w}{p_w}}}$. All the observations are consistent with our hypothesis above, with the similarity between ![]() ${{\gamma }^2_{{w}{p_w}}}$ and
${{\gamma }^2_{{w}{p_w}}}$ and ![]() ${{\gamma }^2_{{u_{a}}{p_w}}}$ expected, considering that
${{\gamma }^2_{{u_{a}}{p_w}}}$ expected, considering that ![]() $w$-signatures are predominantly from active contributions (see (2.2)). The present results offer phenomenological explanations for the conclusions drawn by Gibeau & Ghaemi (Reference Gibeau and Ghaemi2021), who associated
$w$-signatures are predominantly from active contributions (see (2.2)). The present results offer phenomenological explanations for the conclusions drawn by Gibeau & Ghaemi (Reference Gibeau and Ghaemi2021), who associated ![]() $p_w$-fluctuations in the LSP range with Reynolds shear-stress-carrying ejection and sweep events in the log region, i.e. the active components of the wall-scaled eddies. Conversely,
$p_w$-fluctuations in the LSP range with Reynolds shear-stress-carrying ejection and sweep events in the log region, i.e. the active components of the wall-scaled eddies. Conversely, ![]() $p_w$-fluctuations in the VLSP range were linked with the large
$p_w$-fluctuations in the VLSP range were linked with the large ![]() $\delta$-scaled motions and superstructures, which correspond to the inactive components transporting energy from the outer region to the wall. The results also confirm the dependency of
$\delta$-scaled motions and superstructures, which correspond to the inactive components transporting energy from the outer region to the wall. The results also confirm the dependency of ![]() $p_w$ on velocity signatures that are coherent (akin to
$p_w$ on velocity signatures that are coherent (akin to ![]() $u_{ia}$, amongst others) as well as incoherent (akin to
$u_{ia}$, amongst others) as well as incoherent (akin to ![]() $u_{a}$, amongst others) with the wall, consistent with the pressure Poisson equation (Tsuji et al. Reference Tsuji, Fransson, Alfredsson and Johansson2007).
$u_{a}$, amongst others) with the wall, consistent with the pressure Poisson equation (Tsuji et al. Reference Tsuji, Fransson, Alfredsson and Johansson2007).
Since the wall-scaled eddy hierarchy grows by coexisting in a physically taller log region with increasing ![]() $Re_{\tau }$ (figure 2b,e; Deshpande et al. Reference Deshpande, Monty and Marusic2021), the association of the LSP and VLSP ranges, with
$Re_{\tau }$ (figure 2b,e; Deshpande et al. Reference Deshpande, Monty and Marusic2021), the association of the LSP and VLSP ranges, with ![]() $u_{a}$ and
$u_{a}$ and ![]() $u_{ia}$, can be used to explain the
$u_{ia}$, can be used to explain the ![]() $Re_{\tau }$-variation of the
$Re_{\tau }$-variation of the ![]() $p_w$-spectrum. To this end, figure 7(g,h) plots the constant energy contours of
$p_w$-spectrum. To this end, figure 7(g,h) plots the constant energy contours of ![]() ${{\gamma }^2_{{u_{ia}}{p_w}}}$ and
${{\gamma }^2_{{u_{ia}}{p_w}}}$ and ![]() ${{\gamma }^2_{{u_{a}}{p_w}}}$ vs
${{\gamma }^2_{{u_{a}}{p_w}}}$ vs ![]() $T^+$ for 1000
$T^+$ for 1000 ![]() $\lesssim Re_{\tau } \lesssim$ 2000, each of which exhibit their unique
$\lesssim Re_{\tau } \lesssim$ 2000, each of which exhibit their unique ![]() $Re_{\tau }$-trends. Notably, the contours of
$Re_{\tau }$-trends. Notably, the contours of ![]() ${{\gamma }^2_{{u_{a}}{p_w}}}$ can be seen to grow and widen across a larger
${{\gamma }^2_{{u_{a}}{p_w}}}$ can be seen to grow and widen across a larger ![]() $T^+$ range with
$T^+$ range with ![]() $Re_{\tau }$ (indicated by green arrows), which explains the
$Re_{\tau }$ (indicated by green arrows), which explains the ![]() $Re_{\tau }$-growth of the
$Re_{\tau }$-growth of the ![]() $p_w$-spectrum in the intermediate-scale range:
$p_w$-spectrum in the intermediate-scale range: ![]() $ {O}(10^2) \lesssim T^+ \lesssim {O}(10^3)$. It suggests that a physically taller log region comprises a broader hierarchy of TKE-producing active motions, which subsequently increases contributions to the
$ {O}(10^2) \lesssim T^+ \lesssim {O}(10^3)$. It suggests that a physically taller log region comprises a broader hierarchy of TKE-producing active motions, which subsequently increases contributions to the ![]() $p_w$-spectrum. This is analogous to the broadening of the
$p_w$-spectrum. This is analogous to the broadening of the ![]() $\overline {uw}$-plateau (
$\overline {uw}$-plateau (![]() ${\sim }-1$) in the log region with increasing
${\sim }-1$) in the log region with increasing ![]() $Re_{\tau }$ ((2.1), Marusic & Perry Reference Marusic and Perry1995; Baidya et al. Reference Baidya, Philip, Hutchins, Monty and Marusic2017). In contrast, the active motions do not contribute to the
$Re_{\tau }$ ((2.1), Marusic & Perry Reference Marusic and Perry1995; Baidya et al. Reference Baidya, Philip, Hutchins, Monty and Marusic2017). In contrast, the active motions do not contribute to the ![]() ${\tau }_w$-spectrum since they do not physically extend down to the wall, and consequently do not influence the near-wall velocity gradient (de Giovanetti et al. Reference de Giovanetti, Hwang and Choi2016). It is thus obvious that the
${\tau }_w$-spectrum since they do not physically extend down to the wall, and consequently do not influence the near-wall velocity gradient (de Giovanetti et al. Reference de Giovanetti, Hwang and Choi2016). It is thus obvious that the ![]() $Re_{\tau }$ trend of
$Re_{\tau }$ trend of ![]() $f{{\phi }^{+}_{{{p}_w}{{p}_w}}}$ is governed by a broader range of inertial motions than those influencing
$f{{\phi }^{+}_{{{p}_w}{{p}_w}}}$ is governed by a broader range of inertial motions than those influencing ![]() $f{{\phi }^{+}_{{{\tau }_w}{{\tau }_w}}}$, likely explaining the stronger
$f{{\phi }^{+}_{{{\tau }_w}{{\tau }_w}}}$, likely explaining the stronger ![]() $Re_{\tau }$-growth of the former compared with the latter. Note that arriving at this realization was made possible by decomposing the
$Re_{\tau }$-growth of the former compared with the latter. Note that arriving at this realization was made possible by decomposing the ![]() $u$–
$u$–![]() $p_w$ coherence into its active and inactive components.
$p_w$ coherence into its active and inactive components.
Similar to the behaviour of ![]() ${{\gamma }^2_{{u_{a}}{p_w}}}$, the contours of
${{\gamma }^2_{{u_{a}}{p_w}}}$, the contours of ![]() ${{\gamma }^2_{{u_{ia}}{p_w}}}$ can also be seen extending across a larger
${{\gamma }^2_{{u_{ia}}{p_w}}}$ can also be seen extending across a larger ![]() $z^+$-range with
$z^+$-range with ![]() $Re_{\tau }$. This is predominantly associated with the energization and increasing wall-normal extent of the large wall-scaled eddies and superstructures (Mathis et al. Reference Mathis, Marusic, Chernyshenko and Hutchins2013; Lee & Moser Reference Lee and Moser2019). At very high
$Re_{\tau }$. This is predominantly associated with the energization and increasing wall-normal extent of the large wall-scaled eddies and superstructures (Mathis et al. Reference Mathis, Marusic, Chernyshenko and Hutchins2013; Lee & Moser Reference Lee and Moser2019). At very high ![]() $Re_{\tau } \sim {O}(10^6)$, these energetic superstructures along with a broad hierarchy of wall-scaled eddies (figure 2e) contribute to the significantly enhanced VLSP signatures of the
$Re_{\tau } \sim {O}(10^6)$, these energetic superstructures along with a broad hierarchy of wall-scaled eddies (figure 2e) contribute to the significantly enhanced VLSP signatures of the ![]() $p_w$-spectrum through their inactive component (figure 7i). The present analysis, hence, encourages future detailed multi-point measurements and high-fidelity simulations that can quantify the pressure–velocity coupling at high-
$p_w$-spectrum through their inactive component (figure 7i). The present analysis, hence, encourages future detailed multi-point measurements and high-fidelity simulations that can quantify the pressure–velocity coupling at high-![]() $Re_{\tau }$ (
$Re_{\tau }$ (![]() $\gtrsim 10^4$), with particular focus on velocity signatures superimposed by the inertia-dominated motions. Consequently, it also motivates high-
$\gtrsim 10^4$), with particular focus on velocity signatures superimposed by the inertia-dominated motions. Consequently, it also motivates high-![]() $Re_{\tau }$ investigations of the nonlinear interactions between the large-scale velocity signatures and
$Re_{\tau }$ investigations of the nonlinear interactions between the large-scale velocity signatures and ![]() $p_w$ (Thomas & Bull Reference Thomas and Bull1983; Tsuji, Marusic & Johansson Reference Tsuji, Marusic and Johansson2015), which have previously been limited to
$p_w$ (Thomas & Bull Reference Thomas and Bull1983; Tsuji, Marusic & Johansson Reference Tsuji, Marusic and Johansson2015), which have previously been limited to ![]() $Re_{\tau } \lesssim {O}(10^3)$.
$Re_{\tau } \lesssim {O}(10^3)$.
5. Concluding remarks
This study clarifies the ![]() $Re_{\tau }$-dependent, intermediate- and large-scale contributions to the
$Re_{\tau }$-dependent, intermediate- and large-scale contributions to the ![]() ${\tau }_w$- and
${\tau }_w$- and ![]() $p_w$-spectra by invoking the attached (wall-scaled) eddy framework (Townsend Reference Townsend1976), based on active and inactive motions. Unique multi-point data sets are analysed using an energy-decomposition methodology to reveal the contributions from TKE-producing (active) and non-producing (inactive) components, at any wall-normal position in the log region, to the
$p_w$-spectra by invoking the attached (wall-scaled) eddy framework (Townsend Reference Townsend1976), based on active and inactive motions. Unique multi-point data sets are analysed using an energy-decomposition methodology to reveal the contributions from TKE-producing (active) and non-producing (inactive) components, at any wall-normal position in the log region, to the ![]() ${\tau }_w$- and
${\tau }_w$- and ![]() $p_w$-spectra. Inactive components of wall-scaled eddies are found to be responsible for the non-local wall-normal transport of the large-scale inertia-dominated energy, from its origin in the log region (i.e. produced by active components) to the wall. On the other hand, the majority of the TKE-producing (i.e. active) motions in the log region are localized and physically detached from the wall, not influencing
$p_w$-spectra. Inactive components of wall-scaled eddies are found to be responsible for the non-local wall-normal transport of the large-scale inertia-dominated energy, from its origin in the log region (i.e. produced by active components) to the wall. On the other hand, the majority of the TKE-producing (i.e. active) motions in the log region are localized and physically detached from the wall, not influencing ![]() ${\tau }_w$. These results provide strong empirical evidence for the hypotheses proposed by Bradshaw (Reference Bradshaw1967) and de Giovanetti et al. (Reference de Giovanetti, Hwang and Choi2016). They also explain the appearance and
${\tau }_w$. These results provide strong empirical evidence for the hypotheses proposed by Bradshaw (Reference Bradshaw1967) and de Giovanetti et al. (Reference de Giovanetti, Hwang and Choi2016). They also explain the appearance and ![]() $Re_{\tau }$-growth of the large-scale signatures in the
$Re_{\tau }$-growth of the large-scale signatures in the ![]() ${\tau }_w$-spectra, despite the insignificant large-scale TKE production occurring in the near-wall region. In terms of their contribution to wall pressure, the active and inactive components are respectively correlated with the intermediate- and large-scale portions of the
${\tau }_w$-spectra, despite the insignificant large-scale TKE production occurring in the near-wall region. In terms of their contribution to wall pressure, the active and inactive components are respectively correlated with the intermediate- and large-scale portions of the ![]() $p_w$-spectrum. Both components exhibit growth with increasing
$p_w$-spectrum. Both components exhibit growth with increasing ![]() $Re_{\tau }$, which is attributed to the broadening of the wall-scaled eddy hierarchy. This is plausibly responsible for the rapid
$Re_{\tau }$, which is attributed to the broadening of the wall-scaled eddy hierarchy. This is plausibly responsible for the rapid ![]() $Re_{\tau }$-growth of the
$Re_{\tau }$-growth of the ![]() $p_w$-spectra relative to
$p_w$-spectra relative to ![]() ${\tau }_w$ (figure 1a,b). These phenomenological explanations carry potential to influence future real-time control strategies, aimed at achieving energy-efficient drag and flow-noise reduction.
${\tau }_w$ (figure 1a,b). These phenomenological explanations carry potential to influence future real-time control strategies, aimed at achieving energy-efficient drag and flow-noise reduction.
While this study demonstrates the new found capability to extract the instantaneous active and inactive components, also shedding light on their role in the large-scale energy-transfer mechanisms, it does not delve into the nonlinear/inter-scale interactions between these motions. These interactions, which have been previously discussed in the literature to a certain extent (Cho et al. Reference Cho, Hwang and Choi2018; Lee & Moser Reference Lee and Moser2019), can likely explain the transfer of TKE produced by active components to the inactive component of the wall-scaled eddies, before being transported downward to the wall (figure 2). At this stage, these interactions are not accounted for within Townsend's wall-scaled eddy framework, which is inherently limited to the linear superposition of velocity signatures induced by the eddy structures. It does, however, highlight the opportunity for future upgrades/modifications to the model by incorporating the inter-scale interactions between the wall-scaled eddy hierarchy.
Funding
R.D. is supported by the University of Melbourne's Postdoctoral Fellowship and acknowledges insightful discussions with Drs W.J. Baars, B. Gibeau and S. Ghaemi. Funding is gratefully acknowledged from the Office of Naval Research: N62909-23-1-2068 (R.D., I.M.), and to R.V. from the European Research Council grant no. ‘2021-CoG-101043998, DEEPCONTROL’. Views/opinions expressed are, however, those of the author(s) only and do not necessarily reflect those of the European Union or the European Research Council. Neither the European Union nor the granting authority can be held responsible for them.
Declaration of interests
The authors report no conflict of interest.