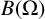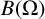1 Introduction
Let
![]() $B(\Omega )$
be a Banach space of holomorphic functions on a bounded connected domain
$B(\Omega )$
be a Banach space of holomorphic functions on a bounded connected domain
![]() $\Omega $
in
$\Omega $
in
![]() ${{\mathbb C}^n}$
. When
${{\mathbb C}^n}$
. When
![]() $ n>1$
, we may assume that
$ n>1$
, we may assume that
![]() $\Omega $
is simply connected since any holomorphic function on
$\Omega $
is simply connected since any holomorphic function on
![]() $\Omega $
can be analytically extended to the holes in
$\Omega $
can be analytically extended to the holes in
![]() $\Omega $
by Hartog’s theorem. Let
$\Omega $
by Hartog’s theorem. Let
![]() $K_{w}$
denote the evaluation function at
$K_{w}$
denote the evaluation function at
![]() $z\in \Omega $
, that is,
$z\in \Omega $
, that is,
The aim of this paper is to build a criterion for reflexivity of the Banach space
![]() $B(\Omega )$
via the evaluation functions on
$B(\Omega )$
via the evaluation functions on
![]() $B(\Omega ).$
$B(\Omega ).$
Characterization of reflexivity of different types of Banach spaces is a fundamental problem in functional analysis. A very famous general criterion is the well-known Kakutani theorem, which uses the closed unit ball of the Banach space.
Turning to the specific Banach space
![]() $B(\Omega )$
on which we focus in this paper, finding a specific criterion for reflexivity is certainly an important problem.
$B(\Omega )$
on which we focus in this paper, finding a specific criterion for reflexivity is certainly an important problem.
Our idea is to use evaluation functions on
![]() $B(\Omega )$
, which play the role of reproducing kernels on a Hilbert space. However, when compared to reproducing kernels (on a Hilbert space), the properties of evaluation functions on Banach spaces are far less known.
$B(\Omega )$
, which play the role of reproducing kernels on a Hilbert space. However, when compared to reproducing kernels (on a Hilbert space), the properties of evaluation functions on Banach spaces are far less known.
To further understand the properties of evaluation functions on
![]() $B(\Omega )$
, we recall that in a reproducing kernel Hilbert space of holomorphic functions, the reproducing kernel has the very important property that
$B(\Omega )$
, we recall that in a reproducing kernel Hilbert space of holomorphic functions, the reproducing kernel has the very important property that
![]() $\{K_ {z} \mid z \in U\}$
spans the full space for any open subset or dense subset U of the domain (see for example, [Reference Cowen and MacCluer2]). A similar conclusion may not hold in the general Banach space of holomorphic functions. Instead, we consider the following question related to the reflexivity of the specific Banach space
$\{K_ {z} \mid z \in U\}$
spans the full space for any open subset or dense subset U of the domain (see for example, [Reference Cowen and MacCluer2]). A similar conclusion may not hold in the general Banach space of holomorphic functions. Instead, we consider the following question related to the reflexivity of the specific Banach space
![]() $B(\Omega )$
.
$B(\Omega )$
.
Question 1.1. Can we characterize the reflexivity of
![]() $B(\Omega )$
via evaluation functions on
$B(\Omega )$
via evaluation functions on
![]() $B(\Omega )$
?
$B(\Omega )$
?
Recall again that
![]() $B(\Omega )$
is the Banach space of holomorphic functions on a bounded connected domain
$B(\Omega )$
is the Banach space of holomorphic functions on a bounded connected domain
![]() $\Omega $
in
$\Omega $
in
![]() ${{\mathbb C}^n}$
. We list the following assumption about
${{\mathbb C}^n}$
. We list the following assumption about
![]() $\Omega $
and
$\Omega $
and
![]() $B(\Omega )$
that is used in stating our main result.
$B(\Omega )$
that is used in stating our main result.
-
(a) For each
 $z\in \Omega $
and for every
$z\in \Omega $
and for every
 $f\in B(\Omega )$
, the evaluation map
$f\in B(\Omega )$
, the evaluation map
 $K_z: f\mapsto f(z)$
is a bounded linear functional on
$K_z: f\mapsto f(z)$
is a bounded linear functional on
 $B(\Omega )$
.
$B(\Omega )$
.
We now state our main result as follows, which answers the Question above.
Theorem 1.2. Let
![]() $\Omega $
and
$\Omega $
and
![]() $B(\Omega )$
satisfy assumption (a). Then
$B(\Omega )$
satisfy assumption (a). Then
![]() $B(\Omega )$
is reflexive if and only if for every arbitrary open subset or dense subset U of
$B(\Omega )$
is reflexive if and only if for every arbitrary open subset or dense subset U of
![]() $\Omega , $
$\Omega , $
where
![]() $\bigvee _{z\in U}\{K_{z}\}$
denotes the space spanned by
$\bigvee _{z\in U}\{K_{z}\}$
denotes the space spanned by
![]() $\{K_{z}\}_{z\in U}$
.
$\{K_{z}\}_{z\in U}$
.
We note that the criterion established in Theorem 1.2 can be applied to various settings. For example, taking
![]() $\Omega $
to be the unit disk
$\Omega $
to be the unit disk
![]() $\mathbb {D}$
in
$\mathbb {D}$
in
![]() $\mathbb {C}$
, we see that our criterion applies to the Hardy spaces, Bergman spaces, Dirichlet spaces, Hardy–Sobolev spaces, and Bloch spaces on
$\mathbb {C}$
, we see that our criterion applies to the Hardy spaces, Bergman spaces, Dirichlet spaces, Hardy–Sobolev spaces, and Bloch spaces on
![]() $\mathbb {D}$
.
$\mathbb {D}$
.
2 Properties of evaluation functions on
 $B(\Omega)$
$B(\Omega)$
In this section, we discuss some properties of evaluation functions. It can be proved that the closed unit ball of the Banach space of analytic functions is a normal family by using the properties of evaluation functions. This conclusion is somewhat unexpected since, in general, the closed unit ball of an infinite dimensional Banach space is not weakly compact, which varies from the Hilbert space case. In fact, Kakutani’s theorem, also called the Eberlein–Shmuleyan theorem, showed that a necessary and sufficient condition for the closed unit ball of a Banach space to be weakly compact is that the space is reflexive (see [Reference Conway1]).
We begin by recalling a basic result in [Reference Rudin6, page 5], followed by several auxiliary lemmas.
Lemma 2.1. If
![]() $\Lambda $
is a uniformly bounded family of holomorphic functions in
$\Lambda $
is a uniformly bounded family of holomorphic functions in
![]() $\Omega $
, then
$\Omega $
, then
![]() $\Lambda $
is equicontinuous on every compact subset of
$\Lambda $
is equicontinuous on every compact subset of
![]() $\Omega .$
In other words,
$\Omega .$
In other words,
![]() $\Lambda $
is a normal family.
$\Lambda $
is a normal family.
Lemma 2.2. Suppose
![]() $\Omega $
and
$\Omega $
and
![]() $B(\Omega )$
satisfy assumption (a), and
$B(\Omega )$
satisfy assumption (a), and
![]() $\mathcal {K}$
is an arbitrary compact subset of
$\mathcal {K}$
is an arbitrary compact subset of
![]() $\Omega .$
Then,
$\Omega .$
Then,
Proof. For any
![]() $f\in B(\Omega ),$
it is clear that
$f\in B(\Omega ),$
it is clear that
since f is holomorphic on
![]() $\Omega .$
By the uniform boundedness principle,
$\Omega .$
By the uniform boundedness principle,
The proof is complete.
Lemma 2.3. Let
![]() $\Omega $
and
$\Omega $
and
![]() $B(\Omega )$
satisfy assumption (a). Then, for an arbitrary sequence
$B(\Omega )$
satisfy assumption (a). Then, for an arbitrary sequence
![]() $\{f_{k}\}$
in
$\{f_{k}\}$
in
![]() $(B(\Omega ))_{1},$
the unit ball in
$(B(\Omega ))_{1},$
the unit ball in
![]() $B(\Omega )$
, we obtain that
$B(\Omega )$
, we obtain that
![]() $ \{f_{k}\}$
is a normal family.
$ \{f_{k}\}$
is a normal family.
Proof. Let
![]() $\mathcal {K}$
be an arbitrary compact subset of
$\mathcal {K}$
be an arbitrary compact subset of
![]() $\Omega .$
Choose another compact subset
$\Omega .$
Choose another compact subset
![]() $\widetilde {\mathcal {K}}\subset \Omega $
and an open subset
$\widetilde {\mathcal {K}}\subset \Omega $
and an open subset
![]() $\widetilde \Omega \subset \Omega $
such that
$\widetilde \Omega \subset \Omega $
such that
then
 $$ \begin{align*} \sup_{k}\sup_{z\in \widetilde{\mathcal{K}}}|f_{k}(z)|&=\sup_{k}\sup_{z\in \widetilde{\mathcal{K}}}|K_{z}(f_{k})|\\ &\leq \sup_{k}\|f_{k}\|\cdot \sup_{z\in \widetilde{\mathcal{K}}}\|K_{z}\| \\ &\leq \sup_{z\in \widetilde{\mathcal{K}}}\|K_{z}\|<\infty \end{align*} $$
$$ \begin{align*} \sup_{k}\sup_{z\in \widetilde{\mathcal{K}}}|f_{k}(z)|&=\sup_{k}\sup_{z\in \widetilde{\mathcal{K}}}|K_{z}(f_{k})|\\ &\leq \sup_{k}\|f_{k}\|\cdot \sup_{z\in \widetilde{\mathcal{K}}}\|K_{z}\| \\ &\leq \sup_{z\in \widetilde{\mathcal{K}}}\|K_{z}\|<\infty \end{align*} $$
by Lemma 2.2. Thus,
This implies that
![]() $\{f_{k}\}$
is uniformly bounded on
$\{f_{k}\}$
is uniformly bounded on
![]() $ \widetilde \Omega $
and also equicontinuous on every compact subset of
$ \widetilde \Omega $
and also equicontinuous on every compact subset of
![]() $\widetilde \Omega $
by Lemma 2.1. In particular,
$\widetilde \Omega $
by Lemma 2.1. In particular,
![]() $\{f_{k}\}$
is equicontinuous on
$\{f_{k}\}$
is equicontinuous on
![]() $\mathcal {K}.$
This completes the proof.
$\mathcal {K}.$
This completes the proof.
It is well known that for
![]() $1<p<\infty ,$
a sequence
$1<p<\infty ,$
a sequence
![]() $\{f_{k}\}$
in Bergman space
$\{f_{k}\}$
in Bergman space
![]() $A^{p}(\mathbb {D})$
converges weakly to zero if and only if
$A^{p}(\mathbb {D})$
converges weakly to zero if and only if
![]() $\{\|f_{k}\|\}_{k}$
is bounded, and
$\{\|f_{k}\|\}_{k}$
is bounded, and
![]() $f_{k}(z)\rightarrow 0$
uniformly on compact subsets of
$f_{k}(z)\rightarrow 0$
uniformly on compact subsets of
![]() $\mathbb {D}$
as
$\mathbb {D}$
as
![]() $k\rightarrow \infty $
(see [Reference Hedenmalm, Kirenblum and Zhu3, Exercise 1.6.1]). Since
$k\rightarrow \infty $
(see [Reference Hedenmalm, Kirenblum and Zhu3, Exercise 1.6.1]). Since
![]() $A^{p}(\mathbb {D}) (1<p<\infty )$
is reflexive, we see that this conclusion is the consequence of Kakutani’s theorem and our Lemma 2.3.
$A^{p}(\mathbb {D}) (1<p<\infty )$
is reflexive, we see that this conclusion is the consequence of Kakutani’s theorem and our Lemma 2.3.
Proposition 2.4. Suppose
![]() $\Omega $
and
$\Omega $
and
![]() $B(\Omega )$
satisfy assumption (a), and U is an open subset or a dense subset of
$B(\Omega )$
satisfy assumption (a), and U is an open subset or a dense subset of
![]() $\Omega $
. If
$\Omega $
. If
![]() $B(\Omega )$
is reflexive, then
$B(\Omega )$
is reflexive, then
Proof. Write
![]() $M=\bigvee _{z\in U}\{K_{z}\}.$
If
$M=\bigvee _{z\in U}\{K_{z}\}.$
If
![]() $M\neq (B(\Omega ))^{*},$
then there is a nonzero
$M\neq (B(\Omega ))^{*},$
then there is a nonzero
![]() $F\in (B(\Omega ))^{**}$
such that
$F\in (B(\Omega ))^{**}$
such that
![]() $F|_M=0.$
Here,
$F|_M=0.$
Here,
![]() $(B(\Omega ))^{**}$
is the second dual space of
$(B(\Omega ))^{**}$
is the second dual space of
![]() $B(\Omega ).$
Since
$B(\Omega ).$
Since
![]() $B(\Omega )$
is reflexive, there is a nonzero function
$B(\Omega )$
is reflexive, there is a nonzero function
![]() $f\in B(\Omega )$
that satisfies
$f\in B(\Omega )$
that satisfies
![]() $F=f^{**}\in (B(\Omega ))^{**},$
and thus
$F=f^{**}\in (B(\Omega ))^{**},$
and thus
This implies that
![]() $f=0.$
Thus,
$f=0.$
Thus,
![]() $F=f^{**}=0,$
which contradicts
$F=f^{**}=0,$
which contradicts
![]() $F\neq 0.$
This contradiction completes the proof.
$F\neq 0.$
This contradiction completes the proof.
Let
![]() $ \mathfrak {B}$
be the Bloch space consisting of functions f with
$ \mathfrak {B}$
be the Bloch space consisting of functions f with
and the little Bloch space of
![]() ${\mathbb D},$
denoted by
${\mathbb D},$
denoted by
![]() $\mathfrak {B}_{0}$
, be the closed subspace of
$\mathfrak {B}_{0}$
, be the closed subspace of
![]() $ \mathfrak {B}$
consisting of functions f with
$ \mathfrak {B}$
consisting of functions f with
The following lemmas are well known.
Lemma 2.5 [Reference Zhu8, Corollary 5.10].
![]() $\mathfrak {B}_{0}$
is the closure in
$\mathfrak {B}_{0}$
is the closure in
![]() $ \mathfrak {B}$
of the set of polynomials. In particular,
$ \mathfrak {B}$
of the set of polynomials. In particular,
![]() $\mathfrak {B}_{0}$
is separable.
$\mathfrak {B}_{0}$
is separable.
Suppose
![]() $L^{1}_{a }(dA_{\alpha })$
is the weighted Bergman space with
$L^{1}_{a }(dA_{\alpha })$
is the weighted Bergman space with
![]() $dA_{\alpha }=c_{\alpha }(1-|z|^{2})^{\alpha }\,dA$
, a positive Borel measure on
$dA_{\alpha }=c_{\alpha }(1-|z|^{2})^{\alpha }\,dA$
, a positive Borel measure on
![]() ${\mathbb D}$
, where
${\mathbb D}$
, where
![]() $c_{\alpha }=\alpha +1$
for
$c_{\alpha }=\alpha +1$
for
![]() $\alpha>-1$
and
$\alpha>-1$
and
![]() $c_{\alpha }=1$
for
$c_{\alpha }=1$
for
![]() $\alpha \leq -1$
(see [Reference Zhu8, page 72]). Then we have the following lemma.
$\alpha \leq -1$
(see [Reference Zhu8, page 72]). Then we have the following lemma.
Lemma 2.6 [Reference Zhu8, Theorem 5.15].
For any
![]() $\alpha> -1$
, we have
$\alpha> -1$
, we have
![]() $(\mathfrak {B}_{0})^{*}=L^{1}_{a }(dA_{\alpha })$
under the integral pairing
$(\mathfrak {B}_{0})^{*}=L^{1}_{a }(dA_{\alpha })$
under the integral pairing
Here,
![]() $ \mathfrak {B}_{0}$
is equipped with the norm
$ \mathfrak {B}_{0}$
is equipped with the norm
![]() $\|f\| = |f(0)| + \|f\|_{ \mathfrak {B}_{0}}.$
$\|f\| = |f(0)| + \|f\|_{ \mathfrak {B}_{0}}.$
It is clear that
![]() $L^{1}_{a }(dA_{0})=A^{1}({\mathbb D}),$
the classical Bergman space.
$L^{1}_{a }(dA_{0})=A^{1}({\mathbb D}),$
the classical Bergman space.
In general, if
![]() $B(\Omega )$
is not reflexive, then the set of evaluation functions may not span the dual space of
$B(\Omega )$
is not reflexive, then the set of evaluation functions may not span the dual space of
![]() $B(\Omega ).$
For example, it is well known that
$B(\Omega ).$
For example, it is well known that
![]() $A^{1}(\mathbb {D}),$
the Bergman space on
$A^{1}(\mathbb {D}),$
the Bergman space on
![]() $\mathbb {D},$
is not reflexive; its dual space is the Bloch space
$\mathbb {D},$
is not reflexive; its dual space is the Bloch space
![]() $\mathfrak {B}$
(see [Reference Hedenmalm, Kirenblum and Zhu3]). The following proposition verifies our conclusion.
$\mathfrak {B}$
(see [Reference Hedenmalm, Kirenblum and Zhu3]). The following proposition verifies our conclusion.
Proposition 2.7. Suppose
![]() $A^{1}(\mathbb {D})$
is the Bergman space on the unit disc
$A^{1}(\mathbb {D})$
is the Bergman space on the unit disc
![]() $\mathbb {D}$
in the complex plane
$\mathbb {D}$
in the complex plane
![]() $\mathbb {C}.$
Then for any
$\mathbb {C}.$
Then for any
![]() $z\in \mathbb {D} ,$
the evaluation function
$z\in \mathbb {D} ,$
the evaluation function
![]() $K_{z}$
is bounded on
$K_{z}$
is bounded on
![]() $A^{1}(\mathbb {D}),$
and
$A^{1}(\mathbb {D}),$
and
Proof. It is obvious that
![]() $K_{z}$
is bounded for any
$K_{z}$
is bounded for any
![]() $z\in \mathbb {D}.$
We prove that
$z\in \mathbb {D}.$
We prove that
and further,
![]() $ \bigvee _{z\in \mathbb {D} }\{K_{z}\}\subsetneqq \mathfrak {B}. $
$ \bigvee _{z\in \mathbb {D} }\{K_{z}\}\subsetneqq \mathfrak {B}. $
It is clear that for any
![]() $z\in {\mathbb D}, K_{z}\in \mathfrak {B}_0.$
Hence,
$z\in {\mathbb D}, K_{z}\in \mathfrak {B}_0.$
Hence,
![]() $ \bigvee _{z\in \mathbb {D} }\{K_{z}\}\subset \mathfrak {B}_0. $
If
$ \bigvee _{z\in \mathbb {D} }\{K_{z}\}\subset \mathfrak {B}_0. $
If
![]() $ \bigvee _{z\in \mathbb {D} }\{K_{z}\}\neq \mathfrak {B}_0, $
then there is a nonzero
$ \bigvee _{z\in \mathbb {D} }\{K_{z}\}\neq \mathfrak {B}_0, $
then there is a nonzero
![]() $F\in (\mathfrak {B}_0)^{*}$
such that
$F\in (\mathfrak {B}_0)^{*}$
such that
![]() $F|_{\bigvee _{z\in \mathbb {D} }\{K_{z}\}}=0.$
By Lemma 2.6, there is an
$F|_{\bigvee _{z\in \mathbb {D} }\{K_{z}\}}=0.$
By Lemma 2.6, there is an
![]() $f\in L^{1}_{a }(dA_{\alpha })$
such that
$f\in L^{1}_{a }(dA_{\alpha })$
such that
In particular, for any
![]() $z\in {\mathbb D},$
$z\in {\mathbb D},$
This shows that
![]() $f(z)=0$
for any
$f(z)=0$
for any
![]() $z\in D,$
and hence
$z\in D,$
and hence
![]() $f=0.$
Consequently,
$f=0.$
Consequently,
![]() $F=0.$
This contradiction completes the proof.
$F=0.$
This contradiction completes the proof.
Proposition 2.8. Let
![]() $\Omega $
and
$\Omega $
and
![]() $B(\Omega )$
satisfy assumption (a). If
$B(\Omega )$
satisfy assumption (a). If
![]() $\{z_{k}\}\subset \Omega $
is a sequence that convergences to
$\{z_{k}\}\subset \Omega $
is a sequence that convergences to
![]() $w\in \Omega ,$
then
$w\in \Omega ,$
then
Proof. Assuming the contrary, then there is an
![]() $\epsilon _{0}>0$
and a sequence
$\epsilon _{0}>0$
and a sequence
![]() $\{f_{k}\}\subset (B(\Omega ))_{1}$
, the unit ball of
$\{f_{k}\}\subset (B(\Omega ))_{1}$
, the unit ball of
![]() $B(\Omega ),$
such that
$B(\Omega ),$
such that
That is,
Write
![]() $F_{k}=f_{k}^{**}\in [(B(\Omega ))^{**}]_{1},$
where
$F_{k}=f_{k}^{**}\in [(B(\Omega ))^{**}]_{1},$
where
![]() $(B(\Omega ))^{**}$
is the second dual space of
$(B(\Omega ))^{**}$
is the second dual space of
![]() $B(\Omega ), [(B(\Omega ))^{**}]_{1}$
is the unit ball of
$B(\Omega ), [(B(\Omega ))^{**}]_{1}$
is the unit ball of
![]() $(B(\Omega ))^{**}.$
Then there is a subsequence
$(B(\Omega ))^{**}.$
Then there is a subsequence
![]() $\{F_{k_{j}}\}$
such that
$\{F_{k_{j}}\}$
such that
![]() $F_{k_{j}}$
converges in the weak-star topology to
$F_{k_{j}}$
converges in the weak-star topology to
![]() $F_{0}\in (B(\Omega ))^{**}.$
In particular,
$F_{0}\in (B(\Omega ))^{**}.$
In particular,
However,
and hence there is a holomorphic function f on
![]() $\Omega $
such that
$\Omega $
such that
Assume
![]() $\mathcal {K}\subset \Omega $
is any compact subset of
$\mathcal {K}\subset \Omega $
is any compact subset of
![]() $\Omega ,$
then
$\Omega ,$
then
By Lemma 2.3, we know that
![]() $\{f_{k_{j}}\}$
is a normal family. Further,
$\{f_{k_{j}}\}$
is a normal family. Further,
This contradicts
![]() $|f_{k}(z_{k})-f_{k}(w)|\geq \epsilon _{0},$
which means
$|f_{k}(z_{k})-f_{k}(w)|\geq \epsilon _{0},$
which means
![]() $\|K_{z_{k}}-K_{w}\|\rightarrow 0 .$
$\|K_{z_{k}}-K_{w}\|\rightarrow 0 .$
According to an example of Manhas and Zhao in [Reference Manhas and Zhao4], it may happen in a reproducing kernel Hilbert space that as z tends to the boundary of
![]() $\Omega $
, the kernel functions satisfy
$\Omega $
, the kernel functions satisfy
![]() $\|K_{z}\|\rightarrow \infty $
but the normalized reproducing kernels
$\|K_{z}\|\rightarrow \infty $
but the normalized reproducing kernels
![]() $k_{z}$
do not converge to 0 weakly. However, if the polynomials are dense in the space, this phenomenon does not occur.
$k_{z}$
do not converge to 0 weakly. However, if the polynomials are dense in the space, this phenomenon does not occur.
Proposition 2.9. Let
![]() $\Omega $
and
$\Omega $
and
![]() $B(\Omega )$
satisfy assumption (a), and
$B(\Omega )$
satisfy assumption (a), and
![]() $P[\Omega ]$
, the ring of polynomials on
$P[\Omega ]$
, the ring of polynomials on
![]() $\Omega ,$
is dense in
$\Omega ,$
is dense in
![]() $B(\Omega ).$
For any sequence
$B(\Omega ).$
For any sequence
![]() $\{z_{k}\}\subset \Omega $
and
$\{z_{k}\}\subset \Omega $
and
![]() $\zeta \in \partial \Omega ,$
if
$\zeta \in \partial \Omega ,$
if
![]() $\|K_{z_{k}}\|\rightarrow \infty $
as
$\|K_{z_{k}}\|\rightarrow \infty $
as
![]() $z_{k}\rightarrow \zeta ,$
then
$z_{k}\rightarrow \zeta ,$
then
 $$ \begin{align*} k_{z_{k}}=\frac{K_{z_{k}}}{\|K_{z_{k}}\|}\stackrel{weak^{*}}{\longrightarrow} 0 \quad\text{as } k\rightarrow \infty. \end{align*} $$
$$ \begin{align*} k_{z_{k}}=\frac{K_{z_{k}}}{\|K_{z_{k}}\|}\stackrel{weak^{*}}{\longrightarrow} 0 \quad\text{as } k\rightarrow \infty. \end{align*} $$
Proof. Assuming
![]() $P\in P[\Omega ],$
it is obvious that
$P\in P[\Omega ],$
it is obvious that
![]() $K_{z_{k}}(P)\rightarrow P(\zeta )$
as
$K_{z_{k}}(P)\rightarrow P(\zeta )$
as
![]() $k\rightarrow \infty .$
Since
$k\rightarrow \infty .$
Since
![]() $\|K_{z_{k}}\|\rightarrow ~\infty ,$
$\|K_{z_{k}}\|\rightarrow ~\infty ,$
 $$ \begin{align*} k_{z_{k}}(P)=\frac{K_{z_{k}}}{\|K_{z_{k}}\|}(P)=\frac{P(z_{k})}{\|K_{z_{k}}\|}\rightarrow 0 \quad\text{as } k\to\infty. \end{align*} $$
$$ \begin{align*} k_{z_{k}}(P)=\frac{K_{z_{k}}}{\|K_{z_{k}}\|}(P)=\frac{P(z_{k})}{\|K_{z_{k}}\|}\rightarrow 0 \quad\text{as } k\to\infty. \end{align*} $$
For any
![]() $f\in B(\Omega ),$
take a sequence
$f\in B(\Omega ),$
take a sequence
![]() $\{P_{m}\}\subset P[\Omega ]$
such that
$\{P_{m}\}\subset P[\Omega ]$
such that
![]() $\|P_{m}-f\|\rightarrow 0$
as
$\|P_{m}-f\|\rightarrow 0$
as
![]() $m\rightarrow \infty .$
Then,
$m\rightarrow \infty .$
Then,
 $$ \begin{align*} |k_{z_{k}}(f)|&=\bigg|\frac{K_{z_{k}}}{\|K_{z_{k}}\|}(f)\bigg|\\ &\leq \bigg|\frac{K_{z_{k}}}{\|K_{z_{k}}\|}(f)-\frac{K_{z_{k}}}{\|K_{z_{k}}\|}(P_{m})\bigg| +\bigg|\frac{K_{z_{k}}}{\|K_{z_{k}}\|}(P_{m})\bigg|\\ &\leq \bigg\|\frac{K_{z_{k}}}{\|K_{z_{k}}\|}\bigg\|\cdot \|f-P_{m}\|+\bigg|\frac{K_{z_{k}}}{\|K_{z_{k}}\|}(P_{m})\bigg|\\ &= \|f-P_{m}\|+\bigg|\frac{K_{z_{k}}}{\|K_{z_{k}}\|}(P_{m})\bigg|. \end{align*} $$
$$ \begin{align*} |k_{z_{k}}(f)|&=\bigg|\frac{K_{z_{k}}}{\|K_{z_{k}}\|}(f)\bigg|\\ &\leq \bigg|\frac{K_{z_{k}}}{\|K_{z_{k}}\|}(f)-\frac{K_{z_{k}}}{\|K_{z_{k}}\|}(P_{m})\bigg| +\bigg|\frac{K_{z_{k}}}{\|K_{z_{k}}\|}(P_{m})\bigg|\\ &\leq \bigg\|\frac{K_{z_{k}}}{\|K_{z_{k}}\|}\bigg\|\cdot \|f-P_{m}\|+\bigg|\frac{K_{z_{k}}}{\|K_{z_{k}}\|}(P_{m})\bigg|\\ &= \|f-P_{m}\|+\bigg|\frac{K_{z_{k}}}{\|K_{z_{k}}\|}(P_{m})\bigg|. \end{align*} $$
This shows that
![]() $k_{z_{k}}(f)\rightarrow 0.$
That is,
$k_{z_{k}}(f)\rightarrow 0.$
That is,
 $$ \begin{align*} k_{z_{k}}=\frac{K_{z_{k}}}{\|K_{z_{k}}\|}\stackrel{\text{weak}^{*}}{\longrightarrow} 0 \quad\text{as } k\rightarrow \infty. \end{align*} $$
$$ \begin{align*} k_{z_{k}}=\frac{K_{z_{k}}}{\|K_{z_{k}}\|}\stackrel{\text{weak}^{*}}{\longrightarrow} 0 \quad\text{as } k\rightarrow \infty. \end{align*} $$
The proof is complete.
It should be noted that, similar to the reproducing kernel of the Hilbert space of analytic functions, the boundary behavior of the evaluation function on the Banach space of analytic functions depends on the structure of the space. For example, as we know, for
![]() $1\leq p<\infty ,$
if
$1\leq p<\infty ,$
if
![]() $\beta>n/p, $
then
$\beta>n/p, $
then
![]() $\|K_{z}\|$
, the norm of the evaluation function
$\|K_{z}\|$
, the norm of the evaluation function
![]() $K_{z}$
on Hardy–Sobolev space
$K_{z}$
on Hardy–Sobolev space
![]() $H_{\beta }^{p}$
(see [Reference Ortega and Fábrega5]), is bounded on
$H_{\beta }^{p}$
(see [Reference Ortega and Fábrega5]), is bounded on
![]() $ \mathbb {B}_{n} .$
As any function in
$ \mathbb {B}_{n} .$
As any function in
![]() $H_{\beta }^{p} (\mathbb {B}_{n})$
is continuous on the boundary of
$H_{\beta }^{p} (\mathbb {B}_{n})$
is continuous on the boundary of
![]() $\mathbb {B}_{n},$
it can be seen that when the sequence
$\mathbb {B}_{n},$
it can be seen that when the sequence
![]() $\{z_{k}\}$
in
$\{z_{k}\}$
in
![]() $\mathbb {B}_{n} $
converges to a point
$\mathbb {B}_{n} $
converges to a point
![]() $\zeta \in \partial \mathbb {B}_{n},$
then according to the norm in
$\zeta \in \partial \mathbb {B}_{n},$
then according to the norm in
![]() $H_{\beta }^{p} (\mathbb {B}_{n}), K_{z_{k}}$
converges to
$H_{\beta }^{p} (\mathbb {B}_{n}), K_{z_{k}}$
converges to
![]() $K_{\zeta },$
the evaluation function at
$K_{\zeta },$
the evaluation function at
![]() $\zeta .$
Thus,
$\zeta .$
Thus,
where
![]() $k_{z_{k}}= {K_{z_{k}}}/{\|K_{z_{k}}\|}, k_{\zeta } = {K_{\zeta }}/{\|K_{\zeta }\|}.$
If
$k_{z_{k}}= {K_{z_{k}}}/{\|K_{z_{k}}\|}, k_{\zeta } = {K_{\zeta }}/{\|K_{\zeta }\|}.$
If
![]() $\beta \leq n/p, $
then
$\beta \leq n/p, $
then
![]() $\|K_{z}\|\rightarrow \infty $
as
$\|K_{z}\|\rightarrow \infty $
as
![]() $z\rightarrow \partial \mathbb {B}_{n}$
and
$z\rightarrow \partial \mathbb {B}_{n}$
and
 $$ \begin{align*} k_{z}=\frac{K_{z}}{\|K_{z}\|}\stackrel{\text{weak}^{*}}{\longrightarrow} 0 \quad\text{as } z\rightarrow \partial \mathbb{B}_{n}. \end{align*} $$
$$ \begin{align*} k_{z}=\frac{K_{z}}{\|K_{z}\|}\stackrel{\text{weak}^{*}}{\longrightarrow} 0 \quad\text{as } z\rightarrow \partial \mathbb{B}_{n}. \end{align*} $$
3 Proof of Theorem 1.2
Proof. We need only to prove the sufficiency by Proposition 2.4. Assume
We prove that
![]() $(B(\Omega ))_{1}$
is weakly compact, and further
$(B(\Omega ))_{1}$
is weakly compact, and further
![]() $B(\Omega )$
is reflexive by Kakutani’s theorem. Assume
$B(\Omega )$
is reflexive by Kakutani’s theorem. Assume
![]() $\{f_{k}\}\subset (B(\Omega ))_{1}.$
By Lemma 2.3, there is a subsequence
$\{f_{k}\}\subset (B(\Omega ))_{1}.$
By Lemma 2.3, there is a subsequence
![]() $\{f_{k_{j}}\}$
such that
$\{f_{k_{j}}\}$
such that
![]() $f_{k_{j}}(z)\rightarrow f(z)$
uniformly on compact subsets of
$f_{k_{j}}(z)\rightarrow f(z)$
uniformly on compact subsets of
![]() $\Omega ,$
where f is a holomorphic function on
$\Omega ,$
where f is a holomorphic function on
![]() $\Omega .$
Thus, for any
$\Omega .$
Thus, for any
![]() $z\in \Omega ,$
$z\in \Omega ,$
This implies that
![]() $ \{K_{z}(f_{k_{j}})\}$
is convergent. Further, for any finite linear combination
$ \{K_{z}(f_{k_{j}})\}$
is convergent. Further, for any finite linear combination
![]() $\sum _{i=1}^{m}\alpha _{i}K_{z_{i}},$
$\sum _{i=1}^{m}\alpha _{i}K_{z_{i}},$
![]() $\{ \sum _{i=1}^{m}\alpha _{i}K_{z_{i}}(f_{k_{j}})\}$
is also convergent. Since
$\{ \sum _{i=1}^{m}\alpha _{i}K_{z_{i}}(f_{k_{j}})\}$
is also convergent. Since
we see that
![]() $\{F(f_{k_{j}})\}$
is convergent for any
$\{F(f_{k_{j}})\}$
is convergent for any
![]() $F\in (B(\Omega ))^{*}.$
Since F is chosen arbitrarily, we know that there is a
$F\in (B(\Omega ))^{*}.$
Since F is chosen arbitrarily, we know that there is a
![]() $G\in (B(\Omega ))^{**}$
such that
$G\in (B(\Omega ))^{**}$
such that
![]() $f_{k_{j}}^{**}(F)\rightarrow G(F)$
for any
$f_{k_{j}}^{**}(F)\rightarrow G(F)$
for any
![]() $F\in (B(\Omega ))^{*}$
since
$F\in (B(\Omega ))^{*}$
since
![]() $ (B(\Omega ))^{**}$
is weak-star compact. Writing
$ (B(\Omega ))^{**}$
is weak-star compact. Writing
![]() $f_{G}(z)=G(K_{z})$
for any
$f_{G}(z)=G(K_{z})$
for any
![]() $z\in \Omega ,$
we see that
$z\in \Omega ,$
we see that
Hence,
![]() $f_{G}$
is a holomorphic function. For any
$f_{G}$
is a holomorphic function. For any
![]() $F\in (B(\Omega ))^{*}, F(f_{G})$
is well defined and
$F\in (B(\Omega ))^{*}, F(f_{G})$
is well defined and
![]() $F(f_{k_{j}})\rightarrow F(f_{G}).$
By
$F(f_{k_{j}})\rightarrow F(f_{G}).$
By
![]() $ \bigvee _{z\in \Omega }\{K_{z}\}=(B(\Omega ))^{*}$
again, there exists a sequence
$ \bigvee _{z\in \Omega }\{K_{z}\}=(B(\Omega ))^{*}$
again, there exists a sequence
![]() $\{P_{m}\}\subset \bigvee _{z\in \Omega }\{K_{z}\}$
such that
$\{P_{m}\}\subset \bigvee _{z\in \Omega }\{K_{z}\}$
such that
 $$ \begin{align*} P_{m}=\sum_{i=1}^{k_{m}}\alpha_{i}^{(m)}K_{z_{i}^{(m)}}\rightarrow F\quad\text{in } (B(\Omega))^{*} \text{ as } m\to\infty. \end{align*} $$
$$ \begin{align*} P_{m}=\sum_{i=1}^{k_{m}}\alpha_{i}^{(m)}K_{z_{i}^{(m)}}\rightarrow F\quad\text{in } (B(\Omega))^{*} \text{ as } m\to\infty. \end{align*} $$
For arbitrary
![]() $\alpha \in \mathbb {C},$
since
$\alpha \in \mathbb {C},$
since
 $$ \begin{align*} F(\alpha f_{G})&=\lim_{m\rightarrow \infty }P_{m}(\alpha f_{G})\\ &=\alpha \lim_{m\rightarrow \infty }P_{m}( f_{G})\\ &=\alpha \lim_{m\rightarrow \infty }G(P_{m})\\ &=\alpha G(F)\\ &=\alpha F(f_{G}), \end{align*} $$
$$ \begin{align*} F(\alpha f_{G})&=\lim_{m\rightarrow \infty }P_{m}(\alpha f_{G})\\ &=\alpha \lim_{m\rightarrow \infty }P_{m}( f_{G})\\ &=\alpha \lim_{m\rightarrow \infty }G(P_{m})\\ &=\alpha G(F)\\ &=\alpha F(f_{G}), \end{align*} $$
we see that
![]() $F(\alpha f_{G})=\lim \limits _{m\rightarrow \infty }P_{m}(\alpha f_{G})$
is well defined and
$F(\alpha f_{G})=\lim \limits _{m\rightarrow \infty }P_{m}(\alpha f_{G})$
is well defined and
![]() $F(\alpha f_{G})=\alpha F(f_{G}).$
Define
$F(\alpha f_{G})=\alpha F(f_{G}).$
Define
Noting that
and
we get that
![]() $\|f_{G}\|<\infty .$
We now prove
$\|f_{G}\|<\infty .$
We now prove
![]() $f_{G}\in B(\Omega )$
by contradiction. Suppose
$f_{G}\in B(\Omega )$
by contradiction. Suppose
![]() $f_{G}\notin B(\Omega ),$
then we define
$f_{G}\notin B(\Omega ),$
then we define
the space spanned by
![]() $B(\Omega )$
and
$B(\Omega )$
and
![]() $f_{G}.$
That is,
$f_{G}.$
That is,
For any
![]() $g=f+\alpha f_{G},$
define the norm of g as
$g=f+\alpha f_{G},$
define the norm of g as
Then
![]() $\|\cdot \|_{1}|_{B(\Omega )}=\|\cdot \|.$
It is easy to check that
$\|\cdot \|_{1}|_{B(\Omega )}=\|\cdot \|.$
It is easy to check that
![]() $B^{*}=(B(\Omega ))^{*}.$
In fact, for any
$B^{*}=(B(\Omega ))^{*}.$
In fact, for any
![]() ${F\in (B(\Omega ))^{*}, F}$
is well defined on B since
${F\in (B(\Omega ))^{*}, F}$
is well defined on B since
![]() $F( f_{G})$
is well defined, and
$F( f_{G})$
is well defined, and
Hence,
![]() $F\in B^{*}.$
Conversely, if
$F\in B^{*}.$
Conversely, if
![]() $F\in B^{*},$
then for any
$F\in B^{*},$
then for any
![]() $f\in B(\Omega ), |F(f)|\leq \|F\|\|f\|_{1}=\|F\|\|f\|,$
this shows that
$f\in B(\Omega ), |F(f)|\leq \|F\|\|f\|_{1}=\|F\|\|f\|,$
this shows that
![]() $F\in (B(\Omega ))^{*}.$
By the assumption
$F\in (B(\Omega ))^{*}.$
By the assumption
![]() $f_{G}\notin B(\Omega ),$
there is an
$f_{G}\notin B(\Omega ),$
there is an
![]() $F_{0}\in B^{*}$
such that
$F_{0}\in B^{*}$
such that
![]() $F_{0}|_{B(\Omega )}=0$
, and
$F_{0}|_{B(\Omega )}=0$
, and
![]() $F_{0}(f_{G})\neq 0.$
However, we know that the sequence
$F_{0}(f_{G})\neq 0.$
However, we know that the sequence
![]() ${\{f_{k_{j}}\}\subset B(\Omega )}$
such that
${\{f_{k_{j}}\}\subset B(\Omega )}$
such that
![]() $F(f_{k_{j}})\rightarrow F(f_{G})$
for any
$F(f_{k_{j}})\rightarrow F(f_{G})$
for any
![]() $F\in (B(\Omega ))^{*}.$
This implies that
$F\in (B(\Omega ))^{*}.$
This implies that
![]() ${F_{0}(f_{G})=0}$
since
${F_{0}(f_{G})=0}$
since
![]() $B^{*}=(B(\Omega ))^{*}.$
This contradiction shows that
$B^{*}=(B(\Omega ))^{*}.$
This contradiction shows that
![]() $f_{G}\in B(\Omega ).$
Thus,
$f_{G}\in B(\Omega ).$
Thus,
![]() $G=f_{G}^{**}.$
This shows that
$G=f_{G}^{**}.$
This shows that
![]() $(B(\Omega ))_{1}$
is weakly compact, and then
$(B(\Omega ))_{1}$
is weakly compact, and then
![]() $ B(\Omega )$
must be reflexive by Kakutani’s theorem.
$ B(\Omega )$
must be reflexive by Kakutani’s theorem.
Now let U be any open subset or dense subset U of
![]() $\Omega .$
We are to prove that
$\Omega .$
We are to prove that
if and only if
We prove this argument by contradiction. Assume that
and there is an open subset or dense subset U of
![]() $\Omega $
with
$\Omega $
with
Then there is a nonzero
![]() $G\in (B(\Omega ))^{**}$
such that
$G\in (B(\Omega ))^{**}$
such that
![]() $G|_{ \bigvee _{z\in U}\{K_{z}\}}=0.$
Since
$G|_{ \bigvee _{z\in U}\{K_{z}\}}=0.$
Since
![]() $B(\Omega )$
is reflexive, there is a nonzero
$B(\Omega )$
is reflexive, there is a nonzero
![]() $g\in B(\Omega )$
such that
$g\in B(\Omega )$
such that
![]() $G=g^{**}.$
Thus, for any
$G=g^{**}.$
Thus, for any
![]() $z\in U,$
$z\in U,$
Further,
![]() $g=0$
on
$g=0$
on
![]() $\Omega $
by the uniqueness of the extension of holomorphic functions. The contradiction completes the proof.
$\Omega $
by the uniqueness of the extension of holomorphic functions. The contradiction completes the proof.
Remark 3.1. It is well known that the dual space of
![]() $H ^ {1}(\mathbb {D})$
is BMOA, the holomorphic function space with bounded mean oscillation. According to Theorem 1.2, the evaluation functions on
$H ^ {1}(\mathbb {D})$
is BMOA, the holomorphic function space with bounded mean oscillation. According to Theorem 1.2, the evaluation functions on
![]() $H ^ {1}(\mathbb {D})$
cannot span BMOA since
$H ^ {1}(\mathbb {D})$
cannot span BMOA since
![]() $H ^ {1}(\mathbb {D})$
is not reflexive. Some other classical holomorphic function spaces, such as the Dirichlet space
$H ^ {1}(\mathbb {D})$
is not reflexive. Some other classical holomorphic function spaces, such as the Dirichlet space
![]() $\mathfrak {D}^{1}(\mathbb {D})$
, the Hardy–Sobolev space
$\mathfrak {D}^{1}(\mathbb {D})$
, the Hardy–Sobolev space
![]() $H_{\beta }^{1}(\mathbb {D}) (\beta \in \mathbb {R}),$
are also nonreflexive, so the evaluation functions on them cannot span their dual spaces.
$H_{\beta }^{1}(\mathbb {D}) (\beta \in \mathbb {R}),$
are also nonreflexive, so the evaluation functions on them cannot span their dual spaces.
4 Further discussion
As we know, an evaluation function need not be well defined even in the Hilbert space of holomorphic functions. For instance, let
![]() $H^{2}(\beta )$
be the weighted Hardy space with weight
$H^{2}(\beta )$
be the weighted Hardy space with weight
![]() $\beta _{n}= {1}/{2^{n}}$
(see [Reference Shields7]). If we set
$\beta _{n}= {1}/{2^{n}}$
(see [Reference Shields7]). If we set
![]() $f(z)=\sum _{n=0}^{\infty }(\tfrac 43)^nz^{n},$
then
$f(z)=\sum _{n=0}^{\infty }(\tfrac 43)^nz^{n},$
then
 $$ \begin{align*} \|f\|=\sqrt{\sum_{n=0}^{\infty }\bigg(\frac{4}{3}\bigg)^{2n}\bigg(\frac{1}{2}\bigg)^{2n}} = \sqrt{\sum_{n=0}^{\infty}\bigg(\frac{2}{3}\bigg)^{2n}} < \infty. \end{align*} $$
$$ \begin{align*} \|f\|=\sqrt{\sum_{n=0}^{\infty }\bigg(\frac{4}{3}\bigg)^{2n}\bigg(\frac{1}{2}\bigg)^{2n}} = \sqrt{\sum_{n=0}^{\infty}\bigg(\frac{2}{3}\bigg)^{2n}} < \infty. \end{align*} $$
However,
![]() $K_{z_{0}}(f)=\sum _{n=0}^{\infty }({16}/{15})^{n}=\infty $
for
$K_{z_{0}}(f)=\sum _{n=0}^{\infty }({16}/{15})^{n}=\infty $
for
![]() $z_0=\tfrac 45$
, a point in the unit disc
$z_0=\tfrac 45$
, a point in the unit disc
![]() ${\mathbb D}$
, which means that for some
${\mathbb D}$
, which means that for some
![]() $z\in {\mathbb D}$
,
$z\in {\mathbb D}$
,
![]() $K_{z}$
is not well defined. This is why we have to make assumption (a).
$K_{z}$
is not well defined. This is why we have to make assumption (a).
An interesting phenomenon is that the evaluation functions may still span the whole dual space of the Banach space
![]() $B(\Omega )$
of analytic functions as long as the evaluation function is well defined and bounded on an open subset or dense subset of the domain
$B(\Omega )$
of analytic functions as long as the evaluation function is well defined and bounded on an open subset or dense subset of the domain
![]() $\Omega .$
In fact, we have the following theorem.
$\Omega .$
In fact, we have the following theorem.
Theorem 4.1. Let
![]() $B(\Omega )$
be a Banach space of holomorphic functions on a bounded connected domain
$B(\Omega )$
be a Banach space of holomorphic functions on a bounded connected domain
![]() $\Omega $
in
$\Omega $
in
![]() ${{\mathbb C}^n}$
. If there is an open subset or dense subset U of the domain
${{\mathbb C}^n}$
. If there is an open subset or dense subset U of the domain
![]() $\Omega $
such that
$\Omega $
such that
![]() $K_{z}$
is bounded on
$K_{z}$
is bounded on
![]() $B(\Omega ),$
then
$B(\Omega ),$
then
![]() $B(\Omega )$
is reflexive if and only if
$B(\Omega )$
is reflexive if and only if
Proof. The proof is similar to that of Theorem 1.2, but for convenience, we give the details here.
First, assume that
![]() $B(\Omega )$
is reflexive. Write
$B(\Omega )$
is reflexive. Write
![]() $M=\bigvee _{z\in U}\{K_{z}\},$
and if
$M=\bigvee _{z\in U}\{K_{z}\},$
and if
![]() $M\neq (B(\Omega ))^{*},$
then there is a nonzero
$M\neq (B(\Omega ))^{*},$
then there is a nonzero
![]() $F\in (B(\Omega ))^{**}$
such that
$F\in (B(\Omega ))^{**}$
such that
![]() $F|_M=0.$
Here,
$F|_M=0.$
Here,
![]() $(B(\Omega ))^{**}$
is the second dual space of
$(B(\Omega ))^{**}$
is the second dual space of
![]() $B(\Omega ).$
Since
$B(\Omega ).$
Since
![]() $B(\Omega )$
is reflexive, there is a nonzero function
$B(\Omega )$
is reflexive, there is a nonzero function
![]() $f\in B(\Omega )$
, which satisfies
$f\in B(\Omega )$
, which satisfies
![]() $F=f^{**}\in (B(\Omega ))^{**},$
and thus
$F=f^{**}\in (B(\Omega ))^{**},$
and thus
This implies that
![]() $f=0.$
Thus,
$f=0.$
Thus,
![]() $F=f^{**}=0,$
which contradicts
$F=f^{**}=0,$
which contradicts
![]() $F\neq 0.$
This contradiction means that
$F\neq 0.$
This contradiction means that
Second, if
we prove that
![]() $(B(\Omega ))_{1}$
is weakly compact and further
$(B(\Omega ))_{1}$
is weakly compact and further
![]() $B(\Omega )$
is reflexive by Kakutani’s theorem. Assume
$B(\Omega )$
is reflexive by Kakutani’s theorem. Assume
![]() $\{f_{k}\}\subset (B(\Omega ))_{1}.$
By Lemma 2.3, there is a subsequence
$\{f_{k}\}\subset (B(\Omega ))_{1}.$
By Lemma 2.3, there is a subsequence
![]() $\{f_{k_{j}}\}$
such that
$\{f_{k_{j}}\}$
such that
![]() $f_{k_{j}}(z)\rightarrow f(z)$
uniformly on compact subsets of
$f_{k_{j}}(z)\rightarrow f(z)$
uniformly on compact subsets of
![]() $\Omega ,$
where f is a holomorphic function on
$\Omega ,$
where f is a holomorphic function on
![]() $\Omega .$
Thus, for any
$\Omega .$
Thus, for any
![]() $z\in U ,$
$z\in U ,$
This implies that
![]() $ \{K_{z}(f_{k_{j}})\}$
is convergent. Further, for any finite linear combination
$ \{K_{z}(f_{k_{j}})\}$
is convergent. Further, for any finite linear combination
![]() $\sum _{i=1}^{m}\alpha _{i}K_{z_{i}},$
$\sum _{i=1}^{m}\alpha _{i}K_{z_{i}},$
![]() $\{ \sum _{i=1}^{m}\alpha _{i}K_{z_{i}}(f_{k_{j}})\}$
is also convergent. Since
$\{ \sum _{i=1}^{m}\alpha _{i}K_{z_{i}}(f_{k_{j}})\}$
is also convergent. Since
we see that
![]() $\{F(f_{k_{j}})\}$
is convergent for any
$\{F(f_{k_{j}})\}$
is convergent for any
![]() $F\in (B(\Omega ))^{*}.$
Since F is chosen arbitrarily, we know that there is a
$F\in (B(\Omega ))^{*}.$
Since F is chosen arbitrarily, we know that there is a
![]() $G\in (B(\Omega ))^{**}$
such that
$G\in (B(\Omega ))^{**}$
such that
![]() $f_{k_{j}}^{**}(F)\rightarrow G(F)$
for any
$f_{k_{j}}^{**}(F)\rightarrow G(F)$
for any
![]() $F\in (B(\Omega ))^{*}$
as
$F\in (B(\Omega ))^{*}$
as
![]() $ (B(\Omega ))^{**}$
is weak-star compact. Writing
$ (B(\Omega ))^{**}$
is weak-star compact. Writing
![]() $f_{G}(z)=G(K_{z})$
for any
$f_{G}(z)=G(K_{z})$
for any
![]() $z\in U ,$
$z\in U ,$
Hence,
![]() $f_{G}$
is a holomorphic function on U, and f is the holomorphic extension of
$f_{G}$
is a holomorphic function on U, and f is the holomorphic extension of
![]() $f_{G}.$
Thus, for any
$f_{G}.$
Thus, for any
![]() $F\in (B(\Omega ))^{*}, F(f_{G})$
is well defined, and
$F\in (B(\Omega ))^{*}, F(f_{G})$
is well defined, and
![]() $F(f_{k_{j}})\rightarrow F(f_{G}).$
By
$F(f_{k_{j}})\rightarrow F(f_{G}).$
By
![]() $ {\bigvee _{z\in U}\{K_{z}\}=(B(\Omega ))^{*}}$
again, there exists a sequence
$ {\bigvee _{z\in U}\{K_{z}\}=(B(\Omega ))^{*}}$
again, there exists a sequence
![]() $\{P_{m}\}\subset \bigvee _{z\in U}\{K_{z}\}$
such that
$\{P_{m}\}\subset \bigvee _{z\in U}\{K_{z}\}$
such that
 $$ \begin{align*} P_{m}=\sum_{i=1}^{k_{m}}\alpha_{i}^{(m)}K_{z_{i}^{(m)}}\rightarrow F \quad\text{in } (B(\Omega))^{*} \text{ as } m\to\infty. \end{align*} $$
$$ \begin{align*} P_{m}=\sum_{i=1}^{k_{m}}\alpha_{i}^{(m)}K_{z_{i}^{(m)}}\rightarrow F \quad\text{in } (B(\Omega))^{*} \text{ as } m\to\infty. \end{align*} $$
For arbitrary
![]() $\alpha \in \mathbb {C},$
since
$\alpha \in \mathbb {C},$
since
 $$ \begin{align*} F(\alpha f_{G})&=\lim_{m\rightarrow \infty }P_{m}(\alpha f_{G})\\ &=\alpha \lim_{m\rightarrow \infty }P_{m}( f_{G})\\ &=\alpha \lim_{m\rightarrow \infty }G(P_{m})\\ &=\alpha G(F)\\ &=\alpha F(f_{G}), \end{align*} $$
$$ \begin{align*} F(\alpha f_{G})&=\lim_{m\rightarrow \infty }P_{m}(\alpha f_{G})\\ &=\alpha \lim_{m\rightarrow \infty }P_{m}( f_{G})\\ &=\alpha \lim_{m\rightarrow \infty }G(P_{m})\\ &=\alpha G(F)\\ &=\alpha F(f_{G}), \end{align*} $$
we see that
![]() $F(\alpha f_{G})=\lim \limits _{m\rightarrow \infty }P_{m}(\alpha f_{G})$
is well defined, and
$F(\alpha f_{G})=\lim \limits _{m\rightarrow \infty }P_{m}(\alpha f_{G})$
is well defined, and
![]() $F(\alpha f_{G})=\alpha F(f_{G}).$
Define
$F(\alpha f_{G})=\alpha F(f_{G}).$
Define
Note that
and
we get that
![]() $\|f_{G}\|<\infty .$
We now prove
$\|f_{G}\|<\infty .$
We now prove
![]() $f_{G}\in B(\Omega )$
by contradiction. Suppose
$f_{G}\in B(\Omega )$
by contradiction. Suppose
![]() $f_{G}\notin B(\Omega ),$
then we define
$f_{G}\notin B(\Omega ),$
then we define
the space spanned by
![]() $B(\Omega )$
and
$B(\Omega )$
and
![]() $f_{G}.$
That is,
$f_{G}.$
That is,
For any
![]() $g=f+\alpha f_{G},$
define the norm of g as
$g=f+\alpha f_{G},$
define the norm of g as
Then
![]() $\|\cdot \|_{1}|_{B(\Omega )}=\|\cdot \|.$
It is easy to check that
$\|\cdot \|_{1}|_{B(\Omega )}=\|\cdot \|.$
It is easy to check that
![]() $B^{*}=(B(\Omega ))^{*}.$
In fact, for any
$B^{*}=(B(\Omega ))^{*}.$
In fact, for any
![]() ${F\in (B(\Omega ))^{*}, F}$
is well defined on B since
${F\in (B(\Omega ))^{*}, F}$
is well defined on B since
![]() $F( f_{G})$
is well defined, and
$F( f_{G})$
is well defined, and
Hence,
![]() $F\in B^{*}.$
Conversely, if
$F\in B^{*}.$
Conversely, if
![]() $F\in B^{*},$
then for any
$F\in B^{*},$
then for any
![]() $f\in B(\Omega ), |F(f)|\leq \|F\|\|f\|_{1}=\|F\|\|f\|,$
which shows that
$f\in B(\Omega ), |F(f)|\leq \|F\|\|f\|_{1}=\|F\|\|f\|,$
which shows that
![]() $F\in (B(\Omega ))^{*}.$
By the assumption
$F\in (B(\Omega ))^{*}.$
By the assumption
![]() $f_{G}\notin B(\Omega ),$
there is an
$f_{G}\notin B(\Omega ),$
there is an
![]() ${F_{0}\in B^{*}}$
such that
${F_{0}\in B^{*}}$
such that
![]() $F_{0}|_{B(\Omega )}=0$
and
$F_{0}|_{B(\Omega )}=0$
and
![]() $F_{0}(f_{G})\neq 0.$
However, we know that there is a sequence
$F_{0}(f_{G})\neq 0.$
However, we know that there is a sequence
![]() $\{f_{k_{j}}\}\subset B(\Omega )$
such that
$\{f_{k_{j}}\}\subset B(\Omega )$
such that
![]() $F(f_{k_{j}})\rightarrow F(f_{G})$
for any
$F(f_{k_{j}})\rightarrow F(f_{G})$
for any
![]() $F\in (B(\Omega ))^{*}.$
This implies that
$F\in (B(\Omega ))^{*}.$
This implies that
![]() ${F_{0}(f_{G})=0}$
since
${F_{0}(f_{G})=0}$
since
![]() $B^{*}=(B(\Omega ))^{*}.$
This contradiction shows that
$B^{*}=(B(\Omega ))^{*}.$
This contradiction shows that
![]() $f_{G}\in B(\Omega ).$
Thus,
$f_{G}\in B(\Omega ).$
Thus,
![]() $G=f_{G}^{**}.$
This shows that
$G=f_{G}^{**}.$
This shows that
![]() $(B(\Omega ))_{1}$
is weakly compact and then
$(B(\Omega ))_{1}$
is weakly compact and then
![]() $ B(\Omega )$
must be reflexive by Kakutani’s theorem. This completes the proof.
$ B(\Omega )$
must be reflexive by Kakutani’s theorem. This completes the proof.
Remark 4.2. We know that
![]() $K_z$
is well defined on the weighted Hardy space
$K_z$
is well defined on the weighted Hardy space
![]() $H^2(\beta )$
with weight
$H^2(\beta )$
with weight
![]() $\beta _n= {1}/{2^n}$
for
$\beta _n= {1}/{2^n}$
for
![]() $z\in U=\{z\in {\mathbb D}||z|<\tfrac 12\}.$
Although the functions in
$z\in U=\{z\in {\mathbb D}||z|<\tfrac 12\}.$
Although the functions in
![]() $H^2(\beta )$
are not necessarily holomorphic on the entire unit disk, still
$H^2(\beta )$
are not necessarily holomorphic on the entire unit disk, still
In fact, if
then there exists a nonzero
![]() $f\in H^2(\beta )$
such that
$f\in H^2(\beta )$
such that
Thus,
Further,
![]() $f=0.$
This contradiction shows that
$f=0.$
This contradiction shows that
![]() $ \bigvee _{z\in U}\{K_{z}\}= H^2(\beta ). $
$ \bigvee _{z\in U}\{K_{z}\}= H^2(\beta ). $
However, there also exists a weighted Hardy space such that for any nonzero
![]() $z\in {\mathbb D}$
,
$z\in {\mathbb D}$
,
![]() $K_z$
cannot be well defined on it. For instance, let
$K_z$
cannot be well defined on it. For instance, let
![]() $H^2(\beta )$
be the weighted Hardy space with the weight
$H^2(\beta )$
be the weighted Hardy space with the weight
![]() $\beta _n= {1}/{n^n}.$
Then, for arbitrary
$\beta _n= {1}/{n^n}.$
Then, for arbitrary
![]() $z\in {\mathbb D}-\{0\}, K_z$
cannot be well defined on
$z\in {\mathbb D}-\{0\}, K_z$
cannot be well defined on
![]() $H^2(\beta ).$
Hence, Theorem 4.1 fails although
$H^2(\beta ).$
Hence, Theorem 4.1 fails although
![]() $H^2(\beta )$
is a Hilbert space.
$H^2(\beta )$
is a Hilbert space.
Remark 4.2 shows that a function in the weighted Hardy space
![]() $H^2(\beta )$
may not necessarily be a holomorphic function on the entire unit disk, and it may even be undefined on a disk without center, or a space composed only of a formal power series. When the weight
$H^2(\beta )$
may not necessarily be a holomorphic function on the entire unit disk, and it may even be undefined on a disk without center, or a space composed only of a formal power series. When the weight
![]() $\beta =\{\beta _n\}_{n=0}^{\infty }$
satisfies some conditions, the space
$\beta =\{\beta _n\}_{n=0}^{\infty }$
satisfies some conditions, the space
![]() $H^2(\beta )$
is indeed composed of holomorphic functions on the disk.
$H^2(\beta )$
is indeed composed of holomorphic functions on the disk.
Proposition 4.3. Assuming
![]() $H^2(\beta )$
is the weighted Hardy space with weight
$H^2(\beta )$
is the weighted Hardy space with weight
![]() $\beta =\{\beta (n)\}$
, then
$\beta =\{\beta (n)\}$
, then
![]() $K_{z}$
is bounded for arbitrary
$K_{z}$
is bounded for arbitrary
![]() $z\in {\mathbb D}$
if and only if
$z\in {\mathbb D}$
if and only if
Proof. Assume for any
![]() $z\in {\mathbb D}$
,
$z\in {\mathbb D}$
,
![]() $K_{z}$
is bounded on
$K_{z}$
is bounded on
![]() $H^2_{\beta }({\mathbb D})$
. Let
$H^2_{\beta }({\mathbb D})$
. Let
![]() $e_{n}(z)= {z^{n}}/{\| z^{n}\|} = ({1}/{\beta (n)}) z^{n}$
, then
$e_{n}(z)= {z^{n}}/{\| z^{n}\|} = ({1}/{\beta (n)}) z^{n}$
, then
![]() $K_{z}(w)=\sum _{n=0}^{\infty }\overline {e_{n}(z)}e_{n}(w)$
(see [Reference Zhu8]) and
$K_{z}(w)=\sum _{n=0}^{\infty }\overline {e_{n}(z)}e_{n}(w)$
(see [Reference Zhu8]) and
 $$ \begin{align*} \| K_{z}\|^2=\langle K_{z}, K_{z}\rangle=\sum_{n=0}^{\infty}|e_{n}(z)|^{2}=\sum_{n=0}^{\infty}\frac{1}{\beta^{2}(n)}|z^{n}|^{2}<+\infty,\end{align*} $$
$$ \begin{align*} \| K_{z}\|^2=\langle K_{z}, K_{z}\rangle=\sum_{n=0}^{\infty}|e_{n}(z)|^{2}=\sum_{n=0}^{\infty}\frac{1}{\beta^{2}(n)}|z^{n}|^{2}<+\infty,\end{align*} $$
and thus,
 $$ \begin{align*} \lim_{n\rightarrow \infty}\frac{1}{\sqrt[n]{\beta^{2}(n)}}|z|^{2}\leq 1. \end{align*} $$
$$ \begin{align*} \lim_{n\rightarrow \infty}\frac{1}{\sqrt[n]{\beta^{2}(n)}}|z|^{2}\leq 1. \end{align*} $$
That is,
![]() $\lim _{n\rightarrow \infty }\sqrt [n]{\beta ^{2}(n)}\geq |z|^{2},$
which means
$\lim _{n\rightarrow \infty }\sqrt [n]{\beta ^{2}(n)}\geq |z|^{2},$
which means
![]() $\lim _{n\rightarrow \infty } \sqrt [n]{\beta ^{2}(n)}\geq 1$
by the arbitrariness of z. Further,
$\lim _{n\rightarrow \infty } \sqrt [n]{\beta ^{2}(n)}\geq 1$
by the arbitrariness of z. Further,
![]() $\lim _{n\rightarrow \infty }\sqrt [n]{\beta (n)}\geq 1$
.
$\lim _{n\rightarrow \infty }\sqrt [n]{\beta (n)}\geq 1$
.
Conversely, assume
![]() $\lim _{n\rightarrow \infty }\sqrt [n]{\beta (n)}\geq 1$
, then
$\lim _{n\rightarrow \infty }\sqrt [n]{\beta (n)}\geq 1$
, then
![]() $\lim _{n\rightarrow \infty } ({1}/{\sqrt [n]{\beta (n)}}) |z|<1$
for arbitrary
$\lim _{n\rightarrow \infty } ({1}/{\sqrt [n]{\beta (n)}}) |z|<1$
for arbitrary
![]() $z\in {\mathbb D}$
. Hence,
$z\in {\mathbb D}$
. Hence,
 $$ \begin{align*} \sum_{n=0}^{\infty }\frac{1}{\beta^{2}(n)}|z^n|^{2}<+\infty, \end{align*} $$
$$ \begin{align*} \sum_{n=0}^{\infty }\frac{1}{\beta^{2}(n)}|z^n|^{2}<+\infty, \end{align*} $$
that is,
![]() $\|K_{z}\|^{2}<+\infty .$
$\|K_{z}\|^{2}<+\infty .$
The following proposition is almost obvious, but it tells us that the boundedness of the evaluation function on the Banach space of the holomorphic functions depends on some regularity of the functions in the space, that is, the convergence of the function sequence in the space means the pointwise convergence of this function sequence.
Proposition 4.4. Let
![]() $\Omega $
be a bounded domain in
$\Omega $
be a bounded domain in
![]() ${\mathbb C}^{n}$
,
${\mathbb C}^{n}$
,
![]() $B(\Omega )$
is the Banach space of holomorphic functions on
$B(\Omega )$
is the Banach space of holomorphic functions on
![]() $\Omega $
. Then for
$\Omega $
. Then for
![]() $z\in \Omega $
, the evaluation function
$z\in \Omega $
, the evaluation function
![]() $K_{z}$
is bounded on
$K_{z}$
is bounded on
![]() $B(\Omega )$
if and only if for arbitrary
$B(\Omega )$
if and only if for arbitrary
![]() $f_{n}$
, f in
$f_{n}$
, f in
![]() $B(\Omega )$
with
$B(\Omega )$
with
![]() $\| f_{n}-f\|\rightarrow 0$
,
$\| f_{n}-f\|\rightarrow 0$
,
Proof. Since for any
![]() $f\in B(\Omega ), f$
is holomorphic on
$f\in B(\Omega ), f$
is holomorphic on
![]() $\Omega ,$
we see that
$\Omega ,$
we see that
![]() $|f(z)|<\infty ,$
which means that
$|f(z)|<\infty ,$
which means that
![]() $K_{z}$
is well defined for arbitrary
$K_{z}$
is well defined for arbitrary
![]() $z\in \Omega .$
If
$z\in \Omega .$
If
![]() $K_{z}$
is bounded, then it is clear that
$K_{z}$
is bounded, then it is clear that
![]() $f_{n}(z)\rightarrow f(z)$
if
$f_{n}(z)\rightarrow f(z)$
if
![]() $\| f_{n}-f\|\rightarrow 0$
.
$\| f_{n}-f\|\rightarrow 0$
.
Conversely, assume
![]() $z\in \Omega $
and for any
$z\in \Omega $
and for any
![]() $\{f_{n}\}\subseteq B(\Omega ), f\in B(\Omega )$
with
$\{f_{n}\}\subseteq B(\Omega ), f\in B(\Omega )$
with
![]() $\| f_{n}-f\|\rightarrow 0$
, we have
$\| f_{n}-f\|\rightarrow 0$
, we have
![]() $f_{n}(z)\rightarrow f(z)$
, that is,
$f_{n}(z)\rightarrow f(z)$
, that is,
![]() $K_z(f_n)\rightarrow K_z(f).$
Then
$K_z(f_n)\rightarrow K_z(f).$
Then
![]() $K_z$
is continuous on
$K_z$
is continuous on
![]() $B(\Omega ).$
Note the continuity of
$B(\Omega ).$
Note the continuity of
![]() $K_z$
is equivalent to the boundedness of
$K_z$
is equivalent to the boundedness of
![]() $K_z$
on
$K_z$
on
![]() $B(\Omega )$
, we see that
$B(\Omega )$
, we see that
![]() $K_{z}$
is bounded.
$K_{z}$
is bounded.
A natural question is as follows. Assume
![]() $\Omega $
is a bounded domain in
$\Omega $
is a bounded domain in
![]() ${\mathbb C}^{n}$
, and
${\mathbb C}^{n}$
, and
![]() $B(\Omega )$
is the Banach space of holomorphic functions on
$B(\Omega )$
is the Banach space of holomorphic functions on
![]() $\Omega $
. Then is
$\Omega $
. Then is
![]() $K_z$
necessarily bounded?
$K_z$
necessarily bounded?
We know that if
![]() $B(\Omega )$
is the Banach space of holomorphic functions on
$B(\Omega )$
is the Banach space of holomorphic functions on
![]() $\Omega $
, due to the fact that any function in
$\Omega $
, due to the fact that any function in
![]() $B(\Omega )$
is holomorphic on the entire
$B(\Omega )$
is holomorphic on the entire
![]() $\Omega $
, then for arbitrary
$\Omega $
, then for arbitrary
![]() ${z\in \Omega , K_z}$
is well defined. Although in the case of common classical holomorphic function spaces, evaluation functionals are bounded as long as
${z\in \Omega , K_z}$
is well defined. Although in the case of common classical holomorphic function spaces, evaluation functionals are bounded as long as
![]() $K_z$
is well defined, we do not know whether
$K_z$
is well defined, we do not know whether
![]() $K_z$
must be bounded on the general Banach space of holomorphic functions on
$K_z$
must be bounded on the general Banach space of holomorphic functions on
![]() $\Omega $
if
$\Omega $
if
![]() $K_z$
is well defined.
$K_z$
is well defined.
Acknowledgments
The authors would like to thank Prof. L. Yan and Prof. K. Zhu for helpful discussions.