Hostname: page-component-669899f699-g7b4s Total loading time: 0 Render date: 2025-04-26T23:11:59.789Z Has data issue: false hasContentIssue false
  • English
  • Français

Solid bases and functorial constructions for (p-)Banach spaces of analytic functions

Part of: Series expansions Special classes of linear operators

Published online by Cambridge University Press:  09 September 2024

Guozheng Cheng ,
Xiang Fang ,
Chao Liu  and
Yufeng Lu
Guozheng Cheng
Affiliation:
School of Mathematical Sciences, Dalian University of Technology, Dalian, P. R. China
Xiang Fang
Affiliation:
Department of Mathematics, National Central University, Chungli, Taiwan
Chao Liu*
Affiliation:
School of Mathematics and Statistics, Yunnan University, Kunming, P. R. China
Yufeng Lu
Affiliation:
School of Mathematical Sciences, Dalian University of Technology, Dalian, P. R. China
*
Corresponding author: Chao Liu, email: chaoliu@ynu.edu.cn
Get access Rights & Permissions [Opens in a new window]

Abstract

Motivated by new examples of functional Banach spaces over the unit disk, arising as the symbol spaces in the study of random analytic functions, for which the monomials 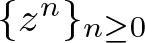$\{z^n\}_{n\geq 0}$ exhibit features of an unconditional basis yet they often don’t even form a Schauder basis, we introduce a notion called solid basis for Banach spaces and p-Banach spaces and study its properties. Besides justifying the rich existence of solid bases, we study their relationship with unconditional bases, the weak-star convergence of Taylor polynomials, the problem of a solid span and the curious roles played by c0. The two features of this work are as follows: (1) during the process, we are led to revisit the axioms satisfied by a typical Banach space of analytic functions over the unit disk, leading to a notion of 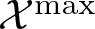$\mathcal{X}^\mathrm{max}$ (and 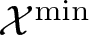$\mathcal{X}^\mathrm{min}$), as well as a number of related functorial constructions, which are of independent interests; (2) the main interests of solid basis lie in the case of non-separable (p-)Banach spaces, such as BMOA and the Bloch space instead of VMOA and the little Bloch space.

Keywords

random analytic functionsnorm convergence of Taylor polynomialssolid spacesBMOABloch spacesunconditional bases

MSC classification

Primary: 30B20: Random power series 47B37: Operators on special spaces (weighted shifts, operators on sequence spaces, etc.)
Type
Research Article
Information
Proceedings of the Edinburgh Mathematical Society , Volume 67 , Issue 4 , November 2024 , pp. 1013 - 1044
Check for updates
Copyright
© The Author(s), 2024. Published by Cambridge University Press on Behalf of The Edinburgh Mathematical Society.

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Article purchase

Temporarily unavailable

References

Albiac, F., Ansorena, J. L., Berná, P. M. and Wojtaszczyk, P., Greedy approximation for biorthogonal systems in quasi-Banach spaces, Dissertationes Math. 560 (2021), 1–88.Google Scholar
Anderson, J. M., Clunie, J. and Pommerenke, C., On Bloch functions and normal functions, J. Reine Angew. Math. 270 (1974), 12–37.Google Scholar
Anderson, J. M. and Shields, A. L., Coefficient multipliers of Bloch functions, Trans. Amer. Math. Soc. 224(2) (1976), 255–265.CrossRefGoogle Scholar
Arendt, W. and Nikolski, N., Vector-valued holomorphic functions revisited, Math. Z. 234(4) (2000), 777–805.CrossRefGoogle Scholar
Bennett, G., Stegenga, D. A. and Timoney, R. M., Coefficients of Bloch and Lipschitz functions, Illinois J. Math. 25(3) (1981), 520–531.CrossRefGoogle Scholar
Billard, P., Séries de Fourier aléatoirement bornées, continues, uniformément convergentes, Studia Math. 22 (1963), 309–329.CrossRefGoogle Scholar
Blasco, O. and Pavlović, M., Coefficient multipliers on Banach spaces of analytic functions, Rev. Mat. Iberoam. 27(2) (2011), 415–447.CrossRefGoogle Scholar
Blasco, O. and Stylogiannis, G., Lipschitz-type conditions on homogeneous Banach spaces of analytic functions, J. Math. Anal. Appl. 445(1) (2017), 612–630.CrossRefGoogle Scholar
Brown, L. and Shields, A. L., Cyclic vectors in the Dirichlet space, Trans. Amer. Math. Soc. 285(1) (1984), 269–303.CrossRefGoogle Scholar
Cheng, G., Fang, X., Guo, K., and Liu, C., A Gaussian version of Littlewood’s theorem for random power series, Proc. Amer. Math. Soc. 150 (8) (2022), 3525–3536.CrossRefGoogle Scholar
Cheng, G., Fang, X., and Liu, C., A Littlewood-type theorem for random Bergman functions, Int. Math. Res. Not. IMRN 2022 (14) (2022), 11056–11091.CrossRefGoogle Scholar
Cheng, G., Fang, X., Liu, C. and Lu, Y., Random Dirichlet multipliers and Littlewood-type theorems, (2021), preprint.Google Scholar
Cochran, W. G., Shapiro, J. H. and Ullrich, D. C., Random Dirichlet functions: multipliers and smoothness, Canad. J. Math. 45(2) (1993), 255–268.CrossRefGoogle Scholar
Conway, J. B., A Course in Functional Analysis. Graduate Texts in Mathematics, second, Volume 96, (Springer-Verlag, New York, 1990).Google Scholar
Diestel, J., Sequences and Series in Banach Spaces. Graduate Texts in Mathematics, Volume 92, (Springer-Verlag, New York, 1984).Google Scholar
Duren, P., Random series and bounded mean oscillation, Michigan Math. J. 32 (1) (1985), 81–86.CrossRefGoogle Scholar
Duren, P. L., Theory of Hp spaces. Pure and Applied Mathematics, Volume 38, (Academic Press, New York-London, 1970).Google Scholar
Gao, F., A characterization of random Bloch functions, J. Math. Anal. Appl. 252 (2) (2000), 959–966.CrossRefGoogle Scholar
Hedenmalm, H., Korenblum, B. and Zhu, K., Theory of Bergman Spaces. Graduate Texts in Mathematics, Volume 199, (Springer-Verlag, New York, 2000).Google Scholar
Hytönen, T., van Neerven, J., Veraar, M. and Weis, L., Analysis in Banach Spaces. Vol. I. Martingales and Littlewood-Paley Theory. Ergebnisse der Mathematik und Ihrer Grenzgebiete. 3. Folge, Volume 63, (Springer, Cham, 2016).Google Scholar
Hytönen, T., van Neerven, J., Veraar, M. and Weis, L., Analysis in Banach Spaces. Vol. II. Probabilistic Methods and Opeator Theory, Ergebnisse der Mathematik und Ihrer Grenzgebiete. 3. Folge, Volume 67, (Springer, Cham, 2017).Google Scholar
Kahane, J. -P., Some Random Series of Functions. Of Cambridge Studies in Advanced Mathematics, Volume 5, (Cambridge University Press, Cambridge, second ed, 1985).Google Scholar
Kalton, N. J., Rademacher series and decoupling, New York J. Math. 11 (2005), 563–595.Google Scholar
Konyagin, S., Queffélec, H., Saksman, E., and Seip, K., Riesz projection and bounded mean oscillation for Dirichlet series, Studia Math. 262 (2) (2022), 121–149.CrossRefGoogle Scholar
Li, D. and Queffélec, H., Introduction to Banach Spaces: Analysis and Probability. Vol. 1. Cambridge Studies in Advanced Mathematics, Volume 1, (Cambridge University Press, Cambridge, 2018) :.Google Scholar
Li, D. and Queffélec, H., Introduction to Banach Spaces: Analysis and Probability. Vol. 2, Cambridge Studies in Advanced Mathematics, Volume 167, (Cambridge University Press, Cambridge, 2018).Google Scholar
Lindenstrauss, J. and Tzafriri, L., Classical Banach Spaces. I, Sequence Spaces. Ergebnisse der Mathematik und Ihrer Grenzgebiete, Volume 92, (Springer-Verlag, Berlin-New York, 1977).Google Scholar
Littlewood, J. E., On the mean values of power series, J. London Math. Soc. 2(25) (1926), 328–337.CrossRefGoogle Scholar
Littlewood, J. E., On the mean values of power series (II), J. London Math. Soc. 5(3) (1930), 179–182.CrossRefGoogle Scholar
Liu, C., Multipliers for Dirichlet type spaces by randomization, Banach J. Math. Anal. 14 (3) (2020), 935–949.CrossRefGoogle Scholar
Marcus, M. B. and Pisier, G., Necessary and Sufficient Conditions for the Uniform Convergence of Random Trigonometric Series. Of Lecture Notes Series, Volume 50, (Aarhus Universitet, Matematisk Institut, Aarhus, 1978).Google Scholar
Marcus, M. B. and Pisier, G., Random Fourier Series with Applications to Harmonic Analysis. Annals of Mathematics Studies, Volume 101, (University of Tokyo Press, Tokyo, 1981).Google Scholar
Megginson, R. E., An Introduction to Banach Space Theory. Graduate Texts in Mathematics, Volume 183, (Springer-Verlag, New York, 1998).Google Scholar
Nishry, A. and Paquette, E., Gaussian analytic functions of bounded mean oscillation, Anal. PDE 16(1) (2023), 89–117.CrossRefGoogle Scholar
Paley, R. E. A. C. and Zygmund, A.. On some series of functions (1), Proc. Camb. Phil. Soc., 26 (1930), 337–357.CrossRefGoogle Scholar
Paley, R. E. A. C. & Zygmund, A.. On some series of functions (2), Proc. Camb. Phil. Soc., 26 (1930), 458–474.CrossRefGoogle Scholar
Rudin, W., Functional Analysis. International Series in Pure and Applied Mathematics, second, (McGraw-Hill, Inc, New York, 1991).Google Scholar
Salem, R. and Zygmund, A., Some properties of trigonometric series whose terms have random signs, Acta Math. 91 (1954), 245–301.CrossRefGoogle Scholar
Sledd, W. T., Random series which are BMO or Bloch, Michigan Math. J. 28 (3) (1981), 259–266.CrossRefGoogle Scholar
Sledd, W. T., and Stegenga, D. A., An H 1 multiplier theorem, Ark. Mat. 19 (2) (1981), 265–270.CrossRefGoogle Scholar
Taylor, A. E., Banach spaces of functions analytic in the unit circle I, Studia Math. 11 (1950), 145–170.CrossRefGoogle Scholar
Wulan, H., Random power series with bounded mean oscillation characteristics, Acta Math. Sci. (Chinese) 14 (4) (1994), 451–457.Google Scholar
Zhu, K., Duality of Bloch spaces and norm convergence of Taylor series, Michigan Math. J. 38(1) (1991), 89–101.CrossRefGoogle Scholar