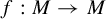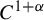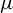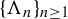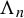1 Introduction
Hyperbolic approximation plays a fundamental role in the study of smooth dynamical systems. Roughly speaking, for a hyperbolic ergodic measure
![]() $\mu $
of positive entropy, one can always find a sequence of horseshoes
$\mu $
of positive entropy, one can always find a sequence of horseshoes
![]() $\{\Lambda _n\}_{n\ge 1}$
so that the dynamical quantities on them are close to the corresponding ones of the measure
$\{\Lambda _n\}_{n\ge 1}$
so that the dynamical quantities on them are close to the corresponding ones of the measure
![]() $\mu $
. Such results can be traced back to the landmark work by Katok [Reference Katok18] or Katok and Hasselblatt [Reference Katok and Hasselblatt19]. An earlier related work was obtained by Misiurewicz and Szlenk [Reference Misiurewicz and Szlenk25] for piecewise continuous and monotone maps of interval. For more results of this type, we would like to refer the reader to [Reference Avila, Crovisier and Wilkinson2, Reference Cao, Pesin and Zhao8, Reference Chung10, Reference Gelfert14, Reference Gelfert15, Reference Persson and Schmeling27, Reference Przytycki and Urbański30, Reference Wang, Qu and Cao34, Reference Yang35] and the references therein.
$\mu $
. Such results can be traced back to the landmark work by Katok [Reference Katok18] or Katok and Hasselblatt [Reference Katok and Hasselblatt19]. An earlier related work was obtained by Misiurewicz and Szlenk [Reference Misiurewicz and Szlenk25] for piecewise continuous and monotone maps of interval. For more results of this type, we would like to refer the reader to [Reference Avila, Crovisier and Wilkinson2, Reference Cao, Pesin and Zhao8, Reference Chung10, Reference Gelfert14, Reference Gelfert15, Reference Persson and Schmeling27, Reference Przytycki and Urbański30, Reference Wang, Qu and Cao34, Reference Yang35] and the references therein.
From the point of dimension theory of dynamical systems, it is natural and non-trivial to use Hausdorff dimension to estimate how large that part of the dynamics described by these horseshoes is. If
![]() $\mu $
is an ergodic hyperbolic Sinai–Ruelle–Bowen (SRB) measure of a surface diffeomorphism, Mendoza [Reference Mendoza24] proved that the Hausdorff dimension of the horseshoes on the unstable manifolds approaches to one. For the higher dimensional case, Sánchez-Salas [Reference Sánchez-Salas31] proved that the measure
$\mu $
is an ergodic hyperbolic Sinai–Ruelle–Bowen (SRB) measure of a surface diffeomorphism, Mendoza [Reference Mendoza24] proved that the Hausdorff dimension of the horseshoes on the unstable manifolds approaches to one. For the higher dimensional case, Sánchez-Salas [Reference Sánchez-Salas31] proved that the measure
![]() $\mu $
can be approximated in the weak topology by ergodic measures supported on the horseshoes
$\mu $
can be approximated in the weak topology by ergodic measures supported on the horseshoes
![]() $\{\Lambda _n\}_{n\ge 1}$
. Moreover, he established some interesting results concerning the Hausdorff dimension of the horseshoes. Using Cao, Pesin and Zhao’s ideas [Reference Cao, Pesin and Zhao8], Wang, Qu and Cao [Reference Wang, Qu and Cao34] generalized Mendoza’s result [Reference Mendoza24] for diffeomorphisms on a higher dimensional manifold. In fact, the authors proved that the Hausdorff dimension of the horseshoes
$\{\Lambda _n\}_{n\ge 1}$
. Moreover, he established some interesting results concerning the Hausdorff dimension of the horseshoes. Using Cao, Pesin and Zhao’s ideas [Reference Cao, Pesin and Zhao8], Wang, Qu and Cao [Reference Wang, Qu and Cao34] generalized Mendoza’s result [Reference Mendoza24] for diffeomorphisms on a higher dimensional manifold. In fact, the authors proved that the Hausdorff dimension of the horseshoes
![]() $\{\Lambda _n\}_{n\ge 1}$
on the unstable manifold tends to the dimension of the unstable manifold. Furthermore, if the stable direction is one dimension, then the Hausdorff dimension of the measure
$\{\Lambda _n\}_{n\ge 1}$
on the unstable manifold tends to the dimension of the unstable manifold. Furthermore, if the stable direction is one dimension, then the Hausdorff dimension of the measure
![]() $\mu $
can be approximated by the Hausdorff dimension of
$\mu $
can be approximated by the Hausdorff dimension of
![]() $\{\Lambda _n\}_{n\ge 1}$
. The first result in this paper shows that the Lyapunov dimension of
$\{\Lambda _n\}_{n\ge 1}$
. The first result in this paper shows that the Lyapunov dimension of
![]() $\mu $
(see equation (1) for the definition) can be approximated gradually by the Hausdorff dimension of a sequence of hyperbolic sets
$\mu $
(see equation (1) for the definition) can be approximated gradually by the Hausdorff dimension of a sequence of hyperbolic sets
![]() $\{\Lambda _n\}_{n\geq 1}$
, provided that the stable direction is one or
$\{\Lambda _n\}_{n\geq 1}$
, provided that the stable direction is one or
![]() $\mu $
satisfies the Pesin’s entropy formula in the stable direction.
$\mu $
satisfies the Pesin’s entropy formula in the stable direction.
The main motivation of our first result is the study of the Kaplan–Yorke conjecture [Reference Frederickson, Kaplan, Yorke and Yorke13]. To be more precise, let
![]() $f: M\rightarrow M$
be a
$f: M\rightarrow M$
be a
![]() $C^{1+\alpha }$
diffeomorphism on an
$C^{1+\alpha }$
diffeomorphism on an
![]() $m_0$
-dimensional compact smooth Riemannian manifold M and let
$m_0$
-dimensional compact smooth Riemannian manifold M and let
![]() $\mu $
be a hyperbolic ergodic f-invariant probability measure. For
$\mu $
be a hyperbolic ergodic f-invariant probability measure. For
![]() $x\in M$
, the pointwise dimension of
$x\in M$
, the pointwise dimension of
![]() $\mu $
at x is defined by
$\mu $
at x is defined by
provided the limit exists, where
![]() $B(x,r)$
denotes the ball of radius r centred at x. A measure
$B(x,r)$
denotes the ball of radius r centred at x. A measure
![]() $\mu $
is called exact dimensional if
$\mu $
is called exact dimensional if
![]() $d_\mu (x)$
is constant almost everywhere and let
$d_\mu (x)$
is constant almost everywhere and let
![]() $\dim _H\mu $
denote the Hausdorff dimension of the measure
$\dim _H\mu $
denote the Hausdorff dimension of the measure
![]() $\mu $
(see [Reference Pesin29] for the detailed definition). Young [Reference Young36] proved that almost all the known characteristics of dimension type of a measure
$\mu $
(see [Reference Pesin29] for the detailed definition). Young [Reference Young36] proved that almost all the known characteristics of dimension type of a measure
![]() $\mu $
coincide if
$\mu $
coincide if
![]() $\mu $
is exact dimensional. This indicates that it is very important to show the exactness of a measure in dimension theory of dynamical systems.
$\mu $
is exact dimensional. This indicates that it is very important to show the exactness of a measure in dimension theory of dynamical systems.
Let
![]() $\Gamma $
be the set of points which are regular in the sense of Oseledec multiplicative ergodic theorem [Reference Oseledets26]. For every
$\Gamma $
be the set of points which are regular in the sense of Oseledec multiplicative ergodic theorem [Reference Oseledets26]. For every
![]() $x\in \Gamma $
, denote the Lyapunov exponents of f at x by
$x\in \Gamma $
, denote the Lyapunov exponents of f at x by
where u and
![]() $s:=m_0-u$
are the dimension of the unstable and stable subspaces of
$s:=m_0-u$
are the dimension of the unstable and stable subspaces of
![]() $T_xM$
, respectively.
$T_xM$
, respectively.
The Lyapunov dimension of
![]() $\mu $
is defined as follows:
$\mu $
is defined as follows:

where
![]() $\ell =\max \{i: \unicode{x3bb} _1(\mu )+\unicode{x3bb} _2(\mu )+\cdots +\unicode{x3bb} _i(\mu )\geq 0\}$
. It is not difficult to show that
$\ell =\max \{i: \unicode{x3bb} _1(\mu )+\unicode{x3bb} _2(\mu )+\cdots +\unicode{x3bb} _i(\mu )\geq 0\}$
. It is not difficult to show that
![]() $\dim _H\mu \leq \dim _L\mu $
, e.g., see [Reference Shu32, Proposition 4.2] for details. It was conjectured in [Reference Frederickson, Kaplan, Yorke and Yorke13] that if
$\dim _H\mu \leq \dim _L\mu $
, e.g., see [Reference Shu32, Proposition 4.2] for details. It was conjectured in [Reference Frederickson, Kaplan, Yorke and Yorke13] that if
![]() $\mu $
is an SRB measure, which is absolutely continuous along the unstable leaves, then generically,
$\mu $
is an SRB measure, which is absolutely continuous along the unstable leaves, then generically,
By Young’s dimension formula in [Reference Young36], the conjecture is true if M is a surface. This paper proves the conjecture in the higher dimensional case under the assumption that the stable direction is one or
![]() $\mu $
satisfies the ‘Pesin’s entropy formula in the stable direction’. Moreover, the measure
$\mu $
satisfies the ‘Pesin’s entropy formula in the stable direction’. Moreover, the measure
![]() $\mu $
is exact dimensional in this case (see Theorem A).
$\mu $
is exact dimensional in this case (see Theorem A).
To summarize, let
![]() $h_{\mu}(f )$
denote the metric entropy of
$h_{\mu}(f )$
denote the metric entropy of
![]() $f$
with respect to
$f$
with respect to
![]() $\mu$
(see Walters’ book [Reference Walters33] for details of metric entropy), the first result is stated as the following theorem.
$\mu$
(see Walters’ book [Reference Walters33] for details of metric entropy), the first result is stated as the following theorem.
Theorem A. Let
![]() $f: M\rightarrow M$
be a
$f: M\rightarrow M$
be a
![]() $C^{1+\alpha }$
diffeomorphism on an
$C^{1+\alpha }$
diffeomorphism on an
![]() $m_0$
-dimensional smooth compact Riemannian manifold M and
$m_0$
-dimensional smooth compact Riemannian manifold M and
![]() $\mu $
a hyperbolic ergodic SRB measure on M. Assume that either one of the following properties holds:
$\mu $
a hyperbolic ergodic SRB measure on M. Assume that either one of the following properties holds:
-
(i)
 $\mu $
has a one-dimensional stable manifold;
$\mu $
has a one-dimensional stable manifold; -
(ii)
 $\mu $
satisfies
$\mu $
satisfies
 $h_\mu (f)=-\unicode{x3bb} _{u+1}(\mu )-\unicode{x3bb} _{u+2}(\mu )-\cdots -\unicode{x3bb} _{m_0}(\mu )$
,
$h_\mu (f)=-\unicode{x3bb} _{u+1}(\mu )-\unicode{x3bb} _{u+2}(\mu )-\cdots -\unicode{x3bb} _{m_0}(\mu )$
,
then
![]() $\dim _H\mu =\dim _L\mu $
. Furthermore, there exists a sequence of hyperbolic sets
$\dim _H\mu =\dim _L\mu $
. Furthermore, there exists a sequence of hyperbolic sets
![]() $\{\Lambda _{n}\}$
such that
$\{\Lambda _{n}\}$
such that
Example 1.1. Let
![]() $f: M\rightarrow M$
be a
$f: M\rightarrow M$
be a
![]() $C^{1+\alpha }$
diffeomorphism on an
$C^{1+\alpha }$
diffeomorphism on an
![]() $m_0$
-dimensional smooth compact Riemannian manifold M. Assume that the volume measure
$m_0$
-dimensional smooth compact Riemannian manifold M. Assume that the volume measure
![]() $\varrho $
is f-invariant ergodic and hyperbolic. Let
$\varrho $
is f-invariant ergodic and hyperbolic. Let
denote the Lyapunov exponents of f with respect to
![]() $\varrho $
. By Pesin’s entropy formula [Reference Pesin28] (see also [Reference Mañé23] for a simple proof), one has that
$\varrho $
. By Pesin’s entropy formula [Reference Pesin28] (see also [Reference Mañé23] for a simple proof), one has that
where the second equality holds since f is volume-preserving. By Theorem A, there exists a sequence of hyperbolic sets
![]() $\{\Lambda _{n}\}$
such that
$\{\Lambda _{n}\}$
such that
since
![]() $\dim _{L}\mu =m_0$
in this case.
$\dim _{L}\mu =m_0$
in this case.
Ledrappier [Reference Ledrappier20] proved the existence of the pointwise dimension of each SRB measure. For a hyperbolic invariant measure
![]() $\mu $
of a
$\mu $
of a
![]() $C^2$
(or
$C^2$
(or
![]() $C^{1+\alpha }$
) diffeomorphism f of a smooth compact Riemannian manifold M without boundary, Ledrappier and Young [Reference Ledrappier and Young22] proved the existence of dimension of
$C^{1+\alpha }$
) diffeomorphism f of a smooth compact Riemannian manifold M without boundary, Ledrappier and Young [Reference Ledrappier and Young22] proved the existence of dimension of
![]() $\mu $
on stable/unstable manifolds, and that the upper pointwise dimension of
$\mu $
on stable/unstable manifolds, and that the upper pointwise dimension of
![]() $\mu $
is upper bounded by the sum of the dimension of
$\mu $
is upper bounded by the sum of the dimension of
![]() $\mu $
on stable and unstable manifolds. Later, Barreira, Pesin and Schmeling [Reference Barreira, Pesin and Schmeling4] proved that the lower pointwise dimension of
$\mu $
on stable and unstable manifolds. Later, Barreira, Pesin and Schmeling [Reference Barreira, Pesin and Schmeling4] proved that the lower pointwise dimension of
![]() $\mu $
is also lower bounded by the sum of the dimension of
$\mu $
is also lower bounded by the sum of the dimension of
![]() $\mu $
on stable and unstable manifolds. This showed that the measure
$\mu $
on stable and unstable manifolds. This showed that the measure
![]() $\mu $
is exact dimensional, which finally solves the Eckmann–Ruelle conjecture.
$\mu $
is exact dimensional, which finally solves the Eckmann–Ruelle conjecture.
Motivated by the work in [Reference Fang, Cao and Zhao12], where it is proved that the unique solution of the measure-theoretic pressure is exactly the dimension of an invariant measure supported on an average conformal repeller, the second result in this paper shows that the unique solution of measure-theoretic pressure gives an upper bound of the dimension of a hyperbolic ergodic measure
![]() $\mu $
on stable/unstable manifolds. To be more precise, we introduce some notation first. For each
$\mu $
on stable/unstable manifolds. To be more precise, we introduce some notation first. For each
![]() $x\in M$
and
$x\in M$
and
![]() $n\geq 1$
, consider the differentiable operator
$n\geq 1$
, consider the differentiable operator
![]() ${D_xf^n: T_xM \to T_{f^n(x)}M}$
and denote the singular values of
${D_xf^n: T_xM \to T_{f^n(x)}M}$
and denote the singular values of
![]() $D_xf^n$
in the decreasing order by
$D_xf^n$
in the decreasing order by
Recall that u and s are the dimension of the unstable and stable subspace of
![]() $T_xM$
, respectively. For every
$T_xM$
, respectively. For every
![]() $t\in [0,u]$
, define
$t\in [0,u]$
, define
 $$ \begin{align*} \phi^t(x,f^n):=\sum_{i=1}^{[t]}\log\alpha_i(x,f^n)+(t-[t])\log\alpha_{[t]+1}(x,f^n) \end{align*} $$
$$ \begin{align*} \phi^t(x,f^n):=\sum_{i=1}^{[t]}\log\alpha_i(x,f^n)+(t-[t])\log\alpha_{[t]+1}(x,f^n) \end{align*} $$
and
 $$ \begin{align*} \psi^t(x,f^n):=\sum_{i=u-[t]+1}^{u}\log\alpha_i(x,f^n)+(t-[t])\log\alpha_{u-[t]}(x,f^n). \end{align*} $$
$$ \begin{align*} \psi^t(x,f^n):=\sum_{i=u-[t]+1}^{u}\log\alpha_i(x,f^n)+(t-[t])\log\alpha_{u-[t]}(x,f^n). \end{align*} $$
For every
![]() $t\in [0, s]$
, define
$t\in [0, s]$
, define
 $$ \begin{align*}\varphi^t(x, f^n) := \sum_{i=u+1}^{u+[t]} \log\alpha_i(x,f^n) + (t-[t])\log\alpha_{u+[t]+1}(x, f^n).\end{align*} $$
$$ \begin{align*}\varphi^t(x, f^n) := \sum_{i=u+1}^{u+[t]} \log\alpha_i(x,f^n) + (t-[t])\log\alpha_{u+[t]+1}(x, f^n).\end{align*} $$
Since f is smooth, the functions
![]() $x\mapsto \alpha _i(x, f^n)$
,
$x\mapsto \alpha _i(x, f^n)$
,
![]() $x\mapsto \phi ^t(x,f^n)$
,
$x\mapsto \phi ^t(x,f^n)$
,
![]() $x\mapsto \psi ^t(x,f^n)$
and
$x\mapsto \psi ^t(x,f^n)$
and
![]() $x\mapsto \varphi ^t(x,f^n)$
are continuous. It is easy to see that the sequences of functions
$x\mapsto \varphi ^t(x,f^n)$
are continuous. It is easy to see that the sequences of functions
are super-additive and
are sub-additive. Ledrappier and Young [Reference Ledrappier and Young22] proved the existence of stable and unstable pointwise dimension
![]() $d_\mu ^s(x)$
,
$d_\mu ^s(x)$
,
![]() $d_\mu ^u(x)$
of a hyperbolic ergodic measure
$d_\mu ^u(x)$
of a hyperbolic ergodic measure
![]() $\mu $
for
$\mu $
for
![]() $\mu $
-almost every (a.e.) x. The following theorem shows that the unique solution of the sub-additive measure-theoretic pressure equation
$\mu $
-almost every (a.e.) x. The following theorem shows that the unique solution of the sub-additive measure-theoretic pressure equation
is an upper bound for the unstable (stable) dimension of
![]() $\mu $
, see §2 for the definitions of measure-theoretic pressure and stable and unstable dimension of an invariant measure.
$\mu $
, see §2 for the definitions of measure-theoretic pressure and stable and unstable dimension of an invariant measure.
Theorem B. Suppose
![]() $f: M\rightarrow M$
is a
$f: M\rightarrow M$
is a
![]() $C^{1+\alpha }$
diffeomorphism on an
$C^{1+\alpha }$
diffeomorphism on an
![]() $m_0$
-dimensional smooth compact Riemannian manifold M and
$m_0$
-dimensional smooth compact Riemannian manifold M and
![]() $\mu $
is a hyperbolic ergodic measure on M. Then one has
$\mu $
is a hyperbolic ergodic measure on M. Then one has
where
![]() $t_u^*$
and
$t_u^*$
and
![]() $t_s^*$
are the unique solutions of the equations
$t_s^*$
are the unique solutions of the equations
![]() $P_\mu (f, \Psi _f(t))=0$
and
$P_\mu (f, \Psi _f(t))=0$
and
![]() $P_\mu (f,\Xi _f(t))=0$
, respectively.
$P_\mu (f,\Xi _f(t))=0$
, respectively.
For each hyperbolic ergodic measure
![]() $\mu $
of positive entropy, there exists a sequence of hyperbolic sets
$\mu $
of positive entropy, there exists a sequence of hyperbolic sets
![]() $\{\Lambda _n\}_{n\geq 1}$
such that the dynamical quantities on
$\{\Lambda _n\}_{n\geq 1}$
such that the dynamical quantities on
![]() $\Lambda _n$
gradually approach to those of the measure
$\Lambda _n$
gradually approach to those of the measure
![]() $\mu $
(see Theorem 2.4). Since the hyperbolic sets
$\mu $
(see Theorem 2.4). Since the hyperbolic sets
![]() $\{\Lambda _n\}_{n\geq 1}$
are non-conformal, it is difficult to compute their Hausdorff dimension. Following the approach described in [Reference Cao, Pesin and Zhao8], this paper introduces the concept of Carathéodory singular dimension of a hyperbolic set on unstable manifolds (see §2 for the detailed definition). The third result of this paper shows that the zero of the super-additive/sub-additive measure-theoretic pressure
$\{\Lambda _n\}_{n\geq 1}$
are non-conformal, it is difficult to compute their Hausdorff dimension. Following the approach described in [Reference Cao, Pesin and Zhao8], this paper introduces the concept of Carathéodory singular dimension of a hyperbolic set on unstable manifolds (see §2 for the detailed definition). The third result of this paper shows that the zero of the super-additive/sub-additive measure-theoretic pressure
![]() $P_\mu (f,\Phi _f(t))/P_\mu (f,\Psi _f(t))$
gives a lower/upper bound of the Carathéodory singular dimension of
$P_\mu (f,\Phi _f(t))/P_\mu (f,\Psi _f(t))$
gives a lower/upper bound of the Carathéodory singular dimension of
![]() $\Lambda _n$
on the unstable manifold. In addition, if
$\Lambda _n$
on the unstable manifold. In addition, if
![]() $\mu $
is an SRB measure, then the Carathéodory singular dimension of
$\mu $
is an SRB measure, then the Carathéodory singular dimension of
![]() $\Lambda _n$
on the unstable manifold tends to the dimension of the unstable manifold, and the Lyapunov dimension of
$\Lambda _n$
on the unstable manifold tends to the dimension of the unstable manifold, and the Lyapunov dimension of
![]() $\mu $
is exactly the sum of
$\mu $
is exactly the sum of
![]() $t_s^*$
and the dimension of the unstable manifold, where
$t_s^*$
and the dimension of the unstable manifold, where
![]() $t_s^*$
is the unique root of the equation
$t_s^*$
is the unique root of the equation
![]() $P_\mu (f,\Xi _f(t))=0$
.
$P_\mu (f,\Xi _f(t))=0$
.
Theorem C. Let
![]() $f: M\rightarrow M$
be a
$f: M\rightarrow M$
be a
![]() $C^{1+\alpha }$
diffeomorphism on an
$C^{1+\alpha }$
diffeomorphism on an
![]() $m_0$
-dimensional smooth compact Riemannian manifold M, and let
$m_0$
-dimensional smooth compact Riemannian manifold M, and let
![]() $\mu $
be a hyperbolic ergodic measure on M. Then there exists a sequence of hyperbolic sets
$\mu $
be a hyperbolic ergodic measure on M. Then there exists a sequence of hyperbolic sets
![]() $\{\Lambda _{\varepsilon }\}_{\varepsilon \ge 0}$
such that the following properties hold:
$\{\Lambda _{\varepsilon }\}_{\varepsilon \ge 0}$
such that the following properties hold:
-
(i)
 ${\liminf _{\varepsilon \to 0}\dim _C^{\Phi _f} (\Lambda _\varepsilon \cap W^u_{\mathrm {loc}}(x,f)) \geq t_{u*}}$
for every
${\liminf _{\varepsilon \to 0}\dim _C^{\Phi _f} (\Lambda _\varepsilon \cap W^u_{\mathrm {loc}}(x,f)) \geq t_{u*}}$
for every
 $x\in \Lambda _\varepsilon $
, where
$x\in \Lambda _\varepsilon $
, where
 $t_{u*}$
is the unique root of the equation
$t_{u*}$
is the unique root of the equation
 $P_\mu (f, \Phi _f(t))=0$
;
$P_\mu (f, \Phi _f(t))=0$
; -
(ii)
 ${\limsup _{\varepsilon \to 0}\dim _C^{\Psi _f} (\Lambda _\varepsilon \cap W^u_{\mathrm {loc}}(x,f)) \leq t_{u}^*}$
for every
${\limsup _{\varepsilon \to 0}\dim _C^{\Psi _f} (\Lambda _\varepsilon \cap W^u_{\mathrm {loc}}(x,f)) \leq t_{u}^*}$
for every
 $x\in \Lambda _\varepsilon $
, where
$x\in \Lambda _\varepsilon $
, where
 $t_{u}^*$
is the unique root of the equation
$t_{u}^*$
is the unique root of the equation
 $P_\mu (f, \Psi _f(t))=0$
.
$P_\mu (f, \Psi _f(t))=0$
.
Furthermore, if
![]() $\mu $
is an SRB measure, then
$\mu $
is an SRB measure, then
![]() $\dim _L\mu =u+t_s^*$
and
$\dim _L\mu =u+t_s^*$
and
for every
![]() $x\in \Lambda _\varepsilon $
, where u is the dimension of the unstable manifold and
$x\in \Lambda _\varepsilon $
, where u is the dimension of the unstable manifold and
![]() $t_s^*$
is the unique root of the equation
$t_s^*$
is the unique root of the equation
![]() $P_\mu (f,\Xi _f(t))=0$
.
$P_\mu (f,\Xi _f(t))=0$
.
The paper is organized as follows. Section 2 gives some basic notions and properties, including Hausdorff dimension, hyperbolic set, pressure and singular dimension. All the proofs of the main results will be given in §3.
2 Preliminaries
In this section, we will recall some definitions and preliminary results which are used in the proofs of the main results.
2.1 Hyperbolic set
Let f be a
![]() $C^{1+\alpha }$
diffeomorphism on an
$C^{1+\alpha }$
diffeomorphism on an
![]() $m_0$
-dimensional smooth compact Riemannian manifold M. We say an f-invariant compact subset
$m_0$
-dimensional smooth compact Riemannian manifold M. We say an f-invariant compact subset
![]() $\Lambda \subset M$
is a hyperbolic set if for any
$\Lambda \subset M$
is a hyperbolic set if for any
![]() $x\in \Lambda $
, the tangent space admits a decomposition
$x\in \Lambda $
, the tangent space admits a decomposition
![]() ${T_{x}M=E^{s}(x)\oplus E^{u}(x)}$
such that the following properties hold:
${T_{x}M=E^{s}(x)\oplus E^{u}(x)}$
such that the following properties hold:
-
(1) the splitting is
 $Df$
-invariant, that is, for every
$Df$
-invariant, that is, for every
 $x\in \Lambda $
,
$x\in \Lambda $
,
 $D_{x}f E^{\sigma }(x)=E^{\sigma }(f(x))$
for
$D_{x}f E^{\sigma }(x)=E^{\sigma }(f(x))$
for
 $\sigma =s,u$
;
$\sigma =s,u$
; -
(2) the stable subspace
 $E^{s}(x)$
is uniformly contracting and the unstable subspace
$E^{s}(x)$
is uniformly contracting and the unstable subspace
 $E^{u}(x)$
is uniformly expanding in the sense that there are constants
$E^{u}(x)$
is uniformly expanding in the sense that there are constants
 $C\geq 1$
and
$C\geq 1$
and
 $0<\chi <1$
such that for every
$0<\chi <1$
such that for every
 $n\geq 0$
and
$n\geq 0$
and
 $v^{\sigma }\in E^{\sigma }(x)$
(
$v^{\sigma }\in E^{\sigma }(x)$
(
 $\sigma =s$
or u), we have
$\sigma =s$
or u), we have  $$ \begin{align*}\|D_{x}f^{n}v^{s}\|\leq C\chi^{n}\|v^{s}\|\quad \text{and} \quad \|D_{x}f^{-n}v^{u}\|\leq C\chi^{n}\|v^{u}\|.\end{align*} $$
$$ \begin{align*}\|D_{x}f^{n}v^{s}\|\leq C\chi^{n}\|v^{s}\|\quad \text{and} \quad \|D_{x}f^{-n}v^{u}\|\leq C\chi^{n}\|v^{u}\|.\end{align*} $$
Recall that a hyperbolic set
![]() $\Lambda $
is locally maximal if there exists an open neighbourhood U of
$\Lambda $
is locally maximal if there exists an open neighbourhood U of
![]() $\Lambda $
such that
$\Lambda $
such that
![]() $\Lambda =\bigcap _{n\in \mathbb {Z}}f^{n}(U)$
, and a diffeomorphism f is called topologically transitive on
$\Lambda =\bigcap _{n\in \mathbb {Z}}f^{n}(U)$
, and a diffeomorphism f is called topologically transitive on
![]() $\Lambda $
if for every two non-empty (relative) open subsets
$\Lambda $
if for every two non-empty (relative) open subsets
![]() $U,V\subset \Lambda $
, there exists
$U,V\subset \Lambda $
, there exists
![]() $n>0$
such that
$n>0$
such that
![]() $f^n(U)\cap V\neq \emptyset $
. Given a point
$f^n(U)\cap V\neq \emptyset $
. Given a point
![]() $x\in \Lambda $
, for each small
$x\in \Lambda $
, for each small
![]() $\beta>0$
, the local stable and unstable manifolds at the point x are defined as follows:
$\beta>0$
, the local stable and unstable manifolds at the point x are defined as follows:
and
The global stable and unstable sets of
![]() $x\in \Lambda $
are given as follows:
$x\in \Lambda $
are given as follows:
Let
![]() $d^s/d^u$
be the metric induced by the Riemannian structure on the stable/unstable manifold
$d^s/d^u$
be the metric induced by the Riemannian structure on the stable/unstable manifold
![]() $W^s/W^u$
.
$W^s/W^u$
.
2.2 Dimension
Let X be a compact Riemannian manifold with a Riemannian metric. Given a subset Z of X, for
![]() $s\geq 0$
and
$s\geq 0$
and
![]() $\delta>0$
, define
$\delta>0$
, define
 $$ \begin{align*} \mathcal{H}_{\delta}^{s}(Z) := \inf \bigg\{\!\sum_{i}|U_i|^s: \ Z\subset \bigcup_{i}U_i,~|U_i|\leq \delta \text{ for all } i\bigg\}, \end{align*} $$
$$ \begin{align*} \mathcal{H}_{\delta}^{s}(Z) := \inf \bigg\{\!\sum_{i}|U_i|^s: \ Z\subset \bigcup_{i}U_i,~|U_i|\leq \delta \text{ for all } i\bigg\}, \end{align*} $$
where
![]() $|\cdot |$
denotes the diameter of a subset. The quantity
$|\cdot |$
denotes the diameter of a subset. The quantity
is called the s-dimensional Hausdorff measure of Z. It is easy to show that there is a jump-up value
which is called the Hausdorff dimension of Z.
Given a Borel probability measure
![]() $\mu $
on X, the Hausdorff dimension of the measure
$\mu $
on X, the Hausdorff dimension of the measure
![]() $\mu $
is defined as
$\mu $
is defined as
The lower and upper pointwise dimension of
![]() $\mu $
at point
$\mu $
at point
![]() $x\in X$
are defined respectively by
$x\in X$
are defined respectively by
where
![]() $B(x,r)$
denotes the ball of radius r centred at x. If
$B(x,r)$
denotes the ball of radius r centred at x. If
![]() $\underline {d}_\mu (x)=\overline {d}_\mu (x)$
, then we denote the common value by
$\underline {d}_\mu (x)=\overline {d}_\mu (x)$
, then we denote the common value by
![]() $d_\mu (x)$
. In particular, Barreira and Wolf [Reference Barreira and Wolf5] proved that
$d_\mu (x)$
. In particular, Barreira and Wolf [Reference Barreira and Wolf5] proved that
where the essential supremum is taken with respect to
![]() $\mu $
. The following well-known result gives the relation between the Hausdorff dimension and the lower pointwise dimension.
$\mu $
. The following well-known result gives the relation between the Hausdorff dimension and the lower pointwise dimension.
Proposition 2.1. The following properties hold:
-
(1) if
 $\underline {d}_\mu (x)\geq \alpha $
for
$\underline {d}_\mu (x)\geq \alpha $
for
 $\mu $
-a.e.
$\mu $
-a.e.
 $x\in X$
, then
$x\in X$
, then
 $\dim _H\mu \geq \alpha $
;
$\dim _H\mu \geq \alpha $
; -
(2) if
 $\underline {d}_\mu (x)\leq \alpha $
for every
$\underline {d}_\mu (x)\leq \alpha $
for every
 $x\in Z\subseteq X$
, then
$x\in Z\subseteq X$
, then
 $\dim _HZ\leq \alpha $
.
$\dim _HZ\leq \alpha $
.
Let
![]() $f:X\rightarrow X$
be a
$f:X\rightarrow X$
be a
![]() $C^{1+\alpha }$
diffeomorphism on an
$C^{1+\alpha }$
diffeomorphism on an
![]() $m_{0}$
-dimensional compact Riemannian manifold X, and let
$m_{0}$
-dimensional compact Riemannian manifold X, and let
![]() $\mu $
be a hyperbolic ergodic measure on X. Let
$\mu $
be a hyperbolic ergodic measure on X. Let
![]() $\Gamma $
be the set of points which are regular in the sense of Oseledets [Reference Oseledets26]. A measurable partition
$\Gamma $
be the set of points which are regular in the sense of Oseledets [Reference Oseledets26]. A measurable partition
![]() $\xi ^u$
/
$\xi ^u$
/
![]() $\xi ^s$
of X is said to be subordinate to the unstable/stable manifold if for
$\xi ^s$
of X is said to be subordinate to the unstable/stable manifold if for
![]() $\mu $
-almost every x,
$\mu $
-almost every x,
![]() $\xi ^u(x)\subset W^u(x,f)$
/
$\xi ^u(x)\subset W^u(x,f)$
/
![]() $\xi ^s(x)\subset W^s(x, f)$
and contains an open neighbourhood of x in
$\xi ^s(x)\subset W^s(x, f)$
and contains an open neighbourhood of x in
![]() $W^u(x,f)$
/
$W^u(x,f)$
/
![]() $W^s(x, f)$
. Let
$W^s(x, f)$
. Let
![]() $\{\mu _x^u\}$
and
$\{\mu _x^u\}$
and
![]() $\{\mu _x^s\}$
be the collections of conditional measures associated with
$\{\mu _x^s\}$
be the collections of conditional measures associated with
![]() $\xi ^u$
and
$\xi ^u$
and
![]() $\xi ^s$
, respectively. For every
$\xi ^s$
, respectively. For every
![]() $x\in \Gamma $
, Ledrappier and Young [Reference Ledrappier and Young22] proved the existence of the following limits:
$x\in \Gamma $
, Ledrappier and Young [Reference Ledrappier and Young22] proved the existence of the following limits:
which are called the stable and unstable dimension of the measure
![]() $\mu $
, respectively. Here
$\mu $
, respectively. Here
![]() $B^\sigma (x,r):=\{y\in W^\sigma (x,f): d^\sigma (x,y)<r\}$
with
$B^\sigma (x,r):=\{y\in W^\sigma (x,f): d^\sigma (x,y)<r\}$
with
![]() $\sigma \in \{u,s\}$
. Since we consider the limit
$\sigma \in \{u,s\}$
. Since we consider the limit
![]() $r\to 0$
in equation (6), the definition of
$r\to 0$
in equation (6), the definition of
![]() $d_\mu ^u(x)$
will remain unchanged if we consider the global metric d in the dynamical ball
$d_\mu ^u(x)$
will remain unchanged if we consider the global metric d in the dynamical ball
![]() $B^\sigma (x,r)$
instead.
$B^\sigma (x,r)$
instead.
2.3 Pressure
Let
![]() $(M,f)$
be a topological dynamical system (TDS for short), that is,
$(M,f)$
be a topological dynamical system (TDS for short), that is,
![]() $f:M\rightarrow M$
is a continuous map on a compact metric space M equipped with the metric d. Denote by
$f:M\rightarrow M$
is a continuous map on a compact metric space M equipped with the metric d. Denote by
![]() $\mathcal {M}_{\mathrm {inv}}(f|_M)$
and
$\mathcal {M}_{\mathrm {inv}}(f|_M)$
and
![]() $\mathcal {M}_\mathrm{erg}(f|_M)$
the set of all f-invariant and ergodic Borel probability measures on M, respectively. Given
$\mathcal {M}_\mathrm{erg}(f|_M)$
the set of all f-invariant and ergodic Borel probability measures on M, respectively. Given
![]() $n\in \mathbb {N}$
and
$n\in \mathbb {N}$
and
![]() $x,y\in M$
, let
$x,y\in M$
, let
Given
![]() $\varepsilon>0$
, denote by
$\varepsilon>0$
, denote by
![]() $B_{n}(x,\varepsilon )=\{y:d_{n}(x,y)<\varepsilon \}$
the Bowen’s ball of radius
$B_{n}(x,\varepsilon )=\{y:d_{n}(x,y)<\varepsilon \}$
the Bowen’s ball of radius
![]() $\varepsilon $
centred at x of length n. A subset
$\varepsilon $
centred at x of length n. A subset
![]() $E\subset M$
is called
$E\subset M$
is called
![]() $(n,\varepsilon )$
-separated if
$(n,\varepsilon )$
-separated if
![]() $d_n(x,y)>\varepsilon $
for any two distinct points
$d_n(x,y)>\varepsilon $
for any two distinct points
![]() $x,y\in E$
. A sequence of continuous functions
$x,y\in E$
. A sequence of continuous functions
![]() $\Psi =\{\psi _n\}_{n\geq 1}$
on M is called sub-additive if
$\Psi =\{\psi _n\}_{n\geq 1}$
on M is called sub-additive if
Similarly, one calls a sequence of continuous functions
![]() $\Phi =\{\phi _n\}_{n\geq 1}$
on M super-additive if
$\Phi =\{\phi _n\}_{n\geq 1}$
on M super-additive if
![]() $-\Phi =\{-\phi _n\}_{n\geq 1}$
is sub-additive.
$-\Phi =\{-\phi _n\}_{n\geq 1}$
is sub-additive.
Let
![]() $\Psi =\{\psi _n\}_{n\geq 1}$
be a sub-additive sequence of continuous potentials on M, set
$\Psi =\{\psi _n\}_{n\geq 1}$
be a sub-additive sequence of continuous potentials on M, set
 $$ \begin{align*} P_n(f,\Psi, \varepsilon)=\sup\bigg\{\!\sum_{x\in E}e^{\psi_n(x)}: E \text{ is an } (n,\varepsilon) \text{-separated subset of } M\bigg\}. \end{align*} $$
$$ \begin{align*} P_n(f,\Psi, \varepsilon)=\sup\bigg\{\!\sum_{x\in E}e^{\psi_n(x)}: E \text{ is an } (n,\varepsilon) \text{-separated subset of } M\bigg\}. \end{align*} $$
The quantity
is called the sub-additive topological pressure of
![]() $\Psi $
.
$\Psi $
.
The sub-additive topological pressure satisfies the following variational principle, see [Reference Cao, Feng and Huang6] for more details.
Theorem 2.1. Let
![]() $\Psi =\{\psi _n\}_{n\geq 1}$
be a sub-additive sequence of continuous potentials on M. Then
$\Psi =\{\psi _n\}_{n\geq 1}$
be a sub-additive sequence of continuous potentials on M. Then
where
![]() $h_\mu (f)$
is the measure theoretic entropy of f with respect to the measure
$h_\mu (f)$
is the measure theoretic entropy of f with respect to the measure
![]() $\mu $
and
$\mu $
and
![]() ${\mathcal {F}_*(\Psi ,\mu )=\lim _{n\to \infty }(1/n)\int \psi _n\,d\mu }.$
${\mathcal {F}_*(\Psi ,\mu )=\lim _{n\to \infty }(1/n)\int \psi _n\,d\mu }.$
Remark 2.1. If
![]() $\Psi =\{\psi _n\}_{n\geq 1}$
is additive in the sense that
$\Psi =\{\psi _n\}_{n\geq 1}$
is additive in the sense that
![]() $\psi _n(x)=\psi (x)+\psi (fx)+\cdots +\psi (f^{n-1}x):= S_n\psi (x)$
for some continuous function
$\psi _n(x)=\psi (x)+\psi (fx)+\cdots +\psi (f^{n-1}x):= S_n\psi (x)$
for some continuous function
![]() $\psi : M\to \mathbb {R}$
, we simply denote the topological pressure
$\psi : M\to \mathbb {R}$
, we simply denote the topological pressure
![]() $P(f, \Psi )$
as
$P(f, \Psi )$
as
![]() $P(f, \psi )$
.
$P(f, \psi )$
.
Next we recall the super-additive topological pressure introduced in [Reference Cao, Pesin and Zhao8] by the variational relation for topological pressure, although it is unknown whether the variational principle holds for super-additive topological pressure defined via separated sets. Given a sequence of super-additive continuous potentials
![]() $\Phi =\{\phi _{n}\}_{n\geq 1}$
on M, the super-additive topological pressure of
$\Phi =\{\phi _{n}\}_{n\geq 1}$
on M, the super-additive topological pressure of
![]() $\Phi $
is defined as
$\Phi $
is defined as
where
The second equality is due to the standard sub-additive argument. The following result gives the relation between the sub-additive (super-additive) topological pressure and the topological pressure for additive potentials.
Proposition 2.2. Let
![]() $\Phi =\{\phi _{n}\}_{n\geq 1}$
be a sequence of continuous potentials on M. Then the following properties hold:
$\Phi =\{\phi _{n}\}_{n\geq 1}$
be a sequence of continuous potentials on M. Then the following properties hold:
-
(1) if
 $\Phi $
is sub-additive and the entropy map
$\Phi $
is sub-additive and the entropy map
 $\mu \mapsto h_\mu (f)$
is upper semi-continuous, then
$\mu \mapsto h_\mu (f)$
is upper semi-continuous, then $$ \begin{align*}{P(f,\Phi )=\lim _{n\rightarrow \infty } P(f, {\phi _{n}}/{n})=\lim _{n\rightarrow \infty } ({1}/{n}) P(f^{n},\phi _{n})};\end{align*} $$
$$ \begin{align*}{P(f,\Phi )=\lim _{n\rightarrow \infty } P(f, {\phi _{n}}/{n})=\lim _{n\rightarrow \infty } ({1}/{n}) P(f^{n},\phi _{n})};\end{align*} $$
-
(2) if
 $\Phi $
is super-additive, then
$\Phi $
is super-additive, then $$ \begin{align*}P(f,\Phi )=\lim _{n\rightarrow \infty } P(f, {\phi _{n}}/{n})=\lim _{n\rightarrow \infty } ({1}/{n}) P(f^{n},\phi _{n}).\end{align*} $$
$$ \begin{align*}P(f,\Phi )=\lim _{n\rightarrow \infty } P(f, {\phi _{n}}/{n})=\lim _{n\rightarrow \infty } ({1}/{n}) P(f^{n},\phi _{n}).\end{align*} $$
The first statement is proved in [Reference Ban, Cao and Hu3], where the sub-additive topological pressure is defined via separated sets, so one requires that the entropy map be upper semi-continuous. The second statement is proved in [Reference Cao, Pesin and Zhao8], and one does not need any additional condition since the super-additive topological pressure is defined via the variational relations.
Following the approach described in [Reference Pesin29], we recall the topological pressure on an arbitrary subset of unstable manifolds. Let
![]() $f: M\to M$
be a
$f: M\to M$
be a
![]() $C^{1+\alpha }$
diffeomorphism on an
$C^{1+\alpha }$
diffeomorphism on an
![]() $m_0$
-dimensional smooth compact Riemannian manifold M and let
$m_0$
-dimensional smooth compact Riemannian manifold M and let
![]() $\Lambda \subset M$
be a hyperbolic set. Let
$\Lambda \subset M$
be a hyperbolic set. Let
![]() $\Psi =\{\psi _n\}_{n\geq 1}$
be a sub-additive sequence of continuous functions on
$\Psi =\{\psi _n\}_{n\geq 1}$
be a sub-additive sequence of continuous functions on
![]() $\Lambda $
. For every
$\Lambda $
. For every
![]() $x\in \Lambda $
, denote
$x\in \Lambda $
, denote
![]() $Z=\Lambda \cap W^u_{\mathrm {loc}}(x,f)$
. Given
$Z=\Lambda \cap W^u_{\mathrm {loc}}(x,f)$
. Given
![]() $s\in \mathbb {R}$
, set
$s\in \mathbb {R}$
, set
 $$ \begin{align} m(Z,\Psi,s,\delta):=\lim_{N\to\infty} \inf \bigg\{\!\sum_i\exp\Big(-sn_i + \sup_{y\in B_{n_i}^u(x_i,\delta)}\psi_{n_i}(y)\Big)\bigg\}, \end{align} $$
$$ \begin{align} m(Z,\Psi,s,\delta):=\lim_{N\to\infty} \inf \bigg\{\!\sum_i\exp\Big(-sn_i + \sup_{y\in B_{n_i}^u(x_i,\delta)}\psi_{n_i}(y)\Big)\bigg\}, \end{align} $$
where the infimum is taken over all collections
![]() $\{B^u_{n_i}(x_i,\delta )\}$
with
$\{B^u_{n_i}(x_i,\delta )\}$
with
![]() $x_i\in \Lambda $
,
$x_i\in \Lambda $
,
![]() $n_i\geq N$
that cover Z, and
$n_i\geq N$
that cover Z, and
It is easy to show that there is a jump-up value
The quantity
is called the topological pressure of
![]() $\Psi $
on the subset Z. It is not difficult to show that
$\Psi $
on the subset Z. It is not difficult to show that
![]() $P_\Lambda (f, \Psi )=P(f|_\Lambda , \Psi )$
(see [Reference Cao, Feng and Huang6, Proposition 4.4]).
$P_\Lambda (f, \Psi )=P(f|_\Lambda , \Psi )$
(see [Reference Cao, Feng and Huang6, Proposition 4.4]).
Let
![]() $\mu $
be an f-invariant Borel probability measure on M. Given a sub-additive potential
$\mu $
be an f-invariant Borel probability measure on M. Given a sub-additive potential
![]() $\Phi =\{\phi _{n}\}_{n \geq 1}$
on M, for
$\Phi =\{\phi _{n}\}_{n \geq 1}$
on M, for
![]() $0<\delta < 1, n \geq 1$
and
$0<\delta < 1, n \geq 1$
and
![]() $\varepsilon>0$
, a subset
$\varepsilon>0$
, a subset
![]() $F\subset M$
is called an
$F\subset M$
is called an
![]() $(n,\varepsilon ,\delta )$
-spanning set if the union
$(n,\varepsilon ,\delta )$
-spanning set if the union
![]() $\bigcup _{x\in F}B_n(x,\varepsilon )$
has
$\bigcup _{x\in F}B_n(x,\varepsilon )$
has
![]() $\mu $
-measure more than or equal to
$\mu $
-measure more than or equal to
![]() $1-\delta $
. Put
$1-\delta $
. Put
 $$ \begin{align*} P_{\mu}(f, \Phi, n, \varepsilon, \delta):=\inf \bigg\{\!\sum_{x \in F} \exp \Big(\sup _{y \in B_{n}(x, \varepsilon)} \phi_{n}(y)\Big) : F \text{ is an } (n, \varepsilon, \delta)\text{-spanning set}\bigg\} \end{align*} $$
$$ \begin{align*} P_{\mu}(f, \Phi, n, \varepsilon, \delta):=\inf \bigg\{\!\sum_{x \in F} \exp \Big(\sup _{y \in B_{n}(x, \varepsilon)} \phi_{n}(y)\Big) : F \text{ is an } (n, \varepsilon, \delta)\text{-spanning set}\bigg\} \end{align*} $$
and let further that
 $$ \begin{align*} P_{\mu}(f, \Phi, \varepsilon, \delta)&:=\limsup _{n \rightarrow \infty} \frac{1}{n} \log P_{\mu}(f, \Phi, n, \varepsilon, \delta), \\ P_{\mu}(f, \Phi, \delta)&:=\liminf_{\varepsilon \rightarrow 0} P_{\mu}(f, \Phi, \varepsilon, \delta), \\ P_{\mu}(f, \Phi)&:=\lim_{\delta \rightarrow 0} P_{\mu}(f, \Phi, \delta), \end{align*} $$
$$ \begin{align*} P_{\mu}(f, \Phi, \varepsilon, \delta)&:=\limsup _{n \rightarrow \infty} \frac{1}{n} \log P_{\mu}(f, \Phi, n, \varepsilon, \delta), \\ P_{\mu}(f, \Phi, \delta)&:=\liminf_{\varepsilon \rightarrow 0} P_{\mu}(f, \Phi, \varepsilon, \delta), \\ P_{\mu}(f, \Phi)&:=\lim_{\delta \rightarrow 0} P_{\mu}(f, \Phi, \delta), \end{align*} $$
and we call
![]() $P_{\mu }(f, \Phi )$
the sub-additive measure-theoretic pressure of
$P_{\mu }(f, \Phi )$
the sub-additive measure-theoretic pressure of
![]() $(f,\Phi )$
with respect to
$(f,\Phi )$
with respect to
![]() $\mu $
. If one considers a super-additive potential
$\mu $
. If one considers a super-additive potential
![]() $\Phi =\{\phi _n\}_{n\ge 1}$
on M, replacing
$\Phi =\{\phi _n\}_{n\ge 1}$
on M, replacing
![]() $\sup _{y \in B_{n}(x, \varepsilon )} \phi _{n}(y)$
by
$\sup _{y \in B_{n}(x, \varepsilon )} \phi _{n}(y)$
by
![]() $\phi _{n}(x)$
in
$\phi _{n}(x)$
in
![]() $P_{\mu }(f, \Phi , n, \varepsilon , \delta )$
, then the corresponding quantity
$P_{\mu }(f, \Phi , n, \varepsilon , \delta )$
, then the corresponding quantity
![]() $P_{\mu }(f, \Phi )$
is called the super-additive measure theoretic pressure of
$P_{\mu }(f, \Phi )$
is called the super-additive measure theoretic pressure of
![]() $(f,\Phi )$
with respect to
$(f,\Phi )$
with respect to
![]() $\mu $
.
$\mu $
.
Remark 2.2.
-
(i) It is easy to see that
 $P_{\mu }(f, \Phi , \delta )$
increases with
$P_{\mu }(f, \Phi , \delta )$
increases with
 $\delta $
decreasing to zero. So the limit in the last formula exists. Moreover, it is proved in [Reference Cao, Hu and Zhao7] that
$\delta $
decreasing to zero. So the limit in the last formula exists. Moreover, it is proved in [Reference Cao, Hu and Zhao7] that
 $P_{\mu }(f, \Phi , \delta )$
is independent of
$P_{\mu }(f, \Phi , \delta )$
is independent of
 $\delta $
. Hence, the limit of
$\delta $
. Hence, the limit of
 $\delta \rightarrow 0$
is redundant in the definition.
$\delta \rightarrow 0$
is redundant in the definition. -
(ii) If
 $\Phi =\{\phi _n\}_{n\ge 1}$
is an additive potential on M, that is,
$\Phi =\{\phi _n\}_{n\ge 1}$
is an additive potential on M, that is,
 $\phi _n(x)=\sum _{i=0}^{n-1}\phi _1(f^ix)$
for some continuous function
$\phi _n(x)=\sum _{i=0}^{n-1}\phi _1(f^ix)$
for some continuous function
 $\phi _1$
, then we simply write
$\phi _1$
, then we simply write
 $P_\mu (f,\Phi )$
as
$P_\mu (f,\Phi )$
as
 $P_\mu (f,\phi _1)$
.
$P_\mu (f,\phi _1)$
.
In the following, we recall some properties of sub-additive/super-additive measure- theoretic pressure which are proved in [Reference Cao, Hu and Zhao7].
Theorem 2.2. [Reference Cao, Hu and Zhao7, Theorem A]
Let
![]() $(M,f)$
be a TDS and
$(M,f)$
be a TDS and
![]() $\Phi =\{\phi _n\}_{n\geq 1}$
a sub-additive potential on M. For every
$\Phi =\{\phi _n\}_{n\geq 1}$
a sub-additive potential on M. For every
![]() $\mu \in \mathcal {M}_\mathrm{erg}(f|_M)$
with
$\mu \in \mathcal {M}_\mathrm{erg}(f|_M)$
with
![]() $\mathcal {F}_*(\Phi , \mu )\neq -\infty $
, we have that
$\mathcal {F}_*(\Phi , \mu )\neq -\infty $
, we have that
Theorem 2.3. [Reference Cao, Hu and Zhao7, Proposition 3.2]
Let
![]() $(M,f)$
be a TDS and
$(M,f)$
be a TDS and
![]() $\Phi =\{\phi _n\}_{n\geq 1}$
a super-additive potential on M. For every
$\Phi =\{\phi _n\}_{n\geq 1}$
a super-additive potential on M. For every
![]() $\mu \in \mathcal {M}_\mathrm{erg}(f|_M)$
, we have that
$\mu \in \mathcal {M}_\mathrm{erg}(f|_M)$
, we have that
Remark 2.3. In Theorem 2.2, to avoid the indeterminate form
![]() $\infty -\infty $
, the condition
$\infty -\infty $
, the condition
![]() $\mathcal {F}_*(\Phi , \mu )\neq -\infty $
is necessary. However, we do not need this condition in Theorem 2.3. If
$\mathcal {F}_*(\Phi , \mu )\neq -\infty $
is necessary. However, we do not need this condition in Theorem 2.3. If
![]() $\Phi =\{\phi _n\}_{n\geq 1}$
is an additive potential on M, that is,
$\Phi =\{\phi _n\}_{n\geq 1}$
is an additive potential on M, that is,
![]() $\phi _n(x)=S_n\phi (x)$
for some continuous function
$\phi _n(x)=S_n\phi (x)$
for some continuous function
![]() $\phi $
, then we have
$\phi $
, then we have
The above formula is also proven in [Reference He, Lv and Zhou16].
2.4 Singular dimension
Let
![]() $f: M\to M$
be a
$f: M\to M$
be a
![]() $C^{1+\alpha }$
diffeomorphism on an
$C^{1+\alpha }$
diffeomorphism on an
![]() $m_0$
-dimensional compact smooth Riemannian manifold M and
$m_0$
-dimensional compact smooth Riemannian manifold M and
![]() $\Lambda \subset M$
a hyperbolic set. Consider the sub-additive singular valued potential
$\Lambda \subset M$
a hyperbolic set. Consider the sub-additive singular valued potential
![]() $\Psi _f(t)=\{-\psi ^t(\cdot , f^n)\}_{n\geq 1}$
given by equation (4). Fix
$\Psi _f(t)=\{-\psi ^t(\cdot , f^n)\}_{n\geq 1}$
given by equation (4). Fix
![]() $x\in \Lambda $
and let
$x\in \Lambda $
and let
![]() $Z=\Lambda \cap W_{\mathrm {loc}}^u(x,f)$
. Following the approach described in [Reference Cao, Pesin and Zhao8], we introduce the Carathéodory singular dimension of Z. Put
$Z=\Lambda \cap W_{\mathrm {loc}}^u(x,f)$
. Following the approach described in [Reference Cao, Pesin and Zhao8], we introduce the Carathéodory singular dimension of Z. Put
 $$ \begin{align} m(Z, \Psi_f(t),\delta):=\lim_{N\to\infty}\inf\bigg\{\!\sum_i\exp \Big[\sup_{y\in B_{n_i}^u(x_i,\delta)} -\psi^t(y, f^{n_i})\Big]\bigg\}, \end{align} $$
$$ \begin{align} m(Z, \Psi_f(t),\delta):=\lim_{N\to\infty}\inf\bigg\{\!\sum_i\exp \Big[\sup_{y\in B_{n_i}^u(x_i,\delta)} -\psi^t(y, f^{n_i})\Big]\bigg\}, \end{align} $$
where the infimum is taken over all collections
![]() $\{B_{n_i}^u(x_i,\delta )\}$
with
$\{B_{n_i}^u(x_i,\delta )\}$
with
![]() $x_i\in \Lambda $
,
$x_i\in \Lambda $
,
![]() $n_i\geq N$
that cover Z. It is easy to see that there is a jump-up value
$n_i\geq N$
that cover Z. It is easy to see that there is a jump-up value
 $$ \begin{align} \nonumber \dim_{C,\delta}^{\Psi_f} Z := &\inf\{t: m(Z, \Psi_f(t), \delta)=0 \} \\ = &\sup\{t: m(Z, \Psi_f(t), \delta)=+\infty \}. \end{align} $$
$$ \begin{align} \nonumber \dim_{C,\delta}^{\Psi_f} Z := &\inf\{t: m(Z, \Psi_f(t), \delta)=0 \} \\ = &\sup\{t: m(Z, \Psi_f(t), \delta)=+\infty \}. \end{align} $$
The quantity
is called the Carathéodory singular dimension of Z with respect to the sub-additive singular valued potential
![]() $\Psi _f$
.
$\Psi _f$
.
Consider the super-additive singular valued potential
![]() $\Phi _f(t)=\{-\phi ^t(\cdot , f^n)\}_{n\geq 1}$
given by equation (3), replacing
$\Phi _f(t)=\{-\phi ^t(\cdot , f^n)\}_{n\geq 1}$
given by equation (3), replacing
![]() $ {\sup _{y\in B_{n_i}^u(x_i,\delta )} -\psi ^t(y, f^{n_i})}$
by
$ {\sup _{y\in B_{n_i}^u(x_i,\delta )} -\psi ^t(y, f^{n_i})}$
by
![]() $-\phi ^t(x_i, f^{n_i})$
in equation (8), one can define
$-\phi ^t(x_i, f^{n_i})$
in equation (8), one can define
![]() $m(Z, \Phi _f(t),\delta )$
and
$m(Z, \Phi _f(t),\delta )$
and
![]() $\dim _{C,\delta }^{\Phi _f} Z$
in a similar fashion as equations (8) and (9). The corresponding quantity
$\dim _{C,\delta }^{\Phi _f} Z$
in a similar fashion as equations (8) and (9). The corresponding quantity
![]() $\dim _C^{\Phi _f} Z$
as in equation (10) is called the Carathéodory singular dimension of Z with respect to the super-additive singular valued potential
$\dim _C^{\Phi _f} Z$
as in equation (10) is called the Carathéodory singular dimension of Z with respect to the super-additive singular valued potential
![]() $\Phi _f$
.
$\Phi _f$
.
2.5 Approximation of hyperbolic measures by hyperbolic sets with dominated splitting
First we recall the definition of the dominated splitting. Let
![]() $f:M\rightarrow M$
be a
$f:M\rightarrow M$
be a
![]() $C^{1+\alpha }$
diffeomorphism on an
$C^{1+\alpha }$
diffeomorphism on an
![]() $m_{0}$
-dimensional compact smooth Riemannian manifold M. Suppose
$m_{0}$
-dimensional compact smooth Riemannian manifold M. Suppose
![]() $\Lambda \subset M$
is a compact f-invariant set. We say
$\Lambda \subset M$
is a compact f-invariant set. We say
![]() $\Lambda $
admits a dominated splitting if there is a continuous invariant splitting
$\Lambda $
admits a dominated splitting if there is a continuous invariant splitting
![]() $T_{\Lambda }M=E\oplus F$
and constants
$T_{\Lambda }M=E\oplus F$
and constants
![]() $C>0,\unicode{x3bb} \in (0,1)$
such that for each
$C>0,\unicode{x3bb} \in (0,1)$
such that for each
![]() $x\in \Lambda $
,
$x\in \Lambda $
,
![]() $n\in \mathbb {N}$
,
$n\in \mathbb {N}$
,
![]() $0\neq u\in E(x)$
and
$0\neq u\in E(x)$
and
![]() $0\neq v\in F(x)$
, it holds that
$0\neq v\in F(x)$
, it holds that
We say F dominates E and write it as
![]() $E\preceq F$
. Furthermore, given
$E\preceq F$
. Furthermore, given
![]() $0<\ell \leq m_{0}$
, we say a continuous invariant splitting
$0<\ell \leq m_{0}$
, we say a continuous invariant splitting
![]() $T_{\Lambda }M=E_{1}\oplus \cdots \oplus E_{\ell }$
dominates if there are numbers
$T_{\Lambda }M=E_{1}\oplus \cdots \oplus E_{\ell }$
dominates if there are numbers
![]() $\chi _{1}<\chi _{2}<\cdots <\chi _{\ell }$
, constants
$\chi _{1}<\chi _{2}<\cdots <\chi _{\ell }$
, constants
![]() $C>0$
and
$C>0$
and
![]() $0<\varepsilon <\min _{1\leq i\leq \ell -1}\{({\chi _{i+1}-\chi _{i}})/{100}\}$
such that for every
$0<\varepsilon <\min _{1\leq i\leq \ell -1}\{({\chi _{i+1}-\chi _{i}})/{100}\}$
such that for every
![]() $x\in \Lambda $
,
$x\in \Lambda $
,
![]() $n\in \mathbb {N}$
and
$n\in \mathbb {N}$
and
![]() $1\leq j\leq \ell $
and each unit vector
$1\leq j\leq \ell $
and each unit vector
![]() $u\in E_{j}(x)$
, it holds that
$u\in E_{j}(x)$
, it holds that
In particular, it is clear that
![]() $E_{1}\preceq \cdots \preceq E_{\ell }$
. We shall use the notion
$E_{1}\preceq \cdots \preceq E_{\ell }$
. We shall use the notion
![]() $\{\chi _{j}\}$
-dominated when we want to stress the dependence on the numbers
$\{\chi _{j}\}$
-dominated when we want to stress the dependence on the numbers
![]() $\{\chi _{j}\}$
.
$\{\chi _{j}\}$
.
Refining Katok’s approximation theory in non-uniformly hyperbolic dynamical systems [Reference Katok18], Avila, Crovisier and Wilkinson [Reference Avila, Crovisier and Wilkinson2] obtained the following approximation result.
Theorem 2.4. [Reference Avila, Crovisier and Wilkinson2]
Let f be a
![]() $C^{1+\alpha }$
diffeomorphism on an
$C^{1+\alpha }$
diffeomorphism on an
![]() $m_0$
-dimensional compact smooth Riemannian manifold M, and let
$m_0$
-dimensional compact smooth Riemannian manifold M, and let
![]() $\mu $
be an ergodic hyperbolic measure with
$\mu $
be an ergodic hyperbolic measure with
![]() $h_{\mu }(f)>0$
. Then for every
$h_{\mu }(f)>0$
. Then for every
![]() $\varepsilon>0$
and weak
$\varepsilon>0$
and weak
![]() $^{\ast }$
neighbourhood
$^{\ast }$
neighbourhood
![]() $\mathcal {V}$
of
$\mathcal {V}$
of
![]() $\mu $
in the space of f-invariant probability measures on M, there exists an f-invariant compact subset
$\mu $
in the space of f-invariant probability measures on M, there exists an f-invariant compact subset
![]() $\Lambda _{\varepsilon }\subset M$
such that:
$\Lambda _{\varepsilon }\subset M$
such that:
-
(a)
 $\Lambda _{\varepsilon }$
is
$\Lambda _{\varepsilon }$
is
 $\varepsilon $
-close to the support set of
$\varepsilon $
-close to the support set of
 $\mu $
in the Hausdorff distance;
$\mu $
in the Hausdorff distance; -
(b)
 $|h_{\text {top}}(f|_{\Lambda _{\varepsilon }}) - h_{\mu }(f)| \leq \varepsilon $
;
$|h_{\text {top}}(f|_{\Lambda _{\varepsilon }}) - h_{\mu }(f)| \leq \varepsilon $
; -
(c) all the invariant probability measures supported on
 $\Lambda _{\varepsilon }$
lie in
$\Lambda _{\varepsilon }$
lie in
 $\mathcal {V}$
;
$\mathcal {V}$
; -
(d) there is a
 $\{\chi _{j}(\mu )\}$
-dominated splitting
$\{\chi _{j}(\mu )\}$
-dominated splitting
 $TM=E_{1}\oplus E_{2}\oplus \cdots \oplus E_{\ell }$
over
$TM=E_{1}\oplus E_{2}\oplus \cdots \oplus E_{\ell }$
over
 $\Lambda _{\varepsilon }$
, where
$\Lambda _{\varepsilon }$
, where
 $\chi _{1}(\mu )<\cdots <\chi _{\ell }(\mu )$
are distinct Lyapunov exponents of f with respect to the measure
$\chi _{1}(\mu )<\cdots <\chi _{\ell }(\mu )$
are distinct Lyapunov exponents of f with respect to the measure
 $\mu $
.
$\mu $
.
In the second statement, the original result does not show that
![]() $h_{\text {top}}(f|_{\Lambda _{\varepsilon }})\le h_{\mu }(f)+\varepsilon $
. However, only a slight modification can give the upper bound of the topological entropy of f on the horseshoe.
$h_{\text {top}}(f|_{\Lambda _{\varepsilon }})\le h_{\mu }(f)+\varepsilon $
. However, only a slight modification can give the upper bound of the topological entropy of f on the horseshoe.
3 Proofs
This section provides the detailed proofs of the main results presented in the previous section.
3.1 Proof of Theorem A
(i) Since
![]() $\mu $
is a hyperbolic ergodic SRB measure for a
$\mu $
is a hyperbolic ergodic SRB measure for a
![]() $C^{1+\alpha }$
diffeomorphism f and has a one-dimensional stable manifold, by [Reference Wang, Qu and Cao34, Lemma 15 and 25], one has
$C^{1+\alpha }$
diffeomorphism f and has a one-dimensional stable manifold, by [Reference Wang, Qu and Cao34, Lemma 15 and 25], one has

for
![]() $\mu $
-a.e. x. Barreira, Pesin and Schmeling [Reference Barreira, Pesin and Schmeling4] proved that
$\mu $
-a.e. x. Barreira, Pesin and Schmeling [Reference Barreira, Pesin and Schmeling4] proved that
![]() $d_\mu (x)=d_\mu ^u(x)+d_\mu ^s(x)$
for
$d_\mu (x)=d_\mu ^u(x)+d_\mu ^s(x)$
for
![]() $\mu $
-a.e. x. As a consequence, one has that
$\mu $
-a.e. x. As a consequence, one has that

for
![]() $\mu $
-a.e. x. Hence, one has
$\mu $
-a.e. x. Hence, one has

If
![]() $\unicode{x3bb} _1(\mu )+\unicode{x3bb} _2(\mu )+\cdots +\unicode{x3bb} _{m_0}(\mu )<0$
, then one can show that
$\unicode{x3bb} _1(\mu )+\unicode{x3bb} _2(\mu )+\cdots +\unicode{x3bb} _{m_0}(\mu )<0$
, then one can show that

since
![]() $\mu $
is an SRB measure and has a one-dimensional stable manifold. Therefore, we have that
$\mu $
is an SRB measure and has a one-dimensional stable manifold. Therefore, we have that
If
![]() $\unicode{x3bb} _1(\mu )+\unicode{x3bb} _2(\mu )+\cdots +\unicode{x3bb} _{m_0}(\mu )\geq 0$
, it follows from the definition of Lyapunov dimension that
$\unicode{x3bb} _1(\mu )+\unicode{x3bb} _2(\mu )+\cdots +\unicode{x3bb} _{m_0}(\mu )\geq 0$
, it follows from the definition of Lyapunov dimension that
![]() $\dim _L\mu =m_0$
. Since
$\dim _L\mu =m_0$
. Since
![]() $\mu $
is an SRB measure for f and has a one-dimensional stable manifold, one has that
$\mu $
is an SRB measure for f and has a one-dimensional stable manifold, one has that
This together with the fact that

implies that
![]() $({h_\mu (f)}/{-\unicode{x3bb} _{m_0}(\mu )})=1$
. This yields that
$({h_\mu (f)}/{-\unicode{x3bb} _{m_0}(\mu )})=1$
. This yields that
![]() $\dim _H\mu =\dim _L\mu $
.
$\dim _H\mu =\dim _L\mu $
.
By [Reference Wang, Qu and Cao34, Theorem B], there exists a sequence of hyperbolic sets
![]() $\Lambda _{n}$
such that
$\Lambda _{n}$
such that
(ii) Since
![]() $\mu $
is a hyperbolic ergodic SRB measure for a
$\mu $
is a hyperbolic ergodic SRB measure for a
![]() $C^{1+\alpha }$
diffeomorphism f, by [Reference Wang, Qu and Cao34, Lemma 15], one has that
$C^{1+\alpha }$
diffeomorphism f, by [Reference Wang, Qu and Cao34, Lemma 15], one has that
Considering
![]() $f^{-1}$
instead of f, since
$f^{-1}$
instead of f, since
![]() $h_\mu (f)=-\unicode{x3bb} _{u+1}(\mu )-\unicode{x3bb} _{u+2}(\mu )-\cdots -\unicode{x3bb} _{m_0}(\mu )$
, by [Reference Ledrappier and Young21, Theorem A], we have that the measure
$h_\mu (f)=-\unicode{x3bb} _{u+1}(\mu )-\unicode{x3bb} _{u+2}(\mu )-\cdots -\unicode{x3bb} _{m_0}(\mu )$
, by [Reference Ledrappier and Young21, Theorem A], we have that the measure
![]() $\mu $
has absolutely continuous conditional measures on stable manifolds of f. Using the same arguments as the proof of [Reference Wang, Qu and Cao34, Lemma 15], we have that
$\mu $
has absolutely continuous conditional measures on stable manifolds of f. Using the same arguments as the proof of [Reference Wang, Qu and Cao34, Lemma 15], we have that
Hence,
![]() $d_\mu (x)=u+s=m_0$
for
$d_\mu (x)=u+s=m_0$
for
![]() $\mu $
-a.e. x, which implies that
$\mu $
-a.e. x, which implies that
![]() $\dim _H\mu =m_0$
. Since
$\dim _H\mu =m_0$
. Since
![]() $\mu $
is an SRB measure for f and
$\mu $
is an SRB measure for f and
![]() $h_\mu (f)=-\unicode{x3bb} _{u+1}(\mu )-\unicode{x3bb} _{u+2}(\mu )-\cdots -\unicode{x3bb} _{m_0}(\mu )$
, one can conclude that
$h_\mu (f)=-\unicode{x3bb} _{u+1}(\mu )-\unicode{x3bb} _{u+2}(\mu )-\cdots -\unicode{x3bb} _{m_0}(\mu )$
, one can conclude that
then
![]() $\dim _L\mu =m_0$
by the definition of Lyapunov dimension. This proves that
$\dim _L\mu =m_0$
by the definition of Lyapunov dimension. This proves that
Finally, for each
![]() $\varepsilon>0$
, there exists a hyperbolic set
$\varepsilon>0$
, there exists a hyperbolic set
![]() $\Lambda _\varepsilon $
satisfying properties (a)–(d) in Theorem 2.4. Fix a positive integer
$\Lambda _\varepsilon $
satisfying properties (a)–(d) in Theorem 2.4. Fix a positive integer
![]() $n\ge 1$
. Let
$n\ge 1$
. Let
![]() $t_n$
be the unique root of Bowen’s equation
$t_n$
be the unique root of Bowen’s equation
![]() $P(f^{2^n}|\Lambda _\varepsilon ,-\psi ^t(\cdot , f^{2^n}))=0$
and let
$P(f^{2^n}|\Lambda _\varepsilon ,-\psi ^t(\cdot , f^{2^n}))=0$
and let
![]() $\mu _n^u$
be the unique equilibrium state for the topological pressure
$\mu _n^u$
be the unique equilibrium state for the topological pressure
![]() $P(f^{2^n}|\Lambda _\varepsilon ,-\psi ^{t_n}(\cdot , f^{2^n}))$
. Similarly, let
$P(f^{2^n}|\Lambda _\varepsilon ,-\psi ^{t_n}(\cdot , f^{2^n}))$
. Similarly, let
![]() $t_n^{\prime }$
be the root of Bowen’s equation
$t_n^{\prime }$
be the root of Bowen’s equation
![]() $P(f^{2^n}|\Lambda _\varepsilon , \phi ^t(\cdot , f^{2^n}))=0$
and let
$P(f^{2^n}|\Lambda _\varepsilon , \phi ^t(\cdot , f^{2^n}))=0$
and let
![]() $\mu _n^s$
be the unique equilibrium state for the topological pressure
$\mu _n^s$
be the unique equilibrium state for the topological pressure
![]() $P(f^{2^n}|\Lambda _\varepsilon , \phi ^{t_n}(\cdot , f^{2^n}))$
. As in the proof of [Reference Wang, Qu and Cao34, Theorem B], the following properties hold:
$P(f^{2^n}|\Lambda _\varepsilon , \phi ^{t_n}(\cdot , f^{2^n}))$
. As in the proof of [Reference Wang, Qu and Cao34, Theorem B], the following properties hold:
-
(e)
 ${\lim _{\varepsilon \to 0}\lim _{n\to \infty }t_n=u}$
and
${\lim _{\varepsilon \to 0}\lim _{n\to \infty }t_n=u}$
and
 ${\lim _{\varepsilon \to 0}\lim _{n\to \infty }t_n'=s}$
;
${\lim _{\varepsilon \to 0}\lim _{n\to \infty }t_n'=s}$
; -
(f) there is a Markov partition
 $\mathcal {P}=\{P_1,P_2,\ldots ,P_\ell \}$
of
$\mathcal {P}=\{P_1,P_2,\ldots ,P_\ell \}$
of
 $\Lambda _\varepsilon $
. For every
$\Lambda _\varepsilon $
. For every
 $i\in \{1,2,\ldots ,\ell \}$
, there is a family of conditional measures
$i\in \{1,2,\ldots ,\ell \}$
, there is a family of conditional measures
 $\{\mu _{n,x}^u\}_{x\in P_i}$
(
$\{\mu _{n,x}^u\}_{x\in P_i}$
(
 $\{\mu _{n,x}^s\}_{x\in P_i}$
) of
$\{\mu _{n,x}^s\}_{x\in P_i}$
) of
 $\mu _n^u$
(
$\mu _n^u$
(
 $\mu _n^s$
) on the local unstable (stable) sets
$\mu _n^s$
) on the local unstable (stable) sets
 $W^u_{P_i}$
(
$W^u_{P_i}$
(
 $W^s_{P_i}$
) such that for every
$W^s_{P_i}$
) such that for every
 $x\in P_i$
, there is small
$x\in P_i$
, there is small
 $r_0>0$
such that for every
$r_0>0$
such that for every
 $r\in (0, r_0)$
, and
$r\in (0, r_0)$
, and $$ \begin{align*}r^{u+\varepsilon} \leq \mu_{n,x}^u(B^u(x,r)) \leq r^{t_n-\varepsilon}\end{align*} $$
where
$$ \begin{align*}r^{u+\varepsilon} \leq \mu_{n,x}^u(B^u(x,r)) \leq r^{t_n-\varepsilon}\end{align*} $$
where $$ \begin{align*}r^{s+\varepsilon} \leq \mu_{n,x}^s(B^s(x,r)) \leq r^{t_n'-\varepsilon},\end{align*} $$
$$ \begin{align*}r^{s+\varepsilon} \leq \mu_{n,x}^s(B^s(x,r)) \leq r^{t_n'-\varepsilon},\end{align*} $$
 $W_{P_i}^u(x,f):=W_{loc}^u(x,f)\cap P_i$
and
$W_{P_i}^u(x,f):=W_{loc}^u(x,f)\cap P_i$
and
 $W_{P_i}^s(x,f):=W_{loc}^s(x,f)\cap P_i$
for every
$W_{P_i}^s(x,f):=W_{loc}^s(x,f)\cap P_i$
for every
 $x\in P_i$
.
$x\in P_i$
.
Define a measure
![]() $\hat {\mu }_n$
on
$\hat {\mu }_n$
on
![]() $P_i$
as follows:
$P_i$
as follows:
for every
![]() $x\in P_i$
and each sufficiently small
$x\in P_i$
and each sufficiently small
![]() $r>0$
. This yields that
$r>0$
. This yields that
for every
![]() $x\in P_i$
. By Proposition 2.1 and the fact that
$x\in P_i$
. By Proposition 2.1 and the fact that
![]() $\Lambda _\varepsilon =\bigcup _{i=1}^\ell P_i$
, we have that
$\Lambda _\varepsilon =\bigcup _{i=1}^\ell P_i$
, we have that
This completes the proof of Theorem A.
3.2 Proof of Theorem B
Let
![]() $\Gamma $
be the set of points which are regular in the sense of Oseledets [Reference Oseledets26] with respect to the measure
$\Gamma $
be the set of points which are regular in the sense of Oseledets [Reference Oseledets26] with respect to the measure
![]() $\mu $
. For every
$\mu $
. For every
![]() $x\in \Gamma $
, denote its Lyapunov exponents by
$x\in \Gamma $
, denote its Lyapunov exponents by
To prove Theorem B, we need a coarse upper bound for the unstable and stable pointwise dimension
![]() $d_\mu ^u(x)$
,
$d_\mu ^u(x)$
,
![]() $d_\mu ^s(x)$
of an ergodic f-invariant hyperbolic probability measure
$d_\mu ^s(x)$
of an ergodic f-invariant hyperbolic probability measure
![]() $\mu $
for almost every x. We now provide the following useful lemma, which estimates the Hausdorff measure of the image of a small ball along unstable/stable direction under f.
$\mu $
for almost every x. We now provide the following useful lemma, which estimates the Hausdorff measure of the image of a small ball along unstable/stable direction under f.
Lemma 3.1. Fix
![]() $t\in [0,u]$
, then for any
$t\in [0,u]$
, then for any
![]() $b_0>2\sqrt {u}$
and
$b_0>2\sqrt {u}$
and
![]() $C_0>2^tu^{{t}/{2}}$
, there is
$C_0>2^tu^{{t}/{2}}$
, there is
![]() $\rho _0>0$
such that for all
$\rho _0>0$
such that for all
![]() $x\in \Gamma $
, if
$x\in \Gamma $
, if
![]() $B^u(x,\rho )\subset B(x,\rho _0)\cap W^u(x,f)$
for some
$B^u(x,\rho )\subset B(x,\rho _0)\cap W^u(x,f)$
for some
![]() $0<\rho <\rho _0$
, then we have
$0<\rho <\rho _0$
, then we have
where
![]() $b=b_0\exp \{-\log \alpha _{u-[t]}(x,f)\} \text { and } C=C_0\exp \{-\psi ^t(x,f)\}$
.
$b=b_0\exp \{-\log \alpha _{u-[t]}(x,f)\} \text { and } C=C_0\exp \{-\psi ^t(x,f)\}$
.
Proof. For simplicity, we just prove the lemma on the assumption that M is the Euclid space
![]() $\mathbb {R}^{m_0}$
. For the general case, one can use local charts to prove it.
$\mathbb {R}^{m_0}$
. For the general case, one can use local charts to prove it.
Given a small positive number
![]() $\varepsilon $
with
$\varepsilon $
with
![]() ${e^\varepsilon }/({1-\varepsilon })<2$
, since
${e^\varepsilon }/({1-\varepsilon })<2$
, since
![]() $f: M\to M$
is a
$f: M\to M$
is a
![]() $C^{1+\alpha }$
diffeomorphism on M, there exists
$C^{1+\alpha }$
diffeomorphism on M, there exists
![]() $\rho _0>0$
such that for every
$\rho _0>0$
such that for every
![]() $y,z\in B(x,\rho _0)\cap W^u(x,f)$
, the following properties hold:
$y,z\in B(x,\rho _0)\cap W^u(x,f)$
, the following properties hold:
-
(a)
 $\|y-z- (D_yf)^{-1}(f(y)-f(z)) \| \leq \varepsilon \|y-z\|$
;
$\|y-z- (D_yf)^{-1}(f(y)-f(z)) \| \leq \varepsilon \|y-z\|$
; -
(b)
 $|\log \alpha _i(y,f) - \log \alpha _i(z,f)|\leq \varepsilon $
for
$|\log \alpha _i(y,f) - \log \alpha _i(z,f)|\leq \varepsilon $
for
 $i=1,2,\ldots ,u$
.
$i=1,2,\ldots ,u$
.
See [Reference Jordan and Pollicott17, Lemma 4] for the detailed proof of the above properties. Fix
![]() $0<\rho <\rho _0$
. Let
$0<\rho <\rho _0$
. Let
![]() $A:= B^u(x,\rho )$
and
$A:= B^u(x,\rho )$
and
![]() $a=\mathcal {H}^t_\rho (f(A))$
. Assume that a is finite, otherwise the conclusion is clear. For every
$a=\mathcal {H}^t_\rho (f(A))$
. Assume that a is finite, otherwise the conclusion is clear. For every
![]() $\eta>0$
, there are points
$\eta>0$
, there are points
![]() $\{z_j\}\subset f(B(x,\rho _0)\cap W^u(x,f))$
such that
$\{z_j\}\subset f(B(x,\rho _0)\cap W^u(x,f))$
such that
 $$ \begin{align*} f(A) \subset \bigcup_{j}B^u(z_j, r_j) \end{align*} $$
$$ \begin{align*} f(A) \subset \bigcup_{j}B^u(z_j, r_j) \end{align*} $$
with
![]() $r_j\leq \rho $
for each j and
$r_j\leq \rho $
for each j and
 $$ \begin{align*} \sum_{j}r_j^t<a+\eta. \end{align*} $$
$$ \begin{align*} \sum_{j}r_j^t<a+\eta. \end{align*} $$
Let
![]() $B_j'=\{y\in A: f(y)\in B^u(z_j,r_j)\}$
, then
$B_j'=\{y\in A: f(y)\in B^u(z_j,r_j)\}$
, then
![]() $A\subset \bigcup _j B_j'$
. By property (a), we conclude that
$A\subset \bigcup _j B_j'$
. By property (a), we conclude that
![]() $B_j'$
is contained in an ellipse with principal axes
$B_j'$
is contained in an ellipse with principal axes
where
![]() $y_j\in B^u(x,\rho )$
and
$y_j\in B^u(x,\rho )$
and
![]() $f(y_j)=z_j$
. This together with property (b) yield that
$f(y_j)=z_j$
. This together with property (b) yield that
![]() $B_j'$
is contained in an ellipse with principal axes
$B_j'$
is contained in an ellipse with principal axes
Hence,
![]() $B_j'$
is covered by
$B_j'$
is covered by
 $$ \begin{align*}\frac{\exp\{-\sum_{j=u-[t]+1}^u \log\alpha_j(x,f) \} }{ \exp\{-[t]\log\alpha_{u-[t]}(x,f) \}}\end{align*} $$
$$ \begin{align*}\frac{\exp\{-\sum_{j=u-[t]+1}^u \log\alpha_j(x,f) \} }{ \exp\{-[t]\log\alpha_{u-[t]}(x,f) \}}\end{align*} $$
balls with radius
![]() $({e^\varepsilon }/({1-\varepsilon })) \sqrt {u} r_j\cdot \exp \{-\log \alpha _{u-[t]}(x,f)\}$
. In fact, the radius
$({e^\varepsilon }/({1-\varepsilon })) \sqrt {u} r_j\cdot \exp \{-\log \alpha _{u-[t]}(x,f)\}$
. In fact, the radius
 $$ \begin{align*} &\hspace{-6pt}\frac{e^\varepsilon}{1-\varepsilon} \sqrt{u} r_j \cdot \exp\{-\log\alpha_{u-[t]}(x,f)\}\\ &\leq \ 2\sqrt{u}\exp\{-\log\alpha_{u-[t]}(x,f)\} \cdot \rho\\ &\leq \ b\rho. \end{align*} $$
$$ \begin{align*} &\hspace{-6pt}\frac{e^\varepsilon}{1-\varepsilon} \sqrt{u} r_j \cdot \exp\{-\log\alpha_{u-[t]}(x,f)\}\\ &\leq \ 2\sqrt{u}\exp\{-\log\alpha_{u-[t]}(x,f)\} \cdot \rho\\ &\leq \ b\rho. \end{align*} $$
Therefore,
 $$ \begin{align*} \mathcal{H}^t_{b\rho}(B_j')&\leq \exp\bigg\{-\sum_{j=u-[t]+1}^u \log\alpha_j(x,f) + [t]\log\alpha_{u-[t]}(x,f) \bigg\}\\ &\quad \cdot \bigg(\frac{e^\varepsilon}{1-\varepsilon} \sqrt{u}\bigg)^t r_j^t \cdot \exp\{-t\log\alpha_{u-[t]}(x,f)\}\\ & \leq (2\sqrt{u})^t \cdot \exp\{-\psi^t(x,f)\} \cdot r_j^t. \end{align*} $$
$$ \begin{align*} \mathcal{H}^t_{b\rho}(B_j')&\leq \exp\bigg\{-\sum_{j=u-[t]+1}^u \log\alpha_j(x,f) + [t]\log\alpha_{u-[t]}(x,f) \bigg\}\\ &\quad \cdot \bigg(\frac{e^\varepsilon}{1-\varepsilon} \sqrt{u}\bigg)^t r_j^t \cdot \exp\{-t\log\alpha_{u-[t]}(x,f)\}\\ & \leq (2\sqrt{u})^t \cdot \exp\{-\psi^t(x,f)\} \cdot r_j^t. \end{align*} $$
Summing up over all j, we have that
 $$ \begin{align*} \mathcal{H}^t_{b\rho}(A)&\leq \sum_{j}\mathcal{H}_{b\rho}^t(B_j')\\ &\leq 2^t (\sqrt{u})^t \exp\{-\psi^t(x,f)\} \cdot \sum_j r_j^t\\ &\leq 2^t (\sqrt{u})^t \exp\{-\psi^t(x,f)\} \cdot (a+\eta). \end{align*} $$
$$ \begin{align*} \mathcal{H}^t_{b\rho}(A)&\leq \sum_{j}\mathcal{H}_{b\rho}^t(B_j')\\ &\leq 2^t (\sqrt{u})^t \exp\{-\psi^t(x,f)\} \cdot \sum_j r_j^t\\ &\leq 2^t (\sqrt{u})^t \exp\{-\psi^t(x,f)\} \cdot (a+\eta). \end{align*} $$
The choice of
![]() $C_0$
and the arbitrariness of
$C_0$
and the arbitrariness of
![]() $\eta>0$
implies the desired result.
$\eta>0$
implies the desired result.
The following result relates the zero of measure-theoretic pressure with the upper bound of the unstable pointwise dimension of
![]() $\mu $
.
$\mu $
.
Lemma 3.2. For
![]() $\mu $
-a.e. x,
$\mu $
-a.e. x,
![]() $d_\mu ^u(x)\leq t_{u,1}^*$
, where
$d_\mu ^u(x)\leq t_{u,1}^*$
, where
![]() $t_{u,1}^*$
is the unique solution of the equation
$t_{u,1}^*$
is the unique solution of the equation
![]() $P_{\mu }(f, -\psi ^t(\cdot ,f))=0$
.
$P_{\mu }(f, -\psi ^t(\cdot ,f))=0$
.
Proof. Fix a small number
![]() $\varepsilon>0$
such that
$\varepsilon>0$
such that
![]() $-\unicode{x3bb} _u(\mu )+2\varepsilon <0$
and choose
$-\unicode{x3bb} _u(\mu )+2\varepsilon <0$
and choose
![]() $t>t_{u,1}^*$
such that
$t>t_{u,1}^*$
such that
Claim. There exists an integer
![]() $N_1$
(depending only on
$N_1$
(depending only on
![]() $\varepsilon $
) such that, for
$\varepsilon $
) such that, for
![]() $\mu $
-a.e. x and every
$\mu $
-a.e. x and every
![]() $N\ge N_1$
, the Birkhoff averages
$N\ge N_1$
, the Birkhoff averages
 $$ \begin{align*} \frac{1}{kN}\sum_{j=0}^{k-1}\log \alpha_{u}(f^{jN}x, f^N) \end{align*} $$
$$ \begin{align*} \frac{1}{kN}\sum_{j=0}^{k-1}\log \alpha_{u}(f^{jN}x, f^N) \end{align*} $$
converge towards a number bigger than
![]() $\unicode{x3bb} _u(\mu )-\varepsilon $
, as k goes to
$\unicode{x3bb} _u(\mu )-\varepsilon $
, as k goes to
![]() $+\infty $
.
$+\infty $
.
Proof of the Claim
We give the proof of the Claim by modifying slightly the arguments in the proof of [Reference Abdenur, Bonatti and Crovisier1, Lemma 8.4].
Since
![]() ${\lim _{n\to \infty } ({1}/{n}) \log \alpha _u(x,f^n) = \lim _{n\to \infty } (1/n) \int \log \alpha _u(x,f^n)\,d\mu = \unicode{x3bb} _u(\mu )}$
for
${\lim _{n\to \infty } ({1}/{n}) \log \alpha _u(x,f^n) = \lim _{n\to \infty } (1/n) \int \log \alpha _u(x,f^n)\,d\mu = \unicode{x3bb} _u(\mu )}$
for
![]() $\mu $
-a.e. x, there exists a positive integer L such that
$\mu $
-a.e. x, there exists a positive integer L such that
The measure
![]() $\mu $
may be not ergodic for
$\mu $
may be not ergodic for
![]() $f^L$
, one can decompose it as
$f^L$
, one can decompose it as
where
![]() $m\in \mathbb {N}^+$
divides L and each
$m\in \mathbb {N}^+$
divides L and each
![]() $\mu _i$
is an ergodic
$\mu _i$
is an ergodic
![]() $f^L$
-invariant measure such that
$f^L$
-invariant measure such that
![]() $f_*\mu _i=\mu _{i+1}$
for each
$f_*\mu _i=\mu _{i+1}$
for each
![]() $i(\text {mod}\,\, m)$
. Let
$i(\text {mod}\,\, m)$
. Let
![]() $A_1\cup A_2\cup \cdots \cup A_m$
be a measurable partition of
$A_1\cup A_2\cup \cdots \cup A_m$
be a measurable partition of
![]() $(M,\mu )$
such that
$(M,\mu )$
such that
![]() $f(A_i)=A_{i+1}$
for each
$f(A_i)=A_{i+1}$
for each
![]() $i(\text {mod}\,\, m)$
and
$i(\text {mod}\,\, m)$
and
![]() $\mu _i(A_i)=1$
. By equation (11), there exists
$\mu _i(A_i)=1$
. By equation (11), there exists
![]() $j_0\in \{1,2,\ldots , m\}$
such that
$j_0\in \{1,2,\ldots , m\}$
such that
For every
![]() $N\ge 1$
and
$N\ge 1$
and
![]() $\mu $
-a.e. x, one decomposes the orbit
$\mu $
-a.e. x, one decomposes the orbit
![]() $\{f^i(x)\}_{i=0}^{N-1}$
as
$\{f^i(x)\}_{i=0}^{N-1}$
as
![]() $(x,\ldots, f^{j-1}(x))$
,
$(x,\ldots, f^{j-1}(x))$
,
![]() $(f^j(x),\ldots ,f^{j+(r-1)L-1}(x))$
and
$(f^j(x),\ldots ,f^{j+(r-1)L-1}(x))$
and
![]() $(f^{j+(r-1)L}(x),\ldots , f^{N-1}(x))$
, where
$(f^{j+(r-1)L}(x),\ldots , f^{N-1}(x))$
, where
![]() $j{\kern-1.2pt}<{\kern-1.2pt}L$
,
$j{\kern-1.2pt}<{\kern-1.2pt}L$
,
![]() $j+rL{\kern-1.2pt}\ge{\kern-1.2pt} N$
and the points
$j+rL{\kern-1.2pt}\ge{\kern-1.2pt} N$
and the points
![]() $\{f^{j+sL}(x)\}_{s=0}^{r}$
belong to
$\{f^{j+sL}(x)\}_{s=0}^{r}$
belong to
![]() $A_{j_0}$
. Using the super-additivity of
$A_{j_0}$
. Using the super-additivity of
![]() $\{\log \alpha _u(x,f^n) \}_{n\ge 1}$
, we have that
$\{\log \alpha _u(x,f^n) \}_{n\ge 1}$
, we have that
 $$ \begin{align*} \log \alpha_u(x,f^N)&\ge \log \alpha_u(x,f^j) + \sum_{s=0}^{r-2} \log \alpha_u(f^{j+sL}x,f^L)\\ &\quad +\log \alpha_u(f^{j+(r-1)L}x,f^{N-j-L(r-1)}). \end{align*} $$
$$ \begin{align*} \log \alpha_u(x,f^N)&\ge \log \alpha_u(x,f^j) + \sum_{s=0}^{r-2} \log \alpha_u(f^{j+sL}x,f^L)\\ &\quad +\log \alpha_u(f^{j+(r-1)L}x,f^{N-j-L(r-1)}). \end{align*} $$
Hence, one has
 $$ \begin{align*} \log \alpha_u(x,f^N)\ge 2C_f + \sum_{s=0}^{r-2} \log \alpha_u(f^{j+sL}x,f^L), \end{align*} $$
$$ \begin{align*} \log \alpha_u(x,f^N)\ge 2C_f + \sum_{s=0}^{r-2} \log \alpha_u(f^{j+sL}x,f^L), \end{align*} $$
where
![]() ${C_f=\max _{0\le i<L}\max _{x\in M}|{\log}\, \alpha _u(x,f^i)|}$
with the convention that
${C_f=\max _{0\le i<L}\max _{x\in M}|{\log}\, \alpha _u(x,f^i)|}$
with the convention that
![]() $|{\log}\, \alpha _u (x,f^0)|=0$
. Since
$|{\log}\, \alpha _u (x,f^0)|=0$
. Since

and
 $$ \begin{align*} \lim_{k\to +\infty}\frac{1}{kN}\sum_{j=0}^{k-1}\log \alpha_{u}(f^{jN}x, f^N)\ge \frac{2C_f}{N}+\lim_{k\to +\infty} \frac{1}{kL}\sum_{\ell=0}^{k-1}\log \alpha_u(f^{j+\ell L}x,f^L), \end{align*} $$
$$ \begin{align*} \lim_{k\to +\infty}\frac{1}{kN}\sum_{j=0}^{k-1}\log \alpha_{u}(f^{jN}x, f^N)\ge \frac{2C_f}{N}+\lim_{k\to +\infty} \frac{1}{kL}\sum_{\ell=0}^{k-1}\log \alpha_u(f^{j+\ell L}x,f^L), \end{align*} $$
and there exists an integer
![]() $N_1$
(depending on
$N_1$
(depending on
![]() $\varepsilon $
) so that
$\varepsilon $
) so that
![]() $|{2C_f}/{N}|< {\varepsilon }/{2}$
for every
$|{2C_f}/{N}|< {\varepsilon }/{2}$
for every
![]() $N>N_1$
, for
$N>N_1$
, for
![]() $\mu $
-a.e. x and every
$\mu $
-a.e. x and every
![]() $N>N_1$
, we have that
$N>N_1$
, we have that

Take
![]() $b_0>2\sqrt {u}$
and
$b_0>2\sqrt {u}$
and
![]() $C_0>2^tu^{{t}/{2}}$
, choose
$C_0>2^tu^{{t}/{2}}$
, choose
![]() $N>N_1$
large enough such that
$N>N_1$
large enough such that
By the above Claim and Birkhoff ergodic theorem, for
![]() $\mu $
-a.e.
$\mu $
-a.e.
![]() $x\in M$
, we have that
$x\in M$
, we have that

and
 $$ \begin{align*} \lim_{n\to\infty}\frac{1}{nN}\sum_{j=0}^{nN-1} \psi^t(f^jx,f)=\int\psi^t(x,f)\,d\mu. \end{align*} $$
$$ \begin{align*} \lim_{n\to\infty}\frac{1}{nN}\sum_{j=0}^{nN-1} \psi^t(f^jx,f)=\int\psi^t(x,f)\,d\mu. \end{align*} $$
Let
![]() $\rho _0$
be as in Lemma 3.1. Fix
$\rho _0$
be as in Lemma 3.1. Fix
![]() $\delta \in (0,\rho _0)$
. Ledrappier and Young [Reference Ledrappier and Young22] proved that
$\delta \in (0,\rho _0)$
. Ledrappier and Young [Reference Ledrappier and Young22] proved that
 $$ \begin{align*} \limsup_{n\to\infty} \frac{-\log\mu_x^u(B^u(x,n,\delta/2))}{n} &\leq \lim_{\delta\to0}\limsup_{n\to\infty} \frac{-\log\mu_x^u(B^u(x,n,{\delta}/{2}))}{n}\\ &=h_\mu(f)\, \mu\text{-a.e.}\, x, \end{align*} $$
$$ \begin{align*} \limsup_{n\to\infty} \frac{-\log\mu_x^u(B^u(x,n,\delta/2))}{n} &\leq \lim_{\delta\to0}\limsup_{n\to\infty} \frac{-\log\mu_x^u(B^u(x,n,{\delta}/{2}))}{n}\\ &=h_\mu(f)\, \mu\text{-a.e.}\, x, \end{align*} $$
where
![]() $B^u(x,n, \delta /2):=\{y\in W^u(x,f): d^u(f^jx,f^jy)< \delta /2 \text { for } 0\leq j<n\}$
. Hence, one can find sets
$B^u(x,n, \delta /2):=\{y\in W^u(x,f): d^u(f^jx,f^jy)< \delta /2 \text { for } 0\leq j<n\}$
. Hence, one can find sets
![]() $A_n\subset \Gamma $
with
$A_n\subset \Gamma $
with
![]() $\mu (A_n)\to 1\, (n\to \infty )$
, for every
$\mu (A_n)\to 1\, (n\to \infty )$
, for every
![]() $x\in A_n$
where the following properties hold:
$x\in A_n$
where the following properties hold:
-
(a)
 ${\exp [-nN(h_\mu (f)+\varepsilon )] \leq \mu _x^u(B^u(x,nN, {\delta }/{2}))}$
;
${\exp [-nN(h_\mu (f)+\varepsilon )] \leq \mu _x^u(B^u(x,nN, {\delta }/{2}))}$
; -
(b)
 ${nN(-\int \psi ^t(x,f)-\varepsilon ) \leq -\sum _{j=0}^{nN-1}\psi ^t(f^{j}x,f) \leq nN(-\int \psi ^t(x,f)+\varepsilon )}$
;
${nN(-\int \psi ^t(x,f)-\varepsilon ) \leq -\sum _{j=0}^{nN-1}\psi ^t(f^{j}x,f) \leq nN(-\int \psi ^t(x,f)+\varepsilon )}$
; -
(c)
 ${\sum _{j=0}^{n-1}\log \alpha _{u-[t]}(f^{jN}x, f^N) \geq nN(\unicode{x3bb} _u(\mu )-2\varepsilon )}$
.
${\sum _{j=0}^{n-1}\log \alpha _{u-[t]}(f^{jN}x, f^N) \geq nN(\unicode{x3bb} _u(\mu )-2\varepsilon )}$
.
Take a point
![]() $x\in A_n$
. Let E be a maximal
$x\in A_n$
. Let E be a maximal
![]() $(nN, \delta )$
-separated subset of
$(nN, \delta )$
-separated subset of
![]() $A_n\cap \xi ^u(x)$
, then
$A_n\cap \xi ^u(x)$
, then
 $$ \begin{align*} A_n\cap\xi^u(x) \subset \bigcup_{x_j\in E} B^u(x_j, nN, \delta). \end{align*} $$
$$ \begin{align*} A_n\cap\xi^u(x) \subset \bigcup_{x_j\in E} B^u(x_j, nN, \delta). \end{align*} $$
Furthermore, by property (a), the number of balls
![]() $B^u(x_j,nN, \delta /2)$
is less than or equal to
$B^u(x_j,nN, \delta /2)$
is less than or equal to
![]() $\exp \{nN[h_\mu (f)+\varepsilon ]\}$
. Let
$\exp \{nN[h_\mu (f)+\varepsilon ]\}$
. Let
 $$ \begin{align*}b_k(x)=(b_0)^k \exp \bigg[-\sum_{j=n-k}^{n-1}\log\alpha_{u-[t]}(f^{jN}x, f^N)\bigg]\end{align*} $$
$$ \begin{align*}b_k(x)=(b_0)^k \exp \bigg[-\sum_{j=n-k}^{n-1}\log\alpha_{u-[t]}(f^{jN}x, f^N)\bigg]\end{align*} $$
for
![]() $k=1,2,\ldots , n$
and
$k=1,2,\ldots , n$
and
![]() $\beta _n=\{b_0\exp [(-\unicode{x3bb} _u(\mu )+2\varepsilon )N]\}^n \cdot \rho $
, where
$\beta _n=\{b_0\exp [(-\unicode{x3bb} _u(\mu )+2\varepsilon )N]\}^n \cdot \rho $
, where
![]() $0<\rho <\rho _0$
. By property (c), we have
$0<\rho <\rho _0$
. By property (c), we have

For each
![]() $x_j\in E$
, using Lemma 3.1 n times, we conclude that
$x_j\in E$
, using Lemma 3.1 n times, we conclude that
 $$ \begin{align*} \mathcal{H}^t_{\beta_n}(B^u(x_j, nN, \delta)) &\leq \mathcal{H}^t_{b_n(x_j)\rho}(B^u(x_j,nN,\delta))\\ &\leq C_0 \exp\{-\psi^t(x_j, f^N)\} \cdot \mathcal{H}^t_{b_{n-1}(x_j)\rho} (f^N(B^u(x_j,nN, \delta)))\\ &\leq C_0 \exp\{-\psi^t(x_j, f^N)\} \cdot \mathcal{H}^t_{b_{n-1}(x_j)\rho} (B^u(f^N(x_j),(n-1)N, \delta))\\ &\leq (C_0)^2 \exp\{-\psi^t(x_j, f^N)\} \cdot \exp\{-\psi^t(f^N(x_j), f^N)\} \\ &\quad\cdot \mathcal{H}^t_{b_{n-2}(x_j)\rho} (B^u(f^{2N}(x_j),(n-2)N, \delta))\\ &\leq \cdots\\&\leq (C_0)^n \exp\bigg\{ -\sum_{j=0}^{n-1}\psi^t(f^{jN}x_j, f^N) \bigg\} \cdot \mathcal{H}^t_\rho(B^u(f^{nN}x_j, \delta))\\ &\leq (C_0)^n C_1 \cdot \exp\bigg\{ -\sum_{j=0}^{n-1}\psi^t(f^{jN}x_j, f^N) \bigg\}, \end{align*} $$
$$ \begin{align*} \mathcal{H}^t_{\beta_n}(B^u(x_j, nN, \delta)) &\leq \mathcal{H}^t_{b_n(x_j)\rho}(B^u(x_j,nN,\delta))\\ &\leq C_0 \exp\{-\psi^t(x_j, f^N)\} \cdot \mathcal{H}^t_{b_{n-1}(x_j)\rho} (f^N(B^u(x_j,nN, \delta)))\\ &\leq C_0 \exp\{-\psi^t(x_j, f^N)\} \cdot \mathcal{H}^t_{b_{n-1}(x_j)\rho} (B^u(f^N(x_j),(n-1)N, \delta))\\ &\leq (C_0)^2 \exp\{-\psi^t(x_j, f^N)\} \cdot \exp\{-\psi^t(f^N(x_j), f^N)\} \\ &\quad\cdot \mathcal{H}^t_{b_{n-2}(x_j)\rho} (B^u(f^{2N}(x_j),(n-2)N, \delta))\\ &\leq \cdots\\&\leq (C_0)^n \exp\bigg\{ -\sum_{j=0}^{n-1}\psi^t(f^{jN}x_j, f^N) \bigg\} \cdot \mathcal{H}^t_\rho(B^u(f^{nN}x_j, \delta))\\ &\leq (C_0)^n C_1 \cdot \exp\bigg\{ -\sum_{j=0}^{n-1}\psi^t(f^{jN}x_j, f^N) \bigg\}, \end{align*} $$
where
![]() $C_1=\sup _{y\in M}\mathcal {H}^t_\rho (B(y,\delta ))$
. By property (b) and the sub-additivity of
$C_1=\sup _{y\in M}\mathcal {H}^t_\rho (B(y,\delta ))$
. By property (b) and the sub-additivity of
![]() $\{-\psi ^t(\cdot ,f^n)\}_{n\geq 1}$
, we have that
$\{-\psi ^t(\cdot ,f^n)\}_{n\geq 1}$
, we have that
 $$ \begin{align*} \mathcal{H}_{\beta_n}^t(A_n\cap\xi^u(x)) &\leq \sum_{x_j\in E}\mathcal{H}^t_{\beta_n}(B^u(x_j, nN, \delta))\\ &\leq \sum_{x_j\in E} (C_0)^n C_1 \cdot \exp\bigg\{ -\sum_{i=0}^{n-1}\psi^t(f^{jN}x_j, f^N) \bigg\}\\ &\leq \sum_{x_j\in E} (C_0)^n C_1 \cdot \exp\bigg\{ -\sum_{i=0}^{nN-1}\psi^t(f^{i}x_j, f) \bigg\}\\ &\leq (C_0)^n C_1 \cdot \exp[nN(h_\mu(f)+\varepsilon)] \cdot \exp\bigg[nN\bigg({-}\int\psi^t(x,f)\,d\mu + \varepsilon\bigg)\kern-1pt\bigg] \\ &= (C_0)^n C_1 \cdot \exp\bigg[nN\bigg(h_\mu(f)- \int\psi^t(x,f)\,d\mu +2\varepsilon\bigg) \bigg]\\ &= (C_0)^n C_1\cdot e^{-nN\varepsilon}\\ &=(C_0 e^{-N\varepsilon})^n C_1. \end{align*} $$
$$ \begin{align*} \mathcal{H}_{\beta_n}^t(A_n\cap\xi^u(x)) &\leq \sum_{x_j\in E}\mathcal{H}^t_{\beta_n}(B^u(x_j, nN, \delta))\\ &\leq \sum_{x_j\in E} (C_0)^n C_1 \cdot \exp\bigg\{ -\sum_{i=0}^{n-1}\psi^t(f^{jN}x_j, f^N) \bigg\}\\ &\leq \sum_{x_j\in E} (C_0)^n C_1 \cdot \exp\bigg\{ -\sum_{i=0}^{nN-1}\psi^t(f^{i}x_j, f) \bigg\}\\ &\leq (C_0)^n C_1 \cdot \exp[nN(h_\mu(f)+\varepsilon)] \cdot \exp\bigg[nN\bigg({-}\int\psi^t(x,f)\,d\mu + \varepsilon\bigg)\kern-1pt\bigg] \\ &= (C_0)^n C_1 \cdot \exp\bigg[nN\bigg(h_\mu(f)- \int\psi^t(x,f)\,d\mu +2\varepsilon\bigg) \bigg]\\ &= (C_0)^n C_1\cdot e^{-nN\varepsilon}\\ &=(C_0 e^{-N\varepsilon})^n C_1. \end{align*} $$
Since N satisfies
![]() $C_0e^{-N\varepsilon }<1$
, we have that
$C_0e^{-N\varepsilon }<1$
, we have that
Since
![]() ${\lim _{n\to \infty }\beta _n=0}$
and
${\lim _{n\to \infty }\beta _n=0}$
and
![]() ${\lim _{n\to \infty }\mu _x^u(A_n\cap \xi ^u(x))= 1}$
for
${\lim _{n\to \infty }\mu _x^u(A_n\cap \xi ^u(x))= 1}$
for
![]() $\mu $
-a.e. x, by [Reference Jordan and Pollicott17, Lemma 6], we obtain that
$\mu $
-a.e. x, by [Reference Jordan and Pollicott17, Lemma 6], we obtain that
for
![]() $\mu $
-a.e. x. Combining with equation (5) and the choice of t yield that
$\mu $
-a.e. x. Combining with equation (5) and the choice of t yield that
![]() $d_\mu ^u(x)\leq t_{u,1}^*$
for
$d_\mu ^u(x)\leq t_{u,1}^*$
for
![]() $\mu $
-a.e. x.
$\mu $
-a.e. x.
Now we are ready to present the proof of Theorem B.
Proof of Theorem B
For each
![]() $n>1$
, the measure
$n>1$
, the measure
![]() $\mu $
is f-invariant ergodic, but it may be not ergodic for
$\mu $
is f-invariant ergodic, but it may be not ergodic for
![]() $f^n$
although
$f^n$
although
![]() $\mu $
is still
$\mu $
is still
![]() $f^n$
-invariant. In either case, one can find an
$f^n$
-invariant. In either case, one can find an
![]() $f^n$
-invariant ergodic probability measure
$f^n$
-invariant ergodic probability measure
![]() $\nu $
such that
$\nu $
such that
where
![]() $m\in \mathbb {N}\setminus \{0\}$
divides n. Let
$m\in \mathbb {N}\setminus \{0\}$
divides n. Let
then one can show that
 $$ \begin{align*} \widetilde{P}_\mu(f^n, -\psi^n(\cdot, f^n))&= \frac1m \sum_{i=0}^{m-1}\bigg( h_{f_*^i\nu}(f^n)-\int \psi^n(x, f^n) \,df_*^i\nu \bigg)\\ &=\frac1m \sum_{i=0}^{m-1} P_{f_*^i\nu}(f^n, -\psi^n(\cdot, f^n)). \end{align*} $$
$$ \begin{align*} \widetilde{P}_\mu(f^n, -\psi^n(\cdot, f^n))&= \frac1m \sum_{i=0}^{m-1}\bigg( h_{f_*^i\nu}(f^n)-\int \psi^n(x, f^n) \,df_*^i\nu \bigg)\\ &=\frac1m \sum_{i=0}^{m-1} P_{f_*^i\nu}(f^n, -\psi^n(\cdot, f^n)). \end{align*} $$
Hence, there exists
![]() $j_0\in \{0,1,\ldots , m-1\}$
such that
$j_0\in \{0,1,\ldots , m-1\}$
such that
Since
![]() $f_*^{j_0}\nu $
is hyperbolic and
$f_*^{j_0}\nu $
is hyperbolic and
![]() $f^n$
-invariant ergodic, by Lemma 3.2, there is a set
$f^n$
-invariant ergodic, by Lemma 3.2, there is a set
![]() $\tilde {A}$
with
$\tilde {A}$
with
![]() $\nu \circ f^{-j_0}(\tilde {A})=1$
such that for each
$\nu \circ f^{-j_0}(\tilde {A})=1$
such that for each
![]() $x\in \tilde {A}$
,
$x\in \tilde {A}$
,
where
![]() $t_{u,n}^*$
is the unique root of the equation
$t_{u,n}^*$
is the unique root of the equation
![]() $P_\mu (f^n,-\psi ^t(\cdot , f^n))=0$
. Note that
$P_\mu (f^n,-\psi ^t(\cdot , f^n))=0$
. Note that
![]() $d_\mu ^u(x), d_{f_*^{j_0}\nu }^u(x)$
are constants almost everywhere (see [Reference Ledrappier and Young22]) and
$d_\mu ^u(x), d_{f_*^{j_0}\nu }^u(x)$
are constants almost everywhere (see [Reference Ledrappier and Young22]) and
![]() $d_\mu ^u(x)\leq d_{f_*^{j_0}\nu }^u(x) \leq t_{u,n}^*$
for each
$d_\mu ^u(x)\leq d_{f_*^{j_0}\nu }^u(x) \leq t_{u,n}^*$
for each
![]() $x\in \tilde {A}$
with
$x\in \tilde {A}$
with
![]() $\mu (\tilde {A})\geq 1/m$
. Consequently, we have that
$\mu (\tilde {A})\geq 1/m$
. Consequently, we have that
for
![]() $\mu $
-a.e. x.
$\mu $
-a.e. x.
By the sub-additive of
![]() $\{-\psi ^t(\cdot , f^n)\}_{n\geq 1}$
, we obtain
$\{-\psi ^t(\cdot , f^n)\}_{n\geq 1}$
, we obtain
 $$ \begin{align*}\frac{1}{2^{k+1}} \bigg[h_\mu(f^{2^{k+1}}) - \int \psi^t(x,f^{2^{k+1}})\, d\mu\bigg] \leq \frac{1}{2^k} \bigg[ h_\mu(f^{2^k}) -\int \psi^t(x,f^{2^k})\, d\mu \bigg]. \end{align*} $$
$$ \begin{align*}\frac{1}{2^{k+1}} \bigg[h_\mu(f^{2^{k+1}}) - \int \psi^t(x,f^{2^{k+1}})\, d\mu\bigg] \leq \frac{1}{2^k} \bigg[ h_\mu(f^{2^k}) -\int \psi^t(x,f^{2^k})\, d\mu \bigg]. \end{align*} $$
Hence,
This yields that
![]() $t_{u,2^{k+1}}^*\leq t_{u,2^k}^*$
for every
$t_{u,2^{k+1}}^*\leq t_{u,2^k}^*$
for every
![]() $k\ge 1$
. Let
$k\ge 1$
. Let
![]() ${t_u^*:=\lim _{k\to \infty } t_{u, 2^k}^*}$
, then one has that
${t_u^*:=\lim _{k\to \infty } t_{u, 2^k}^*}$
, then one has that
Since
![]() $P_\mu (f, \{-\psi ^t(\cdot , f^n)\})$
is continuous and strictly decreasing with respect to t, there exists at most one solution of the equation. To complete the proof of Theorem B, it suffices to show that
$P_\mu (f, \{-\psi ^t(\cdot , f^n)\})$
is continuous and strictly decreasing with respect to t, there exists at most one solution of the equation. To complete the proof of Theorem B, it suffices to show that
![]() $P_\mu (f, \{-\psi ^{t^*}(\cdot , f^n)\})=0$
.
$P_\mu (f, \{-\psi ^{t^*}(\cdot , f^n)\})=0$
.
Since
![]() $t_{u,2^k}^*\ge t_u^{*}$
for every
$t_{u,2^k}^*\ge t_u^{*}$
for every
![]() $k\ge 1$
, by Theorem 2.2, one has that
$k\ge 1$
, by Theorem 2.2, one has that
However, for each small number
![]() $\varepsilon>0$
, there exists K so that
$\varepsilon>0$
, there exists K so that
![]() $t_{u,2^k}^*\le t_u^{*}+\varepsilon $
for every
$t_{u,2^k}^*\le t_u^{*}+\varepsilon $
for every
![]() ${k\ge K}$
. Hence, we have that
${k\ge K}$
. Hence, we have that
 $$ \begin{align*} P_\mu(f, \{-\psi^{t_u^*+\varepsilon}(\cdot, f^n)\}) &= h_\mu(f) - \lim_{n\to\infty} \frac1n\int\psi^{t_u^*+\varepsilon}(x,f^n)\, d\mu\\ &= \lim_{k\to\infty} \frac{1}{2^k}\widetilde{P}_\mu(f^{2^k}, -\psi^{t_u^*+\varepsilon}(\cdot, f^{2^k}))\le 0. \end{align*} $$
$$ \begin{align*} P_\mu(f, \{-\psi^{t_u^*+\varepsilon}(\cdot, f^n)\}) &= h_\mu(f) - \lim_{n\to\infty} \frac1n\int\psi^{t_u^*+\varepsilon}(x,f^n)\, d\mu\\ &= \lim_{k\to\infty} \frac{1}{2^k}\widetilde{P}_\mu(f^{2^k}, -\psi^{t_u^*+\varepsilon}(\cdot, f^{2^k}))\le 0. \end{align*} $$
The previous arguments imply that
![]() $P_\mu (f, \{-\psi ^{t^*}(\cdot , f^n)\})=0$
. One can prove in a similar fashion that
$P_\mu (f, \{-\psi ^{t^*}(\cdot , f^n)\})=0$
. One can prove in a similar fashion that
![]() $d_\mu ^s(x)\leq t_s^*$
for
$d_\mu ^s(x)\leq t_s^*$
for
![]() $\mu $
-a.e. x. This completes the proof of Theorem B.
$\mu $
-a.e. x. This completes the proof of Theorem B.
3.3 Proof of Theorem C
For each
![]() $\varepsilon>0$
, there exists a hyperbolic set
$\varepsilon>0$
, there exists a hyperbolic set
![]() $\Lambda _\varepsilon $
satisfying properties (a)–(d) in Theorem 2.4. The following lemma shows that the zero of the super-additive topological pressure of
$\Lambda _\varepsilon $
satisfying properties (a)–(d) in Theorem 2.4. The following lemma shows that the zero of the super-additive topological pressure of
![]() $\Phi _f(t)$
provides a lower bound of the Carathéodory singular dimension of the hyperbolic set on the local unstable leaf with respect to the super-additive singular valued potential
$\Phi _f(t)$
provides a lower bound of the Carathéodory singular dimension of the hyperbolic set on the local unstable leaf with respect to the super-additive singular valued potential
![]() $\Phi _f(t)$
.
$\Phi _f(t)$
.
Lemma 3.3. Let
![]() $f: M\to M$
be a
$f: M\to M$
be a
![]() $C^{1+\alpha }$
diffeomorphism on an
$C^{1+\alpha }$
diffeomorphism on an
![]() $m_0$
-dimensional compact smooth Riemannian manifold M and let
$m_0$
-dimensional compact smooth Riemannian manifold M and let
![]() $\Lambda \subset M$
be a hyperbolic set. Assume that
$\Lambda \subset M$
be a hyperbolic set. Assume that
![]() $f|_{\Lambda }$
is topologically transitive, then for every
$f|_{\Lambda }$
is topologically transitive, then for every
![]() $x\in \Lambda $
,
$x\in \Lambda $
,
where
![]() $t_*$
is the unique root of the equation
$t_*$
is the unique root of the equation
![]() $P(f|_\Lambda , \Phi _f(t))=0$
.
$P(f|_\Lambda , \Phi _f(t))=0$
.
Proof. For every
![]() $x\in \Lambda $
, we denote
$x\in \Lambda $
, we denote
![]() $Z=\Lambda \cap W^u_{\mathrm {loc}}(x, f)$
and
$Z=\Lambda \cap W^u_{\mathrm {loc}}(x, f)$
and
![]() $P(t)=P(f|_\Lambda , \Phi _f(t))$
. Since the function
$P(t)=P(f|_\Lambda , \Phi _f(t))$
. Since the function
![]() $P(t)$
is strictly decreasing in t, then for each
$P(t)$
is strictly decreasing in t, then for each
![]() $t<t_*$
, we have that
$t<t_*$
, we have that
![]() $P(t)>0$
. Fix such a number t and take
$P(t)>0$
. Fix such a number t and take
![]() $\varepsilon>0$
with
$\varepsilon>0$
with
![]() $P(t)-\varepsilon>0$
. By Proposition 2.2, one has that
$P(t)-\varepsilon>0$
. By Proposition 2.2, one has that
then there exists
![]() $N_1\in \mathbb {N}$
such that for every
$N_1\in \mathbb {N}$
such that for every
![]() $n\geq N_1$
, we obtain
$n\geq N_1$
, we obtain
Fix an integer
![]() $L\geq N_1$
, by [Reference Climenhaga, Pesin and Zelerowicz11, Proposition 5.4], one has that
$L\geq N_1$
, by [Reference Climenhaga, Pesin and Zelerowicz11, Proposition 5.4], one has that
Hence, there is
![]() $\delta _1>0$
such that
$\delta _1>0$
such that
for every
![]() $0<\delta <\delta _1$
. Consequently, fixing such a
$0<\delta <\delta _1$
. Consequently, fixing such a
![]() $\delta>0$
, one has that
$\delta>0$
, one has that
Hence, for each
![]() $K>0$
, there exists
$K>0$
, there exists
![]() $S\in \mathbb {N}$
such that for each
$S\in \mathbb {N}$
such that for each
![]() $N\ge S$
, we have that
$N\ge S$
, we have that
 $$ \begin{align} \nonumber K & \leq \inf \sum_i\exp [-(P(t)-\varepsilon)Lm_i -S_{m_i}\phi^t(x_i, f^L)] \\ &\le e^{-NL(P(t)-\varepsilon)} \inf \sum_i\exp[ -S_{m_i}\phi^t(x_i, f^L)], \end{align} $$
$$ \begin{align} \nonumber K & \leq \inf \sum_i\exp [-(P(t)-\varepsilon)Lm_i -S_{m_i}\phi^t(x_i, f^L)] \\ &\le e^{-NL(P(t)-\varepsilon)} \inf \sum_i\exp[ -S_{m_i}\phi^t(x_i, f^L)], \end{align} $$
where the infimum is taken over all collections
![]() $\{B^u_{m_i}(x_i, \delta , f^L)\}$
with
$\{B^u_{m_i}(x_i, \delta , f^L)\}$
with
![]() $x_i\in \Lambda $
,
$x_i\in \Lambda $
,
![]() $m_i\geq N$
which cover Z,
$m_i\geq N$
which cover Z,
 $-S_{m_i}\phi ^t(x_i, f^L)\kern1.2pt{=}\kern1.2pt{-}\phi ^t(x_i, f^L)\kern1.2pt{-}\kern1.2pt\phi ^t(f^Lx_i,f^L)-\cdots -\phi ^t(f^{(m_i-1)L}x_i, f^L)$
and
$-S_{m_i}\phi ^t(x_i, f^L)\kern1.2pt{=}\kern1.2pt{-}\phi ^t(x_i, f^L)\kern1.2pt{-}\kern1.2pt\phi ^t(f^Lx_i,f^L)-\cdots -\phi ^t(f^{(m_i-1)L}x_i, f^L)$
and
 $$ \begin{align*}\displaystyle{B^u_{m_i}(x_i, \delta, f^L):= \left\{y\in W^u(x_i,f): \max_{0\le j <m_i}d^u(f^{jL}(y), f^{jL}(x_i))<\delta \right\}}.\end{align*} $$
$$ \begin{align*}\displaystyle{B^u_{m_i}(x_i, \delta, f^L):= \left\{y\in W^u(x_i,f): \max_{0\le j <m_i}d^u(f^{jL}(y), f^{jL}(x_i))<\delta \right\}}.\end{align*} $$
Fixing such an N and taking an integer
![]() $R\ge NL$
, let the collection of balls
$R\ge NL$
, let the collection of balls
![]() $\{B_{n_i}^u(x_i,\delta )\}$
with
$\{B_{n_i}^u(x_i,\delta )\}$
with
![]() $x_i\in \Lambda $
,
$x_i\in \Lambda $
,
![]() $n_i\ge R$
be a cover of Z. One can write
$n_i\ge R$
be a cover of Z. One can write
![]() $n_i=m_iL+s_i$
with
$n_i=m_iL+s_i$
with
![]() $0\le s_i<L$
and
$0\le s_i<L$
and
![]() $m_i\geq N$
for each i. Since
$m_i\geq N$
for each i. Since
![]() $B_{n_i}^u(x_i,\delta )\subset B_{m_i}^u(x_i,\delta , f^L)$
for each i, the collection of balls
$B_{n_i}^u(x_i,\delta )\subset B_{m_i}^u(x_i,\delta , f^L)$
for each i, the collection of balls
![]() $B_{m_i}^u(x_i,\delta , f^L)$
is also a cover of Z with
$B_{m_i}^u(x_i,\delta , f^L)$
is also a cover of Z with
![]() $x_i\in \Lambda $
,
$x_i\in \Lambda $
,
![]() $m_i\ge N$
. By the super-additivity of
$m_i\ge N$
. By the super-additivity of
![]() $\{-\phi ^t(\cdot , f^n)\}_{n\ge 1}$
, one has
$\{-\phi ^t(\cdot , f^n)\}_{n\ge 1}$
, one has
 $$ \begin{align*} \sum_{i}\exp [-\phi^t(x_i,f^{n_i})]&\ge \sum_{i}\exp [-S_{m_i}\phi^t(x_i,f^{L})-\phi^t(f^{m_iL}y, f^{s_i})]\\ &\ge C \sum_{i}\exp [-S_{m_i}\phi^t(x_i,f^{L})], \end{align*} $$
$$ \begin{align*} \sum_{i}\exp [-\phi^t(x_i,f^{n_i})]&\ge \sum_{i}\exp [-S_{m_i}\phi^t(x_i,f^{L})-\phi^t(f^{m_iL}y, f^{s_i})]\\ &\ge C \sum_{i}\exp [-S_{m_i}\phi^t(x_i,f^{L})], \end{align*} $$
where
![]() ${C=\min _{0\le s<L}\min _{x\in M}\exp [-\phi ^t(x, f^{s})]}$
. This together with equation (13) yield that
${C=\min _{0\le s<L}\min _{x\in M}\exp [-\phi ^t(x, f^{s})]}$
. This together with equation (13) yield that
Since the cover of Z is taken arbitrarily, one can conclude that
where the infimum is taken over all collections
![]() $\{B^u_{n_i}(x_i, \delta )\}$
with
$\{B^u_{n_i}(x_i, \delta )\}$
with
![]() $x_i\in \Lambda $
,
$x_i\in \Lambda $
,
![]() $n_i\geq NL$
which cover Z. Letting
$n_i\geq NL$
which cover Z. Letting
![]() $N\to \infty $
, we obtain
$N\to \infty $
, we obtain
for every
![]() $t<t_*$
. This implies that
$t<t_*$
. This implies that
Proof of Theorem C(i)
By Lemma 3.3, for every
![]() $x\in \Lambda _\varepsilon $
, we obtain
$x\in \Lambda _\varepsilon $
, we obtain
where
![]() $t_{\varepsilon *}$
is the unique root of the equation
$t_{\varepsilon *}$
is the unique root of the equation
![]() $P(f|_{\Lambda _\varepsilon }, \Phi _f(t))=0$
. By the variational principle of topological entropy, take
$P(f|_{\Lambda _\varepsilon }, \Phi _f(t))=0$
. By the variational principle of topological entropy, take
![]() $\nu \in \mathcal {M}_{\mathrm {inv}}(f|_{\Lambda _\varepsilon })$
such that
$\nu \in \mathcal {M}_{\mathrm {inv}}(f|_{\Lambda _\varepsilon })$
such that
![]() $h_{\text {top}}(f|_{\Lambda _\varepsilon })=h_\nu (f|_{\Lambda _\varepsilon })$
. By properties (b) and (d) in Theorem 2.4, it holds that
$h_{\text {top}}(f|_{\Lambda _\varepsilon })=h_\nu (f|_{\Lambda _\varepsilon })$
. By properties (b) and (d) in Theorem 2.4, it holds that

where
![]() $\unicode{x3bb} _1(\nu )\geq \unicode{x3bb} _2(\nu ) \geq \cdots \geq \unicode{x3bb} _{m_0}(\nu )$
are the Lyapunov exponents of
$\unicode{x3bb} _1(\nu )\geq \unicode{x3bb} _2(\nu ) \geq \cdots \geq \unicode{x3bb} _{m_0}(\nu )$
are the Lyapunov exponents of
![]() $\nu $
. However, let
$\nu $
. However, let
![]() $\tau \in \mathcal {M}_{\mathrm {inv}}(f|_{\Lambda _\varepsilon })$
be an equilibrium state of
$\tau \in \mathcal {M}_{\mathrm {inv}}(f|_{\Lambda _\varepsilon })$
be an equilibrium state of
![]() $P(f|_{\Lambda _\varepsilon }, \Phi _f(t_{\varepsilon *}))$
, then one has that
$P(f|_{\Lambda _\varepsilon }, \Phi _f(t_{\varepsilon *}))$
, then one has that

This together with equation (14) yield that
Hence, we have that
This implies that
![]() ${\lim _{\varepsilon \to 0}t_{\varepsilon *}=t_{u*}}$
, where
${\lim _{\varepsilon \to 0}t_{\varepsilon *}=t_{u*}}$
, where
![]() $t_{u*}$
is the unique root of
$t_{u*}$
is the unique root of
![]() $P_\mu (f, \Phi _f(t))=0$
. Consequently, we have that
$P_\mu (f, \Phi _f(t))=0$
. Consequently, we have that
As a counterpart of Lemma 3.3, we have the following result.
Lemma 3.4. Let
![]() $f: M\to M$
be a
$f: M\to M$
be a
![]() $C^{1+\alpha }$
diffeomorphism on an
$C^{1+\alpha }$
diffeomorphism on an
![]() $m_0$
-dimensional compact smooth Riemannian manifold M and let
$m_0$
-dimensional compact smooth Riemannian manifold M and let
![]() $\Lambda \subset M$
be a hyperbolic set. Assume that
$\Lambda \subset M$
be a hyperbolic set. Assume that
![]() $f|_{\Lambda }$
is topologically transitive. Then for every
$f|_{\Lambda }$
is topologically transitive. Then for every
![]() $x\in \Lambda $
,
$x\in \Lambda $
,
where
![]() $t^*$
is the unique root of the equation
$t^*$
is the unique root of the equation
![]() $P(f|_\Lambda , \Psi _f(t))=0$
.
$P(f|_\Lambda , \Psi _f(t))=0$
.
Proof. Denote
![]() $P(t)=P(f|_\Lambda , \Psi _f(t))$
. For each
$P(t)=P(f|_\Lambda , \Psi _f(t))$
. For each
![]() $t>t_*$
,
$t>t_*$
,
Fix such a number t and take
![]() $\varepsilon>0$
with
$\varepsilon>0$
with
![]() $P(t)+\varepsilon <0$
. Then there exists
$P(t)+\varepsilon <0$
. Then there exists
![]() $N_1\in \mathbb {N}$
such that for every
$N_1\in \mathbb {N}$
such that for every
![]() $n\geq N_1$
, we obtain
$n\geq N_1$
, we obtain
Fix an integer
![]() $L\geq N_1$
such that
$L\geq N_1$
such that
For each
![]() $x\in \Lambda $
, set
$x\in \Lambda $
, set
![]() $Z=\Lambda \cap W^u_{\mathrm {loc}}(x,f)$
. By [Reference Climenhaga, Pesin and Zelerowicz11, Proposition 5.4], one has that
$Z=\Lambda \cap W^u_{\mathrm {loc}}(x,f)$
. By [Reference Climenhaga, Pesin and Zelerowicz11, Proposition 5.4], one has that
Thus, there is
![]() $\delta _1>0$
such that for every
$\delta _1>0$
such that for every
![]() $0<\delta <\delta _1$
, one has
$0<\delta <\delta _1$
, one has
Hence, one has that
For each
![]() $\xi>0$
, there exists
$\xi>0$
, there exists
![]() $N\in \mathbb {N}$
and a cover
$N\in \mathbb {N}$
and a cover
![]() $\{B^u_{n_i}(x_i, \delta , f^L)\}$
of Z with
$\{B^u_{n_i}(x_i, \delta , f^L)\}$
of Z with
![]() $x_i\in \Lambda $
,
$x_i\in \Lambda $
,
![]() $n_i\geq N$
such that
$n_i\geq N$
such that
 $$ \begin{align*} \xi &\geq \sum_i\exp\Big[-(P(t)+\varepsilon)Ln_i + \sup_{y\in B^u_{n_i}(x_i, \delta, f^L)}-S_{n_i}\psi^t(y, f^L)\Big]. \\ &\geq e^{-NL(P(t)+\varepsilon)} \sum_i \exp \Big[\sup_{y\in B^u_{n_i}(x_i, \delta, f^L)}-S_{n_i}\psi^t(y, f^L)\Big]. \end{align*} $$
$$ \begin{align*} \xi &\geq \sum_i\exp\Big[-(P(t)+\varepsilon)Ln_i + \sup_{y\in B^u_{n_i}(x_i, \delta, f^L)}-S_{n_i}\psi^t(y, f^L)\Big]. \\ &\geq e^{-NL(P(t)+\varepsilon)} \sum_i \exp \Big[\sup_{y\in B^u_{n_i}(x_i, \delta, f^L)}-S_{n_i}\psi^t(y, f^L)\Big]. \end{align*} $$
Note that
![]() $d^u(f^Lx, f^Ly)<\delta $
implies
$d^u(f^Lx, f^Ly)<\delta $
implies
![]() $d^u(f^ix, f^iy)<\delta $
for
$d^u(f^ix, f^iy)<\delta $
for
![]() $i=0, 1,\ldots , L-1$
, since f is expanding along the unstable manifold. This implies that
$i=0, 1,\ldots , L-1$
, since f is expanding along the unstable manifold. This implies that
![]() $ B^u_{(n_i-1)L+1}(x_i,\delta ) = B^u_{n_i}(x_i, \delta , f^L) $
for every i. Since
$ B^u_{(n_i-1)L+1}(x_i,\delta ) = B^u_{n_i}(x_i, \delta , f^L) $
for every i. Since
 $$ \begin{align*} -S_{n_i}\psi^t(y, f^L)&=-\psi^t(y, f^L)-\psi^t(f^Ly,f^L)-\cdots -\psi^t(f^{(n_i-1)L}y, f^L)\\ &\geq -\psi^t(y,f^{(n_i-1)L}) +C_1\\ &= -\psi^t(y,f^{(n_i-1)L}) - \psi^t(f^{(n_i-1)L}y,f)+\psi^t(f^{(n_i-1)L}y,f)+C_1\\ &\geq -\psi^t(y,f^{(n_i-1)L+1})+ C_1+C_2, \end{align*} $$
$$ \begin{align*} -S_{n_i}\psi^t(y, f^L)&=-\psi^t(y, f^L)-\psi^t(f^Ly,f^L)-\cdots -\psi^t(f^{(n_i-1)L}y, f^L)\\ &\geq -\psi^t(y,f^{(n_i-1)L}) +C_1\\ &= -\psi^t(y,f^{(n_i-1)L}) - \psi^t(f^{(n_i-1)L}y,f)+\psi^t(f^{(n_i-1)L}y,f)+C_1\\ &\geq -\psi^t(y,f^{(n_i-1)L+1})+ C_1+C_2, \end{align*} $$
where
![]() $C_1=\min _{x\in M}\{-\psi ^t(x,f^L)\}$
and
$C_1=\min _{x\in M}\{-\psi ^t(x,f^L)\}$
and
![]() $C_2=\min _{x\in M}\psi ^t(x,f)$
, we have that
$C_2=\min _{x\in M}\psi ^t(x,f)$
, we have that
 $$ \begin{align*} \xi &\geq e^{-NL(P(t)+\varepsilon)}e^{C_1+C_2} \sum_i \exp\Big[\sup_{y\in B^u_{(n_i-1)L+1}(x_i,\delta) }-\psi^t(y,f^{(n_i-1)L+1}) \Big] \\ &\geq e^{-NL(P(t)+\varepsilon)}e^{C_1+C_2} \inf \sum_i\exp \Big[\sup_{y\in B^u_{m_i}(x_i,\delta)} -\psi^t(y, f^{m_i})\Big] \end{align*} $$
$$ \begin{align*} \xi &\geq e^{-NL(P(t)+\varepsilon)}e^{C_1+C_2} \sum_i \exp\Big[\sup_{y\in B^u_{(n_i-1)L+1}(x_i,\delta) }-\psi^t(y,f^{(n_i-1)L+1}) \Big] \\ &\geq e^{-NL(P(t)+\varepsilon)}e^{C_1+C_2} \inf \sum_i\exp \Big[\sup_{y\in B^u_{m_i}(x_i,\delta)} -\psi^t(y, f^{m_i})\Big] \end{align*} $$
and
 $$ \begin{align*} \inf \sum_i\exp \Big[\sup_{y\in B^u_{m_i}(x_i,\delta)} -\psi^t(y, f^{m_i})\Big]\leq \xi e^{NL(P(t)+\varepsilon)}e^{-C_1-C_2}, \end{align*} $$
$$ \begin{align*} \inf \sum_i\exp \Big[\sup_{y\in B^u_{m_i}(x_i,\delta)} -\psi^t(y, f^{m_i})\Big]\leq \xi e^{NL(P(t)+\varepsilon)}e^{-C_1-C_2}, \end{align*} $$
where the infimum is taken over all collections
![]() $\{B^u_{m_i}(x_i, \delta )\}$
with
$\{B^u_{m_i}(x_i, \delta )\}$
with
![]() $x_i\in \Lambda $
,
$x_i\in \Lambda $
,
![]() $m_i\geq (N-1)L$
which cover Z. Letting
$m_i\geq (N-1)L$
which cover Z. Letting
![]() $N\to \infty $
, we obtain
$N\to \infty $
, we obtain
for every
![]() $t>t_*$
. This yields that
$t>t_*$
. This yields that
Remark 3.1. Let
![]() $f: M\to M$
be a
$f: M\to M$
be a
![]() $C^{1+\alpha }$
diffeomorphism on an
$C^{1+\alpha }$
diffeomorphism on an
![]() $m_0$
-dimensional compact Riemannian manifold M and
$m_0$
-dimensional compact Riemannian manifold M and
![]() $\Lambda \subset M$
be a hyperbolic set. Assume that
$\Lambda \subset M$
be a hyperbolic set. Assume that
![]() $f|_{\Lambda }$
is topologically transitive. Then for every
$f|_{\Lambda }$
is topologically transitive. Then for every
![]() $x\in \Lambda $
,
$x\in \Lambda $
,
where
![]() $t^{\Phi _f}_u$
,
$t^{\Phi _f}_u$
,
![]() $t^{\Psi _f}_u$
are the unique roots of the equations
$t^{\Psi _f}_u$
are the unique roots of the equations
respectively. The proof is a slight modification of Lemmas 3.3 and 3.4. See [Reference Cao, Wang and Zhao9] for more details about the Carathéodory singular dimension of each subset of a repeller. However, we do not know whether
![]() $P_{\Lambda \cap W^u(x,f)}(f, \Phi _f(t))=P_\Lambda (f, \Phi _f(t))$
and
$P_{\Lambda \cap W^u(x,f)}(f, \Phi _f(t))=P_\Lambda (f, \Phi _f(t))$
and
![]() $P_{\Lambda \cap W^u(x,f)}(f, \Psi _f(t))=P_\Lambda (f, \Psi _f(t))$
hold.
$P_{\Lambda \cap W^u(x,f)}(f, \Psi _f(t))=P_\Lambda (f, \Psi _f(t))$
hold.
Proof of Theorem C(ii)
By Lemma 3.4, we have that
where
![]() $t_{\varepsilon }^*$
is the unique root of the equation
$t_{\varepsilon }^*$
is the unique root of the equation
![]() $P(f|_{\Lambda _\varepsilon }, \Psi _f(t))=0$
. Take
$P(f|_{\Lambda _\varepsilon }, \Psi _f(t))=0$
. Take
![]() $\nu \in \mathcal {M}_{\mathrm {inv}}(f|_{\Lambda _\varepsilon })$
such that
$\nu \in \mathcal {M}_{\mathrm {inv}}(f|_{\Lambda _\varepsilon })$
such that
![]() $h_\nu (f|_{\Lambda _\varepsilon })=h_{\text {top}}(f|_{\Lambda _\varepsilon })$
, by properties (b) and (d) in Theorem 2.4, it holds that
$h_\nu (f|_{\Lambda _\varepsilon })=h_{\text {top}}(f|_{\Lambda _\varepsilon })$
, by properties (b) and (d) in Theorem 2.4, it holds that

where
![]() $\unicode{x3bb} _1(\nu )\geq \unicode{x3bb} _2(\nu ) \geq \cdots \geq \unicode{x3bb} _{m_0}(\nu )$
are the Lyapunov exponents of
$\unicode{x3bb} _1(\nu )\geq \unicode{x3bb} _2(\nu ) \geq \cdots \geq \unicode{x3bb} _{m_0}(\nu )$
are the Lyapunov exponents of
![]() $\nu $
. Similarly, let
$\nu $
. Similarly, let
![]() ${\tau \in \mathcal {M}_{\mathrm {inv}}(f|_{\Lambda _\varepsilon })}$
be an equilibrium state of
${\tau \in \mathcal {M}_{\mathrm {inv}}(f|_{\Lambda _\varepsilon })}$
be an equilibrium state of
![]() $P(f|_{\Lambda _\varepsilon }, \Psi _f(t_{\varepsilon }^*))$
, then one has that
$P(f|_{\Lambda _\varepsilon }, \Psi _f(t_{\varepsilon }^*))$
, then one has that

This together with equation (16) yield that
Hence, we have that
This implies that
![]() ${\lim _{\varepsilon \to 0}t_{\varepsilon }^*=t_{u}^*}$
, where
${\lim _{\varepsilon \to 0}t_{\varepsilon }^*=t_{u}^*}$
, where
![]() $t_{u}^*$
is the unique root of
$t_{u}^*$
is the unique root of
![]() $P_\mu (f, \Psi _f(t))=0$
. Consequently, we have that
$P_\mu (f, \Psi _f(t))=0$
. Consequently, we have that
Finally, to complete the proof of Theorem C, assume that
![]() $\mu $
is an SRB measure from now on, then
$\mu $
is an SRB measure from now on, then
![]() $h_\mu (f)=\unicode{x3bb} _1(\mu )+\unicode{x3bb} _2(\mu )+\cdots +\unicode{x3bb} _u(\mu )$
. Thus,
$h_\mu (f)=\unicode{x3bb} _1(\mu )+\unicode{x3bb} _2(\mu )+\cdots +\unicode{x3bb} _u(\mu )$
. Thus,
![]() $P_\mu (f, \Phi _f(u))=0$
. Since the Carathéodory singular dimension with respect to
$P_\mu (f, \Phi _f(u))=0$
. Since the Carathéodory singular dimension with respect to
![]() $\Psi _f$
is always less than u, by property (i) of Theorem C, we have that
$\Psi _f$
is always less than u, by property (i) of Theorem C, we have that
If
![]() $\unicode{x3bb} _1(\mu )+\unicode{x3bb} _2(\mu )+\cdots +\unicode{x3bb} _{m_0}(\mu )\geq 0$
, then
$\unicode{x3bb} _1(\mu )+\unicode{x3bb} _2(\mu )+\cdots +\unicode{x3bb} _{m_0}(\mu )\geq 0$
, then
![]() $P_\mu (f, \Xi _f(m_0-u))\ge 0$
since
$P_\mu (f, \Xi _f(m_0-u))\ge 0$
since
![]() $\mu $
is an SRB measure for f. Consider
$\mu $
is an SRB measure for f. Consider
![]() $f^{-1}$
, by Margulis–Ruelle inequality, we have that
$f^{-1}$
, by Margulis–Ruelle inequality, we have that
which implies that
![]() $P_\mu (f, \Xi _f(m_0-u))\le 0$
. Hence, we have that
$P_\mu (f, \Xi _f(m_0-u))\le 0$
. Hence, we have that
Thus, we have that
![]() $t_s^*=m_0-u$
. By the definition of Lyapunov dimension, we have that
$t_s^*=m_0-u$
. By the definition of Lyapunov dimension, we have that
![]() $\dim _L\mu =m_0=u+t_s^*$
.
$\dim _L\mu =m_0=u+t_s^*$
.
Now, we assume that
![]() $\unicode{x3bb} _1(\mu )+\unicode{x3bb} _2(\mu )+\cdots +\unicode{x3bb} _{m_0}(\mu )< 0$
and let
$\unicode{x3bb} _1(\mu )+\unicode{x3bb} _2(\mu )+\cdots +\unicode{x3bb} _{m_0}(\mu )< 0$
and let
![]() $\ell $
be the largest integer such that
$\ell $
be the largest integer such that
![]() $\unicode{x3bb} _1(\mu )+\unicode{x3bb} _2(\mu )+\cdots +\unicode{x3bb} _{\ell }(\mu )\geq 0$
. By a standard computation, one can show that
$\unicode{x3bb} _1(\mu )+\unicode{x3bb} _2(\mu )+\cdots +\unicode{x3bb} _{\ell }(\mu )\geq 0$
. By a standard computation, one can show that
Combining with
one has
This completes the proof of Theorem C.
Acknowledgements
This work is partially supported by The National Key Research and Development Program of China (2022YFA1005802). Y.C. is partially supported by NSFC (12371194), Y.Z. is partially supported by NSFC (12271386) and Qinglan project of Jiangsu Province.