Hostname: page-component-cd9895bd7-gxg78 Total loading time: 0 Render date: 2024-12-25T19:14:01.510Z Has data issue: false hasContentIssue false
  • English
  • Français

On the Lack of Inverses to C*-Extensions Related to Property T Groups

Published online by Cambridge University Press:  20 November 2018

V. Manuilov  and
K. Thomsen
V. Manuilov
Affiliation:
Department of Mechanics and Mathematics, Moscow State University, Leninskie Gory, Moscow 119992, Russia e-mail: manuilov@mech.math.msu.su
K. Thomsen
Affiliation:
IMF, Department of Mathematics, Ny Munkegade, 8000 Aarhus C, Denmark e-mail: matkt@imf.au.dk
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Using ideas of S. Wassermann on non-exact 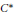${{C}^{*}}$-algebras and property 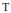$\text{T}$ groups, we show that one of his examples of non-invertible 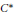${{C}^{*}}$-extensions is not semi-invertible. To prove this, we show that a certain element vanishes in the asymptotic tensor product. We also show that a modification of the example gives a 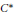${{C}^{*}}$-extension which is not even invertible up to homotopy.

Keywords

19K3346L0646L8020F99C*-algebra extensionproperty T groupasymptotic tensorC*-normhomotopy
Type
Research Article
Information
Canadian Mathematical Bulletin , Volume 50 , Issue 2 , 01 June 2007 , pp. 268 - 283
Copyright
Copyright © Canadian Mathematical Society 2007

References

[1] Anderson, J., A C*-algebra for which Ext() is not a group. Ann. of Math. 107(1978), no. 3, 455–458.Google Scholar
[2] Brown, L. G., Douglas, R. G., and Fillmore, P. A., Extensions of C*-algebras and K-homology. Ann. of Math. 105(1977), no. 2, 265–324.Google Scholar
[3] Connes, A. and Higson, N., Déformations, morphismes asymptotiques et K-théorie bivariante. C. R. Acad. Sci. Paris Sér. I Math. 311(1990), no. 2, 101–106.Google Scholar
[4] Dadarlat, M. and Eilers, S., On the classification of nuclear C*-algebras. Proc. London Math. Soc. (3) 85(2002), no. 1, 168–210.Google Scholar
[5] Effros, E. G., Dimensions and C*-Algebras, CBMS Regional Conference Series 46, American Mathematical Society, Providence, RI, 1981.Google Scholar
[6] Haagerup, U. and Thorbjornsen, S., A new application of random matrices: Ext is not a group. Ann. of Math. 162(2005), no. 2, 711–775.Google Scholar
[7] de la Harpe, P., Robertson, A. G., and Valette, A., On the spectrum of the sum of generators for a finitely generated group. Israel J. Math. 81(1993), no. 1–2, 65–96.Google Scholar
[8] de la Harpe, P. and Valette, A., La propriété (T) de Kazhdan pour les groupes localement compacts. Astérisque, 175.Google Scholar
[9] Kirchberg, E., On non semi-split extensions, tensor products and exactness of C*-algebras. Invent. Math. 112(1993), 449–489.Google Scholar
[10] Manuilov, V. and Thomsen, K., Asymptotically split extensions and E-theory. Algebra i Analiz 12(2000) no. 5, 142–157 (in Russian); English translation: St. Petersburg Math. J. 12(2001), no. 5, 819–830.Google Scholar
[11] Manuilov, V. and Thomsen, K., The Connes–Higson construction is an isomorphism. J. Funct. Anal. 213(2004), no. 1, 154–175.Google Scholar
[12] Manuilov, V. and Thomsen, K., On the asymptotic tensor C*-norm. Arch. Math. 86(2006), no. 2, 138–144.Google Scholar
[13] Ozawa, N., An application of expanders to (l1) ⊗ (l2). J. Funct. Anal. 198(2003), no. 2, 499–510.Google Scholar
[14] Valette, A., Minimal projections, integrable representations and property T . Arch. Math. 43(1984), no. 5, 397–406.Google Scholar
[15] Voiculescu, D., A non-commutative Weyl–von Neumann theorem. Rev. Roumaine Math. Pures. Appl. 21(1976), no. 1, 97–113.Google Scholar
[16] Wang, P. S., On isolated points in the dual space of locally compact groups. Math. Ann. 218(1975), no. 1, 19–34.Google Scholar
[17] Wassermann, S., Liftings in C*-algebras: a counterexample. Bull. London Math. Soc. 9(1976), no. 2, 201–202.Google Scholar
[18] Wassermann, S., Tensor products of free group C*-algebras. Bull. London Math. Soc. 22(1990), no. 4, 375–380.Google Scholar
[19] Wassermann, S., C*-algebras associated with groups with Kazhdan's property T.. Ann. of Math. 134(1991), no. 2, 423–431.Google Scholar
[20] Wassermann, S., Exact C*-Algebras and Related Topics. Lecture Notes Series 19, Seoul National University, Research Institute of Mathematics, Global Analysis Research Center, Seoul, 1994 Google Scholar