Thermodynamics and crystal structures of krautite, Mn[AsO₃(OH)]⋅H₂O, koritnigite, Zn[AsO₃(OH)]⋅H₂O and cobaltkoritnigite, Co[AsO₃(OH)]⋅H₂O

Abstract

Synthetic samples of krautite, Mn[AsO₃(OH)]⋅H₂O, koritnigite, Zn[AsO₃(OH)]⋅H₂O and cobaltkoritnigite, Co[AsO₃(OH)]⋅H₂O, were used for calorimetric experiments. For krautite and koritnigite, single-crystal X-ray diffraction was used to determine positions of all atoms, including the H atoms. These data allowed the hydrogen-bond network and the function of H₂O molecules in these structures to be determined. The structural formulae are Mn₄(H₂^[3]O)₄[AsO₃(OH)]₄ and Zn₄(H₂^[3]O)₂[AsO₃(OH)]₄(H₂^[4]O)₂, where ^[3]H₂O and ^[4]H₂O are the ‘transformer’ and ‘non-transformer’ H₂O groups, respectively. Even though the principal features of these structures are identical, the details, especially those regarding the H₂O groups, differ from one structure to another structure in this group. The solubility products (log K_sp) were determined from calorimetric data, that is, from the experimentally measured enthalpies of formation and entropies. They relate to the reaction M[AsO₃(OH)]⋅H₂O → M²⁺ + HAsO₄^2– + H₂O and are –6.10 for krautite, –6.88 for koritnigite and –6.83 for cobaltkoritnigite. We also estimated the log K_sp for magnesiokoritnigite as –2.0. Calculation of phase diagrams shows that all these phases originate under acidic conditions from solutions with high metal and arsenate concentration. They are restricted to local environments, to pockets that maintain such high concentrations over the time necessary for crystallisation of the krautite-group phases.

Introduction

Natural arsenates constitute a large, chemically and structurally variable group of minerals (Drahota and Filippi, 2009; Majzlan et al., 2014). They can accommodate many divalent metals in various structural types. The vivianite-group arsenates, for example, have the general formula M ₃(AsO₄)₂⋅8H₂O, where M = Ni, Co, Zn, Mg, Mn, Fe and Cu (Sejkora et al., 2014; Plášil et al., 2017; Ciesielczuk et al., 2018; Siuda and Macioch, 2018; Dumańska-Słowik et al., 2018). Natural members of this group are mostly Ni or Co rich. The olivenite group with the general formula M ₂(AsO₄)OH includes members with M = Cu, Zn or Mn, although only the Cu- and Zn-rich compositions are more common in Nature (Southwood et al., 2020). Minerals of the roselite and fairfieldite group with the general formula Ca₂M(AsO₄)₂⋅2H₂O are less common and include members with M = Co, Mn, Zn, Cu, Ni and Mg (Fleck et al., 2002). The krautite group, the subject of this work, hosts M(AsO₃OH)⋅H₂O minerals with M = Mn, Zn, Co, Mg and Ca. The group is not structurally homogeneous. The structures contain heteropoly-hedral sheets with similar, but not identical topology. The sheets must accommodate the coordination requirements of the different divalent cations.

The selection of the phases and their metals is controlled by a combination of geochemical parameters (composition of the primary minerals), physico-chemical properties of the aqueous fluids (e.g. pH) and thermodynamics of the solids. The relative importance of such variables can be judged by detailed investigations of mineral-rich, classical localities, such as Jáchymov in the Czech Republic (Škácha et al., 2019), associated solutions (e.g. Majzlan et al., 2020) and thermodynamic measurements. Zinc and cobalt for such minerals are released by weathering of primary ore minerals. Manganese, on the other hand, could be released from minerals of ore deposits (Holtstam et al., 2022; Cámara et al., 2023) but could have other sources in the environment (e.g. Gatuingt et al., 2021).

This work focuses on thermodynamic properties and crystal structures on selected synthetic equivalents of the minerals in the krautite group. Enthalpies of formation and entropies were measured by acid-solution and relaxation calorimetry, respectively. The data set was augmented by previously acquired data for some of the krautite-group phases (Plumhoff et al., 2020; Majzlan et al., 2020) and estimates for some phases. Phase diagrams document the stability fields of these minerals and their formation conditions. Single-crystal X-ray diffraction experiments were used to determine the position of all atoms (including the H atoms) in these structures and to understand hydrogen bonding schemes in these phases.

Materials

Synthetic krautite was prepared by a method outlined in Buckley et al. (1990), specified already by Catti and Franchini-Angela (1979) and Deiss (1914). One solution was prepared by mixing and dissolving 4.5 g KH₂AsO₄ in 20 mL water. Another solution was prepared with 4.95 g MnCl₂⋅4H₂O and 20 mL water. The arsenical solution was heated slightly to dissolve the solid completely. The two solutions were mixed rapidly and turned immediately to a thick gel. Aggregates of pink krautite crystals grew in the gel. After two months, parts of the gel started to turn to liquid and released some of the aggregates (Fig. 1a). They were picked up with tweezers, dried with a paper towel and examined under binocular microscope for morphology or colour that deviate from that of krautite.

Fig. 1. Aggregates of synthetic (a) krautite, (b) koritnigite and (c) cobaltkoritnigite.

Koritnigite was synthesised by the same procedure, taking 3.41 g ZnCl₂ instead of the manganese chloride. Again, thick gel formed and turned slowly to liquid. In this case, even after one year, more than half of the volume still consisted of gel, however a sufficient number of crystals (Fig. 1b) were available for all analyses.

A similar synthesis was attempted with CoCl₂⋅6H₂O but the gel remained thick and essentially unchanged for more than one year. No crystals were seen in the purple, compact gel. Therefore, cobaltkoritnigite was prepared by the procedure described by Zettler et al. (1979). As specified therein, 0.33 g As₂O₅ and 1.4 g CoSO₄⋅7H₂O were mixed with 5 mL water and maintained in a Teflon-lined pressure bomb at 150°C for six days. Afterwards, the bomb was allowed to cool slowly to room temperature and the crystals (Fig. 1c) were filtered from the remaining liquid.

Methods

Selected single crystals were investigated by a Rigaku SuperNova single-crystal diffractometer equipped with the Atlas S2 detector and using the mirror-monochromatised MoKα radiation (λ = 0.71073 Å) from a micro-focus X-ray tube. Correction for background, Lorentz effect and polarisation, as well as the empirical (multi-scan) absorption correction were applied to the data during reduction in the CrysAlis package (Rigaku v.2018). The structure has been solved independently from previous structure investigations (Catti and Franchini-Angela, 1979; Keller et al., 1980) using an intrinsic-phasing algorithm of the program SHELXT (Sheldrick, 2015) and subsequently treated by the least-squares refinement in Jana2020 (Petříček et al., 2020).

The aggregates of crystals used for calorimetry were ground to powder and tested for their purity with a Bruker D8 ADVANCE powder X-ray diffractometer with DAVINCI design, and with CuKα radiation, Ni filter and a Lynxeye 1D detector. A step size of 0.02°2θ and a 0.25 s per step were used. Lattice parameters were refined using JANA2006 (Petříček et al., 2014).

Acid-solution calorimetry and relaxation calorimetry were used to measure enthalpies of dissolution in 5 N HCl and low-temperature heat capacities, respectively. Both methods were used in an identical fashion as in our previous work (e.g. Majzlan et al., 2020). The details on acid-solution calorimetry of secondary minerals are summarised by Majzlan (2017). The principles of relaxation calorimetry, the accuracy and precision of the method have been described and evaluated by Dachs and Benisek (2011) and Kennedy et al. (2007).

Results

Crystal structure of koritnigite

A prismatic large, 0.140 × 0.099 × 0.023 mm, fragment of synthetic koritnigite crystal (also used for calorimetric study) was investigated. The structure solution revealed nearly all atomic positions except those of the H atoms. Those were localised subsequently from the difference-Fourier maps and refined keeping soft constraints of 1.00 Å on the O–H distances as well as H–O–H angles, 105° within the H₂O molecules, and with the U _iso of each H set to 1.2 times that of the donor O atom. The final refinement including 265 parameters, 12 restraints and 12 constraints, converged to R = 0.0270 and wR = 0.0663 for 3330 unique observed reflections, of I > 3σ(I), with goodness of fit = 1.37. The structure was modelled as twinned due to reticular merohedry (see Petříček et al., 2016) as a two-component twin (Table 1). Crystallographic details, data collection and refinement parameters are given in Table 1. The bond-valence analysis (after Brown 2002) is provided in Supplementary Table S1, based on the refined interatomic distances (Supplementary Table S2) and the bond-valence parameters given by Gagné and Hawthorne (2015). Hydrogen-bond geometry is summarised in Supplementary Table S3. The crystallographic information file has been deposited with the Principal Editor of Mineralogical Magazine and is available as Supplementary material (see below).

Table 1. Crystal data, collection and refinement parameters for synthetic koritnigite and krautite.

Crystal structure of krautite

A short-prismatic, 0.093 × 0.054 × 0.007 mm, fragment of synthetic krautite crystal (also used for calorimetric study) was investigated. The structure solution revealed nearly all atomic positions except those of the H atoms. The structure is non-centrosymmetric, of the space-group P2₁; the absolute structure has been refined (as an inversion-twin). Afterwards, the positions of all H atoms have been successfully localised from the difference-Fourier maps corrected for the F ² from the second twin-domain contribution (Petříček et al., 2016). H atoms were refined keeping soft constraints of 1.00 Å on the O–H distances as well as H–O–H angles, 105° within the H₂O molecules, and with the U _iso of each H set to 1.2 times that of the donor O atom. The final refinement including 284 parameters, 15 restraints and 19 constraints, converged to R = 0.0338 and wR = 0.0666 for 3895 unique observed reflections, of I > 3σ(I ), with goodness of fit = 1.24. Crystallographic details, data collection and refinement parameters are given in Table 1. The bond-valence analysis (after Brown, 2002) is provided in Supplementary Table S4, based on the refined interatomic distances (Supplementary Table S5) and the bond-valence parameters given by Gagné and Hawthorne (2015). The hydrogen-bond geometry is summarised in Supplementary Table S6. The crystallographic information file and the structure factors list have been deposited with the Principal Editor of Mineralogical Magazine and are available as Supplementary material (see below).

Thermodynamic measurements

Apart from the title compounds, additional phases must be measured in order to convert the dissolution enthalpies to enthalpies of formation. These additional phases, so-called reference phases, were ZnO, MnO, CoSO₄⋅7H₂O, KH₂AsO₄, HCl⋅9.96H₂O, H₂O and KCl. The enthalpies of dissolution of all these phases in 5 N HCl are given in Table 2, together with an appropriate thermochemical cycle. The calculated enthalpies of formation are listed in Table 3.

Table 2. Thermochemical cycle and the enthalpies of the individual reactions used in this work. The bottom portion of the table lists the equations needed for the calculation of formation enthalpies of the arsenates of the krautite group.

Table 3. Summary of the thermodynamic data for the minerals of the krautite group.

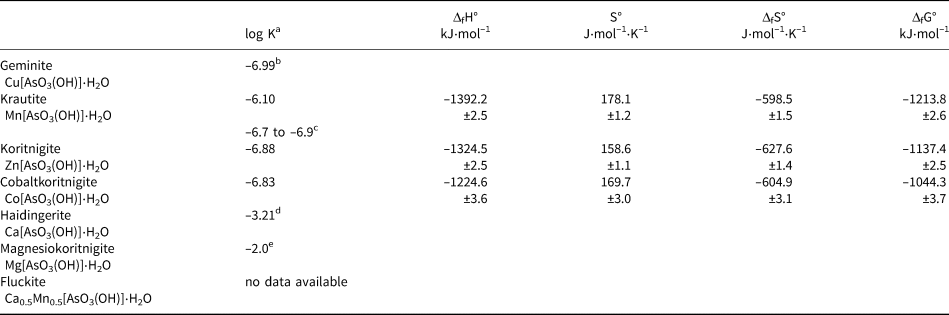
^a All log K _sp values refer to a dissolution reaction M[AsO₃(OH)]⋅H₂O → M ²⁺ + HAsO₄^2– + H₂O

^b Plumhoff et al. (2020)

^c calculated from Tournassat et al. (2002)

^d Majzlan et al. (2020)

^e estimated, see text

The low-temperature heat capacities (Fig. 2a) were measured and integrated for koritnigite (Supplementary Tables S7, S8) and krautite (Supplementary Tables S9, S10). Thermodynamic functions calculated from the smoothed C _p data are presented in Supplementary Tables S8 and S10. Both data sets show anomalies at very low temperatures. In the data for koritnigite, there is a very weak, broad C _p anomaly at 3 K. The nature of this anomaly is unclear but its contribution to overall C _p and S is negligible.

Fig. 2. Experimentally measured heat capacity of koritnigite and krautite. The panel (a) shows the full data sets and (b) shows a detail at low temperatures.

In the data for krautite, there is a sharp lambda-shaped anomaly at 3 K (Fig. 2b). This anomaly can be assigned to the magnetic ordering below this temperature. The position of the anomaly is in excellent agreement with magnetic-susceptibility data of Bramwel et al. (1988). They determined that synthetic krautite is antiferromagnetic, with a Curie temperature of 3.2 K.

The presence of the anomalies complicated the fitting necessary to extract entropies for the two phases. There are no appropriate functions for the fitting of the lambda-shaped anomalies (e.g. Shapiro et al., 1999). Both data sets were fitted with a series of polynomials that have no physical meaning. The polynomials were joined at temperatures where the differences in smoothed C _p and their slopes were minimal. These polynomial segments were then used to calculate thermodynamic functions between 0 and 300 K and the results are listed in Tables S8 and S10.

The calculated entropies were converted to entropies of formation, using the entropies of pure elements in their standard state from Robie and Hemingway (1995). Enthalpies and entropies of formation were then used to calculate the Gibbs free energies of formation (Table 3) and the solubility products, using auxiliary data from Robie and Hemingway (1995) and Nordstrom et al. (2014).

Entropy estimate for cobaltkoritnigite

In the absence of low-temperature heat capacity data for Co[AsO₃(OH)]⋅H₂O, its entropy can be estimated from solid-state reactions:

$$\!\!M^{\prime}[ {{\rm As}{\rm O}_3( {{\rm OH}} ) } ] \cdot {\rm H}_2{\rm O} + M^{\prime\prime}{\rm O} = M^{\prime\prime}[ {{\rm As}{\rm O}_3( {{\rm OH}} ) } ] \cdot {\rm H}_2{\rm O\ } + M^{\prime}{\rm O},$$

where M′ and M″ are two different divalent metals. We have chosen oxides MO because of their simple structures and accurately known entropies. Because all phases that participate in this reaction are solids, it could be assumed that the entropy change associated with this reaction (Δ_rS) is 0 J⋅mol^–1⋅K^–1. This assumption can be tested for M' = Zn and M'' = Mn with the data from this work and entropies for simple oxides from Robie and Hemingway (1995). The calculated Δ_rS_Zn–Mn = 3.0±1.7 J⋅mol^–1⋅K^–1, a small entropy value. The reason for the small difference could be a different structural type for MnO (halite) and ZnO (wurtzite). For the estimate for cobaltkoritnigite, pairs of MnO–CoO (both halite structures) and ZnO–CoO (different structure types) can be tested. Assuming that Δ_rS_Zn–Co and Δ_rS_Mn–Co are both 0 J⋅mol^–1⋅K^–1, the entropy of Co[AsO₃(OH)]⋅H₂O can be estimated as 168.2 and 171.2 J⋅mol^–1⋅K^–1, respectively. Hence, even though there is a structural difference between ZnO and CoO, the entropy estimate is similar to that obtained from the calculation with the MnO–CoO pair. We adopted the average value 169.7 J⋅mol^–1⋅K^–1, with a conservative error estimate of ±3.0 J⋅mol^–1⋅K^–1.

Discussion

Role of H₂O in the crystal structures of solids

To analyse and evaluate the role of molecular water in the structures of solids, a useful scheme was introduced in the literature by Frank Hawthorne and co-workers (Hawthorne, 1992, 2012; Hawthorne and Schindler, 2008; Hawthorne and Sokolova, 2012; Schindler and Hawthorne, 2008). Generally, there are several types of H₂O moieties in crystal structures and each of them play a distinct role in structure bonding. Particular types of H₂O can be distinguished based on the coordination number of O atoms in these H₂O groups. In the structures of oxysalts, in general, there are ‘transformer’, ‘non-transformer’ and ‘inverse’ transformer H₂O groups with [3], [4] and [5]-fold coordinated O atoms, respectively. Their role is generally to transfer the bond-valence from cations (Lewis acids) to anions (Lewis bases), keeping the structure together, as the strengths of these components are equal or similarly matching, following the valence-matching principle of the bond-valence theory (Brown, 2002, 2009; Hawthorne 2012).

Symmetry of the structures of krautite and koritnigite

In this work, the crystal structure of krautite was refined in the monoclinic space group P2₁, in agreement with the earlier (Catti and Franchini-Angela, 1979) or newer (Weil et al., 2022) models. On the other hand, other M[AsO₃(OH)]⋅H₂O structures with M = Co, Cu, Zn and Mg are triclinic (Keller et al., 1980; Cooper and Hawthorne, 1995; Kampf et al., 2013). The most recent addition to this group is a triclinic polymorph of Mn[AsO₃(OH)]⋅H₂O (Weil et al., 2022), not known from Nature so far. The differences between the monoclinic and triclinic structures in this group of phases are subtle and seem to be related to the hydrogen-bonding scheme in these structures. Our refinements confirmed the choice of symmetry for krautite and koritnigite. There were no indications that the symmetry should be changed.

Crystal structure and hydrogen-bonding network of koritnigite

The structure of koritnigite has been determined first by Keller et al. (1980). It contains four Zn sites, four As sites, twenty O sites and twelve H sites. In the general view, the structure consists of zig-zag edge-sharing chains of ZnO₅(H₂O) octahedra that run parallel to [10$\bar{1}$]. They are joined by corner-sharing with AsO₃(OH) tetrahedra to form sheets parallel to {010} (Fig. 3). Two of the four vertices of each of the AsO₃(OH) tetrahedra are O atoms of Zn-octahedra, while the other two are unlinked, except by hydrogen bonding. The sheets are linked in the [010] direction only by hydrogen bonds (Fig. 3). The Zn1 and Zn4 octahedra form one set of chains linked into a sheet centred at 0 along b and the Zn2 and Zn3 octahedra form another set of chains linked into a sheet centred at ½ along b.

Fig. 3. Hydrogen-bonding in the structure of koritnigite. (a) {ZnO₅(H₂O)AsO₃(OH)}^∞ sheets, stacked perpendicular to b, are linked via loose H-bonds only, whereas (b) the intrasheet H-bonding network is denser (shown is a single layer). Zn is in violet colour, As is green, blue dashed are H-bonds, H atoms are whitish. Unit-cell edges are outlined in black lines. (c) a detail of the intrasheet H-bonding network. Zn is in pale-green colour, As is green, pale-green dashed are H-bonds.

The structure of koritnigite contains four symmetrically independent OH groups and four symmetrically independent H₂O molecules. Each of those H₂O molecules participates in the bonding within the single ZnO₅(H₂O) octahedron. Those linked to Zn3 and Zn4 (O20 and O17 respectively; see Table S1) are transformer H₂O groups with the 3-coordinated O atom. On the other hand, the O15 and O11 that are linked to Zn1 and Zn2, respectively, seem to be non-transformer H₂O groups, as each of them accepts one additional weak H-bond from the interlayer. These findings are consistent with observations and predictions made by Keller et al. (1980) for koritnigite and by Kampf et al. (2013) for magnesiokoritnigite. Nevertheless, neither of these papers found H atoms from the diffraction data and were based only on the crystal-chemical considerations. The geometry of hydrogen bonds in the crystal structure of synthetic koritnigite is summarised in Supplementary Table S3. The structural formula of synthetic koritnigite is Zn₄(H₂^[3]O)₂[AsO₃(OH)]₄(H₂^[4]O)₂, Z = 2, D _calc. = 3.5956 g cm^–3.

Crystal structure and hydrogen-bonding network of krautite

The structure of krautite has been first determined by Catti and Franchini-Angela (1979). It contains four Mn, four As and twenty O sites. Furthermore, it hosts twelve H atoms, associated with OH and H₂O, which have not been found in previous work. The structure consists of (010) layers composed of chains of Mnφ₆ octahedra running in the [101] direction (Fig. 4). These chains are interconnected by the As tetrahedra. Each of the four independent arsenate tetrahedra attaches to the octahedral chains via three oxygen atoms; the fourth oxygen is protonated, staggered towards the interlayer, and it participates in hydrogen-bonding between the layers (Fig. 4). Adjacent layers are held together only by hydrogen bonds resulting in the excellent (010) cleavage of krautite. The layers are stacked along and related by the screw 2₁ axis.

Fig. 4. Hydrogen-bonding in the structure of krautite. (a) {MnO₅(H₂O)AsO₃(OH)}^∞ sheets, stacked perpendicular to b, are linked via loose H-bonds only, whereas (b) the intrasheet H-bonding network (detail only displayed) is dense and strong. Mn is light-pink colour, As is green, blue dashed are H bonds, labels in red belong to O^2– and blue to H₂O/OH. Unit-cell edges are outlined in black lines.

The structure of krautite contains four symmetrically independent OH groups and four symmetrically independent H₂O molecules in the asymmetric part of the unit cell. Similarly to koritnigite, each of those H₂O participates in the bonding within the single MnO₅(H₂O) octahedron. Nevertheless, based on the current structure refinement it is likely that three H₂O groups within the krautite structure, O10 (Mn2), O13 (Mn3) and O20 (Mn4) (see Table S4), are transformer H₂O groups with a 3-coordinated O atom. The O7 atom seems to be a part of a non-transformer H₂O group with a fourfold coordinated O atom. The geometry of hydrogen bonds in the crystal structure of synthetic krautite is summarised in Table S6. The structural formula of synthetic krautite is Mn₄(H₂^[3]O)₃[AsO₃(OH)]₄(H₂^[4]O), Z = 2, D _calc. = 3.2739 g cm^–3.

The structural models of synthetic krautite from this work and that of Bramwell et al. (1994) are very similar. The calculated arithmetic mean (dav) of the equivalent atoms distances is 0.1331 Å. The measure of similarity (Δ) (after Bergerhoff et al., 1999) is 0.012, which is a function of the differences in atomic positions, weighted by the multiplicities of the sites, and the ratios of the corresponding lattice parameters of the structures. The maximum difference in those two structures has been found for the atom H1O10 and is 0.6609 Å. This difference is seen as reasonable as we are comparing X-ray diffraction (this work) and neutron diffraction (Bramwell et al., 1994) techniques.

Stability and formation conditions of koritnigite, krautite and related phases

The thermodynamic data acquired in this work allow for quantitative assessment of the stability of the M[AsO₃(OH)]⋅H₂O, including krautite, koritnigite and cobaltkoritnigite, and comparison of these phases to the chemically related geminite [Cu[AsO₃(OH)]⋅H₂O] and haidingerite [Ca[AsO₃(OH)]⋅H₂O]. Given the presence of the acidic AsO₃(OH) group in their structures, it is not surprising that they are predicted to crystallise from acidic solutions (Figs 5, 6). Another property, not obvious from their composition, is their relatively high solubility. Hence, in general, these phases precipitate from oxidised acidic (acid mine drainage) solutions with high metal and arsenic concentrations.

Fig. 5. pH–pɛ diagrams for the system (a) ZnO–As₂O₅–H₂O–S and (b) Cu–O₂–S–As₂O₅–H₂O. Constructed for log a[Zn(II)] or log a[Cu(II)] = –1, log a[As(V)] = –1, log a(S) = –3 and T = 298.15 K.

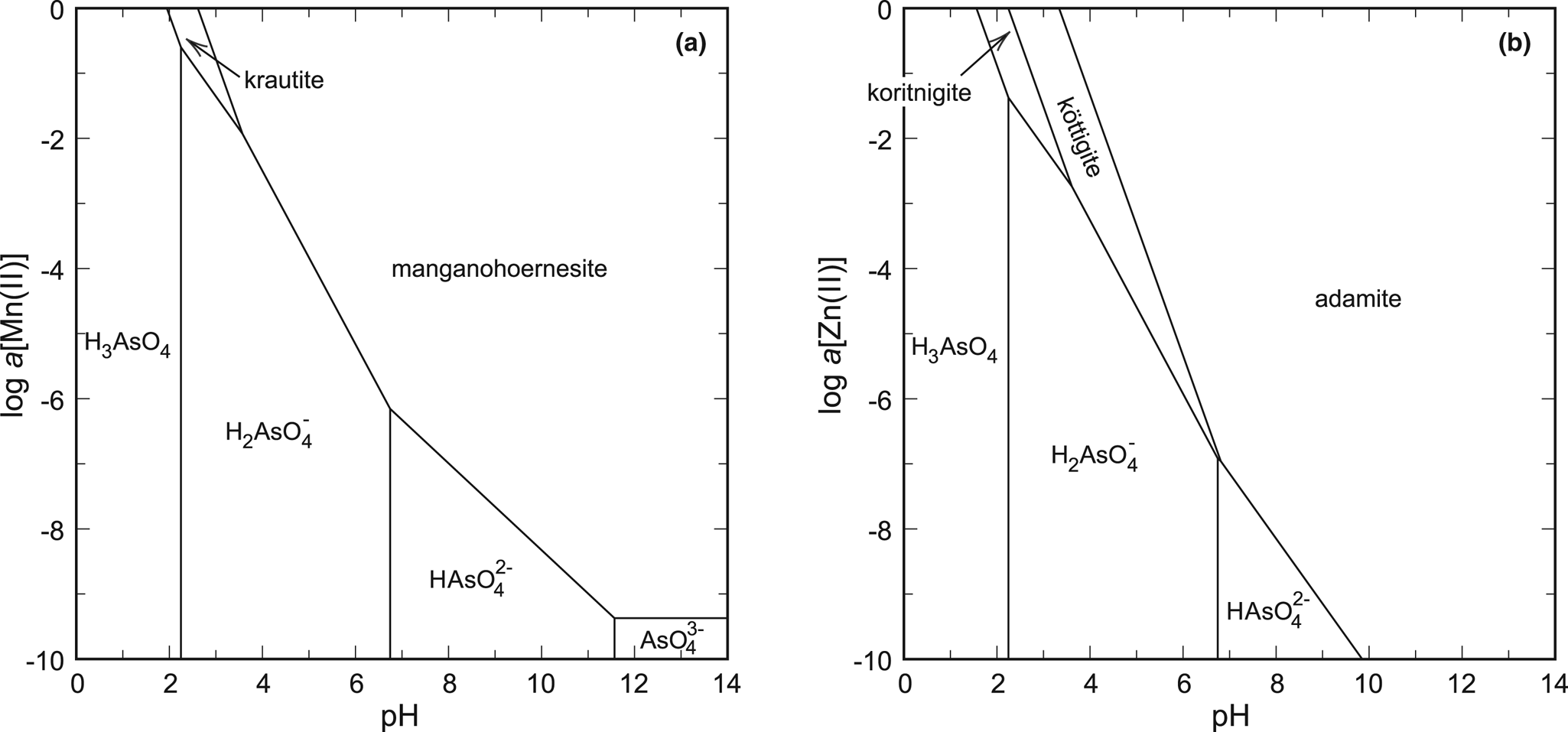
Fig. 6. pH-activity phase diagrams for the systems (a) MnO–As₂O₅–H₂O and (b) ZnO–As₂O₅–H₂O. Constructed for log a[As(V)] = –1 and T = 298.15 K.

The best example is the system ZnO–As₂O₅–H₂O, with the phases koritnigite, köttigite [vivianite group, Zn₃(AsO₄)₂⋅8H₂O] and adamite [olivenite group, Zn₂(AsO₄)(OH)]. Thermodynamic data for köttigite were taken from Lee and Nriagu (2007) and those for adamite from Magalhães et al. (1988). A phase diagram that represents only an oxidised solution (Fig. 5a, 6b) shows the relationships between the three zinc arsenates. The stability field of koritnigite is restricted to low pH values and high Zn and As concentrations. The greatest stability field belongs to adamite, a Zn arsenate that is much more common than koritnigite.

The evaluation in the system MnO–As₂O₅–H₂O is more difficult. The data for manganohörnesite [vivianite group, Mn₃(AsO₄)₂⋅8H₂O] from Johnston and Singer (2007) are uncertain and there are no thermodynamic data for eveite [olivenite group, Mn₂(AsO₄)(OH)], to our best knowledge. There is another datum for the solubility product of krautite available (Tournassat et al., 2002). Converting their data to the reaction used in this present work (see footnote in Table 3), their value of log K _sp is –6.7 to –6.9, comparing fairly well to our datum of –6.10. Calculations suggest that manganohörnesite is stable over most of the diagram (Fig. 6a). We have to emphasise that the thermodynamic data for eveite are not known and this phase, similarly to adamite in the system with zinc, could displace much of the stability field of manganohörnesite. A small field for krautite appears under low pH and high aqueous ion concentrations.

The system CuO–As₂O₅–H₂O is complex because of the number of phases, resulting from the flexibility of coordination of Cu²⁺ in their structures. The stable phase in the system is olivenite [Cu₂(AsO₄)(OH)], the mineral structurally very similar to adamite and eveite. The structural analogue of köttigite and manganohörnesite is the rare mineral babánekite [vivianite group, Cu₃(AsO₄)₂⋅8H₂O] (Plášil et al., 2017) for which no thermodynamic data are available. Lide (2005) gave a solubility product for Cu₃(AsO₄)₂, suggesting that it may perhaps relate to a phase such as babánekite, but implying that such a phase would be quite stable. Such stability is in stark contrast with the rare occurrence of such a phase. Suppressing olivenite from the calculations, a small geminite field appears at low pH and high Cu and As concentrations (Fig. 5b), as expected. These predictions agree with the occurrence and assemblages of geminite as a relatively abundant secondary mineral in Jáchymov (Škácha et al., 2019; Majzlan et al., 2020).

Limited natural observations on aqueous solutions that precipitate M[AsO₃(OH)]⋅H₂O minerals support our findings. Recently, we have described solutions that precipitate cobaltkoritnigite [(Co,Zn)[AsO₃(OH)]⋅H₂O] in underground spaces in Jáchymov, Czech Republic (Majzlan et al., 2020, their tables 2 and 3). The pH of these two solutions were 2.41 and 2.96. Calculations with PHREEQC (Parkhurst and Appelo, 1999; LLNL database) returned saturation indices for koritnigite of –0.48 and +0.78. This result proves that a koritnigite-like phase is near equilibrium with these aqueous solutions, in good agreement with the active precipitation of cobaltkoritnigite at this site.

Haidingerite [Ca[AsO₃(OH)]⋅H₂O] and the chemically related pharmacolite [Ca[AsO₃(OH)]⋅2H₂O] are predicted to crystallise from Ca- and As-rich solutions (Majzlan et al., 2020). In this case, however, their solubility is higher than for the M[AsO₃(OH)]⋅H₂O with M ²⁺ = Cu, Zn or Mn. Because of the much larger ionic radius of Ca²⁺ (in comparison to Cu²⁺, Zn²⁺ or Mn²⁺), there are limited structural relationships between the systems CaO–As₂O₅–H₂O and those with CuO, ZnO, or MnO.

In the system MgO–As₂O₅–H₂O, the mineral magnesiokoritnigite [Mg[AsO₃(OH)]⋅H₂O] was described recently by Kampf et al. (2013). It occurs together with anhydrite, halite, lavendulan, quartz and scorodite at the Torrecillas mine in the Atacama Desert in Chile. The association with scorodite and lavendulan suggests precipitation from acidic solutions and the presence of anhydrite implies low activity of H₂O, elevated formation temperature, low relative air humidity, or combination of such factors. The Atacama Desert is dry but not particularly hot (McKay et al., 2003), with average temperatures of 16–17°C and air humidity of 25%. The humidity of the air and soil could occasionally increase during the rare rain events. Having recently derived a thermodynamic data set for brassite [Mg[AsO₃(OH)]⋅4H₂O] and rösslerite [Mg[AsO₃(OH)]⋅7H₂O] (Majzlan et al., 2020), we can estimate roughly the thermodynamic properties of magnesiokoritnigite. Considering the reaction:

$${\rm Mg}[ {{\rm As}{\rm O}_3( {{\rm OH}} ) } ] \cdot 4{\rm H}_2{\rm O\ }( {{\rm cr}} ) \to {\rm Mg}[ {{\rm As}{\rm O}_3( {{\rm OH}} ) } ] \cdot {\rm H}_2{\rm O\ }( {{\rm cr}} ) + 3{\rm H}_2{\rm O\ }( {\rm g} ) .$$

If these phases are in equilibrium at 25°C and relative air humidity (RH) of 25%, the calculation gives Δ_fG°(magnesiokoritnigite) of –1418 kJ⋅mol^–1 and the solubility product of –2.0. The equilibrium may lie at even lower air humidities and in that case, the solubility product would be higher. For example, if RH = 10%, then log K _sp = –0.9. We adopt log K _sp of –2.0 as a rough estimate and compare this value to the log K _sp values for other minerals in this group.

Field observations of assemblages with koritnigite and krautite

Our field observations in underground spaces in Jáchymov showed that precipitation of cobaltkoritnigite and koritnigite is connected to strongly acidic solutions (Majzlan et al., 2020). They are derived from oxidative weathering of nickelskutterudite or krutovite in the absence of carbonate gangue minerals which could serve as a pH buffer. Cobaltkoritnigite seems much scarcer than koritnigite, in agreement with the general prevalence of Ni over Co in the ores in Jáchymov. As there are no carbonates as potential sources of Ca or Mn, haidingerite (which otherwise can precipitate under similar pH conditions as koritnigite) has never been observed together with koritnigite and its occurrences are linked to other locations in the mines.

Koritnigite and cobaltkoritnigite are rarely associated with the vivianite-group minerals köttigite and erythrite. The latter minerals should form from mildly acidic or neutral solutions and grow centimetres to decimetres away from the koritnigite and cobaltkoritnigite aggregates. Such associations document strong pH gradients over short distances that could be driven only by neutralisation by primary carbonates in specific local environments.

Krautite occurs in the same parts of the galleries of the Geschieber vein in the Svornost mine as koritnigite and cobaltkoritnigite. The source of Mn is probably a small amount of the gangue carbonates. This notion could be supported by the fact that unlike cobaltkoritnigite/koritnigite, which occur without any directly associated secondary phases, krautite usually grows on gypsum crystals. Therefore, both Ca and Mn can be derived from the dissolved carbonates. Calcium reacts preferably with sulfate in the solution to form gypsum, whereas Mn, and probably also Zn and Co, prefer the arsenates structures of the krautite-type.