1 Introduction
Suppose a simple game in which a player wins when she or he draws a red ball from a box. The box contains 100 balls and six of them are red. A hundred players take turns to draw a ball and then return the ball they drew back to the box. Which turn would you want to take in playing the game? This type of game is called a game with replacement. The winning odds of such a game do not vary with playing order because the preceding outcomes (e.g., your friend won the prize) do not affect the probabilities of later draws.
Another type of a game is a game without replacement. In the same game, one does not return the ball into the box after a draw. Then, previous results change the winning chance of later draws (e.g., your friend’s winning of the prize means that you have a reduced chance). This is typical of a game without replacement. Let us consider another example. Suppose that an electronic shop launches a mystery box promotion. The mystery box includes Apple’s products and 100 eligible customers can buy one. The customers do not know what products are inside the box until they purchase and open one. The shop announced that six out of the 100 mystery boxes include a brand-new MacBook that you desire badly. Luckily, you were one of the 100 persons. On the mystery box promotion day, the 100 people wait in line, with most of them dreaming of winning a jackpot. The purchase order is according to the position of the waiting line. The shop sells boxes in completely random order. This mystery box promotion is an example of a game without replacement if the boxes purchased by consumers are not replaced with the same ones.
Suppose that the purchase has not started yet. Then, which turn do you prefer? First, second, third, middle, or last? Do you think that the probability of winning a MacBook changes with your purchase order? Now, the purchase has started and 90 people have bought a mystery box. You are one of the last ten people in line. Surprisingly, none of the 90 people got a MacBook. Therefore, out of the ten remaining boxes, six include a brand-new MacBook. This circumstance still falls under a game without replacement, with a change in winning odds from 6% to 60%. Then when do you want to purchase? Right now, or wait for longer? Does the order matter in winning a MacBook now?
The answer is that in any case, the purchase order does not matter. In games without replacement, previous outcomes change the winning probabilities of later draws, such as from 6% to 60% in the mystery box case. Given the changed probability, however, the draw order does not alter the winning probabilities. Nevertheless, a simple pre-test (N = 95) showed that more than 60% of the respondents answered that the chance of winning differs depending on the game order for games without replacement.
In summary, a rational decision maker should be indifferent to the draw order in both games with and without replacement if the chance of winning is the only consideration. The current research, however, suggests that people have biased preference for playing order. We posit that motivational factors and probability misestimation affect preference for draw orders and that their influences vary depending on the game type. For games with replacement, motivational factors such as uncertainty aversion make people prefer earlier orders. However, probability misestimation should have a weak or no influence because of the game’s simple structure. For games without replacement, both motivational and probability (mis)estimation would affect draw order preference because every draw keeps changing the winning probability. People prefer to take earlier than later draws because of motivational factors such as uncertainty aversion, ambiguity aversion, and uncontrollability aversion, and this tendency is moderated by game valence as a result of probability misestimation. Specifically, compared with a neutral game (50–50 chance), an earlier draw is preferred in a gain-dominant game (high probability of winning), whereas a later draw is preferred in a loss-dominant game (high probability of losing).
From a theoretical perspective, this study investigates preference for game order, a feature that has been received little attention in decision-making research. Previous studies have mostly focused on one-shot binary-choices with fixed probabilities of winning (e.g., choices between two options with different probabilities and amounts of gains). This work examines games with multiple players and explores the mechanism whereby people choose the playing order. In particular, studying a game without replacement provides insights about decision making processes when dynamic changes occur in the probability of winning.
This paper is organized as follows. First, we demonstrate that winning probabilities are unaffected by the playing order in games with and without replacement. We then propose motivational factors and probability misestimation as the main forces that drive playing order preferences. Studies 1 and 2 test games without replacement and report empirical evidence for biases in playing order preference. Study 3 tests a game with replacement. Lastly, we discuss the theoretical and practical implications of the current research.
1.1 Game order and probability of winning
Games with replacement are simple to understand because the preceding outcome does not change the winning probability of later draws. Thus, it is straightforward that all draws have the same chance of winning. People with very basic knowledge of probability are aware of this fact. Therefore, a rational decision maker should not show preference for some specific draw orders if the chance of winning is the only concern.
By contrast, games without replacement are complex because the winning probability changes every time a draw is made as the game moves onward. However, the winning probability is unaffected by the draw order, although prior outcomes affect the winning likelihoods themselves. The following example illustrates that the expected probability of winning is not affected by the draw order in a game without replacement. In a game, players draw balls from an urn containing n balls, with x red balls and n−x white balls. A player who draws a red ball wins the game (outcome: W), whereas one who draws a white ball loses the game (outcome: L). Balls are drawn from the urn one at a time, and the drawn balls are not replaced. Players decide the order in which they draw.
In this game, the expected probability of winning at the i-th turn, p(W i), follows a hypergeometric distribution and has two important properties. First, if the result of the draw is unknown (e.g., before the draw), every draw in the sequence has the same probability of winning, p(W i) = x/n = p. In the first draw, the probability of winning is simply p(W 1) = p. In the second and later draws, the calculation is more complicated and involves joint and conditional probabilities. In the second draw, the expected probability of winning is the sum of the conditional winning probabilities in that draw given the result of the first draw, as shown in Equation (1). The expected winning probability of the second draw is also p(W 2) = p. Similarly, the expected probabilities of winning in the third and later draws are the same as p but require a more complicated computation.
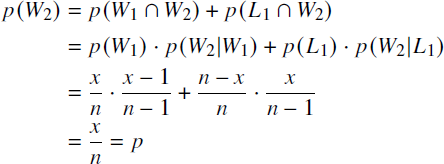
Second, once the game starts, the results of earlier draws change the expected probability of winning at the i-th turn, p *(W i). Given this altered probability, however, the draw order does not affect the winning probabilities for later draws. That is, the game resets to a game with the altered probability of winning that is determined by the remaining balls. For example, the probability of winning in the second draw, p *(W 2), is determined by the first draw result. With the remaining balls, the game resets to the changed probability, and the expected probability of winning for later draws is the same as p *(W 2).
Figure 1 presents a specific example of the game results and probabilities. Five players participate in a game of drawing a ball from an urn containing three red (winning) balls and two white (losing) balls. The expected probability of winning in the i-th draw, p(W i), is always .60, regardless of the draw order. For the first player, the expected probability of winning, p(W 1), is merely .60. For the second player, the probability of winning, p(W 2), is the same as that of the first player despite being dependent on the first draw result. When the first player wins by drawing a red ball (probability of .60), the urn then contains two red balls and two white balls. Given the first result, the conditional probability of winning for the second drawer is .50. The probability that the second player would win in this case is p(W 1 ∩ W 2) = .60 · .50 = .30. However, if the first player draws a white ball (probability of .40), three red balls and a white ball would remain in the urn. In this case, the second player’s conditional probability of winning is .75. The probability that this outcome would occur is p(L 1 ∩ W 2) = .40 · .75 = .30. Thus, the expected probability of the second player’s winning is expressed as p(W 2) = p(W 1 ∩ W 2) + p(L 1 ∩ W 2) = .60, which is equal to the first player’s winning probability.
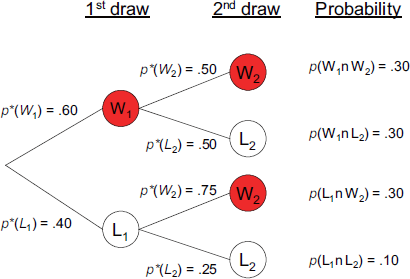
Figure 1: Outcomes and their probabilities for a hypothetical game without replacement. This figure shows the possible outcomes and their probabilities in the first two draws of a no-replacement game with three red (winning) balls and two white (losing) balls.
Once the game starts and the outcome of the first draw is known, the probabilities of winning for later draws change. In the example, if the result of the first draw is a win, the probabilities of winning in the later draws would change from .60 to .50. Given the changed winning odds, the game resets as one with two winning balls and two losing balls. Moreover, the expected winning probabilities for all remaining draws are the same as .50 until the draw of the next ball. Therefore, a rational decision maker should be indifferent to the draw order in a game without replacement because the order does not affect the chances of winning.
Taken together, the draw order does not matter for games with and without replacement. However, theories on decision making suggest that players prefer to draw early in the games and this tendency is moderated by game valence, especially for no-replacement games.
1.2 Psychological factors affecting playing order
1.2.1 Motivational factors
In games with and without replacement, some motivational factors should favor earlier rather than later draws. These factors include the tendencies of people to avoid uncertainty, ambiguity, and uncontrollability. Uncertainty aversion affects both games with and without replacement. However, ambiguity and uncontrollability aversion influence only the games without replacement.
Uncertainty aversion
People have a tendency to disfavor uncertainty when outcomes are probabilistic. People feel anxious in uncertain situations, and this anxiety motivates them to resolve the uncertainty as soon as possible. Uncertainty aversion is a robust phenomenon that characterizes decision making in gambling, investments, and consumer choice (Reference Gneezy, List and WuGneezy et al., 2006; Reference Kahneman and TverskyKahneman & Tversky, 1979; Reference Newman and MochonNewman & Mochon, 2012; Reference SimonsohnSimonsohn, 2009). The principle also predicts that for games with and without replacement, people are likely to resolve uncertainty by taking an early draw because it frees them from anxiety and also gratifies curiosity about the result (Reference Calvo and CastilloCalvo & Castillo, 2001; Reference LoewensteinLoewenstein, 1994; Reference Lovallo and KahnemanLovallo & Kahneman, 2000). Therefore, we expect that regardless of replacement, the need to resolve uncertainty drives preference for drawing early, rather than late.
Ambiguity aversion
Individuals prefer situations wherein the apparent “objective” probabilities of outcomes are known with certitude, rather than are unknown or uncertain (Reference Camerer and WeberCamerer & Weber, 1992; Reference Güney and NewellGüney & Newell, 2015). Disliking this lack of information is called ambiguity aversion. In Reference EllsbergEllsberg’s (1961) well-known example, an urn contains 90 red, black, and yellow balls. Of the 90 balls, 30 balls are red. The other 60 balls are either black or yellow but the exact number of each color is unknown. Participants bet on the color of the ball drawn from the urn. In this case, people tend to select red, although the other colors have the same odds of winning. This example shows a tendency to prefer known chances (e.g., red balls) to the unknown (e.g., black or yellow balls).
The tendency to avoid an “unknown probability” should motivate an early draw in a no-replacement game. The available proportions of later winning draws are not clearly known in advance because they can be altered according to the results of earlier draws. In the first or earlier draw, however, the chance of winning is rather clearly known. Therefore, ambiguity aversion is expected to lead to preference for early draws. By contrast, the winning chances of games with replacement are fixed, and therefore ambiguity aversion should have little effect.
Uncontrollability aversion
The desire for control also predicts preference for an early draw in no-replacement games. People have a desire for control and tend to avoid uncontrollable situations (Reference CutrightCutright, 2012; Reference Cutright and SamperCutright & Samper, 2014; Reference LangerLanger, 1975). For example, people are happier when they believe outcomes are due to their own decisions and actions rather than due to external forces (Reference Shojaee and FrenchShojaee & French, 2014). In a no-replacement game, an early draw’s outcome is determined by the player’s own action, but the results of later draws are determined by the previous draws. Therefore, the desire for control should lead to preference for early draws. In a game with replacement in which others’ decisions do not have any influence, uncontrollability aversion should have little effect on order preference.
1.2.2 Probability misestimation
Another factor affecting playing order preference is probability misestimation, one that we expect to have a significant effect only for no-replacement games. We posit that the extent to which one prefers early draws is moderated by the winning probability of a game. Suppose that three no-replacement games with different odds of winning are carried out. In the first game, 75% of the balls are of a winning color and 25% are of a losing color (i.e., a gain-dominant game). In the second game, 25% of the balls are of a winning color and 75% are of a losing color (i.e., a loss-dominant game). The third game has an equal number (50%) of winning and losing balls (i.e., a neutral game). The inclination to prefer an early draw is expected to be greater in a gain game than in a loss game. We conjecture that this effect occurs because of the erroneous estimates of the winning probability as people tend to rely on judgment heuristics rather than on computations of relative frequencies (Reference Gilovich, Griffin and KahnemanGilovich et al., 2002; Reference Kahneman and TverskyKahneman et al., 1982).
Judgment heuristics related to probability misestimation in a no-replacement game arise from ignorance of conditional probabilities and the representativeness heuristic (Reference Kahneman and TverskyKahneman & Tversky, 1972; Reference Tversky and KahnemanTversky & Kahneman, 1981). The winning probability in the first draw is rather simple. However, probability estimation for later draws requires a more complicated calculation involving conditional probabilities and joint probabilities, and thus individuals tend to ignore conditional probability (Reference Tversky and KahnemanTversky & Kahneman, 1981). This ignorance results in a greater chance of probability misestimation (Reference Bar-HillelBar-Hillel, 1973; Reference GneezyGneezy, 1996; Reference Tentori, Crupi and RussoTentori et al., 2013).
Furthermore, the representativeness heuristic systemically biases the direction of misestimation. According to the representativeness heuristic, the likelihood of a specific event is overestimated when it resembles the salient features of the population (Reference Bordalo, Coffman, Gennaioli and ShleiferBordalo et al., 2016; Reference Kahneman and TverskyKahneman & Tversky, 1972). If players rely on the representativeness heuristic in a no-replacement game, then they would estimate the likelihood of a winning or losing outcome based on the similarity with the population’s salient features. Consider a game with six winning balls and two losing balls. When guessing for earlier turns, the representativeness heuristic suggests that one would overestimate the likelihood of a winning ball because the population includes more winning balls which constitute a salient feature. This bias naturally leads to the belief that for later draws, the less salient feature (i.e., a losing ball) is more likely to be drawn. This pattern in a no-replacement game does not necessarily imply the gambler’s fallacy, which explains the perceived negative autocorrelation in games with replacement with constant probabilities.
In short, ignorance of conditional probabilities and the representativeness heuristic imply that the subjective probability for an early (late) draw is biased toward the event with the higher (lower) objective probability of occurrence. Thus, when gains are salient, individuals estimate the chance of winning as higher for earlier draws and lower for later draws. On the other hand, when losses are more salient, the estimation of the winning probability would decrease for earlier draws but increase for later draws. In a neutral frame game with a 50–50 chance of winning and losing, neither gains nor losses are more salient. Therefore, the misestimation of the probabilities is less likely compared with the gain or loss games.
2 Study 1: A game without replacement
Study 1 aims to show the draw order preference in a game without replacement and the moderating role of game valence. Participants were asked to indicate their preferred draw order under a scenario that varies in the game’s valence and size.
2.1 Method
A total of 168 university students (34.3% females, M age = 21.9) participated in a 3 (game valence: gain vs. loss vs. neutral) x 2 (game size: 8 vs. 24) between-subjects. Participants were presented with a hypothetical game scenario. They were asked to imagine themselves playing the game with other people. In the game, each person draws a ball from an urn containing red and white balls. The drawn balls are not replaced. The player who picks a red ball wins $10 and the one who picks a white ball loses $10.
The valence of game was manipulated by the ratio of red and white balls. In the gain condition, the game offered a 75% chance of winning and a 25% chance of losing (p(W) = .75). In the loss condition, these probabilities were reversed, with a 75% chance of losing and a 25% chance of winning (p(W) = .25). In the neutral condition, the odds were 50:50 (p(W) = .50). The game size was also manipulated. The scenario described either a game with eight participants drawing balls from an urn containing eight balls (small size) or a game with 24 participants with 24 balls (large size). Because the game size is added for testing generalizabilityFootnote 1, the main test compares the three valence conditions. Our sample size is close to a minimum of 64 participants in each valence group for having a statistical power of .80, given the assumption of moderate effect size (Cohen’s d = .50) because no similar previous studies exist.
Participants indicated their preferred draw order through an open-ended question and then wrote down the reasons for their preference. We also measured the estimates of the winning probability if they drew first, middle (4th in the 8-person game and 12th in the 24-person game), and last on a 101-point sliding-bar scale ranging from 0% to 100%.
2.2 Results
Preference for draw Order
First, the draw order preferences in the large game (range of 1 to 24) were converted to a range between 1 and 8. Specifically, a preference for 1st, 2nd, or 3rd draw in the large game was defined as a 1st draw preference, a preference for a 4th, 5th, or 6th draw as a 2nd draw preference, and so forth.Footnote 2
The distribution of preferences showed that most participants favored early draws (Figure 2). Overall, 51.2% preferred to draw first, and 83.3% preferred to draw first, second, or third. In all six conditions, the average preferred draw order was significantly lower than the median of 4.5 (t > 5.59, p < .001, Cohen’s d > 1.08). Table 1 presents the average of the preferred order in each condition. These results provide strong evidence that people prefer early draws in games without replacement.

Figure 2: Distributions of draw order preference in Study 1.
Table 1: Preferred draw order as a function of game valence and size in Study 1.

Note. The preferred order in the large game condition was transformed so that the value ranged between 1 and 8. The raw preferred orders in the large game condition (1 to 24) are in brackets.
Game valence
Table 1 also shows that the draw
order preferences were influenced by how the game was valanced. A 3 (game
valence: gain vs. neutral vs. order) x 2 (game size: 8 vs. 24) ANOVA on the
preferred draw order revealed that only the main effect of valence was
significant (M gain = 1.84 vs.
M neutral = 2.02 vs.
M loss = 2.65); F(2, 162) =
4.31, p = .015, ![]() = 0.05).
Neither the main effect of game size (M 8 = 2.28
vs. M 24 = 2.05; F(1, 162) =
0.95, p = .332,
= 0.05).
Neither the main effect of game size (M 8 = 2.28
vs. M 24 = 2.05; F(1, 162) =
0.95, p = .332, ![]() = 0.01)
nor the interaction (F(2, 162) = 0.01, p =
.988,
= 0.01)
nor the interaction (F(2, 162) = 0.01, p =
.988, ![]() < 0.01) was
significant. In the analyses reported below, the small and large size
conditions were combined because the effect of game size was not
significant.
< 0.01) was
significant. In the analyses reported below, the small and large size
conditions were combined because the effect of game size was not
significant.
The follow-up marginal mean comparisons indicated that participants preferred earlier draws for the gain game than for the loss game (M gain = 1.84 vs. M loss = 2.65; F(1, 162) = 7.85, p = .006, d = 0.53). The preferred order with the neutral game (M = 2.02) fell between the gain and loss games and was significantly earlier than the loss game (F(1, 162) = 4.75, p = .031, d = 0.41) but was not significantly different from the gain game (F(1, 162) = 0.39, p = .534, d = 0.12).
Similarly, the percentage of participants preferring to draw first differed as a function of valence (Figure 2). The percentages of participants who preferred to draw first were 68% in the gain game, 54% in the neutral game, and 32% in the loss game (χ2(2, N=168) = 14.48, p = .001, ϕ = 0.29).
Reasons for order preference
We also analyzed participants’ stated reasons for their draw order preferences. Two trained judges conducted content analysis of these reasons. On the basis of the hypothesis of this study, the judges classified the stated reasons into (1) uncertainty aversion, (2) ambiguity aversion, (3) uncontrollability aversion, (4) probability (mis)estimation, and (5) others. The statement of a participant could include more than one type of reason. Disagreements were resolved by discussion.
Statements revealing discomfort about the uncertainty of the game (e.g., “I want to know the result as early as possible because I am too nervous to wait.”) were classified as uncertainty aversion statements. Those statements that expressed concern about not being able to determine the exact odds of winning on later draws were classified as ambiguity aversion. Uncontrollability aversion statements expressed concern about the results possibly being determined by others, instead of by the player herself or himself. Statements regarding probabilities were further divided into four categories: (i) early draws have a higher probability of winning, (ii) middle draws have a higher probability of winning, (iii) later draws have a higher probability of winning, and (iv) draw order is unrelated to the probability of winning. The first three categories were considered misperceptions regarding the probability of winning.
First, a multiple regression analysisFootnote 3 was employed to test whether the reasons are related to the preferred draw order in all the valence conditions combined. Specifically, the preferred draw order was regressed on the cited reasons for preference. The estimated standardized beta coefficients are presented in Column 2 of Table 2. Motivational factors were related to the preference for early draws, as indicated by the significant negative beta coefficients for uncertainty aversion (β = –.30, t(160) = 4.36, p < .001), ambiguity aversion (β = –.20, t(160) = 2.97, p = .003), and uncontrollability aversion (β = –.15, t(160) = 2.30, p = .023). Tests about probability estimation showed that mentioning an early-draw advantage was significantly related to preference for earlier draws (β = –.36, t(160) = 4.80, p < .001), whereas mentioning a late-draw advantage was significantly related to preference for later draws (β =.29, t(160) = 4.45, p < .001). However, statements that expressed an advantage for a middle draw (β = .05, t(160) = 0.68, p = .500) or statements that mentioned no relation between draw order and probability (β = –.14, t(160) = 1.81, p = .073) were not significantly related to draw order preference.
Table 2: Stated reasons for preferred draw order as a function of game valence in Study 1.
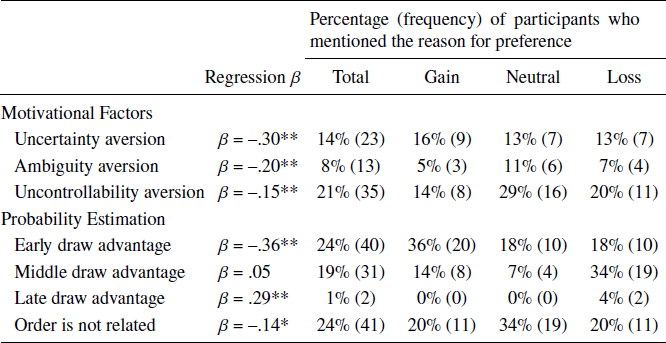
Note. Regression betas are standardized beta coefficients from a multiple regression analysis that tests the relationship between each type of reason and preferred order. The number of participants is in parentheses.
* p < .10
** p < .05.
Second, chi-square tests examined whether the stated reasons differed across the game valence conditions. Note that “ambiguity aversion” and “late draw advantage” were excluded from the test because they failed to satisfy the assumptions for chi-square tests (e.g., minimum 5 expected observations per cell). Table 2 (Columns 4 to 6) presents the percentage and frequency of participants who stated each type of reason in each valence condition. Mentioning motivational forces (aversions to uncertainty and uncontrollability) related to a preference for early draws did not differ as a function of valence, as indicated by the insignificant chi-square tests (ps > .171). However, the percentages of participants who indicated that “early draws have a higher probability of winning” (χ2(2, N=168) = 6.56, p = .038, ϕ = 0.20) and “middle draws have a higher probability of winning” (χ2(2, N=168) = 14.32, p = .001, ϕ = 0.29) differed as a function of game valence. Follow-up tests revealed that the percentage of participants whose statements favored early draws was significantly higher in the gain game (36%) than in the neutral game (18%; z = 2.13, p = .033, h = 0.41) and in the loss game (18%; z = 2.13) p = .033, h = 0.41. On the contrary, the advantage of middle draws was mentioned more frequently in the loss game (34%) than in the gain game (14%; z = 2.43, p = .015, h = 0.48) and the neutral game (7%; z = 3.51, p < .001, h = 0.71).
Winning odds estimation
We also tested whether the estimates of the winning probability for the
first, middle, and last draws were influenced by game valence (Figure 3). The results of a 3 (game
valence: gain vs. neutral vs. loss) x 3 (draw order: first vs. middle vs.
last) mixed ANOVA showed a significant main effect of game valence
F(2, 165) = 164.51, p < .001,
![]() = 0.67), a significant main
effect of draw order (F(2, 330) = 58.64, p
< .001,
= 0.67), a significant main
effect of draw order (F(2, 330) = 58.64, p
< .001, ![]() = 0.26), and a significant
two-way interaction (F(4, 330) = 5.98, p
< .001,
= 0.26), and a significant
two-way interaction (F(4, 330) = 5.98, p
< .001, ![]() = 0.07). The significant
main effect of game valence was expected because the odds of winning were
manipulated to be different across the game valence conditions
(M gain = .63 vs.
M neutral = .47 vs.
M loss = .26). The significant main effect of
the draw order indicates that participants believed that the winning
probabilities were higher for the earlier draws
(M first = .50 vs.
M middle = .48 vs.
M last = .37).
= 0.07). The significant
main effect of game valence was expected because the odds of winning were
manipulated to be different across the game valence conditions
(M gain = .63 vs.
M neutral = .47 vs.
M loss = .26). The significant main effect of
the draw order indicates that participants believed that the winning
probabilities were higher for the earlier draws
(M first = .50 vs.
M middle = .48 vs.
M last = .37).
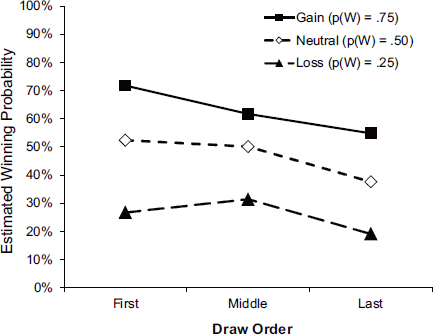
Figure 3: Estimated probabilities of winning with the first, middle, and last draws in Study 1
The significant interaction indicates that the influence of draw order on estimated probability differed as a function of game valence. Cell-mean contrasts were conducted for each valence condition. For the gain game, the estimated probabilities of winning were highest in the first draw, second highest in the middle draw, and lowest in the last draw (M first = .72 vs. M middle = .62 vs. M last = .55). All these estimated probabilities significantly differed from one another (F(1, 330) > 9.54, p < .002, d > 0.58). For the loss game, the estimated probabilities of winning were highest in the middle draw, second highest in the first draw, and lowest in the last draw (M first = .27 vs. M middle = .31 vs. M last = .19). Again, all the differences were significant (F(1, 330) > 4.47, p < .035, d > 0.40). For the neutral game, the estimated probabilities in the first and middle draws were the highest. They did not differ significantly from each other (M first = .52 vs. M middle = .50; F(1, 330) = 0.90, p = .343, d = 0.18) but were significantly higher than the last draw (M last = .38; F(1, 330) < 31.95, p < .001, d > 1.07). Figure 3 shows the estimated probabilities in each condition.
2.3 Discussion
The results of Study 1 provide evidence that individuals prefer early draws in a game without replacement. This preference can be explained by motivational factors such as the avoidance of uncertainty, ambiguity, and uncontrollability. In addition, game valence affects draw order preferences. When winning is more salient, participants prefer an early draw. Conversely, participants opt for a later draw when the loss is more prominent. This influence of game valence on draw order is related to the misestimation of winning probability. Specifically, participants tend to believe that the winning probability is higher for early draws than for later draws when the winning odds are high. However, participants tend to believe that middle draws have the highest probability of winning when the odds of losing are high.
A noteworthy finding of Study 1 is that the estimated probability of winning was lowest in the last draw, regardless of game valence (Figure 3). Although unexpected, this finding can be explained by vividness and uncontrollability. First, imagining what will happen (i.e., vividness) is more difficult for very late draws. People tend to underestimate the likelihood of events that are difficult to imagine (Reference Gregory, Cialdini and CarpenterGregory et al., 1982; Reference Sherman, Cialdini, Schwartzman and ReynoldsSherman et al., 1985). Thus, low vividness for late draws leads to an underestimation of winning probabilities. Second, people tend to underestimate probabilities when they have no control over the outcome, whereas they overestimate the likelihood of success when the source of uncertainty is internal (Reference Brown and BaneBrown & Bane, 1975; Reference HowellHowell, 1971). This finding implies that participants underestimated the odds for later draws because they perceived themselves as having less control in later draws than in earlier draws.
In summary, Study 1 demonstrates a preference for drawing order and tests the factors that influence this preference in a hypothetical game. However, it is uncertain whether the same findings would be observed in a real game with actual players and real monetary incentives. Thus, Study 2 tests preferences for draw orders in a real game situation.
3 Study 2: A game without replacement with monetary outcomes
In Study 2, groups of six participants played a real game without replacement. Players drew a ball from a bag one by one, and those who drew a ball of a winning color received a $3 prize, whereas those who drew a ball of a losing color lost $3. The dependent variable was the participants’ decisions regarding whether to draw right away or defer their draw. Although the results of preceding draws determine the winning probability for a given draw, the same probability applies to all later draws including the given draw. We tested whether the “draw-or-defer” decision is influenced by the probability of winning (i.e., game valence) at each turn.
3.1 Method
A total of 84 university students (32.1% females, M age = 21.3) participated on a voluntary basis. Participants were informed that they would receive $6 as game money and play a game twice, betting $3 on each gamble. They were divided into 14 groups of six persons. Participants who knew one another well were assigned to different groups to avoid potential social influence.
Before the game, the experimenter explained the procedure of the game in detail. Participants would draw a ball out of a pouch containing three orange and three white ping-pong balls. A participant who draws an orange ball would receive $3, whereas one who draws a white ball would lose $3.
The priority to decide whether to draw or defer was randomly determined by having participants pick a card with a number from 1 to 6. The person with the lower number had priority in deciding whether he or she would draw or defer at each turn. At each draw, the participant with the top priority (with the lowest number) was asked to decide whether he or she would draw at this turn. Once a participant drew a ball, he or she no longer had a chance to draw again for the game. Everyone else could see the outcome and the experimenter told them the number of remaining winning and losing balls. If the participant decided to defer, then the one with the next priority would decide whether or not to draw. If everyone decided to defer, the one with the lowest priority had to draw a ball (This case was excluded from the analyses because the draw was forced). The game continued until all six people in a group drew a ball. However, when the remaining balls were of the same color (e.g., three orange balls remain in the pouch after a streak of a white ball for the first three draws) or only one ball remained, the gamble turned into a certain game without uncertainty. Then, these game outcomes were determined without participants’ draw or defer decisions. After the game, the winners received $3, whereas the losers paid $3 to the experimenter.
The same game was played twice. The procedure of the second game was the same, except that the priority to make a decision was randomly determined again for the second game. During the game, the experimenter recorded the draw-or-defer decisions and the results of the draws. A total of 172 draw-or-defer decisions (79 decisions in game 1 and 93 decisions in game 2) were made by the players. Note that the dependent variable we analyzed was the decisions rather than the players. For example, if the first two players deferred their decisions and the third players drew a ball, then they were counted as two defer decisions and one draw decision. When a game outcome was decided without a player’s decision (e.g., only one ball remaining), it was not counted as a player’s draw-or-defer decision.
3.2 Results
We tested the influence of the winning probability at each turn on the draw-or-defer decisions. Due to the nature of the game, the odds of winning changed as the game progressed, depending on which ball was drawn in the preceding draws. For example, if the first ball was orange, then the pouch would contain two orange balls and three white balls, thereby resulting in a 40% probability of winning. For each draw, the winning probability was calculated and the influence of this probability on the draw-or-defer decision was tested. If the outcome was determined without the player’s decision (e.g., one ball remains or the remaining balls are of the same color) or a draw decision was forced, then we excluded those draws from the analysis.
Before testing the hypotheses, the differences between the two games were tested. No significant differences were found between the two games in the draw-or-defer decisions (χ2(1, N=172) = 0.37, p = .374, ϕ = 0.04). Thus, the games were collapsedFootnote 4, thereby resulting in 28 games.
Table 3 presents the percentages of the draw and defer decisions for each turn. Participants chose to draw more frequently than defer, and the probability of winning moderated this decision. In the first draw, participants were more likely to draw (76%) rather than to defer (24%). A goodness-of-fit test shows that the decisions deviated significantly from randomness, thereby indicating a preference for drawing to deferring (χ2(1, N=37) = 9.76, p = .002, ϕ = 0.51). In the second, third, and fourth draws, the winning probability strongly influenced the decision. In the second draw, the winning probability was .60 when the first draw was a loss and .40 when the first draw was a win. When the winning probability was .60, 79% chose to draw, compared with 46% when the winning probability was .40, and the difference was significant (χ2(1, N=45) = 4.92, p = .027, ϕ = 0.33). Similarly, in the third draw, the percentages of participants who chose to draw were 100%, 79%, and 45%, when the winning odds were .75, .50, and .25, respectively (χ2(2, N=38) = 7.65, p = .022, ϕ = 0.45). In the fourth draw, 100% of participants chose to draw when the winning probability was .67, whereas the draw rate was 53% when the winning probability was .33 (χ2(1, N=33) = 9.12, p = .003, ϕ = 0.53). In the fifth draw, 95% decided to draw when the winning probability was 50% (one orange ball and one white ball remaining), thereby indicating a strong preference for drawing to deferral (χ2(1, N=19) = 15.21, p < .001, ϕ = 0.89). Figure 4 graphically illustrates the participants’ decisions in the first, second, and third draw turns given the results of the preceding draws.
Table 3: Draw or defer decisions as a function of the probability of winning in Study 2.
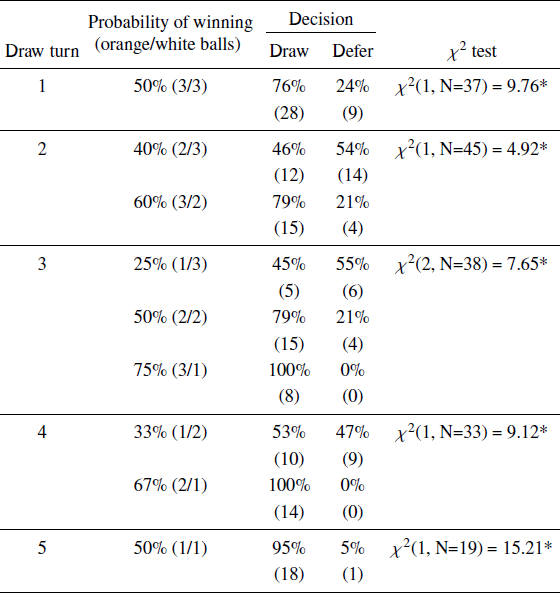
Note. Chi-square tests in the first and fifth draws tested goodness-of-fit assuming a 50% draw-defer split. Chi-square tests in the second, third, and fourth draws examined whether a draw-or-defer decision was associated with the winning probability. The number of each decision is in parentheses.
* p < .05.
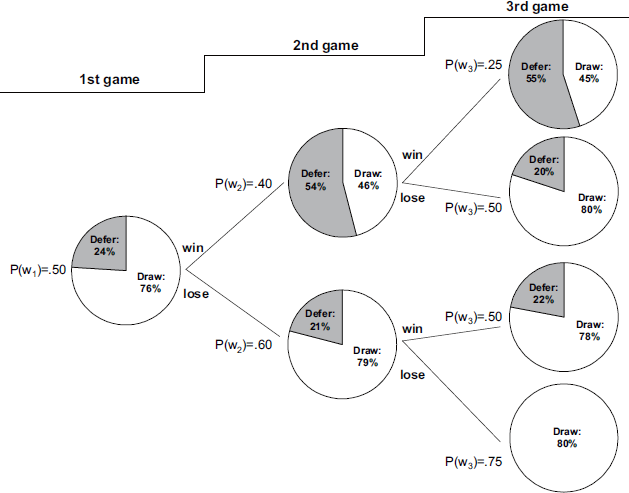
Figure 4: Draw-or-defer decisions for the first, second, and third turns in Study 2. The pie charts show the percentages of participants who decided to draw and defer with odds of winning p *(wi). These odds were determined by how many winning and losing balls remained in the pouch after the preceding draw was made.
In addition, the potential influence of various factors on the draw-or-defer decision was tested. First, the draw-or-defer decisions were not influenced by the decision of the person in the immediately preceding turn (χ2(1, N=142) = 3.00, p = .083, ϕ = 0.15).Footnote 5 Second, no significant influence of decision priority was found on the draw-or-defer decisions (χ2(4, N=172) = 3.91, p = .419, ϕ = 0.15). Third, the outcome of the first game (winning or losing $3) had no significant effect on the draw-or-defer decisions in the second game (χ2(1, N=93) = 0.15, p = .701), ϕ = 0.04.)
3.3 Discussion
Study 2 replicated the findings of Study 1 in a different setting. First, real monetary incentives were provided. Second, participants had real competitors and made actual decisions given the outcomes of earlier draws. Thus, the same findings were obtained for a situation wherein decision making is more consequential and complicated.
We have explored decision making in games without replacement. In the next study, we change a game type, from without replacement to with replacement. This aims to test whether preference for playing order differs depending on with or without replacement.
4 Study 3: A game with replacement
Unlike our previous studies, Study 3 tests preference for play order in the game of rolling dice, a typical game with replacement. Participants were asked to imagine that six people including themselves would roll the dice once and that winning or losing some money depends on the number they get. This game with replacement is simple and easy for estimating the winning chance because the previous results do not affect the later one (i.e., the dice have no memory). Given the transparency of the game, we expect that ambiguity aversion, uncontrollability aversion, and probability misestimation hardly cause biases. However, uncertainty aversion would remain because the outcomes of dice rolling are still probabilistic. Consequently, participants in the dice game would still favor early rolls as in games without replacements. Furthermore, this tendency would not be moderated by game valence in contrast to games without replacement because probability misestimation is less likely to occur.
4.1 Method
A total of 105 university students (48.6% females, M age = 22.4) participated in a one-way between-subjects design study that manipulated game valence (gain vs. loss vs. neutral). The sample size meets a minimum of 17 participants per cell for a power of .80, according to the effect size about playing order of Study 1 (Cohen’s d = 1.0). Participants were asked to imagine that six players including themselves were taking turns rolling dice and that a player gains or loses $10 depending on the outcome number.Footnote 6 In the gain condition, a player wins if the number is 1, 2, 3, or 4 but loses if it is 5 or 6 (66.7% chance of winning). In the loss condition, the winning number is 1 or 2 and the others are losing numbers (33.3% chance of winning). In the neutral condition, the winning number is 1, 2, or 3 and the others are losing (50% chance of winning).
Participants indicated their preferred rolling order and wrote down the reasons for their choices. They then estimated the winning probability on a sliding bar ranging from 0 to 100% if they roll dice first, third, and last. Lastly, we asked them whether the rolling order changes the winning probability and removed 12 participants (58.3% females, M age = 22.3) who said yes, apparently because they failed to understand that the dice game was a replacement game or they did not pay enough attention to the study. Thus, 93 participants remained.
4.2 Results and discussion
Preference for play order
As expected, a major portion of participants favored early rolls (Figure 5). Overall, 53.8% chose the
first roll and 66.7% chose the first or second roll combined. The overall
mean of preferred order was 2.23 that was significantly lower than the
median of 3.5 (t(92) = 7.33, p <
.001, d = 0.76). We also performed a one-way ANOVA on
preferred order and found no significant difference across the three valence
conditions (M gain = 1.97 vs.
M neutral = 2.30 vs.
M loss = 2.39; F(2, 90) =
0.55, p = .579, ![]() = 0.01).
Similarly, the percentage of participants who chose the first roll did not
differ across the conditions (gain game = 67%, neutral game = 50%, loss game
= 46%; χ2(2, N=93) = 3.10,
p = .213, ϕ = 0.18). That is, in a game with
replacement, the tendency to favor early rolls was not influenced by game
valence.
= 0.01).
Similarly, the percentage of participants who chose the first roll did not
differ across the conditions (gain game = 67%, neutral game = 50%, loss game
= 46%; χ2(2, N=93) = 3.10,
p = .213, ϕ = 0.18). That is, in a game with
replacement, the tendency to favor early rolls was not influenced by game
valence.

Figure 5: Distributions of rolling order preference in Study 3.
However, this effect might occur because of misestimation of the winning
probability, that is, early rolls are perceived as having higher winning
probabilities than late rolls, rather than because of uncertainty aversion.
To test this account, we analyzed participants’ estimates of winning
probability with a 3(game valence: gain vs. neutral vs. loss) x 3(roll
order: first vs. third vs. last) mixed ANOVA. The results showed that only
the main effect of game valence was significant
(M gain = .65 vs.
M neutral = .46 vs.
M loss = .32; F(2, 90) =
129.08, p < .001, ![]() = 0.74).
Neither the main effect of roll order (M first =
.48 vs. M third = .49 vs.
M last = .47; F(2, 180) =
2.27, p = .106,
= 0.74).
Neither the main effect of roll order (M first =
.48 vs. M third = .49 vs.
M last = .47; F(2, 180) =
2.27, p = .106, ![]() = 0.03)
nor the interaction, (F(4, 180) = 1.50, p
= .203,
= 0.03)
nor the interaction, (F(4, 180) = 1.50, p
= .203, ![]() = 0.03) was significant.
This result implies that participants understood the winning probability
correctly and recognized that the rolling order did not induce any change in
the winning probability in all three conditions. This finding also implies
that participants favor early rolls even though they were aware of the lack
of a relationship between rolling order and winning probabilities. In
summary, early rolls are preferred to later ones even in a game with
replacement and this effect is not related with probability
misestimation.
= 0.03) was significant.
This result implies that participants understood the winning probability
correctly and recognized that the rolling order did not induce any change in
the winning probability in all three conditions. This finding also implies
that participants favor early rolls even though they were aware of the lack
of a relationship between rolling order and winning probabilities. In
summary, early rolls are preferred to later ones even in a game with
replacement and this effect is not related with probability
misestimation.
Reasons for order preference
Similar with Study 1, two judges categorized the stated reasons for preference into three types of motivational factors and four types of probability estimation. Table 4 presents the results. First, a multiple regressionFootnote 7 was conducted to test the relationship between each reason and preferred order. The results showed that only uncertainty aversion was significant, β= –.39, t(86) = 3.81, p < .001, thereby indicating that uncertainty aversion alone affects rolling order preference in games with replacement. This outcome is consistent with our expectation that the simplicity of games with replacement lessens probability misestimation and aversion to ambiguity and uncontrollability. Second, the third column revealed that a major portion of participants mentioned uncertainty aversion (43%) and no relationship between rolling order and winning probabilities (53%) as the reasons for their choices. By contrast, only a few mentioned ambiguity aversion, uncontrollability aversion, and early/middle/late draw advantage. This finding is in line with the multiple regression results. For more detailed analyses, a series of chi-square tests examined whether a certain reason is associated with game valence only for “uncertainty aversion” and “order is not related” statements that satisfied the assumptions for chi-square tests. The analyses revealed that both reasons were mentioned with a similar frequency across all the game valence (χ2(2, N=93) < 3.21, p > .201, ϕ < 0.19). These chi-square results generally support our contention that probability misestimation hardly occurs in games with replacement.
Table 4: Stated reasons for preferred play order as a function of game valence in Study 3.
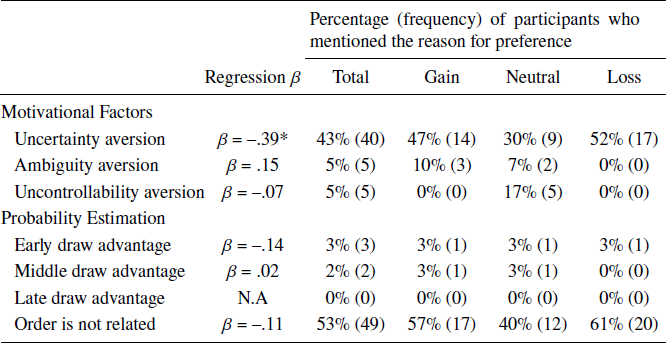
Note. Regression betas are standardized beta coefficients from a multiple regression analysis that tests the relationship between each type of reason and preferred order. The number of participants is in parentheses.
* p < .05.
5 General Discussion
The results of the three studies reveal biases in preference for playing order in the context of a game with and without replacement. Both motivational forces and biased probability perceptions affect these preferences. For games without replacement, Studies 1 and 2 show that early draws were preferred and this tendency was related to the motivational factors such as uncertainty, ambiguity, and uncontrollability aversions. Further, such a preference was modified according to game valence. A gain game strengthened the tendency to prefer earlier draws, whereas a loss game weakened it. This moderating effect was related to the misestimation of winning probability. However, game valence did not affect preference for early draws in games with replacement (Study 3), which implies that uncertainty aversion that is not related to probability estimation is a main motivational factor that leads people to favor early draws.
Understanding decision making in games with and without replacement provides insight into how people make decisions under uncertainty. Research on human decision making has used various types of games and gambles. For example, Reference EllsbergEllsberg (1961) has demonstrated ambiguity aversion by comparing games in which the exact numbers of balls of different colors are known versus unknown. Reference Bar-HillelBar-Hillel (1973) has devised gambles consisting of compounds of simple gambles to present the conjunction fallacy. However, little attention has been directed at decision making biases in games with and without replacement. In particular, examining the judgment biases in a no-replacement game expands the scope of the research on decision under uncertainty. Such a game allows for the winning probability to be changed by the outcomes of earlier draws and further complicates the probability estimation. This characteristic is in contrast to a typical two-gamble choice setting with a simple one-shot decision without co-participants.
This study demonstrates that the factors driving the biased order preferences are both motivational and cognitive. Previous research on decision biases has focused mostly on either motivational forces or cognitive forces. For example, Reference Kahneman and TverskyKahneman and Tversky (1982) have differentiated between errors of comprehension and errors of application. That is, biases are ascribed to either an incorrect understanding of a decision problem (errors of comprehension) or incorrect decisions despite a correct understanding of a problem (errors of application). This work suggests that both comprehension and application errors affect the draw order preference, specifically in the no-replacement game context.
The results of this study provide further insights into human decision making and behavior in situations where people compete for limited resources and opportunities, such as gambling and investment decisions. For example, this work contributes to understanding people’s behavior when buying apartments in South Korea. The funding for building large apartment complexes in South Korea is unique. A construction company pre-sells the rights of residency in new apartments before construction begins. The specific apartment that each buyer receives in a housing complex is decided by lottery when the construction is nearly complete. The interesting point is that the apartments are not equally desirable. The apartments located on higher floors and facing south represent “premium housing” and are strongly preferred to ones located on lower floors and facing north. Thus, premium housing is similar to the winning balls in games without replacement. Like a no-replacement game, once a specific apartment is picked by a buyer, it cannot be returned to the lottery. Although the drawing order makes no difference in determining how likely the home buyer will receive a desirable apartment, South Koreans line up several hours before the lottery starts to draw lots as early as possible.
Preference for playing order in a game without replacement can also be observed in gambling involving no replacement. One example is the game of blackjack, in which whether the players’ win or lose is determined by the cards they receive. In blackjack, players receive two cards from a deck of shuffled cards, and the drawn cards are not replaced until the next shuffle. Therefore, this situation is similar to a game without replacement. Our theory predicts that people prefer to draw early (late) when the remaining cards in the deck are more (less) favorable. People (N = 182) who were familiar with the blackjack game participated in a short survey. Participants were asked to imagine that they were at a blackjack table where several plays had already been made after multiple decks of cards had been shuffled and there would be several more plays until the next shuffle. In the gain valence condition, participants were informed that many favorable cards (10, J, Q, K, and A) had not been drawn yet (i.e., a higher chance of receiving favorable cards). In the loss valence condition, participants were informed that many favorable cards had already been drawn (i.e., a lower chance of receiving favorable cards). The results showed that the average playing position was significantly earlier in the gain condition than in the loss condition (M gain = 2.71 vs. M loss = 3.07; F(1, 180) = .035, d = .31). This result indicated that the valence of a game also affected the preference for playing order in a gambling situation.
Despite these implications, the current research has limitations that future research can improve. First, the three empirical studies examined the hypotheses in the game context. Showing the effects only in a particular situation can undermines the external validity of scientific research. Future research may test the hypotheses in real life contexts other than games to assess the robustness of the effect. Second, the method for identifying the underlying mechanisms lacked rigorousness, because we relied only on the analyses of the open-ended questions asking the reasons for choices. Future research may elaborate more on the study design to find evidence for the proposed mechanism. For example, highly optimistic people are less ambiguity averse than less optimistic people (Reference PulfordPulford, 2009). If future research measures optimism and finds the relation between optimism and preference for early draws, then it may corroborate our contention. Third, the sample size of Study 3 (games with replacement) was relatively small despite being larger than the minimum requirement. One may argue that the small sample size caused the nonsignificant effect of game valence. Thus, reexamining the effect of game valence with additional samples to obtain reliable results would be worthwhile.
Another avenue for future research is to investigate the potential moderating factors that may strengthen or weaken the biases. Individual difference variables worth exploring include rationality, the need for cognition, numeracy, and statistical training (Reference Cacioppo and PettyCacioppo & Petty, 1982; Reference Peters, Västfjäll, Slovic, Mertz, Mazzocco and DickertPeters et al., 2006; Reference Stanovich and WestStanovich & West, 2008). For example, the bias exhibited by a person with a strong need for cognition may stem from an error of application, whereas the counterpart exhibited by a person with a weak need for cognition is likely to stem from an error of comprehension. Additional research can focus on ways to reduce judgment biases. The effective bias-reducing methods can also depend on individual characteristics such as their capabilities and traits. For instance, methods to attenuate motivational forces such as uncertainty, ambiguity, and uncontrollability aversion would be useful for persons who score low on rationality measures, whereas methods to reduce probability misestimation should be effective for persons who score high on such measures.
Appendix A: Separate analyses of two games in Study 2
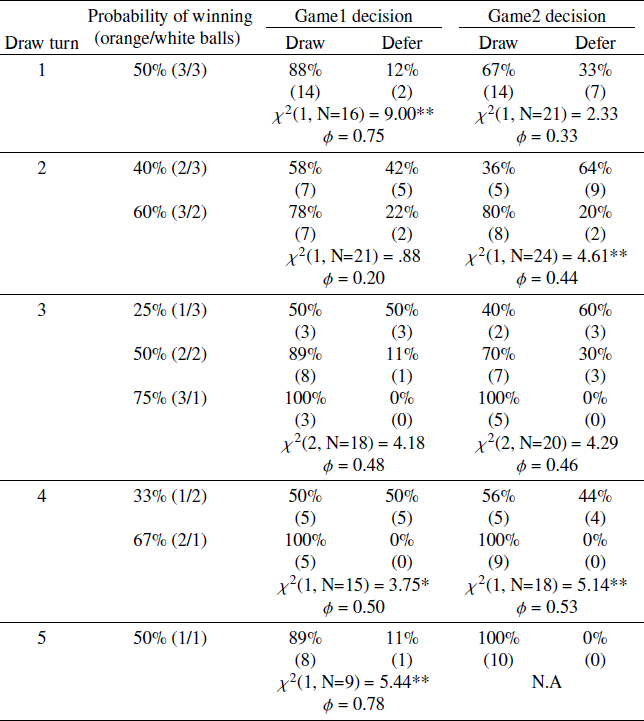
Note: Overall, the pattern of each game was similar to the aggregate data analyses reported in the article. However, some tests results were not significant. This is due to a lack of statistical power with a smaller sample size when the data are separated into two games. Moreover, nearly all the tests violated the assumption of the chi-square test, which requires a minimum of five observations per cell. It is noteworthy that all the effect sizes were greater than 0.20.
* p < .10
** p < .05.












