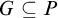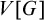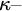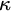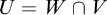1 Introduction
Let P be a forcing notion and
![]() $G\subseteq P$
its generic subset. Suppose that we have in
$G\subseteq P$
its generic subset. Suppose that we have in
![]() $V[G]$
a
$V[G]$
a
![]() $\kappa{-}$
complete ultrafilterFootnote
1
,
Footnote
2
W over
$\kappa{-}$
complete ultrafilterFootnote
1
,
Footnote
2
W over
![]() $\kappa $
. Set
$\kappa $
. Set
![]() $U=W\cap V$
.
$U=W\cap V$
.
We will deal here with the following three basic questions:
-
1. Is U in V?
-
2. Suppose that
 $U \in V$
. How many extensions of U to
$U \in V$
. How many extensions of U to
 $\kappa{-}$
complete ultrafilters do we have in
$\kappa{-}$
complete ultrafilters do we have in
 $V[G]$
?
$V[G]$
? -
3. What is
 $j_W\upharpoonright V$
?
$j_W\upharpoonright V$
?
Note that it is possible that U not in V and even
![]() $\kappa $
is not a measurable in V. The result is due to Kunen—just start with measurable cardinal
$\kappa $
is not a measurable in V. The result is due to Kunen—just start with measurable cardinal
![]() $\kappa $
and iterate with Easton support at each inaccessible the forcing of Suslin tree followed by adding a branch to it. At
$\kappa $
and iterate with Easton support at each inaccessible the forcing of Suslin tree followed by adding a branch to it. At
![]() $\kappa $
itself add only a Suslin tree. Denote the resulting extension by
$\kappa $
itself add only a Suslin tree. Denote the resulting extension by
![]() $V_1$
. Clearly,
$V_1$
. Clearly,
![]() $\kappa $
is not a measurable in
$\kappa $
is not a measurable in
![]() $V_1$
. However, after a further forcing which adds a branch to the Suslin tree it will be a measurable cardinal back.
$V_1$
. However, after a further forcing which adds a branch to the Suslin tree it will be a measurable cardinal back.
Hamkins [Reference Hamkins10] introduced a gap forcingFootnote
3
and showed that if P is such a forcing with a gap below
![]() $\kappa $
, then
$\kappa $
, then
![]() $U\in V$
and
$U\in V$
and
![]() $j_W\upharpoonright V$
is definable in V.Footnote
4
$j_W\upharpoonright V$
is definable in V.Footnote
4
Assume that there is no inner model with a Woodin cardinal. Then by the celebrated results of Jensen and Steel [Reference Jensen and Steel11], the core model
![]() $\mathcal K$
exists and then by Schindler [Reference Schindler17],
$\mathcal K$
exists and then by Schindler [Reference Schindler17],
![]() $j_W\upharpoonright V$
is an iterated ultrapower of
$j_W\upharpoonright V$
is an iterated ultrapower of
![]() $\mathcal K$
by its extenders.
$\mathcal K$
by its extenders.
Note that
![]() $j_W\upharpoonright V$
need not be definable in V already if there is a measurable limit of measurables.
$j_W\upharpoonright V$
need not be definable in V already if there is a measurable limit of measurables.
Namely, we add first a Cohen real r to V, and then, iterate with Easton support (as in [Reference Gitik and Foreman7, Section 6]) the following forcing
![]() $Q_\nu $
below such measurable
$Q_\nu $
below such measurable
![]() $\kappa $
: for every
$\kappa $
: for every
![]() $\nu <\kappa $
, let
$\nu <\kappa $
, let
![]() ${\langle } \nu _n \mid n<\omega {\rangle }$
be the first
${\langle } \nu _n \mid n<\omega {\rangle }$
be the first
![]() $\omega $
many measurable cardinals above
$\omega $
many measurable cardinals above
![]() $\nu $
. Let
$\nu $
. Let
![]() $Q_\nu $
be the Magidor iteration [Reference Magidor15] of Prikry forcings which change cofinality of
$Q_\nu $
be the Magidor iteration [Reference Magidor15] of Prikry forcings which change cofinality of
![]() $\nu _n$
, but only if
$\nu _n$
, but only if
![]() $r(n)=0$
.
$r(n)=0$
.
The purpose of the present paper is to extend the Hamkins approach to a wider class of forcing notions.
Our prime interest will be in Prikry type forcing notions.
Magidor in his celebrated paper [Reference Magidor15] showed that the first measurable cardinal can be a strongly compact.
The method was to use the Magidor (full support) iteration of Prikry forcings and this way to destroy measurable cardinals.
Another approach was used by Kimchi and Magidor (see [Reference Apter and Cummings1])—they destroyed measurability by adding a non-reflecting stationary subset to measurables. The iteration used was the Easton support iteration. Note that this type of forcing falls into the Hamkins schema.
Here we will deal with this type of iterated forcing notions and in addition to Easton and full supports, we will consider also a nonstationary support.
2 Some sufficient conditions
Proposition 2.1. Let
![]() $V[G]$
is a generic extension of V by a forcing P.
$V[G]$
is a generic extension of V by a forcing P.
Suppose that
![]() $\kappa $
is a measurable cardinal in
$\kappa $
is a measurable cardinal in
![]() $V[G]$
and W is a
$V[G]$
and W is a
![]() $\kappa{-}$
complete ultrafilter over
$\kappa{-}$
complete ultrafilter over
![]() $\kappa $
.
$\kappa $
.
Let
![]() $U=V\cap W$
. Then
$U=V\cap W$
. Then
![]() $U \in V$
if the following hold:
$U \in V$
if the following hold:
-
1. all cardinals of V in the interval
 $[\kappa , (2^\kappa )^V]$
are preserved,
$[\kappa , (2^\kappa )^V]$
are preserved, -
2. no fresh subsets are added to a cardinal
 $\lambda $
,Footnote
5
$\lambda $
,Footnote
5
 $ \kappa \leq \lambda \leq (2^\kappa )^V$
.
$ \kappa \leq \lambda \leq (2^\kappa )^V$
.
Proof Let
![]() $\delta =(2^\kappa )^V$
. For every
$\delta =(2^\kappa )^V$
. For every
![]() $f\!:\delta \leftrightarrow \mathcal P(\kappa )$
in V set
$f\!:\delta \leftrightarrow \mathcal P(\kappa )$
in V set
Clearly, if for some such
![]() $f, X_f \in V$
, then also
$f, X_f \in V$
, then also
![]() $U \in V$
.
$U \in V$
.
Suppose that
![]() $X_f $
never is in
$X_f $
never is in
![]() $ V$
.
$ V$
.
Define, for such f’s,
![]() $\alpha _f\leq \delta $
be the least
$\alpha _f\leq \delta $
be the least
![]() $\alpha $
such that
$\alpha $
such that
![]() $X_f \cap \alpha \not \in V$
.
$X_f \cap \alpha \not \in V$
.
Set
Claim.
![]() $\alpha ^*<\kappa $
.
$\alpha ^*<\kappa $
.
Proof Suppose otherwise. By the first two assumption of the theorem,
![]() $\alpha ^*$
cannot be a cardinal in the interval
$\alpha ^*$
cannot be a cardinal in the interval
![]() $[\kappa ,\delta ]$
.
$[\kappa ,\delta ]$
.
So, there is a cardinal
![]() $\eta , \kappa \leq \eta <\delta $
such that
$\eta , \kappa \leq \eta <\delta $
such that
![]() $\eta <\alpha ^*<\eta ^+$
.
$\eta <\alpha ^*<\eta ^+$
.
Pick, in V,
![]() $g:\delta \leftrightarrow \delta $
such that
$g:\delta \leftrightarrow \delta $
such that
![]() $g\upharpoonright \eta $
maps
$g\upharpoonright \eta $
maps
![]() $\eta $
onto
$\eta $
onto
![]() $\alpha ^*$
.
$\alpha ^*$
.
Then
![]() $X_{f\circ g}\cap \eta \not \in V$
, and hence,
$X_{f\circ g}\cap \eta \not \in V$
, and hence,
![]() $\alpha _{f\circ g}\leq \eta $
.
$\alpha _{f\circ g}\leq \eta $
.
But
![]() $\eta <\alpha ^*\leq \alpha _{f\circ g}$
. Contradiction.
$\eta <\alpha ^*\leq \alpha _{f\circ g}$
. Contradiction.
So,
![]() $\alpha ^*<\kappa $
. Fix
$\alpha ^*<\kappa $
. Fix
![]() $f:\delta \leftrightarrow \mathcal P(\kappa )$
with
$f:\delta \leftrightarrow \mathcal P(\kappa )$
with
![]() $\alpha _f=\alpha ^*$
. So, the set
$\alpha _f=\alpha ^*$
. So, the set
Work in
![]() $V[G]$
. Set
$V[G]$
. Set
![]() $A_0=\bigcap _{\alpha \in X_f \cap \alpha ^*} f(\alpha )$
and
$A_0=\bigcap _{\alpha \in X_f \cap \alpha ^*} f(\alpha )$
and
![]() $A_1=\bigcap _{\alpha \in \alpha ^* \setminus X_f } \kappa \setminus f(\alpha )$
. Both sets are in W due to
$A_1=\bigcap _{\alpha \in \alpha ^* \setminus X_f } \kappa \setminus f(\alpha )$
. Both sets are in W due to
![]() $\kappa{-}$
completeness. So,
$\kappa{-}$
completeness. So,
![]() $A=A_0\cap A_1 \in W$
, as well. Pick
$A=A_0\cap A_1 \in W$
, as well. Pick
![]() $\zeta \in A_0\cap A_1$
.
$\zeta \in A_0\cap A_1$
.
Then
but clearly,
![]() $\{\alpha <\alpha ^* \mid \zeta \in f(\alpha )\}$
is in V.
$\{\alpha <\alpha ^* \mid \zeta \in f(\alpha )\}$
is in V.
So,
![]() $X_f \cap \alpha ^* \in V$
. Contradiction.
$X_f \cap \alpha ^* \in V$
. Contradiction.
Remark 2.2. 1. By Hamkins it is possible to collapse cardinals without adding new fresh subsets to them. Just add a Cohen real and then do the collapse.
2. The conditions of the theorem are not necessary by no means. For example, starting with a supercompact cardinal
![]() $\kappa $
, one can collapse
$\kappa $
, one can collapse
![]() $\kappa ^+$
preserving supercompactness (after making an appropriate preparation) and still it will be possible to find W a normal measure in the extension such that
$\kappa ^+$
preserving supercompactness (after making an appropriate preparation) and still it will be possible to find W a normal measure in the extension such that
![]() $W\cap V \in V$
.
$W\cap V \in V$
.
3. Originally we used a stronger assumption which suffices for the further applications:
every bounded subset of
![]() $\kappa $
is added by some subforcing
$\kappa $
is added by some subforcing
![]() $R\lessdot P$
of cardinality
$R\lessdot P$
of cardinality
![]() $<\kappa $
.
$<\kappa $
.
Magidor pointed out that it is possible to drop it. The final lines of the argument are due to him.
4. Note that if
![]() $\delta $
is the least regular cardinal of V which has a new subset in
$\delta $
is the least regular cardinal of V which has a new subset in
![]() $V[G]$
, then every cardinal
$V[G]$
, then every cardinal
![]() $\tau $
of cofinality
$\tau $
of cofinality
![]() $\delta $
in V will have a fresh subset. In particular our assumption implies that there is no such
$\delta $
in V will have a fresh subset. In particular our assumption implies that there is no such
![]() $\tau $
’s in the interval
$\tau $
’s in the interval
![]() $[\kappa , (2^\kappa )^V]$
.
$[\kappa , (2^\kappa )^V]$
.
Our main interest will be in the case
![]() $2^\kappa =\kappa ^+$
.
$2^\kappa =\kappa ^+$
.
Proposition 2.3. Let U be a
![]() $\kappa{-}$
complete ultrafilter over
$\kappa{-}$
complete ultrafilter over
![]() $\kappa $
in V. Let
$\kappa $
in V. Let
![]() $V[G]$
be a generic extension of V by a forcing P. Suppose that for every
$V[G]$
be a generic extension of V by a forcing P. Suppose that for every
![]() $A\subseteq \kappa $
in
$A\subseteq \kappa $
in
![]() $V[G]$
there is
$V[G]$
there is
![]() $p \in G$
such that
$p \in G$
such that
![]() .
.
Suppose that in
![]() $V[G]$
there is a
$V[G]$
there is a
![]() $\kappa{-}$
complete ultrafilter which extends U.
$\kappa{-}$
complete ultrafilter which extends U.
Then, in
![]() $V[G]$
,
$V[G]$
,
![]() is a
is a
![]() $\kappa{-}$
complete ultrafilter which extends U. Moreover,
$\kappa{-}$
complete ultrafilter which extends U. Moreover,
![]() $U^*$
is the unique
$U^*$
is the unique
![]() $\kappa{-}$
complete ultrafilter which extends U.
$\kappa{-}$
complete ultrafilter which extends U.
Proof Suppose that W is a
![]() $\kappa{-}$
complete ultrafilter which extends U in
$\kappa{-}$
complete ultrafilter which extends U in
![]() $V[G]$
. Clearly, then
$V[G]$
. Clearly, then
![]() $W\cap V=U$
.
$W\cap V=U$
.
Also,
Consider
![]() $j_W:V[G]\to M_W$
. Then,
$j_W:V[G]\to M_W$
. Then,
![]() $M_W=M[j_W(G)]$
.Footnote
6
$M_W=M[j_W(G)]$
.Footnote
6
Define
![]() $k:M_U \to M$
by setting
$k:M_U \to M$
by setting
![]() $k([f]_U)=[f]_W$
.
$k([f]_U)=[f]_W$
.
Lemma 2.4. k is an elementary embedding.
Proof Clearly, k respects
![]() $\in $
and
$\in $
and
![]() $=$
.
$=$
.
Let
![]() $\varphi (v_1,\ldots ,v_n)$
be a formula and
$\varphi (v_1,\ldots ,v_n)$
be a formula and
![]() $f_1,\ldots ,f_n$
be functions in V from
$f_1,\ldots ,f_n$
be functions in V from
![]() $\kappa $
.
$\kappa $
.
Suppose that
Then
So,
Hence,
Lemma 2.5.
![]() $j_W\upharpoonright V= k\circ j_U$
.
$j_W\upharpoonright V= k\circ j_U$
.
Proof Let
![]() $x \in V$
. Then
$x \in V$
. Then
![]() $j_W(x) = [c_x]_W$
, where
$j_W(x) = [c_x]_W$
, where
![]() $c_x$
is the constant function with value x. So,
$c_x$
is the constant function with value x. So,
and we are done.
Now, let A be subset of
![]() $ \kappa $
in
$ \kappa $
in
![]() $V[G]$
. Then, there is
$V[G]$
. Then, there is
![]() $p \in G$
such that
$p \in G$
such that
Suppose that
Apply k.
Then
Recall that
![]() $M_W=M[j_W(G)]$
. So,
$M_W=M[j_W(G)]$
. So,
![]() $j_W(p)\in j_W(G)$
, and then, in
$j_W(p)\in j_W(G)$
, and then, in
![]() $M_W$
,
$M_W$
,
Hence,
![]() $A \in W$
.
$A \in W$
.
This shows that
![]() $U^*=W$
, and we are done.
$U^*=W$
, and we are done.
Let us show the following:
Proposition 2.6. Let U be a
![]() $\kappa{-}$
complete ultrafilter over
$\kappa{-}$
complete ultrafilter over
![]() $\kappa $
in V. Let
$\kappa $
in V. Let
![]() $V[G]$
be a generic extension of V by a forcing P. Then the following two conditions are equivalent:
$V[G]$
be a generic extension of V by a forcing P. Then the following two conditions are equivalent:
-
1. for every
 $A\subseteq \kappa $
in
$A\subseteq \kappa $
in
 $V[G]$
there is
$V[G]$
there is
 $p \in G$
such that
$p \in G$
such that

-
2. U generates an ultrafilter in
 $V[G]$
, i.e.,
$V[G]$
, i.e.,
 $\{ A\subseteq \kappa \mid \exists B\in U (B\subseteq A)\}$
is an ultrafilter in
$\{ A\subseteq \kappa \mid \exists B\in U (B\subseteq A)\}$
is an ultrafilter in
 $V[G]$
.
$V[G]$
.
Proof
![]() $(1)\Rightarrow (2)$
.
$(1)\Rightarrow (2)$
.
Let
![]() $A\subseteq \kappa $
in
$A\subseteq \kappa $
in
![]() $V[G]$
and suppose that there is
$V[G]$
and suppose that there is
![]() $p \in G$
such that
$p \in G$
such that
. Let, in V,
Then,
![]() $B\in U$
, by the Los Theorem. In addition,
$B\in U$
, by the Los Theorem. In addition,
![]() $B\subseteq A$
, since
$B\subseteq A$
, since
![]() $p\in G$
.
$p\in G$
.
![]() $(2)\Rightarrow (1)$
. Let
$(2)\Rightarrow (1)$
. Let
![]() $A\subseteq \kappa $
in
$A\subseteq \kappa $
in
![]() $V[G]$
and suppose that
$V[G]$
and suppose that
![]() $A\supseteq B$
, for some
$A\supseteq B$
, for some
![]() $B\in U$
.
$B\in U$
.
Pick
![]() $p\in G$
such that
$p\in G$
such that
. Then, in
![]() $M_U$
,
$M_U$
,
By the assumption, U generates an ultrafilter, hence for every
![]() $A\subseteq \kappa $
in
$A\subseteq \kappa $
in
![]() $V[G]$
, either A or
$V[G]$
, either A or
![]() $\kappa \setminus A$
contain an element of U. So, (1) holds.
$\kappa \setminus A$
contain an element of U. So, (1) holds.
Clearly, the condition (2) of the proposition provides the uniqueness.
Concerning the completeness of U in extensions, we do not know the following:
Question. Suppose that U is a
![]() $\kappa{-}$
complete (or normal) ultrafilter over
$\kappa{-}$
complete (or normal) ultrafilter over
![]() $\kappa $
in V. Let
$\kappa $
in V. Let
![]() $V[G]$
be a generic extension of V by a forcing P. Is it possible that U generates an ultrafilter in
$V[G]$
be a generic extension of V by a forcing P. Is it possible that U generates an ultrafilter in
![]() $V[G]$
which is not
$V[G]$
which is not
![]() $\sigma{-}$
complete?
$\sigma{-}$
complete?
Assuming some forms of covering it is possible to argue that U must be complete in the extension. Let us state a simplest form of this type of arguments.
Proposition 2.7. Assume
![]() $2^\kappa =\kappa ^+$
. Let U be a
$2^\kappa =\kappa ^+$
. Let U be a
![]() $\kappa{-}$
complete filter over
$\kappa{-}$
complete filter over
![]() $\kappa $
in V. Let
$\kappa $
in V. Let
![]() $V[G]$
be a generic extension of V by a forcing P. Suppose that both
$V[G]$
be a generic extension of V by a forcing P. Suppose that both
![]() $\kappa $
and
$\kappa $
and
![]() $\kappa ^+$
remain regular in
$\kappa ^+$
remain regular in
![]() $V[G]$
.
$V[G]$
.
Then the filter generated by U remains
![]() $\kappa{-}$
complete in
$\kappa{-}$
complete in
![]() $V[G]$
.Footnote
7
$V[G]$
.Footnote
7
In addition, if U was normal in V, then it remains
![]() $\kappa ^+$
-directed in
$\kappa ^+$
-directed in
![]() $V[G]$
.Footnote
8
$V[G]$
.Footnote
8
Proof Let us deal with
![]() $\kappa{-}$
completeness. Directedness is similar.
$\kappa{-}$
completeness. Directedness is similar.
We assume
![]() $2^\kappa =\kappa ^+$
holds in V. Hence,
$2^\kappa =\kappa ^+$
holds in V. Hence,
![]() $U=\{B_\alpha \mid \alpha <\kappa ^+\}$
.
$U=\{B_\alpha \mid \alpha <\kappa ^+\}$
.
Now let
![]() $a\subseteq \kappa ^+, |a|<\kappa $
in
$a\subseteq \kappa ^+, |a|<\kappa $
in
![]() $V[G]$
. Pick
$V[G]$
. Pick
![]() $f:\kappa \to \sup (a)+1$
in V to be onto. Then, due to regularity of
$f:\kappa \to \sup (a)+1$
in V to be onto. Then, due to regularity of
![]() $\kappa $
, there is
$\kappa $
, there is
![]() $\delta <\kappa $
, such that
$\delta <\kappa $
, such that
![]() $f"\delta \supseteq a$
. Set
$f"\delta \supseteq a$
. Set
![]() $b=f"\delta $
. Clearly,
$b=f"\delta $
. Clearly,
![]() $b\in V$
and
$b\in V$
and
![]() $|b|<\kappa $
there. Then
$|b|<\kappa $
there. Then
The following is a slight generalization of 2.3:
Proposition 2.8. Let U be a
![]() $\kappa{-}$
complete ultrafilter over
$\kappa{-}$
complete ultrafilter over
![]() $\kappa $
in V. Suppose that
$\kappa $
in V. Suppose that
![]() $i:V\to N$
is an elementary embedding such that for some
$i:V\to N$
is an elementary embedding such that for some
![]() $\delta $
,
$\delta $
,
![]() $U=\{ X \subseteq \kappa \mid \delta \in i(X)\}$
. Let
$U=\{ X \subseteq \kappa \mid \delta \in i(X)\}$
. Let
![]() $V[G]$
be a generic extension of V by a forcing P. Suppose that for every
$V[G]$
be a generic extension of V by a forcing P. Suppose that for every
![]() $A\subseteq \kappa $
in
$A\subseteq \kappa $
in
![]() $V[G]$
there is
$V[G]$
there is
![]() $p \in G$
such that
$p \in G$
such that
![]() .
.
Then U generates an ultrafilter in
![]() $V[G]$
.
$V[G]$
.
Proof Define
![]() $\sigma :M_U \to N$
by setting
$\sigma :M_U \to N$
by setting
![]() $\sigma ([f]_U)=i(f)(\delta )$
.
$\sigma ([f]_U)=i(f)(\delta )$
.
Then
![]() $i=\sigma \circ j_U$
and
$i=\sigma \circ j_U$
and
![]() $\sigma ([id]_U)=\delta $
. So, by elementarity of
$\sigma ([id]_U)=\delta $
. So, by elementarity of
![]() $\sigma $
,
$\sigma $
,
for every
![]() $p \in P$
and a name
$p \in P$
and a name
. In particular, the assumption of the proposition implies that for every
![]() $A\subseteq \kappa $
in
$A\subseteq \kappa $
in
![]() $V[G]$
there is
$V[G]$
there is
![]() $p \in G$
such that
$p \in G$
such that
. Now the conclusion follows by 2.6.
It is tempting to try to apply the above proposition together with Friedman, Magidor [Reference Friedman and Magidor6] in order to obtain
![]() $2^\kappa>\kappa ^+$
but there is a normal ultrafilter generated by
$2^\kappa>\kappa ^+$
but there is a normal ultrafilter generated by
![]() $\kappa ^+$
many sets. The problem is that a non-trivial forcing should be made over
$\kappa ^+$
many sets. The problem is that a non-trivial forcing should be made over
![]() $\kappa $
itself and, then statements of the form
$\kappa $
itself and, then statements of the form
![]() are decided by
are decided by
![]() $p^\frown j(p)\setminus \kappa +1$
and not by
$p^\frown j(p)\setminus \kappa +1$
and not by
![]() $j(p)$
alone, since in
$j(p)$
alone, since in
![]() $j(p)$
the
$j(p)$
the
![]() $\kappa{-}$
th coordinate is just empty.
$\kappa{-}$
th coordinate is just empty.
We have the following parallel to 2.6 statement for normal ultrafilters:
Proposition 2.9. Let U be a normal ultrafilter over
![]() $\kappa $
in V. Let
$\kappa $
in V. Let
![]() $V[G]$
be a generic extension of V by a forcing P. Then the following two conditions are equivalent:
$V[G]$
be a generic extension of V by a forcing P. Then the following two conditions are equivalent:
-
1. for every regressive function f on
 $\kappa $
in
$\kappa $
in
 $V[G]$
, there is
$V[G]$
, there is
 $p \in G$
such that
$p \in G$
such that

-
2. U generates a normal ultrafilter in
 $V[G]$
, i.e.,
$V[G]$
, i.e.,
 $W=\{ A\subseteq \kappa \mid \exists B\in U (B\subseteq A)\}$
is a normal ultrafilter in
$W=\{ A\subseteq \kappa \mid \exists B\in U (B\subseteq A)\}$
is a normal ultrafilter in
 $V[G]$
.
$V[G]$
.
Proof
![]() $(1)\Rightarrow (2)$
.
$(1)\Rightarrow (2)$
.
Let f be a regressive function on
![]() $\kappa $
in
$\kappa $
in
![]() $V[G]$
and suppose that there is
$V[G]$
and suppose that there is
![]() $p \in G$
such that for some
$p \in G$
such that for some
![]() $\alpha <\kappa $
,
$\alpha <\kappa $
,
. Let, in V,
Then,
![]() $B\in U$
, by the Los Theorem. In addition,
$B\in U$
, by the Los Theorem. In addition,
![]() $B\subseteq \{\nu <\kappa \mid f(\nu )=\alpha \}$
, since
$B\subseteq \{\nu <\kappa \mid f(\nu )=\alpha \}$
, since
![]() $p\in G$
.
$p\in G$
.
![]() $(2)\Rightarrow (1)$
. Let f be a regressive function on
$(2)\Rightarrow (1)$
. Let f be a regressive function on
![]() $\kappa $
in
$\kappa $
in
![]() $V[G]$
and suppose that for some
$V[G]$
and suppose that for some
![]() $\alpha <\kappa $
, the set
$\alpha <\kappa $
, the set
![]() $A=\{\nu <\kappa \mid f(\nu )=\alpha \}\in W$
.
$A=\{\nu <\kappa \mid f(\nu )=\alpha \}\in W$
.
Then,
![]() $A\supseteq B$
, for some
$A\supseteq B$
, for some
![]() $B\in U$
.
$B\in U$
.
Pick
![]() $p\in G$
such that
$p\in G$
such that
. Then, in
![]() $M_U$
,
$M_U$
,
So, (1) holds.
The following question seems natural:
Question. Suppose that U is a normal ultrafilter over
![]() $\kappa $
in V. Let
$\kappa $
in V. Let
![]() $V[G]$
be a generic extension of V by a forcing P. Is it possible that U generates a
$V[G]$
be a generic extension of V by a forcing P. Is it possible that U generates a
![]() $\kappa{-}$
complete ultrafilter in
$\kappa{-}$
complete ultrafilter in
![]() $V[G]$
which is not normal?
$V[G]$
which is not normal?
3 Nonstationary support iteration of Prikry type forcing notions
An iterated forcing with nonstationary support was introduced by Jensen [Reference Beller, Jensen and Welch3] in his famous work on codding the universe by a real, Friedman and Magidor [Reference Friedman and Magidor6] used it to solve a long standing problem on number of normal measures, Ben-Neria [Reference Ben-Neria4], Ben-Neria and Unger [Reference Ben-Neria and Unger5], Apter and Cummings [Reference Apter and Cummings2] found more interesting applications.
Definition 3.1. An iterated forcing notion
![]() $P_\eta $
of length
$P_\eta $
of length
![]() $\eta $
is an iteration with nonstationary support if for every
$\eta $
is an iteration with nonstationary support if for every
![]() $\gamma \leq \eta $
:
$\gamma \leq \eta $
:
-
1. If
 $\gamma $
is not inaccessible, then
$\gamma $
is not inaccessible, then
 $P_\gamma $
is the inverse limit of
$P_\gamma $
is the inverse limit of
 ${\langle } P_\alpha \mid \alpha <\gamma {\rangle }$
.
${\langle } P_\alpha \mid \alpha <\gamma {\rangle }$
. -
2. If
 $\gamma $
is inaccessible, then
$\gamma $
is inaccessible, then
 $P_\gamma $
is the set of conditions in the inverse limit of
$P_\gamma $
is the set of conditions in the inverse limit of
 ${\langle } P_\alpha \mid \alpha <\gamma {\rangle }$
whose support is a nonstationary subset of
${\langle } P_\alpha \mid \alpha <\gamma {\rangle }$
whose support is a nonstationary subset of
 $\gamma $
.
$\gamma $
.
Definition 3.2. A triple
![]() ${\langle } \mathcal P, \leq , \leq ^*{\!\rangle }$
is called a Prikry type forcing notion iff
${\langle } \mathcal P, \leq , \leq ^*{\!\rangle }$
is called a Prikry type forcing notion iff
-
1.
 $\leq , \leq ^*$
are two partial orderings of a set
$\leq , \leq ^*$
are two partial orderings of a set
 $\mathcal P$
.
$\mathcal P$
. -
2.
 $\leq ^*\subseteq \leq $
.
$\leq ^*\subseteq \leq $
. -
3. For every statement
 $\varphi $
of the forcing language
$\varphi $
of the forcing language
 ${\langle } \mathcal P,\leq {\rangle }$
and every
${\langle } \mathcal P,\leq {\rangle }$
and every
 $q \in \mathcal P$
, there is
$q \in \mathcal P$
, there is
 $p\in \mathcal P$
,
$p\in \mathcal P$
,
 $p\geq ^* q$
which decides
$p\geq ^* q$
which decides
 $\varphi $
.
$\varphi $
.
Suppose now that
![]() is a nonstationary support iteration of a Prikry type forcing notions
is a nonstationary support iteration of a Prikry type forcing notions
![]() such that for every
such that for every
![]() $\beta <\kappa $
the following hold:
$\beta <\kappa $
the following hold:
-
1.
 is trivial unless
is trivial unless
 $\beta $
is an inaccessible (or measurable),
$\beta $
is an inaccessible (or measurable), -
2.
 is
is
 $\beta{-}$
strategically closed,
$\beta{-}$
strategically closed, -
3.
 is less than the first inaccessible cardinal above
is less than the first inaccessible cardinal above
 $\beta $
.
$\beta $
.
Two specific forcing notions of this type in which we are interested are
-
A. The Prikry forcing for changing cofinality of a measurable
 $\beta $
to
$\beta $
to
 $\omega $
.
$\omega $
. -
B. The forcing for adding a stationary non-reflecting subset to
 $\beta $
which consists of inaccessible cardinals, for a Mahlo cardinal
$\beta $
which consists of inaccessible cardinals, for a Mahlo cardinal
 $\beta $
.
$\beta $
.In this case
 $\leq =\leq ^*$
.
$\leq =\leq ^*$
.
The basic properties of this type of iterations are identical to the Easton or full support iterations, see for example [Reference Gitik and Foreman7]. We refer to Ben-Neria and Unger [Reference Ben-Neria and Unger5] for iterating Prikry forcings and to Apter and Cummings [Reference Apter and Cummings1] for iterating forcings adding a stationary non-reflecting subset, with non-stationary support.
Suppose that
![]() $\kappa $
is a measurable cardinal and U a normal ultrafilter over
$\kappa $
is a measurable cardinal and U a normal ultrafilter over
![]() $\kappa $
.
$\kappa $
.
Assume that on a set I of
![]() $\beta $
’s in U the forcing
$\beta $
’s in U the forcing
![]() is trivial.
is trivial.
The next lemma follows basically an argument of Friedman and Magidor [Reference Friedman and Magidor6].
Lemma 3.3. Let
![]() $G_\kappa $
be generic subset of
$G_\kappa $
be generic subset of
![]() $P_\kappa $
. Then the set
$P_\kappa $
. Then the set
![]() $H=\{j_U(p)\setminus \kappa \mid p \in G_\kappa \}$
Footnote
9
is
$H=\{j_U(p)\setminus \kappa \mid p \in G_\kappa \}$
Footnote
9
is
![]() $M_U[G_\kappa ]{-}$
generic for
$M_U[G_\kappa ]{-}$
generic for
![]() ${\langle } j_U(P_\kappa )/G_\kappa , \leq ^*{\!\rangle } $
.
${\langle } j_U(P_\kappa )/G_\kappa , \leq ^*{\!\rangle } $
.
Proof Let
![]() be a name which is forced, say by the weakest condition in
be a name which is forced, say by the weakest condition in
![]() $P_\kappa $
, to be a dense open subset of
$P_\kappa $
, to be a dense open subset of
![]() . Pick a function F which represents
. Pick a function F which represents
![]() in the ultrapower
in the ultrapower
![]() $M_U$
.
$M_U$
.
Assume, for simplification of the notation, that for every
![]() $\beta \in I$
,
$\beta \in I$
,
![]() $F(\beta )$
is forced by the weakest condition in
$F(\beta )$
is forced by the weakest condition in
![]() $P_\beta $
to be a dense open subset of
$P_\beta $
to be a dense open subset of
![]() ${\langle } P_\kappa /P_\beta , \leq ^*{\!\rangle }$
.
${\langle } P_\kappa /P_\beta , \leq ^*{\!\rangle }$
.
Fix
![]() $p \in P_\kappa $
. We define by induction a
$p \in P_\kappa $
. We define by induction a
![]() $\leq ^*$
-increasing sequence
$\leq ^*$
-increasing sequence
![]() ${\langle } p_i \mid i<\kappa {\rangle }$
of extensions of p and a decreasing sequence
${\langle } p_i \mid i<\kappa {\rangle }$
of extensions of p and a decreasing sequence
![]() ${\langle } C_i \mid i<\kappa {\rangle } $
of closed unbounded subsets of
${\langle } C_i \mid i<\kappa {\rangle } $
of closed unbounded subsets of
![]() $\kappa $
such that for every
$\kappa $
such that for every
![]() $i<\kappa $
the following hold:
$i<\kappa $
the following hold:
-
1.
 $C_i \cap {\textrm {supp}}(p_i)=\emptyset $
,
$C_i \cap {\textrm {supp}}(p_i)=\emptyset $
, -
2.
 $p\upharpoonright {i} \Vdash p_{i}\setminus {i} \in F({i})$
, if
$p\upharpoonright {i} \Vdash p_{i}\setminus {i} \in F({i})$
, if
 $i \in I\cap \bigcap _{j<i}C_j$
,
$i \in I\cap \bigcap _{j<i}C_j$
, -
3.
 $p_i \upharpoonright {i +1}=p_{i'}\upharpoonright {i+1}$
, for every
$p_i \upharpoonright {i +1}=p_{i'}\upharpoonright {i+1}$
, for every
 $i<i'<\kappa $
.
$i<i'<\kappa $
.
Proceed as follows.
Set
![]() $p_0=p$
. Let
$p_0=p$
. Let
![]() $C_0$
be a club disjoint with
$C_0$
be a club disjoint with
![]() ${\textrm {supp}}(p_0)$
. Set
${\textrm {supp}}(p_0)$
. Set
![]() $i_0=\min (I\cap C_0)$
.
$i_0=\min (I\cap C_0)$
.
For every
![]() $i<i_0$
, set
$i<i_0$
, set
![]() $p_i=p_0$
and
$p_i=p_0$
and
![]() $C_i=C_0$
.
$C_i=C_0$
.
Now let
![]() $p_{i_0}\geq ^* p_0$
be such that
$p_{i_0}\geq ^* p_0$
be such that
![]() $p_{i_0}\upharpoonright i_0=p_0\upharpoonright i_0$
and
$p_{i_0}\upharpoonright i_0=p_0\upharpoonright i_0$
and
![]() $p\upharpoonright i_0 \Vdash p_{i_0}\setminus i_0 \in F(i_0)$
.
$p\upharpoonright i_0 \Vdash p_{i_0}\setminus i_0 \in F(i_0)$
.
Let
![]() $C_{i_0}$
be a proper club subset of
$C_{i_0}$
be a proper club subset of
![]() $C_0$
which is disjoint from
$C_0$
which is disjoint from
![]() ${\textrm {supp}}(p_{i_0})$
.
${\textrm {supp}}(p_{i_0})$
.
Suppose now that
![]() $p_j,C_j$
are defined for every
$p_j,C_j$
are defined for every
![]() $j<i$
. Define
$j<i$
. Define
![]() $p_i$
and
$p_i$
and
![]() $C_i$
. If there is
$C_i$
. If there is
![]() $i'<i$
such that
$i'<i$
such that
![]() $C_{i'}=C_j$
, for every
$C_{i'}=C_j$
, for every
![]() $j, i'\leq j<i$
, then we proceed as above.
$j, i'\leq j<i$
, then we proceed as above.
Suppose that this is not the case. Note that then
![]() $i \not \in {\textrm {supp}}(p_j)$
, for every
$i \not \in {\textrm {supp}}(p_j)$
, for every
![]() $j<i$
.
$j<i$
.
Set
![]() $C_i'=\bigcap _{j<i}C_j$
. There is a
$C_i'=\bigcap _{j<i}C_j$
. There is a
![]() $\leq ^*$
-extension
$\leq ^*$
-extension
![]() of the sequence
of the sequence
![]() ${\langle } p_j \mid j<i{\rangle }$
. Just put together the conditions using the inductive assumption (3) above and the strategic closure. Note that
${\langle } p_j \mid j<i{\rangle }$
. Just put together the conditions using the inductive assumption (3) above and the strategic closure. Note that
![]() $|P_{\xi }|$
is below the first inaccessible above
$|P_{\xi }|$
is below the first inaccessible above
![]() $\xi $
, so we have enough strategic closure in order to proceed. The only problematic coordinate may be i itself, however,
$\xi $
, so we have enough strategic closure in order to proceed. The only problematic coordinate may be i itself, however,
![]() $i \not \in {\textrm {supp}}(p_j)$
, for every
$i \not \in {\textrm {supp}}(p_j)$
, for every
![]() $j<i$
, and so we will have the i-th coordinate just empty.
$j<i$
, and so we will have the i-th coordinate just empty.
This is actually the crucial point in the use of nonstationary support.
Now, if
![]() $i \not \in I$
, then set
$i \not \in I$
, then set
![]() $C_i = C_i'$
and
$C_i = C_i'$
and
![]() $p_i=p_i'$
.
$p_i=p_i'$
.
If
![]() $i \in I$
, we proceed as above using
$i \in I$
, we proceed as above using
![]() $p_i'$
and
$p_i'$
and
![]() $C_i'$
.
$C_i'$
.
This completes the construction.
Now set
![]() $C=\Delta _{i<\kappa }C_i$
and put the sequence
$C=\Delta _{i<\kappa }C_i$
and put the sequence
![]() ${\langle } p_i \mid i<\kappa {\rangle }$
into a single condition
${\langle } p_i \mid i<\kappa {\rangle }$
into a single condition
![]() $p^*$
using the inductive assumption (3) above.
$p^*$
using the inductive assumption (3) above.
Then for every
![]() $i \in C\cap I$
,
$i \in C\cap I$
,
![]() $p\upharpoonright i \Vdash p_i\setminus i+1\leq ^* p^*\setminus i+1.$
$p\upharpoonright i \Vdash p_i\setminus i+1\leq ^* p^*\setminus i+1.$
Hence,
![]() $p^*\upharpoonright i \Vdash p^*\setminus i+1 \in F(i)$
.
$p^*\upharpoonright i \Vdash p^*\setminus i+1 \in F(i)$
.
Finally, in
![]() $M_U$
,
$M_U$
,
![]() .
.
Recall that
![]() $p \in P_\kappa $
was arbitrary. So there is such
$p \in P_\kappa $
was arbitrary. So there is such
![]() $p^* $
in
$p^* $
in
![]() $G_\kappa $
.
$G_\kappa $
.
Now it is easy to show that
![]() $\kappa $
remains a measurable cardinal in
$\kappa $
remains a measurable cardinal in
![]() $V[G_\kappa ]$
.
$V[G_\kappa ]$
.
Namely set
Lemma 3.4.
![]() $U^*$
is a normal ultrafilter over
$U^*$
is a normal ultrafilter over
![]() $\kappa $
in
$\kappa $
in
![]() $V[G_\kappa ]$
.
$V[G_\kappa ]$
.
Proof Note that for every
![]() $X\subseteq \kappa $
in
$X\subseteq \kappa $
in
![]() $V[G_\kappa ]$
, the set
$V[G_\kappa ]$
, the set
is a
![]() $\leq ^*{-}$
dense open in
$\leq ^*{-}$
dense open in
![]() $M_U[G_\kappa ]$
, by the Prikry condition of the forcing
$M_U[G_\kappa ]$
, by the Prikry condition of the forcing
![]() $ j_U(P_\kappa )/G_\kappa $
. Now, use Lemma 3.3.
$ j_U(P_\kappa )/G_\kappa $
. Now, use Lemma 3.3.
The proof of Lemma 3.3 gives the followingFootnote 10 :
Lemma 3.5. Let
![]() $ p\in P_{\kappa } $
and
$ p\in P_{\kappa } $
and
![]() $ I\subseteq \kappa $
be a stationary subset such that for every
$ I\subseteq \kappa $
be a stationary subset such that for every
![]() $\beta \in I$
,
$\beta \in I$
,
![]() $Q_\beta $
is trivial
$Q_\beta $
is trivial
![]() $($
for example, I consists of accessible or non-measurable cardinals, etc.
$($
for example, I consists of accessible or non-measurable cardinals, etc.
![]() $)$
. For every
$)$
. For every
![]() $ \beta \in I $
, let
$ \beta \in I $
, let
![]() $ F(\beta ) $
be a
$ F(\beta ) $
be a
![]() $ P_{\beta } $
-name for a
$ P_{\beta } $
-name for a
![]() $ \leq ^* $
-dense open subset of
$ \leq ^* $
-dense open subset of
![]() $ P_{\kappa } \setminus \beta $
above
$ P_{\kappa } \setminus \beta $
above
![]() $ p\setminus \beta $
, and assume that this is forced by
$ p\setminus \beta $
, and assume that this is forced by
![]() $ p\restriction {\beta } $
. Then there exist
$ p\restriction {\beta } $
. Then there exist
![]() $p^* \geq ^{*} p$
and a club
$p^* \geq ^{*} p$
and a club
![]() $ C\subseteq \kappa $
such that for every
$ C\subseteq \kappa $
such that for every
![]() $\beta \in C\cap I$
,
$\beta \in C\cap I$
,
Lemma 3.6. Suppose that
![]() $\kappa $
was a supercompact in V and there is no inaccessible
$\kappa $
was a supercompact in V and there is no inaccessible
![]() $($
or measurable
$($
or measurable
![]() $)$
cardinals above it, then it will be a strongly compact in
$)$
cardinals above it, then it will be a strongly compact in
![]() $V[G_\kappa ]$
.
$V[G_\kappa ]$
.
Proof Let U be as above and
![]() $\lambda $
be a regular cardinal above
$\lambda $
be a regular cardinal above
![]() $\kappa $
. Fix a normal ultrafilter F over
$\kappa $
. Fix a normal ultrafilter F over
![]() $\mathcal P_\kappa (\lambda )$
.
$\mathcal P_\kappa (\lambda )$
.
Consider the embedding
![]() $j=j_{j_U(F)}\circ j_U: V\to M$
.
$j=j_{j_U(F)}\circ j_U: V\to M$
.
We have the following:
-
A.
 ,
, -
B.
 $M_U \cap {}^{j_U(\lambda )}M \subseteq M$
,
$M_U \cap {}^{j_U(\lambda )}M \subseteq M$
, -
C.
 $j_U(\lambda )<j(\kappa )=j_{j_U(F)}(j_U(\kappa ))$
.
$j_U(\lambda )<j(\kappa )=j_{j_U(F)}(j_U(\kappa ))$
.
Now instead of deciding statements
![]() we would like to decide
we would like to decide
![]() or
or
![]() .
.
Note that
So, the former decisions will define a uniform
![]() $\kappa{-}$
complete ultrafilter over
$\kappa{-}$
complete ultrafilter over
![]() $\lambda $
. Similar, the latter will define a fine
$\lambda $
. Similar, the latter will define a fine
![]() $\kappa{-}$
complete ultrafilter over
$\kappa{-}$
complete ultrafilter over
![]() $\mathcal P_\kappa (\lambda )$
, since for every
$\mathcal P_\kappa (\lambda )$
, since for every
![]() $\alpha <\lambda $
,
$\alpha <\lambda $
,
Apply Lemma 3.3. The set
![]() $H=\{j_U(p)\setminus \kappa \mid p \in G_\kappa \}$
is
$H=\{j_U(p)\setminus \kappa \mid p \in G_\kappa \}$
is
![]() $M_U[G_\kappa ]$
-generic for
$M_U[G_\kappa ]$
-generic for
![]() ${\langle } j_U(P_\kappa )/G_\kappa , \leq ^*{\!\rangle } $
. Construct also (in
${\langle } j_U(P_\kappa )/G_\kappa , \leq ^*{\!\rangle } $
. Construct also (in
![]() $V[G_\kappa ]$
) an
$V[G_\kappa ]$
) an
![]() $M_U[G_\kappa ,H]$
-generic set R for
$M_U[G_\kappa ,H]$
-generic set R for
![]() ${\langle } Q_{j_U(\kappa )}, \leq ^*_{Q_{j_U(\kappa )}} {\!\rangle }$
. Note that in case of the Prikry forcings iteration this will be just an atomic forcing, since any two conditions with the same trunk will be compatible.
${\langle } Q_{j_U(\kappa )}, \leq ^*_{Q_{j_U(\kappa )}} {\!\rangle }$
. Note that in case of the Prikry forcings iteration this will be just an atomic forcing, since any two conditions with the same trunk will be compatible.
Next, consider
![]() $j_{j_U(F)}:M_U \to M$
. It is an ultrapower embedding by a normal ultrafilter
$j_{j_U(F)}:M_U \to M$
. It is an ultrapower embedding by a normal ultrafilter
![]() $j_U(F)$
over
$j_U(F)$
over
![]() $\mathcal P_{j_U(\kappa )}(j_U(\lambda ))$
of
$\mathcal P_{j_U(\kappa )}(j_U(\lambda ))$
of
![]() $M_U$
.
$M_U$
.
Take
![]() . Actually, only H is moved. By supercompactness, it can be made into a single condition s. Just take the union of all supports of this conditions, it is a union of less than
. Actually, only H is moved. By supercompactness, it can be made into a single condition s. Just take the union of all supports of this conditions, it is a union of less than
![]() $j(\kappa )$
nonstationary subsets of
$j(\kappa )$
nonstationary subsets of
![]() $j(\kappa )$
, and so is nonstationary etc. Also, note that
$j(\kappa )$
, and so is nonstationary etc. Also, note that
![]() $j_U(\kappa )$
does not belong to
$j_U(\kappa )$
does not belong to
![]() ${\textrm {supp}}(s)$
, since
${\textrm {supp}}(s)$
, since
![]() $j_U(F)$
is normal.
$j_U(F)$
is normal.
Now, using the closure, find in
![]() $M_U[G_\kappa ,H,R]$
an
$M_U[G_\kappa ,H,R]$
an
![]() $M[G_\kappa ,H,R]$
-generic subset S for the forcing
$M[G_\kappa ,H,R]$
-generic subset S for the forcing
![]() ${\langle } j(P_\kappa )/ G_\kappa *H*R, \leq ^*{\!\rangle }$
with
${\langle } j(P_\kappa )/ G_\kappa *H*R, \leq ^*{\!\rangle }$
with
![]() $s \in S$
.
$s \in S$
.
Finally, for every
![]() $D \in M[G_\kappa ]$
which is a dense open for the forcing
$D \in M[G_\kappa ]$
which is a dense open for the forcing
![]() ${\langle } j(P_\kappa )/ G_\kappa , \leq ^* {\!\rangle }$
there will be
${\langle } j(P_\kappa )/ G_\kappa , \leq ^* {\!\rangle }$
there will be
![]() $p \in G_\kappa $
and
$p \in G_\kappa $
and
![]() $q \in R*S$
such that
$q \in R*S$
such that
![]() $j_U(p)^\frown q \geq j(p)$
and
$j_U(p)^\frown q \geq j(p)$
and
![]() $j_U(p)^\frown q \in D$
.
$j_U(p)^\frown q \in D$
.
The next lemma shows that strongness implies tallness in such extensions. Apter and Cummings were first to show this (see Lemma 2.30 of [Reference Apter and Cummings2]).
The argument below follows their lines.
Lemma 3.7. Suppose that
![]() $\kappa $
was a strong cardinal in V and there is no inaccessible
$\kappa $
was a strong cardinal in V and there is no inaccessible
![]() $($
or measurable
$($
or measurable
![]() $)$
cardinals above it, then it will be a tall cardinal in
$)$
cardinals above it, then it will be a tall cardinal in
![]() $V[G_\kappa ]$
.
$V[G_\kappa ]$
.
Proof (sketch)
Let E be a
![]() $(\kappa , \lambda )$
-extender for some regular
$(\kappa , \lambda )$
-extender for some regular
![]() $\lambda>\kappa $
. Proceed as in the previous lemma, only use E instead of F and
$\lambda>\kappa $
. Proceed as in the previous lemma, only use E instead of F and
![]() $j=j_{j_U(E)}\circ j_U: V\to M$
. We do not construct a
$j=j_{j_U(E)}\circ j_U: V\to M$
. We do not construct a
![]() $\leq ^*$
-master condition sequence now, but rather use the image of a
$\leq ^*$
-master condition sequence now, but rather use the image of a
![]() $\leq ^*$
-generic over
$\leq ^*$
-generic over
![]() $M_{E(\kappa )}$
to generate a
$M_{E(\kappa )}$
to generate a
![]() $\leq ^*$
-generic over
$\leq ^*$
-generic over
![]() $M_{E}$
, and then over M, where
$M_{E}$
, and then over M, where
![]() $E(\kappa )=\{X\subseteq \kappa \mid \kappa \in j_E(X)\}$
is the normal measure of E.
$E(\kappa )=\{X\subseteq \kappa \mid \kappa \in j_E(X)\}$
is the normal measure of E.
4 Applications
If there is a supercompact cardinal, then, by Solovay (see, for example [Reference Kanamori12]) there are many normal ultrafilters. But what about strongly compact?
Question. Suppose that there is a strongly compact cardinal, does it follow that there is more than one normal ultrafilter?
Questions of this type appear in the book The Higher Infinite, by Kanamori [Reference Kanamori12].
Goldberg informed us that he and Woodin gave a negative answer starting from a measurable which is a limit of supercompact cardinals and the Ultrapower Axiom (UA). We will show this from a single supercompact and UA.
The following consequence of UA over a supercompact cardinal
![]() $\kappa $
will be used:
$\kappa $
will be used:
-
(ℵ) There exists a unique normal measure over
 $\kappa $
which concentrates on non-measurable cardinals.
$\kappa $
which concentrates on non-measurable cardinals.
Goldberg proved series of striking consequences of UA (see for example [Reference Goldberg9]). The statement above is one of them.
Using the argument of 3.6 it is possible to show that (
![]() $\aleph $
) does not imply UA.
$\aleph $
) does not imply UA.
Let us start with the following observation:
Proposition 4.1. Let
![]() $\kappa $
be a cardinal. Force with
$\kappa $
be a cardinal. Force with
![]() $Cohen(\omega )*Col(\kappa ^+,2^\kappa )$
. Let H be a generic.
$Cohen(\omega )*Col(\kappa ^+,2^\kappa )$
. Let H be a generic.
Suppose that
![]() $\kappa $
is a measurable in
$\kappa $
is a measurable in
![]() $V[H]$
and W is a
$V[H]$
and W is a
![]() $\kappa{-}$
complete ultrafilter over
$\kappa{-}$
complete ultrafilter over
![]() $\kappa $
.
$\kappa $
.
Then
![]() $U=V\cap W$
is in V
Footnote
11
and W is a unique extension of it to
$U=V\cap W$
is in V
Footnote
11
and W is a unique extension of it to
![]() $V[H]$
.
$V[H]$
.
Proof
![]() $U=V\cap W$
is in V by the Hamkins Gap Theorem, since
$U=V\cap W$
is in V by the Hamkins Gap Theorem, since
![]() $j_W\upharpoonright V$
is definable in V and
$j_W\upharpoonright V$
is definable in V and
Now, by Kunen-Paris [Reference Kunen and Paris14],
![]() generates a generic subset
generates a generic subset
![]() $H^*$
of
$H^*$
of
![]() $j_U(Cohen(\omega )*Col(\kappa ^+,2^\kappa ))$
over
$j_U(Cohen(\omega )*Col(\kappa ^+,2^\kappa ))$
over
![]() $M_U$
.
$M_U$
.
Then, in
![]() $V[H]$
,
$V[H]$
,
![]() is a
is a
![]() $\kappa {-}$
complete ultrafilter which extends U. By 2.3,
$\kappa {-}$
complete ultrafilter which extends U. By 2.3,
![]() $U^*$
is the unique
$U^*$
is the unique
![]() $\kappa {-}$
complete ultrafilter which extends U and so
$\kappa {-}$
complete ultrafilter which extends U and so
![]() $W=U^*$
.
$W=U^*$
.
The forcing used above may destroy supercompactness of
![]() $\kappa $
. In order to preserve it let us use instead the nonstationary support iteration of the forcings
$\kappa $
. In order to preserve it let us use instead the nonstationary support iteration of the forcings
![]() $Col(\alpha ^+,2^\alpha )$
for every inaccessible (or Mahlo)
$Col(\alpha ^+,2^\alpha )$
for every inaccessible (or Mahlo)
![]() $\alpha \leq \kappa $
.
$\alpha \leq \kappa $
.
Denote this iteration up to stage
![]() $\kappa $
by
$\kappa $
by
![]() $P_\kappa $
and let
$P_\kappa $
and let
![]() $P_{\kappa +1}=P_\kappa *Col(\kappa ^+,2^\kappa )$
. Let
$P_{\kappa +1}=P_\kappa *Col(\kappa ^+,2^\kappa )$
. Let
![]() $G_\kappa $
be a generic subset of
$G_\kappa $
be a generic subset of
![]() $P_\kappa $
and H a generic for
$P_\kappa $
and H a generic for
![]() $Col(\kappa ^+,2^\kappa )$
over
$Col(\kappa ^+,2^\kappa )$
over
![]() $V[G_\kappa ]$
.
$V[G_\kappa ]$
.
Proposition 4.2. Suppose that
![]() $\kappa $
is a supercompact cardinal in V and there is no inaccessible cardinal above it.
$\kappa $
is a supercompact cardinal in V and there is no inaccessible cardinal above it.
Then it remains such in
![]() $V[G_\kappa ,H]$
. If W is a
$V[G_\kappa ,H]$
. If W is a
![]() $\kappa{-}$
complete ultrafilter over
$\kappa{-}$
complete ultrafilter over
![]() $\kappa $
in
$\kappa $
in
![]() $V[G_\kappa ,H]$
.
$V[G_\kappa ,H]$
.
Then
![]() $U=V\cap W$
is in V and W is a unique extension of it in
$U=V\cap W$
is in V and W is a unique extension of it in
![]() $V[G_\kappa ,H]$
.
$V[G_\kappa ,H]$
.
Proof The argument for supercompactness is rather standard. Namely, let
![]() $\lambda>2^\kappa $
be a regular cardinal and let F be a normal ultrafilter over
$\lambda>2^\kappa $
be a regular cardinal and let F be a normal ultrafilter over
![]() $\mathcal P_\kappa (\lambda )$
in V. We will extend the embedding
$\mathcal P_\kappa (\lambda )$
in V. We will extend the embedding
![]() $j_F:V\to M_F$
to an elementary embedding
$j_F:V\to M_F$
to an elementary embedding
![]() $j^*:V[G_\kappa ,H] \to M_F[G_\kappa ^*,H^*]$
.
$j^*:V[G_\kappa ,H] \to M_F[G_\kappa ^*,H^*]$
.
Thus consider first
![]() . Note that
. Note that
![]() $\kappa $
does not appear in supports of its elements due to normality of F. So, we can using the closure of the forcing and the ultrapower, combine elements of this set into a single condition s in
$\kappa $
does not appear in supports of its elements due to normality of F. So, we can using the closure of the forcing and the ultrapower, combine elements of this set into a single condition s in
![]() $j_F(P_\kappa *Col(\kappa ^+,2^\kappa ))/ G_\kappa *H$
. Finally, build a master condition sequence above s for
$j_F(P_\kappa *Col(\kappa ^+,2^\kappa ))/ G_\kappa *H$
. Finally, build a master condition sequence above s for
![]() $M_F[G_\kappa *H]$
.
$M_F[G_\kappa *H]$
.
Now let us deal with second part of the statement.
Let W be a
![]() $\kappa{-}$
complete ultrafilter over
$\kappa{-}$
complete ultrafilter over
![]() $\kappa $
in
$\kappa $
in
![]() $V[G_\kappa ,H]$
.
$V[G_\kappa ,H]$
.
Again, as in 4.1, by Hamkins Gap Theorem,
![]() $U=V\cap W \in V$
(a gap now, for example, at the second inaccessible below
$U=V\cap W \in V$
(a gap now, for example, at the second inaccessible below
![]() $\kappa $
). Still,
$\kappa $
). Still,
![]() generates a generic subset
generates a generic subset
![]() $G^**H^*$
of
$G^**H^*$
of
![]() $j_U(P_\kappa *Col(\kappa ^+,2^\kappa ))$
over
$j_U(P_\kappa *Col(\kappa ^+,2^\kappa ))$
over
![]() $M_U$
. We can apply 3.3 together with Kunen–Paris for this.
$M_U$
. We can apply 3.3 together with Kunen–Paris for this.
The final stage is as in 4.1.
Now the following follows:
Corollary 4.3. Suppose that
![]() $\kappa $
is a supercompact cardinal with no inaccessible cardinal above it and with a unique normal measure which concentrates on non-measurable cardinals.
$\kappa $
is a supercompact cardinal with no inaccessible cardinal above it and with a unique normal measure which concentrates on non-measurable cardinals.
Then there is a generic extension in which
![]() $2^\kappa =\kappa ^+$
and
$2^\kappa =\kappa ^+$
and
![]() $\kappa $
is still a supercompact cardinal with a unique normal measure which concentrates on non-measurable cardinals.
$\kappa $
is still a supercompact cardinal with a unique normal measure which concentrates on non-measurable cardinals.
Now we are ready to prove the result:
Theorem 4.4. Suppose that
![]() $\kappa $
is a supercompact cardinal with a unique normal measure which concentrates on non-measurable cardinals and
$\kappa $
is a supercompact cardinal with a unique normal measure which concentrates on non-measurable cardinals and
![]() $2^\kappa =\kappa ^+$
.
$2^\kappa =\kappa ^+$
.
Assume that there is no inaccessible cardinals above
![]() $\kappa $
.
$\kappa $
.
Let
![]() $P_\kappa $
be the forcing adding non-reflecting stationary subset of inaccessibles to every measurable cardinal below
$P_\kappa $
be the forcing adding non-reflecting stationary subset of inaccessibles to every measurable cardinal below
![]() $\kappa $
with the nonstationary support. Let
$\kappa $
with the nonstationary support. Let
![]() $G_\kappa \subseteq P_\kappa $
be a generic.
$G_\kappa \subseteq P_\kappa $
be a generic.
Then, in
![]() $V[G_\kappa ]$
,
$V[G_\kappa ]$
,
![]() $\kappa $
is a strongly compact, the only measurable cardinal and there is a unique normal measure.Footnote
12
$\kappa $
is a strongly compact, the only measurable cardinal and there is a unique normal measure.Footnote
12
Proof By Lemma 3.6,
![]() $\kappa $
is strongly compact in
$\kappa $
is strongly compact in
![]() $V[G_\kappa ]$
. Clearly, it is a unique measurable there.
$V[G_\kappa ]$
. Clearly, it is a unique measurable there.
Let W be a normal ultrafilter over
![]() $\kappa $
in
$\kappa $
in
![]() $V[G_\kappa ]$
. Note that W concentrates on cardinals which are non-measurable in V. Just otherwise,
$V[G_\kappa ]$
. Note that W concentrates on cardinals which are non-measurable in V. Just otherwise,
![]() $\kappa $
will be a measurable in the ground model of
$\kappa $
will be a measurable in the ground model of
![]() $M_W$
, and so, a non-reflecting stationary set S should be added there. But such S will be stationary in
$M_W$
, and so, a non-reflecting stationary set S should be added there. But such S will be stationary in
![]() $V[G_\kappa ]$
as well, since
$V[G_\kappa ]$
as well, since
![]() ${}^\kappa M_W\subseteq M_W$
. However,
${}^\kappa M_W\subseteq M_W$
. However,
![]() $\kappa $
is a measurable cardinal in
$\kappa $
is a measurable cardinal in
![]() $V[G_\kappa ]$
.
$V[G_\kappa ]$
.
By Hamkins [Reference Hamkins10], no new fresh subsets are added to
![]() $\kappa ,\kappa ^+$
. So, by 2.1,
$\kappa ,\kappa ^+$
. So, by 2.1,
![]() $U=W\cap V$
is in V. Hence, U is a normal ultrafilter on
$U=W\cap V$
is in V. Hence, U is a normal ultrafilter on
![]() $\kappa $
in V which concentrates on non-measurable cardinals.
$\kappa $
in V which concentrates on non-measurable cardinals.
By our assumption, U is a unique ultrafilter like these.
Now let us turn to the Prikry forcings. We will be to show a parallel result for a nonstationary iteration:
Theorem 4.5. Suppose that
![]() $\kappa $
is a supercompact cardinal with a unique normal measure which concentrates on non-measurable cardinals and
$\kappa $
is a supercompact cardinal with a unique normal measure which concentrates on non-measurable cardinals and
![]() $2^\kappa =\kappa ^+$
.
$2^\kappa =\kappa ^+$
.
Assume that there is no inaccessible cardinals above
![]() $\kappa $
.
$\kappa $
.
Let
![]() $P_\kappa $
be a nonstationary support iteration of Prikry forcing changing cofinality of every measurable cardinal below
$P_\kappa $
be a nonstationary support iteration of Prikry forcing changing cofinality of every measurable cardinal below
![]() $\kappa $
to
$\kappa $
to
![]() $\omega $
. Let
$\omega $
. Let
![]() $G_\kappa \subseteq P_\kappa $
be a generic.
$G_\kappa \subseteq P_\kappa $
be a generic.
Then, in
![]() $V[G_\kappa ]$
,
$V[G_\kappa ]$
,
![]() $\kappa $
is a strongly compact, the only measurable cardinal and there is a unique normal measure.
$\kappa $
is a strongly compact, the only measurable cardinal and there is a unique normal measure.
The proof repeats completely the proof of 4.4, only we cannot appeal anymore to Hamkins result about fresh subsets, since now we use a forcing which is not strategically closed. Instead, let us argue directly that there is no fresh subsets of
![]() $\kappa $
and of
$\kappa $
and of
![]() $\kappa ^+$
in
$\kappa ^+$
in
![]() $V[G_\kappa ]$
.
$V[G_\kappa ]$
.
Let us do this for all three supports—Easton, full and nonstationary (see [Reference Gitik and Foreman7] for Easton and full supports).
We start with
![]() $\kappa $
.
$\kappa $
.
Lemma 4.6. Let
![]() $V[G]$
be a generic extension of V by a forcing P which preserves
$V[G]$
be a generic extension of V by a forcing P which preserves
![]() $\kappa ^+$
. Suppose that there is a normal ultrafilter W over
$\kappa ^+$
. Suppose that there is a normal ultrafilter W over
![]() $\kappa $
in
$\kappa $
in
![]() $V[G]$
such that
$V[G]$
such that
![]() ${U=V\cap W \in V}$
.
${U=V\cap W \in V}$
.
Assume that there is a set
![]() $X\in U$
such that for every
$X\in U$
such that for every
![]() $\alpha \in X$
, GCH holds at
$\alpha \in X$
, GCH holds at
![]() $\alpha $
in V.
$\alpha $
in V.
Then there is no fresh subsets of
![]() $\kappa $
in
$\kappa $
in
![]() $V[G]$
.
$V[G]$
.
Proof Suppose otherwise. Let A be a fresh subset of
![]() $\kappa $
. Consider
$\kappa $
. Consider
![]() $j_W:V[G]\to M_W$
. Then
$j_W:V[G]\to M_W$
. Then
![]() $M_W=M[j_W(G))]$
. By freshness of A, we have then
$M_W=M[j_W(G))]$
. By freshness of A, we have then
Set
![]() $k([f]_U)=[f]_W$
. By 2.4, it is an elementary embedding from
$k([f]_U)=[f]_W$
. By 2.4, it is an elementary embedding from
![]() $M_U$
to M. The critical point of k (if exists) should be
$M_U$
to M. The critical point of k (if exists) should be
![]() $>\kappa ^+$
, due to the canonical functions from
$>\kappa ^+$
, due to the canonical functions from
![]() $\kappa $
to
$\kappa $
to
![]() $\kappa $
.
$\kappa $
.
Finally, both
![]() $M_U$
and M satisfy GCH at
$M_U$
and M satisfy GCH at
![]() $\kappa $
. Hence,
$\kappa $
. Hence,
![]() $A\in M_U\subseteq V$
. Contradiction.
$A\in M_U\subseteq V$
. Contradiction.
We can conclude the following:
Corollary 4.7. Easton, full and nonstationary support iterations of Prikry type forcing notions of a measurable length
![]() $\kappa $
which satisfy the assumptions of 4.6 do not add fresh subsets to
$\kappa $
which satisfy the assumptions of 4.6 do not add fresh subsets to
![]() $\kappa $
.
$\kappa $
.
In particular, assuming
![]() $2^\kappa =\kappa ^+$
, Easton, full and nonstationary support iterations of Prikry forcings do not add fresh subsets to
$2^\kappa =\kappa ^+$
, Easton, full and nonstationary support iterations of Prikry forcings do not add fresh subsets to
![]() $\kappa $
.
$\kappa $
.
Let us deal now with
![]() $\kappa ^+$
.
$\kappa ^+$
.
The following lemma is obvious:
Lemma 4.8. Let
![]() $\lambda $
be a regular cardinal and P be a forcing notion of cardinality
$\lambda $
be a regular cardinal and P be a forcing notion of cardinality
![]() $<\lambda $
. Then P does not add fresh subsets to
$<\lambda $
. Then P does not add fresh subsets to
![]() $\lambda $
.
$\lambda $
.
Proof Suppose otherwise. Let
![]() $G\subseteq P $
be a generic and let A be a fresh subset of
$G\subseteq P $
be a generic and let A be a fresh subset of
![]() $\lambda $
in
$\lambda $
in
![]() $V[G]$
. For every
$V[G]$
. For every
![]() $\nu \in A$
pick a condition
$\nu \in A$
pick a condition
![]() $p_\nu \in G$
which decides
$p_\nu \in G$
which decides
![]() . By regularity of
. By regularity of
![]() $\lambda $
, there is a single
$\lambda $
, there is a single
![]() $p\in G$
which decides
$p\in G$
which decides
![]() for unboundedly many
for unboundedly many
![]() $\nu $
’s. Set B to be the union of such decisions made by p. Clearly,
$\nu $
’s. Set B to be the union of such decisions made by p. Clearly,
![]() $B\in V$
and
$B\in V$
and
![]() . So,
. So,
![]() $A=B\in V$
. Contradiction.
$A=B\in V$
. Contradiction.
As a corollary we obtain:
Corollary 4.9. Easton support iterations of Prikry type forcing notions each of cardinality
![]() $<\kappa $
of a regular length
$<\kappa $
of a regular length
![]() $\kappa $
do not add fresh subsets to
$\kappa $
do not add fresh subsets to
![]() $\kappa ^+$
.
$\kappa ^+$
.
Using a similar idea, it is possible to show the following:
Lemma 4.10. Let
![]() $P_\kappa $
be a full support iteration of Prikry type forcing notions
$P_\kappa $
be a full support iteration of Prikry type forcing notions
![]() of a regular length
of a regular length
![]() $\kappa $
such that
$\kappa $
such that
-
•
 ,
, -
• if
 and
and
 , then there is
, then there is
 .
.
For example a full support iteration of Prikry forcings is like this.
Then
![]() $P_\kappa $
does not add fresh subsets to
$P_\kappa $
does not add fresh subsets to
![]() $\kappa ^+$
.
$\kappa ^+$
.
Proof Suppose otherwise. Let
![]() $G_\kappa \subseteq P_\kappa $
be a generic and let A be a fresh subset of
$G_\kappa \subseteq P_\kappa $
be a generic and let A be a fresh subset of
![]() $\lambda $
in
$\lambda $
in
![]() $V[G]$
.
$V[G]$
.
Fix some
![]() . For every
. For every
![]() $\nu \in A$
pick a condition
$\nu \in A$
pick a condition
![]() $p^\nu \in G_\kappa , p^\nu \geq s $
which decides
$p^\nu \in G_\kappa , p^\nu \geq s $
which decides
![]() . Then there is a finite
. Then there is a finite
![]() $a^\nu \subseteq \kappa $
such that at every coordinate
$a^\nu \subseteq \kappa $
such that at every coordinate
![]() $\beta <\kappa $
outside of
$\beta <\kappa $
outside of
![]() $a^\nu $
,
$a^\nu $
,
![]() .
.
By regularity of
![]() $\kappa ^+$
, there will be a single a such that
$\kappa ^+$
, there will be a single a such that
![]() $a=a_\nu $
, for unboundedly many
$a=a_\nu $
, for unboundedly many
![]() $\nu $
’s.
$\nu $
’s.
By shrinking more if necessary, we can assume that there is a single condition
![]() $q \in P_{\max (a)+1}$
such that
$q \in P_{\max (a)+1}$
such that
![]() $q=p^\nu \upharpoonright \max (a)+1$
, for unboundedly many
$q=p^\nu \upharpoonright \max (a)+1$
, for unboundedly many
![]() $\nu $
’s.
$\nu $
’s.
Then, by the assumption of the lemma, if
![]() $t \in P_\kappa $
,
$t \in P_\kappa $
,
![]() $t\upharpoonright \max (a)+1=q$
and
$t\upharpoonright \max (a)+1=q$
and
![]() $t\setminus \max (a)+1\geq ^* s\setminus \max (a)+1$
then t is compatible with all such
$t\setminus \max (a)+1\geq ^* s\setminus \max (a)+1$
then t is compatible with all such
![]() $p^\nu $
’s.
$p^\nu $
’s.
Set

Clearly,
![]() $B\in V$
and
$B\in V$
and
![]() $A=B$
. Contradiction.
$A=B$
. Contradiction.
Finally let us deal with a nonstationary support iteration.
Lemma 4.11. Let
![]() $ I\subseteq \kappa $
be a stationary subset which includes all accessible cardinals and let
$ I\subseteq \kappa $
be a stationary subset which includes all accessible cardinals and let
![]() be a nonstationary support iteration of a Prikry type forcing notions
be a nonstationary support iteration of a Prikry type forcing notions
![]() of a regular length
of a regular length
![]() $\kappa $
such that for every
$\kappa $
such that for every
![]() $\beta <\kappa $
the following hold:
$\beta <\kappa $
the following hold:
-
1.
 is trivial for every
is trivial for every
 $\beta \in I$
,
$\beta \in I$
, -
2.
 is
is
 $\beta {-}$
strategically closed,
$\beta {-}$
strategically closed, -
3.
 is less than the first inaccessible cardinal above
is less than the first inaccessible cardinal above
 $\beta $
.
$\beta $
.
Then
![]() $P_\kappa $
does not add fresh subsets to
$P_\kappa $
does not add fresh subsets to
![]() $\kappa ^+$
.
$\kappa ^+$
.
Proof Assume that
![]() is a
is a
![]() $ P_{\kappa } $
-name for a fresh function in
$ P_{\kappa } $
-name for a fresh function in
![]() $ 2^{\kappa ^{+}} $
, and this is forced by the weakest condition in
$ 2^{\kappa ^{+}} $
, and this is forced by the weakest condition in
![]() $ P_{\kappa } $
.
$ P_{\kappa } $
.
At least one of
![]() is non-trivial, since otherwise a generic extension will be just V.
is non-trivial, since otherwise a generic extension will be just V.
Case 1. There exists
![]() $ \mu \in I $
which is above the first
$ \mu \in I $
which is above the first
![]() $\beta <\kappa $
for which
$\beta <\kappa $
for which
is non-trivial, and a condition
![]() $ p^* \in P_{\mu } $
which forces that the following property holds:
$ p^* \in P_{\mu } $
which forces that the following property holds:

(here,
![]() $ G_{\mu } $
is the canonical name for the generic set for
$ G_{\mu } $
is the canonical name for the generic set for
![]() $ P_{\mu } $
). By extending
$ P_{\mu } $
). By extending
![]() $ p^* $
, we can decide the value of
$ p^* $
, we can decide the value of
![]() $ p $
in the statement above, and thus assume that
$ p $
in the statement above, and thus assume that
![]() $ p^* \geq p $
. Let
$ p^* \geq p $
. Let
be a
![]() $ P_{\mu } $
-name for
$ P_{\mu } $
-name for
![]() $ s $
from the above property, and assume that this is forced by
$ s $
from the above property, and assume that this is forced by
![]() $ p^* $
.
$ p^* $
.
Fix a
![]() $ P_{\mu } $
-name for a strategy,
$ P_{\mu } $
-name for a strategy,
![]() , for the second player in the game of length
, for the second player in the game of length
![]() $ \mu +1 $
, which witnesses the
$ \mu +1 $
, which witnesses the
![]() $ \mu +1 $
-strategically closure of
$ \mu +1 $
-strategically closure of
![]() $ \langle P\setminus \mu , \leq ^* \rangle $
. Note that such a strategy exists since
$ \langle P\setminus \mu , \leq ^* \rangle $
. Note that such a strategy exists since
![]() $ \mu \in I$
. Assume that
$ \mu \in I$
. Assume that
![]() $ p^* $
forces the
$ p^* $
forces the
![]() is such a strategy.
is such a strategy.
Let us apply the same methods as in the main lemma in [Reference Hamkins10]. We construct, in
![]() $ V $
, a binary tree of conditions,
$ V $
, a binary tree of conditions,
![]() and a tree of functions
and a tree of functions
![]() $ \langle b_{\sigma } \colon \sigma \in {}^{\mu>}2 \rangle $
such that
$ \langle b_{\sigma } \colon \sigma \in {}^{\mu>}2 \rangle $
such that
![]() , and for every
, and for every
![]() $ \sigma \in {}^{\mu>}2$
:
$ \sigma \in {}^{\mu>}2$
:
-
1.
 .
. -
2.
 .
. -
3. If
 $ \mbox {lh}(\sigma ) $
is limit, then
$ \mbox {lh}(\sigma ) $
is limit, then
 $ p^* $
forces that
$ p^* $
forces that
 is an upper bound, with respect to the direct extension order, of
is an upper bound, with respect to the direct extension order, of
 .
. -
4.
 $ b_{\sigma } $
is an end extension of
$ b_{\sigma } $
is an end extension of
 $ b_{\sigma \restriction { \xi }} $
for every
$ b_{\sigma \restriction { \xi }} $
for every
 $ \xi <\mbox {lh}(\sigma ) $
.
$ \xi <\mbox {lh}(\sigma ) $
. -
5. For every
 $ \xi <\mbox {lh}(\sigma ) $
,
$ \xi <\mbox {lh}(\sigma ) $
,
 $ p^* $
forces that
$ p^* $
forces that
 is the sequence of moves of the first player in the game, where the second player plays according to the strategy
is the sequence of moves of the first player in the game, where the second player plays according to the strategy
 $ \tau $
.
$ \tau $
.
Now assume that
![]() $ g\subseteq P_{\mu } $
is generic over
$ g\subseteq P_{\mu } $
is generic over
![]() $ V $
with
$ V $
with
![]() $ p^* \in g $
. In
$ p^* \in g $
. In
![]() $ V\left [g\right ] $
, let
$ V\left [g\right ] $
, let
![]() $ h\in 2^{<\mu } $
be the characteristic function of a new subset of
$ h\in 2^{<\mu } $
be the characteristic function of a new subset of
![]() $\mu $
. Clearly,
$\mu $
. Clearly,
![]() $ h \notin V $
.
$ h \notin V $
.
![]() $ h $
defines a branch through the binary tree,
$ h $
defines a branch through the binary tree,
![]() . The forcing
. The forcing
![]() $ P\setminus \mu $
has a direct extension order which is more than
$ P\setminus \mu $
has a direct extension order which is more than
![]() $ \mu {-}$
closed, so there exists an upper bound for the conditions in the branch, of the form
$ \mu {-}$
closed, so there exists an upper bound for the conditions in the branch, of the form
![]() . It forces that
. It forces that
is an initial segment of
![]() . Therefore,
. Therefore,
![]() $ b\in V $
, and thus
$ b\in V $
, and thus
![]() $ h $
can be defined, in
$ h $
can be defined, in
![]() $ V $
, using the binary tree and the set
$ V $
, using the binary tree and the set
![]() $ b $
. This is a contradiction to the choice of
$ b $
. This is a contradiction to the choice of
![]() $ h $
.
$ h $
.
Case 2. For every
![]() $ \mu \in I $
which is above the first
$ \mu \in I $
which is above the first
![]() $\beta <\kappa $
for which
$\beta <\kappa $
for which
is non-trivial, every condition in
![]() $ P_{\mu } $
forces that
$ P_{\mu } $
forces that

so, for every
![]() $ \mu \in I $
, the following set is forced to be
$ \mu \in I $
, the following set is forced to be
![]() $ \leq ^* $
-dense open in
$ \leq ^* $
-dense open in
![]() $ P\setminus \mu $
:
$ P\setminus \mu $
:

Note that we used here the fact that
![]() $ \left | G_{\mu } \right |$
is below the first inaccessible above
$ \left | G_{\mu } \right |$
is below the first inaccessible above
![]() $ \mu $
, and
$ \mu $
, and
![]() $ P\setminus \mu $
has a direct extension order which has enough strategic closure (since
$ P\setminus \mu $
has a direct extension order which has enough strategic closure (since
![]() $ \mu $
is in I).
$ \mu $
is in I).
Let us apply lemma 3.5. There exists
![]() $ p\in P_{\kappa } $
and a club
$ p\in P_{\kappa } $
and a club
![]() $ C\subseteq \kappa $
such that for every
$ C\subseteq \kappa $
such that for every
![]() $ \mu \in C\cap I$
,
$ \mu \in C\cap I$
,
Now, assume that
![]() $ G\subseteq P_{\kappa } $
is generic over
$ G\subseteq P_{\kappa } $
is generic over
![]() $ V $
and
$ V $
and
![]() $ p\in G $
. For every
$ p\in G $
. For every
![]() $ \xi <\kappa ^{+} $
, there exists
$ \xi <\kappa ^{+} $
, there exists
![]() $ p_{\xi }\in G $
above
$ p_{\xi }\in G $
above
![]() $ p $
which decides
$ p $
which decides
![]() . For every such
. For every such
![]() $ \xi $
, there exists
$ \xi $
, there exists
![]() $ \mu _{\xi } \in C\cap I $
such that coordinates above
$ \mu _{\xi } \in C\cap I $
such that coordinates above
![]() $ \mu _{\xi } $
in
$ \mu _{\xi } $
in
![]() $ p_{\xi } $
are direct extensions of
$ p_{\xi } $
are direct extensions of
![]() $ p $
. There exists
$ p $
. There exists
![]() $ \mu ^*<\kappa $
such that, for unboundedly many values of
$ \mu ^*<\kappa $
such that, for unboundedly many values of
![]() $ \xi <\kappa ^{+}$
,
$ \xi <\kappa ^{+}$
,
![]() $ \mu _{\xi } = \mu ^* $
. Similarly, there exists
$ \mu _{\xi } = \mu ^* $
. Similarly, there exists
![]() $ p^* \in P_{\mu ^*} $
such that for unboundedly many values of
$ p^* \in P_{\mu ^*} $
such that for unboundedly many values of
![]() $ \xi <\kappa ^{+} $
,
$ \xi <\kappa ^{+} $
,
![]() $ p_{\xi }\restriction { \mu ^* } = p^* $
. Let
$ p_{\xi }\restriction { \mu ^* } = p^* $
. Let
![]() $ q = {p^*}^{\frown } p\setminus {\mu ^*} $
. So
$ q = {p^*}^{\frown } p\setminus {\mu ^*} $
. So
![]() $ q\in G $
has the following property: For every
$ q\in G $
has the following property: For every
![]() $ \xi <\kappa ^{+} $
there exists
$ \xi <\kappa ^{+} $
there exists
![]() $ r_{\xi }\in P\setminus {\mu ^*} $
such that
$ r_{\xi }\in P\setminus {\mu ^*} $
such that
![]() $ {q\restriction { {\mu ^*} }}^{\frown } r_{\xi } \in G $
decides
$ {q\restriction { {\mu ^*} }}^{\frown } r_{\xi } \in G $
decides
![]() and
and
![]() $ q\restriction {{\mu ^*}} \Vdash r_{\xi }\geq ^* q\setminus {\mu ^*}$
.
$ q\restriction {{\mu ^*}} \Vdash r_{\xi }\geq ^* q\setminus {\mu ^*}$
.
Let
![]() $ q' \in G $
be a condition which forces that the property above holds for
$ q' \in G $
be a condition which forces that the property above holds for
![]() $ \check {q} $
.
$ \check {q} $
.
In
![]() $ V $
, let
$ V $
, let
![]() $ g \colon \kappa ^{+} \to 2 $
be the union of all the functions which are forced to be an initial segment of
$ g \colon \kappa ^{+} \to 2 $
be the union of all the functions which are forced to be an initial segment of
![]() , by some direct extension of
, by some direct extension of
![]() $ q $
of the form
$ q $
of the form
![]() $ {q\restriction { {\mu ^*} }}^{\frown } r $
, where
$ {q\restriction { {\mu ^*} }}^{\frown } r $
, where
![]() $ q\restriction {{\mu ^*}} \Vdash r\geq ^* q\setminus {\mu ^*} $
.
$ q\restriction {{\mu ^*}} \Vdash r\geq ^* q\setminus {\mu ^*} $
.
![]() $ g $
is a function since
$ g $
is a function since
![]() $ q\setminus {\mu ^*}= p\setminus {\mu ^*}\in e({\mu ^*}) $
.
$ q\setminus {\mu ^*}= p\setminus {\mu ^*}\in e({\mu ^*}) $
.
Finally, let us note that
![]() $ q' $
forces that
$ q' $
forces that
![]() , a contradiction.
, a contradiction.
Note that neither Easton (specially) and full support share the uniqueness properties of a nonstationary support. However, we still have the following conclusions:
Theorem 4.12. Suppose the following:
-
1.
 $\kappa $
is a measurable cardinal.
$\kappa $
is a measurable cardinal. -
2.
 $2^\kappa =\kappa ^+$
.
$2^\kappa =\kappa ^+$
. -
3.
 $P_\kappa $
is an Easton support iteration of Prikry type forcing notions
$P_\kappa $
is an Easton support iteration of Prikry type forcing notions
 each of cardinality
each of cardinality
 $<\kappa $
.
$<\kappa $
.Let
 $G_\kappa \subseteq P_\kappa $
be a generic.
$G_\kappa \subseteq P_\kappa $
be a generic. -
4. There is a normal ultrafilter F in V over
 $\kappa $
which extends to a normal ultrafilter in
$\kappa $
which extends to a normal ultrafilter in
 $V[G_\kappa ]$
and a set
$V[G_\kappa ]$
and a set
 $X\in F$
such that for every
$X\in F$
such that for every
 $\alpha \in X$
, GCH holds in V at
$\alpha \in X$
, GCH holds in V at
 $\alpha $
.
$\alpha $
.
Then for every
![]() $\kappa{-}$
complete ultrafilter W over
$\kappa{-}$
complete ultrafilter W over
![]() $\kappa $
in
$\kappa $
in
![]() $V[G_\kappa ]$
,
$V[G_\kappa ]$
,
![]() $U=V\cap W$
is in V.
$U=V\cap W$
is in V.
Proof Note that
![]() $|P_\kappa |=\kappa $
, and so,
$|P_\kappa |=\kappa $
, and so,
![]() $\kappa ^+$
is preserved and
$\kappa ^+$
is preserved and
![]() $2^\kappa =\kappa ^+$
in
$2^\kappa =\kappa ^+$
in
![]() $V[G_\kappa ]$
.
$V[G_\kappa ]$
.
By Lemma 4.6, no fresh subsets are added to
![]() $\kappa $
and by Corollary 4.9, no fresh subsets are added to
$\kappa $
and by Corollary 4.9, no fresh subsets are added to
![]() $\kappa ^+$
.
$\kappa ^+$
.
Now, by Proposition 2.1,
![]() $U\in V$
.
$U\in V$
.
Theorem 4.13. Suppose the following:
-
1.
 $\kappa $
is a measurable cardinal.
$\kappa $
is a measurable cardinal. -
2.
 $2^\kappa =\kappa ^+$
.
$2^\kappa =\kappa ^+$
. -
3.
 $P_\kappa $
be a full support iteration of Prikry type forcing notions
$P_\kappa $
be a full support iteration of Prikry type forcing notions
 such that
such that-
•
 ,
, -
• if
 and
and
 , then there is
, then there is
 .
.
Let
 $G_\kappa \subseteq P_\kappa $
be a generic.
$G_\kappa \subseteq P_\kappa $
be a generic. -
-
4. There is a normal ultrafilter F in V over
 $\kappa $
which extends to a normal ultrafilter in
$\kappa $
which extends to a normal ultrafilter in
 $V[G_\kappa ]$
and a set
$V[G_\kappa ]$
and a set
 $X\in F$
such that for every
$X\in F$
such that for every
 $\alpha \in X$
, GCH holds in V at
$\alpha \in X$
, GCH holds in V at
 $\alpha $
.
$\alpha $
.
Then for every
![]() $\kappa{-}$
complete ultrafilter W over
$\kappa{-}$
complete ultrafilter W over
![]() $\kappa $
in
$\kappa $
in
![]() $V[G_\kappa ]$
,
$V[G_\kappa ]$
,
![]() $U=V\cap W$
is in V.
$U=V\cap W$
is in V.
Proof Note that
![]() $P_\kappa $
preserves
$P_\kappa $
preserves
![]() $\kappa ^+$
and
$\kappa ^+$
and
![]() $2^\kappa =\kappa ^+$
in
$2^\kappa =\kappa ^+$
in
![]() $V[G_\kappa ]$
.
$V[G_\kappa ]$
.
By Lemma 4.6, no fresh subsets are added to
![]() $\kappa $
and by Lemma 4.10, no fresh subsets are added to
$\kappa $
and by Lemma 4.10, no fresh subsets are added to
![]() $\kappa ^+$
.
$\kappa ^+$
.
Now, by Proposition 2.1,
![]() $U\in V$
.
$U\in V$
.
The work by Ben-Neria [Reference Ben-Neria4] allows to say more about number of possible extensions of ultrafilters from V to a full support iterations
![]() $V[G_\kappa ]$
. Let
$V[G_\kappa ]$
. Let
![]() $P_\kappa $
be just the usual Magidor (full support) iteration of Prikry forcings [Reference Magidor15].
$P_\kappa $
be just the usual Magidor (full support) iteration of Prikry forcings [Reference Magidor15].
Following [Reference Ben-Neria4], denote
Let
![]() $d:\Delta \to \kappa $
is the function which takes a measurable in V cardinal
$d:\Delta \to \kappa $
is the function which takes a measurable in V cardinal
![]() $\nu $
to the first element of its Prikry sequence.
$\nu $
to the first element of its Prikry sequence.
Ben-Neria proved in [Reference Ben-Neria4, Proposition 3.4] assuming
![]() , that there is a unique normal measure in
, that there is a unique normal measure in
![]() $V[G_\kappa ]$
that does not concentrates on
$V[G_\kappa ]$
that does not concentrates on
![]() $d"\Delta $
. He make use of existence of the core model and restrictions of ultrapower embeddings to it.
$d"\Delta $
. He make use of existence of the core model and restrictions of ultrapower embeddings to it.
It is not hard to replace this inner models part by appealing to 4.13. So, the following holds:
Theorem 4.14. Suppose that
![]() $\kappa $
is a supercompact cardinal with a unique normal measure which concentrates on non-measurable cardinals and
$\kappa $
is a supercompact cardinal with a unique normal measure which concentrates on non-measurable cardinals and
![]() $2^\kappa =\kappa ^+$
.
$2^\kappa =\kappa ^+$
.
Assume that there is no inaccessible cardinals above
![]() $\kappa $
.
$\kappa $
.
Let
![]() $P_\kappa $
be a full support iteration of Prikry forcing changing cofinality of every measurable cardinal below
$P_\kappa $
be a full support iteration of Prikry forcing changing cofinality of every measurable cardinal below
![]() $\kappa $
to
$\kappa $
to
![]() $\omega $
. Let
$\omega $
. Let
![]() $G_\kappa \subseteq P_\kappa $
be a generic.
$G_\kappa \subseteq P_\kappa $
be a generic.
Then, in
![]() $V[G_\kappa ]$
,
$V[G_\kappa ]$
,
![]() $\kappa $
is a strongly compact, the only measurable cardinal and there is a unique normal measure which does not concentrate on
$\kappa $
is a strongly compact, the only measurable cardinal and there is a unique normal measure which does not concentrate on
![]() $d"\Delta $
.
$d"\Delta $
.
Let us return to 4.4, 4.5, but use a bit more strength of the Ultrapower Axiom.
By Goldberg [Reference Goldberg9], there exists not only a unique normal measure over a supercompact
![]() $\kappa $
which concentrates over non-measurable cardinals, but also a unique normal measure which concentrates on measurable cardinals of the Mitchell order 1 (i.e., having a unique normal measure), of the Mitchell order 2 etc.
$\kappa $
which concentrates over non-measurable cardinals, but also a unique normal measure which concentrates on measurable cardinals of the Mitchell order 1 (i.e., having a unique normal measure), of the Mitchell order 2 etc.
Let
![]() $\eta <\kappa $
. Now, instead of (
$\eta <\kappa $
. Now, instead of (
![]() $\aleph $
) above we assume the following consequence of UA:
$\aleph $
) above we assume the following consequence of UA:
![]() $(\beth )_\eta $
For every
$(\beth )_\eta $
For every
![]() $\tau <\eta $
there exists a unique normal measure of the Mitchell order
$\tau <\eta $
there exists a unique normal measure of the Mitchell order
![]() $\tau $
over
$\tau $
over
![]() $\kappa $
.
$\kappa $
.
The following follows from 4.2:
Corollary 4.15. Suppose that
![]() $\kappa $
is a supercompact cardinal with no inaccessible cardinal above it and
$\kappa $
is a supercompact cardinal with no inaccessible cardinal above it and
![]() $(\beth )_\eta $
holds.
$(\beth )_\eta $
holds.
Then there is a generic extension in which no new subsets are added to
![]() $\eta $
,
$\eta $
,
![]() $2^\kappa =\kappa ^+$
and
$2^\kappa =\kappa ^+$
and
![]() $\kappa $
is still a supercompact cardinal and
$\kappa $
is still a supercompact cardinal and
![]() $(\beth )_\eta $
holds.
$(\beth )_\eta $
holds.
Now we can deduce the following:
Theorem 4.16. Suppose that
![]() $\kappa $
is a supercompact cardinal,
$\kappa $
is a supercompact cardinal,
![]() $(\beth )_\eta $
holds and
$(\beth )_\eta $
holds and
![]() $2^\kappa =\kappa ^+$
.
$2^\kappa =\kappa ^+$
.
Assume that there is no inaccessible cardinals above
![]() $\kappa $
.
$\kappa $
.
Let
![]() $P_\kappa $
be the forcing adding non-reflecting stationary subset of inaccessibles (or alternatively the Prikry forcing) for every measurable cardinal below
$P_\kappa $
be the forcing adding non-reflecting stationary subset of inaccessibles (or alternatively the Prikry forcing) for every measurable cardinal below
![]() $\kappa $
of the Mitchell order
$\kappa $
of the Mitchell order
![]() $\geq \eta $
with the nonstationary support. Let
$\geq \eta $
with the nonstationary support. Let
![]() $G_\kappa \subseteq P_\kappa $
be a generic.
$G_\kappa \subseteq P_\kappa $
be a generic.
Then, in
![]() $V[G_\kappa ]$
,
$V[G_\kappa ]$
,
![]() $\kappa $
is a strongly compact, there is no measurable cardinals of the Mitchell order
$\kappa $
is a strongly compact, there is no measurable cardinals of the Mitchell order
![]() $\geq \eta $
and
$\geq \eta $
and
![]() $(\beth )_\eta $
holds.
$(\beth )_\eta $
holds.
Proof We repeat the arguments of 4.4, 4.5, only use
![]() $I=\{\nu <\kappa \mid \text { the Mitchell order of } \nu <\eta \}$
instead of
$I=\{\nu <\kappa \mid \text { the Mitchell order of } \nu <\eta \}$
instead of
![]() $I=\{\nu <\kappa \mid \nu \text { is not measurable} \}$
.
$I=\{\nu <\kappa \mid \nu \text { is not measurable} \}$
.
By Lemma 3.6,
![]() $\kappa $
is strongly compact in
$\kappa $
is strongly compact in
![]() $V[G_\kappa ]$
.
$V[G_\kappa ]$
.
Let W be a normal ultrafilter over
![]() $\kappa $
in
$\kappa $
in
![]() $V[G_\kappa ]$
. Note that W concentrates on cardinals which has the Mitchell order
$V[G_\kappa ]$
. Note that W concentrates on cardinals which has the Mitchell order
![]() $<\eta $
, in V. Just otherwise,
$<\eta $
, in V. Just otherwise,
![]() $\kappa $
will be a measurable in the ground model of
$\kappa $
will be a measurable in the ground model of
![]() $M_W$
of the Mitchell order
$M_W$
of the Mitchell order
![]() $\geq \eta $
, and so, a non-reflecting stationary set S or a Prikry sequence should be added there. Say that a non-reflecting stationary set S was added. But such S will be stationary in
$\geq \eta $
, and so, a non-reflecting stationary set S or a Prikry sequence should be added there. Say that a non-reflecting stationary set S was added. But such S will be stationary in
![]() $V[G_\kappa ]$
as well, since
$V[G_\kappa ]$
as well, since
![]() ${}^\kappa M_W\subseteq M_W$
. However,
${}^\kappa M_W\subseteq M_W$
. However,
![]() $\kappa $
is a measurable cardinal in
$\kappa $
is a measurable cardinal in
![]() $V[G_\kappa ]$
.
$V[G_\kappa ]$
.
By Hamkins [Reference Hamkins10], for non-reflecting stationary set or by 4.6, 4.11, for Prikry, no new fresh subsets are added to
![]() $\kappa ,\kappa ^+$
. So, by 2.1,
$\kappa ,\kappa ^+$
. So, by 2.1,
![]() $U=W\cap V$
is in V. Hence, U is a normal ultrafilter on
$U=W\cap V$
is in V. Hence, U is a normal ultrafilter on
![]() $\kappa $
in V which concentrates on measurable cardinals of the Mitchell order
$\kappa $
in V which concentrates on measurable cardinals of the Mitchell order
![]() $<\eta $
.
$<\eta $
.
Then, by
![]() $\kappa{-}$
completeness, there is
$\kappa{-}$
completeness, there is
![]() $\zeta <\eta $
such that U concentrates exactly on measurable cardinals of the Mitchell order
$\zeta <\eta $
such that U concentrates exactly on measurable cardinals of the Mitchell order
![]() $\zeta $
.
$\zeta $
.
By our assumption, U is a unique ultrafilter like these.
It is possible to continue and preserving strong compactness of
![]() $\kappa $
to turn it into the first measurable with exactly
$\kappa $
to turn it into the first measurable with exactly
![]() $\eta $
normal measures.
$\eta $
normal measures.
We force with the full support iteration of the Prikry forcings below
![]() $\kappa $
and turn every measurable
$\kappa $
and turn every measurable
![]() $\nu <\kappa $
into a cardinal of cofinality
$\nu <\kappa $
into a cardinal of cofinality
![]() $\omega $
.
$\omega $
.
Then by Magidor [Reference Magidor15],
![]() $\kappa $
will remain strongly compact cardinal. The number of normal measures over
$\kappa $
will remain strongly compact cardinal. The number of normal measures over
![]() $\kappa $
will remain
$\kappa $
will remain
![]() $\eta $
, by the arguments of Ben-Neria [Reference Ben-Neria4] and 4.13, used to replace the inner models part in his argument. So, the following holds:
$\eta $
, by the arguments of Ben-Neria [Reference Ben-Neria4] and 4.13, used to replace the inner models part in his argument. So, the following holds:
Theorem 4.17. Suppose that
![]() $\kappa $
is a supercompact cardinal,
$\kappa $
is a supercompact cardinal,
![]() $(\beth )_\eta $
holds and
$(\beth )_\eta $
holds and
![]() $2^\kappa =\kappa ^+$
.
$2^\kappa =\kappa ^+$
.
Assume that there is no inaccessible cardinals above
![]() $\kappa $
.
$\kappa $
.
Then there is a generic extension adding no new subsets to
![]() $\eta $
, in which
$\eta $
, in which
![]() $\kappa $
is a strongly compact, least measurable and there are exactly
$\kappa $
is a strongly compact, least measurable and there are exactly
![]() $\eta $
normal measures.
$\eta $
normal measures.
Proof (sketch)
We preserve the notation of [Reference Ben-Neria4]:
![]() .
.
Proceed as on p. 382 of [Reference Ben-Neria4].
Let W be a normal ultrafilter over
![]() $\kappa $
and
$\kappa $
and
![]() $\Gamma \in W$
.
$\Gamma \in W$
.
![]() $\Gamma \in W$
implies
$\Gamma \in W$
implies
![]() which in turn,implies that in
which in turn,implies that in
![]() $M_W = M[G_W]$
,
$M_W = M[G_W]$
,
![]() $|d^{-1}(\{\kappa \})|= 1$
. Denote
$|d^{-1}(\{\kappa \})|= 1$
. Denote
![]() $\mu = d^{-1}(\kappa )$
. Consider, in
$\mu = d^{-1}(\kappa )$
. Consider, in
![]() $V[G]$
,
$V[G]$
,
It is a
![]() $\kappa{-}$
complete ultrafilter over
$\kappa{-}$
complete ultrafilter over
![]() $\kappa $
which is Rudin–Keisler equivalent to W, since d is one to one on
$\kappa $
which is Rudin–Keisler equivalent to W, since d is one to one on
![]() . In particular,
. In particular,
![]() $j_W=j_{W_\mu }$
.
$j_W=j_{W_\mu }$
.
We have
![]() $\Delta \in W_\mu $
and
$\Delta \in W_\mu $
and
![]() $\mu \in j_W(\Delta )$
.
$\mu \in j_W(\Delta )$
.
Set
![]() $U_\mu =W_\mu \cap V$
. Then by 2.1 and 4.10,
$U_\mu =W_\mu \cap V$
. Then by 2.1 and 4.10,
![]() $U_\mu \in V$
.
$U_\mu \in V$
.
Lemma 4.18.
![]() $U_\mu $
is a normal ultrafilter in V.
$U_\mu $
is a normal ultrafilter in V.
Proof Proceed as in 3.6 of [Reference Ben-Neria4]. Suppose otherwise. Then there is a regressive f which represents
![]() $\kappa $
in the ultrapower
$\kappa $
in the ultrapower
![]() $M_{U_\mu }$
.
$M_{U_\mu }$
.
We will find a condition
![]() $q \in G$
such that
$q \in G$
such that
![]() , which is impossible, since
, which is impossible, since
![]() , and so,
, and so,
![]() $d(\mu )>\kappa $
in
$d(\mu )>\kappa $
in
![]() $M_W$
.
$M_W$
.
For every
![]() $p \in P_\kappa $
define
$p \in P_\kappa $
define
![]() $ p^{-f}\geq ^* p$
by reducing the (name of) every measure one set
$ p^{-f}\geq ^* p$
by reducing the (name of) every measure one set
![]() $X_\nu (p)$
for all
$X_\nu (p)$
for all
![]() $\nu \in \Delta $
such that
$\nu \in \Delta $
such that
The definition of
![]() $ p^{-f}$
implies that there is a finite subset
$ p^{-f}$
implies that there is a finite subset
![]() $b \subseteq \Delta $
such that for every
$b \subseteq \Delta $
such that for every
![]() $\nu \in \Delta \setminus b$
,
$\nu \in \Delta \setminus b$
,
![]() .
.
Now, using the density argument, find such
![]() $ p^{-f}\in G$
. Then
$ p^{-f}\in G$
. Then
since
![]() $\Delta \in W_\mu $
and
$\Delta \in W_\mu $
and
![]() $\kappa \leq k(\kappa )=k([f]_{U_\mu })=[f]_{W_\mu }=j_{W_\mu }(f)(\mu )=j_W(f)(\mu )$
, where
$\kappa \leq k(\kappa )=k([f]_{U_\mu })=[f]_{W_\mu }=j_{W_\mu }(f)(\mu )=j_W(f)(\mu )$
, where
![]() $k:M_{U_\mu }\to M_{W_\mu }$
defined as usual:
$k:M_{U_\mu }\to M_{W_\mu }$
defined as usual:
![]() $k([h]_{U_\mu })=[h]_{W_\mu }$
.
$k([h]_{U_\mu })=[h]_{W_\mu }$
.
Let us argue now that
![]() $W=U^{\times }_\mu $
. It is sufficient to prove that
$W=U^{\times }_\mu $
. It is sufficient to prove that
![]() $W\supseteq U^{\times }_\mu $
. As in [Reference Ben-Neria4], it is enough to show that
$W\supseteq U^{\times }_\mu $
. As in [Reference Ben-Neria4], it is enough to show that
![]() $d"A \in W$
, for every
$d"A \in W$
, for every
![]() $A \in U_\mu $
.
$A \in U_\mu $
.
Let
![]() $A\in U_\mu $
, then
$A\in U_\mu $
, then
![]() $A\in W_\mu \supseteq U_\mu $
, and so,
$A\in W_\mu \supseteq U_\mu $
, and so,
![]() $d"A \in W=d_*W_\mu $
.
$d"A \in W=d_*W_\mu $
.
It is possible to give an alternative proof of Apter and Cummings [Reference Apter and Cummings2] result about tall cardinals. We use exactly the same construction as in the previous theorem, only a strong cardinal replaces a supercompact.
Theorem 4.19. Suppose that
![]() $\kappa $
is a strong cardinal,
$\kappa $
is a strong cardinal,
![]() $(\beth )_\eta $
holds and
$(\beth )_\eta $
holds and
![]() $2^\kappa =\kappa ^+$
.
$2^\kappa =\kappa ^+$
.
Assume that there is no inaccessible cardinals above
![]() $\kappa $
.
$\kappa $
.
Then there is a generic extension adding no new subsets to
![]() $\eta $
, in which
$\eta $
, in which
![]() $\kappa $
is a tall cardinal, least measurable and there are exactly
$\kappa $
is a tall cardinal, least measurable and there are exactly
![]() $\eta $
normal measures.
$\eta $
normal measures.
Note that by results of Mitchell, see [Reference Mitchell and Foreman16], the property
![]() $(\beth )_\eta $
holds in inner models with a strong cardinal.
$(\beth )_\eta $
holds in inner models with a strong cardinal.
5 Elementary embeddings
Let
![]() $P_\kappa $
be either Easton or full or nonstationary support iteration of Prikry type forcing notions,
$P_\kappa $
be either Easton or full or nonstationary support iteration of Prikry type forcing notions,
![]() $G_\kappa \subseteq P_\kappa $
generic, W a normal ultrafilter over
$G_\kappa \subseteq P_\kappa $
generic, W a normal ultrafilter over
![]() $\kappa $
in
$\kappa $
in
![]() $V[G_\kappa ]$
and
$V[G_\kappa ]$
and
![]() $U=V\cap W \in V$
. So,
$U=V\cap W \in V$
. So,
![]() $j_W: V[G] \to M_W=M[j_W(G_\kappa )]$
.
$j_W: V[G] \to M_W=M[j_W(G_\kappa )]$
.
In this section we would like to analyze
![]() $j_W\upharpoonright V:V\to M$
$j_W\upharpoonright V:V\to M$
In order to do so, let us study the elementary embedding
![]() $k:M_U \to M$
defined by setting
$k:M_U \to M$
defined by setting
![]() $k([f]_U)=[f]_W$
.
$k([f]_U)=[f]_W$
.
If k is the identity, then
![]() $j_W$
is just an extension of
$j_W$
is just an extension of
![]() $j_U$
. However, even when the Hamkins Gap Theorem [Reference Hamkins10] applies, k need not be the identity. Starting with a single measurable, it is possible to construct a generic extension in which
$j_U$
. However, even when the Hamkins Gap Theorem [Reference Hamkins10] applies, k need not be the identity. Starting with a single measurable, it is possible to construct a generic extension in which
![]() $j_W\upharpoonright V$
is, for example, the ultrapower embedding with
$j_W\upharpoonright V$
is, for example, the ultrapower embedding with
![]() $U\times U$
.
$U\times U$
.
Let us first try to understand possibilities for the critical point of k, assuming that k is not the identity map.
Clearly,
![]() ${\textrm {crit}}(k)>\kappa ^+$
, due to the canonical functions.
${\textrm {crit}}(k)>\kappa ^+$
, due to the canonical functions.
Let us start with the Easton support iteration.
5.1 Easton support
We will start with two examples in which
![]() ${\textrm {crit}}(k)=(\kappa ^{++})^{M_U}$
.
${\textrm {crit}}(k)=(\kappa ^{++})^{M_U}$
.
Example 1. Assume GCH. Let
![]() $A \subseteq \kappa $
be such that
$A \subseteq \kappa $
be such that
![]() $\kappa \setminus A$
contains
$\kappa \setminus A$
contains
![]() $\kappa{-}$
many measurable cardinals.
$\kappa{-}$
many measurable cardinals.
Let
![]() be the Easton support iteration, where for every
be the Easton support iteration, where for every
![]() $\beta <\kappa $
,
$\beta <\kappa $
,
![]() $Q_\beta $
is trivial, unless
$Q_\beta $
is trivial, unless
![]() $\beta $
is a measurable in
$\beta $
is a measurable in
![]() $V^{P_\beta }$
and
$V^{P_\beta }$
and
![]() $\beta \not \in A$
. If this is the case, then
$\beta \not \in A$
. If this is the case, then
![]() $Q_\beta $
is the Prikry forcing with a normal measure over
$Q_\beta $
is the Prikry forcing with a normal measure over
![]() $\beta $
.
$\beta $
.
Suppose that there is a
![]() $(\kappa ,\kappa ^{++})$
-extender E in V such that A belongs to its normal measure
$(\kappa ,\kappa ^{++})$
-extender E in V such that A belongs to its normal measure
![]() $E(\kappa )=\{X\subseteq \kappa \mid \kappa \in j_E(X)\}$
. Denote
$E(\kappa )=\{X\subseteq \kappa \mid \kappa \in j_E(X)\}$
. Denote
![]() $E(\kappa )$
by U.
$E(\kappa )$
by U.
Set
![]() $\delta =(\kappa ^{++})^{M_{E(\kappa )}}$
. Consider F on
$\delta =(\kappa ^{++})^{M_{E(\kappa )}}$
. Consider F on
![]() $\kappa ^2$
defined as follows:
$\kappa ^2$
defined as follows:
Denote by
![]() $i:M_{E(\kappa )} \to M_E$
the natural embedding derived from
$i:M_{E(\kappa )} \to M_E$
the natural embedding derived from
![]() $j_E$
. Then
$j_E$
. Then
![]() $\delta $
will be its critical point. Let
$\delta $
will be its critical point. Let
![]() $[id]_F=(\kappa , \delta _F)$
. Then
$[id]_F=(\kappa , \delta _F)$
. Then
![]() $|\delta _F|=\kappa ^+$
, since
$|\delta _F|=\kappa ^+$
, since
![]() $M_E\models |\delta |=\kappa ^+$
.
$M_E\models |\delta |=\kappa ^+$
.
Now let
![]() $G_\kappa $
be a generic subset of
$G_\kappa $
be a generic subset of
![]() $P_\kappa $
.
$P_\kappa $
.
Define an extension W of
![]() $U=E(\kappa )$
in
$U=E(\kappa )$
in
![]() $ V[G_\kappa ]$
as follows.
$ V[G_\kappa ]$
as follows.
Consider
![]() $j_F:V\to M_F$
. Let
$j_F:V\to M_F$
. Let
![]() $\mu $
be the least measurable of
$\mu $
be the least measurable of
![]() $M_F$
above
$M_F$
above
![]() $\kappa $
which is not in
$\kappa $
which is not in
![]() $j_F(A)$
.
$j_F(A)$
.
We define
![]() $\leq ^*$
-increasing sequence of conditions
$\leq ^*$
-increasing sequence of conditions
![]() ${\langle } q_\alpha \mid \alpha <\kappa ^+{\!\rangle }$
in
${\langle } q_\alpha \mid \alpha <\kappa ^+{\!\rangle }$
in
![]() $j_F(P_\kappa )/G_\kappa $
meeting all
$j_F(P_\kappa )/G_\kappa $
meeting all
![]() $\leq ^*$
-dense sets of
$\leq ^*$
-dense sets of
![]() $M_F[G_\kappa ]$
, only start with a condition which puts
$M_F[G_\kappa ]$
, only start with a condition which puts
![]() $\delta $
to be the first member of the Prikry sequence for
$\delta $
to be the first member of the Prikry sequence for
![]() $\mu $
.
$\mu $
.
Set
![]() $X\in W$
iff there is
$X\in W$
iff there is
![]() $p \in G_\kappa $
and
$p \in G_\kappa $
and
![]() $\alpha <\kappa ^+$
such that
$\alpha <\kappa ^+$
such that
![]() .
.
Then W will be a normal measure over
![]() $\kappa $
in
$\kappa $
in
![]() $V[G]$
,
$V[G]$
,
![]() $W\cap V=U$
.
$W\cap V=U$
.
In addition we will have
![]() $\delta <(\kappa ^{++})^{M_W}$
, since the function f
$\delta <(\kappa ^{++})^{M_W}$
, since the function f
will represent
![]() $\delta $
in
$\delta $
in
![]() $M_W$
and the set
$M_W$
and the set
by its definition.
Example 2. Let us use adding a non-reflecting stationary sets instead of the Prikry forcing.
Proceed exactly as in the first example only start the master condition sequence
![]() ${\langle } q_\alpha \mid \alpha <\kappa ^+{\!\rangle }$
in
${\langle } q_\alpha \mid \alpha <\kappa ^+{\!\rangle }$
in
![]() $j_F(P_\kappa )/G_\kappa $
meeting all
$j_F(P_\kappa )/G_\kappa $
meeting all
![]() $\leq ^*$
-dense sets of
$\leq ^*$
-dense sets of
![]() $M_F[G_\kappa ]$
, with only start with a condition which puts
$M_F[G_\kappa ]$
, with only start with a condition which puts
![]() $\delta $
to be the first member of the generic stationary non-reflecting subset of
$\delta $
to be the first member of the generic stationary non-reflecting subset of
![]() $\mu $
.
$\mu $
.
Then, as above, we will have
![]() ${\textrm {crit}}(k)=(\kappa ^{++})^{M_U}$
.
${\textrm {crit}}(k)=(\kappa ^{++})^{M_U}$
.
Recall that by Hamkins [Reference Hamkins10],
![]() $j_W\upharpoonright V$
, and so k, are definable over V, whenever the forcing
$j_W\upharpoonright V$
, and so k, are definable over V, whenever the forcing
![]() $P_\kappa $
has a gap below
$P_\kappa $
has a gap below
![]() $\kappa $
. Also,
$\kappa $
. Also,
![]() $j_W(V)=\bigcup _{\alpha \in On}j_W((V_\alpha )^V)$
is definable in V.
$j_W(V)=\bigcup _{\alpha \in On}j_W((V_\alpha )^V)$
is definable in V.
Remark. There is no need in a
![]() $(\kappa , \kappa ^{++})$
-extender in order to produce such examples, i.e., with
$(\kappa , \kappa ^{++})$
-extender in order to produce such examples, i.e., with
![]() ${\textrm {crit}}(k)=(\kappa ^{++})^{M_U}$
. It is possible to start with a measurable
${\textrm {crit}}(k)=(\kappa ^{++})^{M_U}$
. It is possible to start with a measurable
![]() $\kappa $
which is a limit of measurable cardinals.
$\kappa $
which is a limit of measurable cardinals.
Let us sketch the idea. Start with V in which
![]() $\kappa $
is a limit of measurable cardinals. Let U be a normal ultrafilter over
$\kappa $
is a limit of measurable cardinals. Let U be a normal ultrafilter over
![]() $\kappa $
which concentrates on non-measurable cardinals. We will use an extensions of
$\kappa $
which concentrates on non-measurable cardinals. We will use an extensions of
![]() $U\times U$
and of
$U\times U$
and of
![]() $U\times U\times U$
as replacements of U and F of the previous construction.
$U\times U\times U$
as replacements of U and F of the previous construction.
Denote
![]() $j_U(\kappa )$
by
$j_U(\kappa )$
by
![]() $\kappa _1$
,
$\kappa _1$
,
![]() $j_{U\times U}(\kappa )$
by
$j_{U\times U}(\kappa )$
by
![]() $\kappa _2$
and
$\kappa _2$
and
![]() $j_{U\times U\times U}(\kappa )$
by
$j_{U\times U\times U}(\kappa )$
by
![]() $\kappa _3$
. We force (with a suitable preparation) a Cohen function
$\kappa _3$
. We force (with a suitable preparation) a Cohen function
![]() $f_\kappa :\kappa \to \kappa $
. Then do collapses
$f_\kappa :\kappa \to \kappa $
. Then do collapses
![]() $Col(\nu ^+,<f_\kappa (\nu ))$
, on a set
$Col(\nu ^+,<f_\kappa (\nu ))$
, on a set
![]() $\nu $
’s in U. Set the value of the corresponding
$\nu $
’s in U. Set the value of the corresponding
![]() $f_{\kappa _2}(\kappa )$
to be
$f_{\kappa _2}(\kappa )$
to be
![]() $\kappa _1$
in
$\kappa _1$
in
![]() $M_{U\times U}$
. Set
$M_{U\times U}$
. Set
![]() $f_{\kappa _3}(\kappa )$
to be
$f_{\kappa _3}(\kappa )$
to be
![]() $\kappa _2$
in
$\kappa _2$
in
![]() $M_{U\times U\times U}$
.
$M_{U\times U\times U}$
.
5.2 More examples
We would like to use iterations of Prikry forcings in order to construct examples with
![]() $j_W\upharpoonright V$
not definable in V, but still
$j_W\upharpoonright V$
not definable in V, but still
![]() $W\cap V\in V$
. This type of situation is different from those produced by the Hamkins Gap Theorem [Reference Hamkins10].
$W\cap V\in V$
. This type of situation is different from those produced by the Hamkins Gap Theorem [Reference Hamkins10].
Let us observe that the methods above do not allow to construct models with
![]() $j_W(V)$
definable (in V), but
$j_W(V)$
definable (in V), but
![]() $j_W\upharpoonright V$
is not.
$j_W\upharpoonright V$
is not.
The next proposition shows a definability of
![]() $j_W(V)$
together with non-definability of
$j_W(V)$
together with non-definability of
![]() $j_W\upharpoonright V$
should be rather strong, if consistent.
$j_W\upharpoonright V$
should be rather strong, if consistent.
Proposition 5.1. Suppose that the core model
![]() $\mathcal K$
exists and V is a generic extension of
$\mathcal K$
exists and V is a generic extension of
![]() $\mathcal K$
, i.e., for some forcing notion
$\mathcal K$
, i.e., for some forcing notion
![]() $P\in \mathcal K$
and some
$P\in \mathcal K$
and some
![]() $H\subseteq P$
generic over
$H\subseteq P$
generic over
![]() $\mathcal K$
,
$\mathcal K$
,
![]() $V=\mathcal K[H]$
.Footnote
13
$V=\mathcal K[H]$
.Footnote
13
Suppose that
![]() $V^*\supseteq V$
is a generic extension of V (or both have the same core model) and
$V^*\supseteq V$
is a generic extension of V (or both have the same core model) and
![]() $j^*:V^* \to M^*$
is an elementary embedding such that
$j^*:V^* \to M^*$
is an elementary embedding such that
![]() $j^*(V)$
is definable in V.
$j^*(V)$
is definable in V.
Then, also,
![]() $j^*\upharpoonright V$
is definable in V.
$j^*\upharpoonright V$
is definable in V.
Proof Denote the V-class
![]() $j^*(V)$
by M. Let
$j^*(V)$
by M. Let
![]() $j=j^*\upharpoonright V$
.
$j=j^*\upharpoonright V$
.
By elementarity,
![]() $M=j(\mathcal K)[H']$
, where
$M=j(\mathcal K)[H']$
, where
![]() $H'=j(H)$
is
$H'=j(H)$
is
![]() $\mathcal K^M=j(\mathcal K)$
-generic over
$\mathcal K^M=j(\mathcal K)$
-generic over
![]() $\mathcal K^M$
.
$\mathcal K^M$
.
![]() $\mathcal K^M$
is a class in V, so we can compare inside V with the real
$\mathcal K^M$
is a class in V, so we can compare inside V with the real
![]() $\mathcal K$
(by using least disagreements). This will produce the embedding
$\mathcal K$
(by using least disagreements). This will produce the embedding
![]() $i:\mathcal K \to \mathcal K^M$
. Given
$i:\mathcal K \to \mathcal K^M$
. Given
![]() $\mathcal K^M$
, such i is unique.
$\mathcal K^M$
, such i is unique.
So,
![]() $j\upharpoonright K=i$
and i is definable in K.
$j\upharpoonright K=i$
and i is definable in K.
Now,
So, we can define j using i and
![]() $H'$
.
$H'$
.
Remark 5.2. The assumption that
![]() $V^*\supseteq V$
is a generic extension of V or at least both have the same core model, is necessary. Just otherwise, we can take
$V^*\supseteq V$
is a generic extension of V or at least both have the same core model, is necessary. Just otherwise, we can take
![]() $V=L[U]$
and
$V=L[U]$
and
![]() $V^*=L[\vec {W}]$
, where
$V^*=L[\vec {W}]$
, where
![]() $\vec {W}$
contains at least two measures, and then, form
$\vec {W}$
contains at least two measures, and then, form
![]() $j^*$
by iterating the second measure.
$j^*$
by iterating the second measure.
Start with a GCH model with a measurable cardinal
![]() $\kappa $
which is a limit of measurables. We can assume, for simplicity that
$\kappa $
which is a limit of measurables. We can assume, for simplicity that
![]() $V=\mathcal K$
, but it is not necessary.
$V=\mathcal K$
, but it is not necessary.
First we force with Easton support product the Cohen forcing which adds a Cohen function
![]() $h_\nu :\nu ^+\to \nu ^+$
to every inaccessible
$h_\nu :\nu ^+\to \nu ^+$
to every inaccessible
![]() $\nu <\kappa $
. Let
$\nu <\kappa $
. Let
![]() ${\langle } R_\alpha , {C}_\beta \mid \beta <\kappa , \alpha \leq \kappa {\rangle }$
be this forcing.
${\langle } R_\alpha , {C}_\beta \mid \beta <\kappa , \alpha \leq \kappa {\rangle }$
be this forcing.
By Kunen–Paris (see for example Kanamori’s book [Reference Kanamori12] on this), it preserves measurability of every measurable cardinal.
Let us be more specific here.
Let
![]() $\eta \leq \kappa $
be a measurable in the ground model. Fix some normal ultrafilter
$\eta \leq \kappa $
be a measurable in the ground model. Fix some normal ultrafilter
![]() $U(\eta )$
which concentrates on non-measurable cardinals. Let
$U(\eta )$
which concentrates on non-measurable cardinals. Let
![]() $j_\eta =j_{U(\eta )}:V\to M_{U(\eta )}=M_\eta $
be the corresponding elementary embedding.
$j_\eta =j_{U(\eta )}:V\to M_{U(\eta )}=M_\eta $
be the corresponding elementary embedding.
We define in the extension
![]() $\eta $
-many normal ultrafilters which extend
$\eta $
-many normal ultrafilters which extend
![]() $U(\eta )$
, but have the same ultrapower.
$U(\eta )$
, but have the same ultrapower.
Consider the relevant for
![]() $\eta $
part of the forcing, i.e.,
$\eta $
part of the forcing, i.e.,
![]() $R_{\eta }$
. It adds Cohen functions
$R_{\eta }$
. It adds Cohen functions
![]() $\vec {h}={\langle } h_\nu \mid \nu < \eta \text { is an inaccessible} {\rangle }$
.
$\vec {h}={\langle } h_\nu \mid \nu < \eta \text { is an inaccessible} {\rangle }$
.
Pick in
![]() $V[\vec {h}]$
, a sequence
$V[\vec {h}]$
, a sequence
![]() such that
such that
-
•
 , for every inaccessible
, for every inaccessible
 $\nu <\eta $
,
$\nu <\eta $
, -
•
 is
is
 $M_\eta {-}$
generic for the forcing
$M_\eta {-}$
generic for the forcing
 $j_\eta (R_{\eta })$
.
$j_\eta (R_{\eta })$
.
Denote
![]() $V[\vec {h}]$
by
$V[\vec {h}]$
by
![]() $V'$
and
$V'$
and
![]() $M_\eta [\vec {h}']$
by
$M_\eta [\vec {h}']$
by
![]() $M_\eta '$
. Then
$M_\eta '$
. Then
![]() $j_\eta $
extends to
$j_\eta $
extends to
![]() and
and
![]() is the ultrapower of
is the ultrapower of
![]() $V'$
by an extension of
$V'$
by an extension of
![]() of
of
![]() $U_\eta $
.
$U_\eta $
.
Let
![]() . Now, for every
. Now, for every
![]() $i<\eta $
, let us change the value
$i<\eta $
, let us change the value
![]() to i. Denote the result by
to i. Denote the result by
![]() .
.
Set
Clearly that such
![]() is in
is in
![]() $M_\eta '$
and it is
$M_\eta '$
and it is
![]() $j_\eta (R_{\eta })$
-generic over
$j_\eta (R_{\eta })$
-generic over
![]() $M_\eta $
.
$M_\eta $
.
We have so,
![]() .
.
Define
according to
, i.e., by setting
Define the corresponding ultrafilter
Note that such
![]() ’s will be different.
’s will be different.
Namely, let
![]() $i, i'<\eta , i\not = i'$
. Then the set
$i, i'<\eta , i\not = i'$
. Then the set
and the set
Clearly this sets are disjoint.
Now, let us define in
![]() $V'$
an iteration of Prikry forcings
$V'$
an iteration of Prikry forcings
![]() (either Easton, non-stationary or full support can be used for this).
(either Easton, non-stationary or full support can be used for this).
Set
![]() .
.
Let
![]() ${\langle } \eta _\xi \mid \xi <\kappa {\rangle }$
be the increasing enumeration of all measurable cardinals
${\langle } \eta _\xi \mid \xi <\kappa {\rangle }$
be the increasing enumeration of all measurable cardinals
![]() $\tau <\kappa $
.
$\tau <\kappa $
.
Define an iteration
![]() with either nonstationary or full support.Footnote
14
with either nonstationary or full support.Footnote
14
For every
![]() $\beta <\kappa $
, let
$\beta <\kappa $
, let
![]() $Q_\beta $
be trivial, unless
$Q_\beta $
be trivial, unless
![]() $\beta $
is a measurable in
$\beta $
is a measurable in
![]() $V^{'P_\beta }$
. Let
$V^{'P_\beta }$
. Let
![]() $Q_{\eta _0}$
be the Prikry forcing with
$Q_{\eta _0}$
be the Prikry forcing with
![]() . Let
. Let
![]() be the canonical name of the Prikry sequence for
be the canonical name of the Prikry sequence for
![]() $Q_{\eta _0}$
.
$Q_{\eta _0}$
.
Now, suppose that
![]() $ 0<\xi <\kappa $
and
$ 0<\xi <\kappa $
and
![]() $P_{\eta _\xi }$
is defined. Define
$P_{\eta _\xi }$
is defined. Define
![]() $Q_{\eta _\xi }$
.
$Q_{\eta _\xi }$
.
If
![]() $\xi $
is a limit ordinal then let
$\xi $
is a limit ordinal then let
![]() $Q_{\eta _\xi }$
be the Prikry forcing with
$Q_{\eta _\xi }$
be the Prikry forcing with
![]() .
.
If
![]() $\xi =\xi '+k$
, where
$\xi =\xi '+k$
, where
![]() $\xi '$
is a limit and
$\xi '$
is a limit and
![]() $k,0<k<\omega $
, then let
$k,0<k<\omega $
, then let
![]() $Q_{\eta _\xi }$
be the Prikry forcing with
$Q_{\eta _\xi }$
be the Prikry forcing with
![]() .
.
Let
![]() $G_\kappa $
be a generic subset of
$G_\kappa $
be a generic subset of
![]() $P_\kappa $
. Then U extends in
$P_\kappa $
. Then U extends in
![]() $V'[G_\kappa ]$
to a normal ultrafilter W. We have
$V'[G_\kappa ]$
to a normal ultrafilter W. We have
![]() $W\cap V=U\in V'$
.
$W\cap V=U\in V'$
.
Let us argue that
![]() $j_W\upharpoonright V'$
is not definable in
$j_W\upharpoonright V'$
is not definable in
![]() $V'$
.
$V'$
.
Recall that
![]() $j_W= k\circ j_U$
.
$j_W= k\circ j_U$
.
Thus,
![]() $j_W\upharpoonright V'$
is an iterated ultrapower of
$j_W\upharpoonright V'$
is an iterated ultrapower of
![]() $V'$
by its measures. So, U must be applied first, since
$V'$
by its measures. So, U must be applied first, since
![]() $\kappa $
is the critical point and it is not measurable in the ultrapower. Let
$\kappa $
is the critical point and it is not measurable in the ultrapower. Let
![]() be the list of all measurable cardinals below
be the list of all measurable cardinals below
![]() $j_U(\kappa )$
in
$j_U(\kappa )$
in
![]() $M_U$
. So the next cardinal addressed should be
$M_U$
. So the next cardinal addressed should be
![]() which is the first measurable above
which is the first measurable above
![]() $\kappa $
in
$\kappa $
in
![]() $M_U$
. The recipe that now we should apply the Prikry forcing with
$M_U$
. The recipe that now we should apply the Prikry forcing with
![]() . However,
. However,
![]() is an ordinal of cofinality
is an ordinal of cofinality
![]() $\kappa ^+$
and cardinals are preserved. So, an iterated ultrapower with the measure
$\kappa ^+$
and cardinals are preserved. So, an iterated ultrapower with the measure
![]() should be used
should be used
![]() $\omega $
-many times in order to produce the desired Prikry sequence, see [Reference Gitik and Kaplan8], for a non-stationary support and [Reference Kaplan13], for a full support. Then we proceed to
$\omega $
-many times in order to produce the desired Prikry sequence, see [Reference Gitik and Kaplan8], for a non-stationary support and [Reference Kaplan13], for a full support. Then we proceed to
![]() ,
,
![]() , etc.
, etc.
Now if
![]() $j_W\upharpoonright V'$
was definable in
$j_W\upharpoonright V'$
was definable in
![]() $V'$
, then we will be able to reconstruct the Prikry over
$V'$
, then we will be able to reconstruct the Prikry over
![]() $V'$
sequence
$V'$
sequence
![]() ${\langle } \eta _0^n\mid n<\omega {\rangle }$
.
${\langle } \eta _0^n\mid n<\omega {\rangle }$
.
Namely, consider the sets
![]() in
in
![]() $M_U$
. Only image
$M_U$
. Only image
![]() $k(X_{\eta _\kappa , i})$
from them which contains a final segment of the Prikry sequence for
$k(X_{\eta _\kappa , i})$
from them which contains a final segment of the Prikry sequence for
![]() is
is
![]() . Which means that
. Which means that
![]() $\eta ^0_0$
reconstructible from the iteration. Proceed similar with each
$\eta ^0_0$
reconstructible from the iteration. Proceed similar with each
![]() $n, 0<n<\omega $
.
$n, 0<n<\omega $
.
Acknowledgments
The authors are grateful to the referee of the paper for his kind corrections, remarks and suggestions. The work was partially supported by ISF grant No 1216/18.