Introduction
Radio-echo sounding (RES) of ice sheets is commonly used to investigate their internal structure and extent at frequencies between ten and several hundred MHz (Reference Robin, Evans and BaileyRobin and others, 1969; Reference Bogorodsky, Bentley and GudmandsenBogorodsky and others, 1985). Changes in radar returns are caused by changes in the complex permittivity,ε*=ε'-iε", of layers of ice that comprise the ice sheets. Internal reflection horizons from within the ice sheets are often observed and several causes of these changes in the complex permittivity have been proposed. They are (1) density fluctuation, (2) changes in conductivity due to changes in acid concentration, and (3) changes in crystal-orientation fabrics (ice fabrics)..
Density fluctuation can account for echoes at depths above about 1500m, e.g. Reference Paren and RobinParen and Robin, 1975; Reference CloughClough, 1977). I\" ear the surface of ice sheets, ice lenses or more extensive features of melt or depth hoar may cause changes in the permittivity and they are large enough to cause large reflections. However, since density contrasts between adjacent layers arc smoothed out rapidly with increasing depth, the reflection horizons at deeper layers cannot be explained by this mechanism.
Several of the internal reflection horizons have been correlated to depths corresponding to the dates of large volcanic eruptions (Reference MillarMillar, 1981a, Reference Millar1981b). Reference HammerHammer (1980) has shown that volcanic eruptions can produce an increase in the level of acidity of precipitation in polar regions for one or more years after the eruption. It is therefore tempting to assign changes in conductivity at radar frequencies that lead to internal reflection horizons to changes in acidity. Reference MooreMoore (1988) used changes in conductivity at low frequency (LF) arising from the presence of both acid and salt impurities in an ice core to model the radar reflections that would come from an ice sheet from which the core was sampled. However, until recently, there has been no study on the effect of acid impurities on the e1ectrical properties of ice at frequencies appropriate for RES. In order to investigate the effect of acid on the dielectric properties of ice at the microwave frequency, Reference Fujita, Shiraishi and MaeFujita and others (1992) measured the complex permittivity of artificially grown polycrystalline ice containing strong acids (H2S04, HN03 and HCL) at 9.7GHz. They found a linear relation between the complex permittivity and molarity of doped acid, which is independent of the kind of acid. Using this result and the LF-conductivity data of ice cores, Reference Moore and FujitaMoore and Fujita (1993) compared the microwave and LF range conductivity in artificial and natural ice containing an acid impurity as a function of acid concentration and temperature. They found that the conductivity is almost the same at a microwave frequency and at an LF, and showed that there is no evidence of dielectric dispersion in conductivity arising from the presence of acid impurity between two frequency ranges. In addition, they found that the conductivity arising from the presence of acid is well fitted by a model where concentrated liquid acid at three grain boundaries forms a network, earlier proposed by Reference WoW and ParenWolffand Paren (1984) as an explanation for d.c. conductivity of polar ice. Therefore, the properties of internal reflection can now be estimated as a function of acid concentration, frequency and temperature, because conductivity arising from the presence of acid is known as a function of them.
On the other hand, it is well known that ice fabrics in polar ice sheets change with depth. Reference HarrisonHarrison 1973) proposed an hypothesis that the variation of ice fabrics was one of the dominant causes of internal reflections. However, this was not supported, mainly because the dielectric anisotropy of ice, the difference between ε∥c, ε' parallel to the c axis and ε⊥cε' perpendicular to the c axis, was unknown quantitatively at frequencies used in RES until recently. Reference CloughClough (1977) estimated that sharp changes in crystal orientation may produce significant reflection coefficients if the anisotropy is as high as 1%. Reference Bogorodsky, Bentley and GudmandsenGow and Wi11iamson (1976) showed examples of sharp changes in crystal orientation. They showed that the c axes of ice containing volcanic ash are more tightly clustered around the vertical than those in enclosing ice layers in the Byrd Station core. Reference Robin and MillarRobin and Millar (1982) discussed the possibility that the presence of additional acid impurities in ice may change the mechanical properties of ice and that consequently the radio-echo layers may indicate the easy glide plane and hence the ice fabrics, as a possible explanation of the coincidence between the depths of internal reflection layers and the depths corresponding to the ages of the past volcanic eruptions.
Recently, Reference Fujita, Mae and MatsuokaFujita and others (1993a) discovered that the dielectric anisotropy, Δε' = ε' ∥c - ε' ⊥c is 0.037 (± o.oo7) or 1.2% (± 0.2%) at 9.7 GHz. This value is large enough that changes in ice fabrics produce detectable internal reflections. Since Reference Fujita, Matsuoka, Morishima and MaeFujita and others (1993b) confirmed that Δε' is of a similar magnitude at frequencies around 5 MHz, Δε' is constant at frequencies used in RES. Using Δε ', Reference Fujita and MaeFujita and Mae (1993) calculated the dielectric permittivity tensors of ice in the ice sheet at Mizuho Station, Antarctica, using the ice fabric data of the 700 m Mizuho ice core. They showed that the changes in permittivity tensors between adjacent depths were of the order of 0.1% and were large enough to produce dominant reflections.
In the light of new knowledge on the dielectric properties of ice, in this paper, we estimate the possible maximum reflection coefficients due to changes in ice fabrics and those due to changes in conductivity, as a function of frequency and temperature. The purpose of the estimation is to assess and to compare the significance of these two mechanisms. This leads to a conclusion that changes in ice fabrics are the predominant cause of the internal reflections at deep layers. In contrast, changes in conductivity can be one of the dominant causes only when frequencies below about 60 MHz are used. When even lower frequencies are used, it is the predominant cause.
Reflection due to changes in crystal. orientation fabrics
Reflection-coefficient calculation
When electromagnetic waves are normally incident on a single layer which has thickness l, with bulk impedance . Z2, from a side of an enclosing layer with bulk impedance Z1, the power-reflection coefficient (PRC) has been given by Reference Paren and RobinParen and Robin (1975) and Reference ParenParen (1981) as follows


Here, Rs is the reflection coefficient at a single plane boundary. The factor "F" expresses the effect of interference between waves that are reflected at the upper boundary and lower boundary of the layer. λm is the wavelength in the ice. Since F is a periodical function of l and λm, the PRC is also a periodical function of them. The properties of Rs are discussed first in this paper. The effect of F is discussed later.
If the change in impedance is only due to a change in permittivity from ε' to ε' + δε' then Rs has been given by Reference Paren and RobinParen and Robin (1975) and Reference ParenParen (1981) as

PRC due to density fluctuation and changes in ice fabrics is calculated by Equations (1a) and (2) .If the change in impedance is only due to changes in dielectric loss, from tan δ to tan δ+ Δ (tan δ), then Rs has been given by Reference Paren and RobinParen and Robin (1975) and Reference ParenParen (1981) as

Dielectric anisotropy of ϵ'
Reference Fujita, Mae and MatsuokaFujita and others (1993a) measured ε'∥c and ε'∥c at 9.7 GHz by the wave-guide method at temperatures between 32.5° and 2.5°C. The difference between them that they found, i.e. dielectric anisotropy, was given by following equation
where T is the temperature expressed in °C. The temperature-dependence of Δε' is negligibly small in the temperature range of the cryosphere. In addition, .de I is constant at frequencies used in RES.
The contribution of Δε' to the changes in permittivity in ice sheets is formulated below. Reference Fujita and MaeFujita and Mae (1993) expressed the component of the dielectric permittivity tensor of polycrystalline ice as follows

Here, Da was introduced as a factor that expresses the degree of the contribution of Δε' to the component of the dielectric permittivity tensor. It was assumed that polycrystalline ice is composed of limited number, N, of crystal grains. We took the angle between the electric field vector of incident wave and the c axis of the jth grain as θj. When electromagnetic waves reflect due to only the changes in ice fabrics, Rs becomes, using Δε' and Da, from Equation (2)(Reference Fujita and MaeFujita and Mac, 1993)

where δ Da is the change of Da at boundary between adjacent ice layers.
Changes in crystal-orientation fabrics
In order to estimate possible 8Da in Equation (7) and the thickness of the layers in actual ice sheets, we briefly introduce some examples of changes in ice fabrics with depth in actual polar ice sheets. An example is the study by Reference Gow and WilliamsonGow and Williamson (1976) in which they showed the variation of ice fabrics with depth in the Byrd Station ice core in Antarctica. They investigated the ice fabrics of six cloudy bands that contain visible volcanic glass shards and the ice fabrics of the enclosing ice, and they found that crystal axes tend to be more tightly clustered about the vertical in the cloudy bands than those in the enclosing ice at depths below 910 m. The thicknesses of each band were in the range from 1mm to 6 cm. They reported that as many as 2000 individual bands were present in the Byrd Station ice cores, mostly in the zone from 1200 to 1800m (Reference Gow and WilliamsonGow and Williamson, 1971). Because the crystal sizes of such layers were always very much smaller than those of the enclosing ice and had a fragmented appearance, they pointed out that such layers may also constitute zones of actual shear displacement in the ice sheet. The ice-fabric pattern that they observed ill the cloudy bands is often called a vertical single-maximum pattern that is formed in a high-shear zone (Reference Russell-Head and Budde.g. Russell-Head and Budd, 1979). Another example is the study by Reference Thwaites, Wilson and MeCrayThwaites and others (1984), who investigated the relationship between borehole-closure rate and ice fabrics in the ice cores from Cape Folger, Antarctica. They found that closure rate was highly variable from one depth to another and was closely related to the fabrics of the ice. The zones at which the non-uniform closure occurred were 0.5-3 m wide. This indicates that the thicknesses of the layers in which the ice fabrics are uniform are of the same magnitude, at least at Cape Folger.
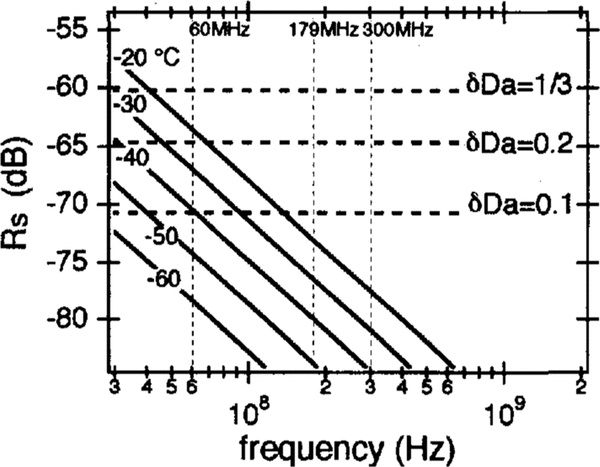
To calculate possible Rs from Equation (7),the parameter δDa should be estimated. It is apparent that δDa can easily be 0.1, on the basis of the variations in the dielectric permittivity tensors of the Mizuho ice core given by Reference Fujita and MaeFujita and Mae(1993).A value of 0.1 means that 10% of . Δε' contributes to the changes in permittivity between adjacent layers. The possible upper limit of δDa was estimated as follows. If a randomly oriented ice fabric changes suddenly at a boundary to a vertical single-maximum pattern, δDa becomes . 1/3 Since a similar contrast in ice fabrics is seen in the Byrd Station ice core at a depth of 2006m, reported by Reference Gow and WilliamsonGow and Williarnson (1976), this value seems to be the realistic upper limit of δDa that exists in ice sheets. Therefore, Rs was estimated for values of δDa between 0.1 and. 1/3 The calculated results are shown in Figure 1. In the figure, Rs. is expressed in dB.It is between -60 and -70 (dB) and independent of both frequency and temperature, since Δε'. is independent of them.

fig. 1. The possihle maximum reflection coefficients ( Rs) due to changes in Clystal-orientation fabrics (dashed bold lines) and those due to changes in conductivily (solid bold lines) in ice against frequency. The frequencies that were often used in radar sounding in Antarctica by Reference MillarMillar ( 1981a, Reference Millarb) and by the Japanese Antarctic Research Expedition are denoted by thin vertical dashed lines. Parameters used in estimations are described in the text.
Reflection due to changes in conductivity
Conductivity arising from the presence of acid impurity
Reference Moore and FujitaMoore and Fujita (1993) rewrote the increase in tan 15 arising from the presence of acid impurity in acid-doped ice measured by Reference Fujita, Shiraishi and MaeFujita and others (1992) at 9.7 GHz as molar conductivity that means an increase in conductivity due to acid equivalent to 1 mole per litre, using the relation between conductivity, σ∞, and tan δ, σ∞ = ε0ε'2πf tan δ,. Here, ε0 is the permittivity in a vacuum. Since the molar conductivity was well fitted by the Arrhenius equation by Moore and Reference Fujita and MaeFujita (1993), σ∞ can be written as

where R is the gas constant, E is an activation energy and TK is absolute temperature. C is the concentration of acid or hydrogen ions in ice in molarity. Based on the molar conductivity in the experimental results at 9.7GHz, we obtain σ0 = exp (10.3)(Sm-1mol1 ) and E = 18.8 (kJ mol1) at temperatures between -5° and -30°C. The increase in tan δ arising from the acid impurity in ice, Δ(tan δ).
Substituting Equation (8) into σ∞ in Equation (9)becomes

Rs is calculated as a function of change in acid concentration by substituting Equation (10) into Equation Then Equation (3) is

Here, R, could be expressed as a function of acid concentration, frequency and temperature.
Changes in acid concentration
In order to estimate possible Rs from Equation (11), the parameter C should be estimated. As a possible upper limit of C, we consider 7 (μM) in Antarctica and 12 (μM) in Greenland. The reason is as follows. In Antarctica, for example in the Byrd Station ice core, the levels of acidity peaks were 10 ~ 15 (μeq. H + kg-1) (Reference Hammer, Clausen and LangwayHammer and others, 1985). The level of the acidity peak in the Vostok ice core and the South Pole ice core is of a similar magnitude (figures 3 and 4 in Reference Legrand, Petit and KorotkevichLegrand and others (1987)). In contrast, in Greenland, most of the acidity peaks in the Crete ice core, Dye 3 ice core and Camp Century ice core were below 15 (μeq. H + kg 1) but in some extreme cases the acidity reached as high as about 25 (μeq. H+ kg-l) (figure 2 in Reference HammerHammer (1980)). When the acid concentration is expressed in molarity, that is the unit C in Equation (11), the upper limit roughly becomes 7 (μM) in Antarctica and 12 (μM) in Greenland, respectively. This conversion is based on the fact that most acid peaks in polar ice cores arc a result of volcanic eruptions and are usually dominated by H2S04, In polar ice, H2S04 is considered to exist at three-grain boundaries (Reference WoW and ParenWollf and Paren, 1984) as the liquid phase. In the liquid phase, only almost one hydrogen ion is dissociated from each molecule of H2So4 and hydrogen ions dominate the conductivity in d.c., LF and microwave frequencies (Reference Moore and FujitaMoore and Fujita, 1993). Therefore, I (eq. H+ kg-1) is 0.46 (M). Considering the upper limit, 7 (μM) in Antarctica and 12 (μM) in Greenland, 7 (μM) was used as C for the calculation of Rs in Equation (11). The estimated Rs is shown in Figure 1 for various temperatures between -600 and-20°C. If one considers 12 (μM) as the upper limit in Greenland, + 4.5 (dB) should be acided to the PRC in Figure 1. In the calculation, the conductivity with C = 7 (μM) was calculated first using Equation (8). It is shown in Figure 2 as a function of temperature. Because the parameters σ0 and E in Equation (8)were derived from experiments at temperatures between 5° and -30°C in Reference Fujita, Shiraishi and MaeFujita and others (1992), the calculated values below -30°C are extrapolated values
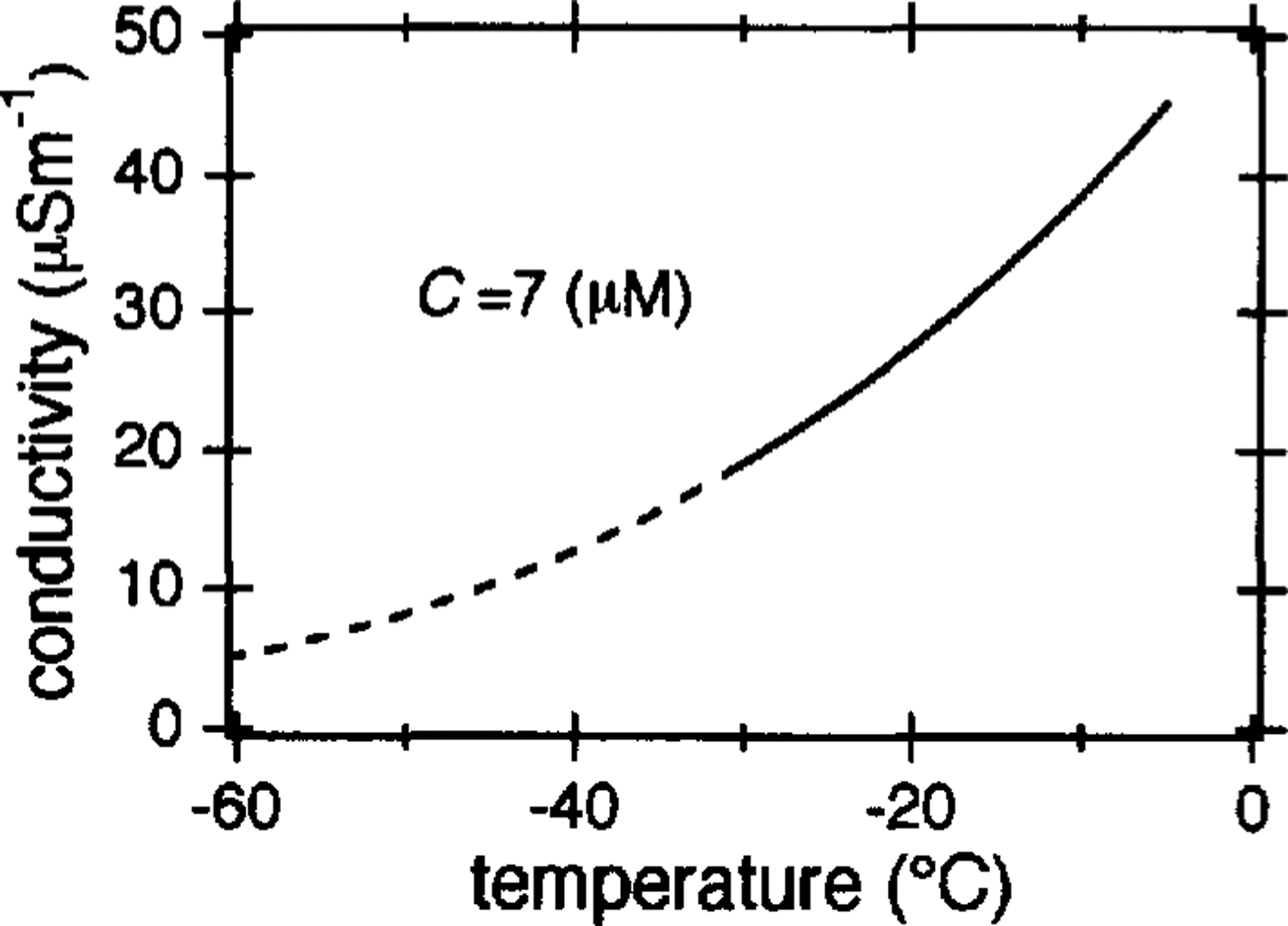
fig. 2. Conductivity 0f ice containing acid of 7μM, calculated using Equation (8). It is assumed that 7μM is the approximate upper limit of acid concentration in acid peaks in the Antarctic ice sheet.
Effect of layer thickness
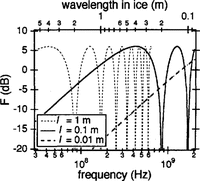
Since F is a periodical function of l and λm as in Equation (1 c), we must consider the effect of F in discussing the PRC in Equation (1a). Figure 3 is the variation of F as a function of them. Here, F was calculated for various l and was expressed in dB. From the figure, clearly F is very small when l is below the order of 1 cm. When l is the order of 10 cm, F increases with increasing frequency. It reaches a maximum value, about 6 (dB), when λm/4 reaches l However, it suddenly decreases at a higher frequency and repeats increasing and decreasing. When l is of the order of 1m, F oscillates with increasing frequency. These analyses indicate that layers thicker than several centimetres are important for the internal reflections. The layer thickness can be investigated if we observe the frequency at which a sudden decrease in the signal occurs. If the layer thickness is greater than 1 m, the signal repeats fading and reappearing with increasing frequency

fig. 3. F (dB) in Equation (it) as a function of frequency calculated for various layer thicknesses, l.
When the PRC is expressed in dB, it is a summation of Rs (dB) and F (dB). An example of the PRC due to two causes is shown in Figure 4. The parameters used in the calculation are typical ones in an inland region of the Antarctic ice sheet. This figure shows that the PRC due to changes in permittivity increase with increasing frequency when λm/4 is smaller than l, and that the PRC due to changes in conductivity is constant.

fig. 4. PRC (dB) as the summation of Rs (dB) and F (dB). Typical parameters found in the Antarctic ice sheet are used for calculation.
Discussion
Frequency-dependence and temperature-dependence
Now we can assess and compare the magnitude of the PRC and Rs due to two mechanisms. Since Δε' is independent of frequency and temperature, It, due to changes in ice fabrics is independent of them. The upper limit of Rs is between about -60 and -70 dB. These values are large enough to cause predominant reflections. In contrast, since changes in loss tangent due to changes in conductivity are inversely proportional to frequency in Equation (9), Rs is also inversely proportional to it. It strongly depends also on temperature. It has a similar magnitude to R due to changes in ice fabrics only when the frequencies are below about 100 MHz as in Figure 1. These results strongly suggest that the predominant cause of the internal reflections is not changes in acidity but changes in ice fabrics, at least when frequencies about 100 MHz are used. When frequencies between about several tens of MHz and about 100 MHz are used, two causes equally dominate internal reflections. If further lower frequencies are used, then the predominant cause is change in acidity. Considering the temperature-dependence, the results also suggest that only when changes in conductivity are the predominant cause, It, is larger in Greenland than in the central part of Antarctica. The difference in the PRC, Rs and F in both polar ice sheets is discussed later.
Inversely, the results mean that the dominant cause of reflection horizons can be investigated by observing frequency-dependence and temperature-dependence of Rs or the PRC. If there are two kinds of reflection horizon in ice sheets, due to permittivity and due to conductivity, the dependence on frequency will be different from each other. If the acid concentration in ice sheets and the mechanical properties of ice (hence ice fabrics) are correlated as has been discussed by Robin and Reference MillarMillar (1982), the contribution of both causes in single layers can be analysed by investigating the frequency-dependence of Rs or the PRC. Such analyses will provide information of the relation between the changes in acidity and ice fabrics in single layers. Therefore, analysis of multi-frequency RES data will provide further information about the internal reflection layers and the internal structure of the ice sheets.
Examination of previously published RES data
The difference in PRC in both polar ice sheets
In the light of new knowledge on the causes and the nature of the internal reflections, we review RES data that have been published so far. To discuss the predominant cause of internal reflections, it is important to compare Rs and the PRC that were observed under different temperature conditions. A good example is the difference in the PRC in the Antarctic ice sheet and in the Greenland ice sheet found by Reference MillarMillar (1982). He found that the PRC at Crete in Greenland was typically 10- 20 dB greater than the PRC at several sites in East Antarctica. The frequency he used was 60 MHz.
To estimate the difference in Rs in both polar ice sheets from the difference in PRC, we should first consider F in both polar ice sheets. Since F is a function of l, we should consider the difference in l between both ice sheets. If we consider the difference in the annual layer thickness between both ice sheets, assuming that the thicknesses of the reflection layers are of the order of the annual accumulation rate, F in Greenland is 7.6 ~ 20.4 (dB) larger than those in Antarctica. Table 1 shows the factor F when the annual accumulation rate is taken as l. The annual accumulation rate at each site is cited from Reference Oeschger and LangwayOeschger and Langway (1989).
The assumption that we used is based on the observation by Reference MillarMillar (1981 a, b) that the depths of the internal reflection layers are highly correlated with depths corresponding to the dates of past large volcanic eruptions. Since Reference HammerHammer (1980) found that volcanic eruptions can produce an increased level in the acidity of precipitation in polar regions 1 or more years after the eruption, thicknesses of the layers containing acid impurity are of the order of the annual accumulation rate. The calculated difference in F agreed well with the difference in the PRC quantitatively. This coincidence means that Rs is almost the same for both ice sheets. This result is very important, because Rs is almost the same in spite of the temperature conditions and the concentration
The calculated difference in F agreed well with the difference ill the PRC quantitatively. This coincidence means that Rs is almost the same for both ice sheets. This result is very important, because Rs is almost the same in spite of the temperature conditions and the concentration of acid in the acidity peak being different for both polar ice sheets. Therefore, this result suggests that conductivity was not the predominant cause of internal reflection even when 60 MHz was used. If the predominant cause of internal reflections is changes in conductivity, differences in the acidity peak, 12 μM and 7 μM, between Greenland and Antarctica, this should cause a difference in the R, by about 4.5 dB. The difference between the temperature in Greenland and in the central part of Antarctica by 20 30°C should also cause a difference in Rs of about 10dB. Their summation, 14.5 dB, should be observed as the difference in Rs when changes in conductivity are a predominant cause. The lack of such a large difference in Rs means that internal reflections observed by Reference MillarMillar (1982) were not dominated by changes in conductivity in spite of the fact that it was observed at 60 MHz.
Table 1. F (dB) calculated from the annual accumulation rate of both ice sheets

Correspondence between the layer depths in multi-frequency
RES As for the multi-frequency RES, z-scope records from the South Pole and Dome C in Antarctica at 60 and 300 MHz have been given by Reference MillarMillar (1981b) and Reference WalfordWalford (1986). The records were ones used to investigate the correlation between the depths of the reflection layers and the dates of past volcanic eruptions (Reference MillarMillar, 1981a). Although the internal-layer records at 300 MHz are affected by the spatial fading pattern, several layer echoes are seen to be common between the records for Dome e observed at 60 MHz and at 300 MHz at depths between 1 and 2 km. Furthermore, considering the Rs at 300 MHz in Figure I, the cause of the layer echoes in the record at 300 MHz should be attributed to changes in the icc fabrics. It is noteworthy that Reference MillarMillar (1981a) found a correlation between the depths of the reflection layers and the dates of past volcanic eruptions using the same records at 60 MHz. This fact and the interpretation of the 300 MHz data suggest that the observed internal layers in the 300 MHz records also have high correlation with the dates of past volcanic eruptions. This further suggests that changes in ice fabrics exist at these depths. Since Reference MillarMillar (1981a, Reference Millarb) indicated that the PRC/age profiles have common features for layers observed at the South Pole, Vostok, Domee and two other sites in East Antarctica in 60 MHz records, the reflection layers due presumably to changes in ice fabrics, in the 300 MHz record for Domee, may also exist at the other sites in East Antarctica.
The only scenario that consistently explains the above estimation, observations and discussions are as follows. Layers that contain matter originating from past volcanic eruptions have a different fabric pattern from the enclosing ice layers. It does not depend on whether the matter is visible or not because most acidity layers are invisible. This idea is supported by the fact that the acid peaks in the Byrd Station ice core were not found in association with the visible ash layers reported by Gow and Williamson (Reference Hammer, Clausen and LangwayHammer and others, 1985). The cause of changes in ice fabrics can be explained partly by the discussion by Reference Robin and MillarRobin and Millar (1982) that the presence of additional acid impurities in the ice may change the mechanical properties of the ice and that consequently the radio-echo layers may indicate the easy glide plane and hence the ice fabrics. The changes in ice fabrics predominantly caused internal reflections both at 60 MHz and at 300 MHz, although changes in acidity might be one of the dominant causes at 60 MHz. However, since the echoes are very clear at the crest of the ice sheet and around the summits of the ice sheet in the central part of East Antarctica (Reference Robin, Drewry and Meldrume.g. Robin and others, 1977; Reference MillarMillar, 1981b), around such places the formation process of the contrast of fabric patterns cannot be explained only by the shear motion of the ice sheet. Another mechanism that is related to grain growth may exist, such as, crystal size is highly correlated with acidity (Reference Langway, Shoji and Azumae.g. Langway and others, 1988).
Conclusion
The present study showed that possible maximum Rs and PRC are due to changes in crystal-orientation fabrics and are due to changes in acid concentration as a function of frequency and temperature. The estimations were based on the dielectric properties of ice measured in the laboratory. The former mechanism can produce predominant radio-echo reflections at deep layers in polar ice sheets. In contrast, the latter mechanism can be dominant at frequencies below about 60 MHz. The different frequency-dependence of different mechanisms means that the predominant cause of internal reflections can be finally determined by observing the frequency-dependence of the reflection coefficients. An examination of the RES data that have been published so far suggested that changes in ice fabrics exist at depths corresponding to the dates of past volcanic eruptions. If our discussions are entirely correct, the next interest is the quantitative relation between acidity, mechanical properties of ice, strain in each layer and the grain-growth process. This can be investigated quantitatively by careful investigations of the multi-frequency RES data and/or directly by ice-core analysis. These studies arc necessary for a correct understanding of the internal horizons and of the internal structure of polar ice sheets.
Acknowledgements
The authors thank an anonymous referee for his critical review from which the discussion has been improved significantly.