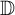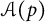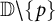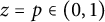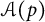Improved Bloch and Landau constants for meromorphic functions

Abstract

Let ${\mathbb D}$ be the open unit disk, and let $\mathcal {A}(p)$ be the class of functions f that are holomorphic in ${\mathbb D}\backslash \{p\}$ with a simple pole at $z=p\in (0,1)$, and $f'(0)\neq 0$. In this article, we significantly improve lower bounds of the Bloch and the Landau constants for functions in ${\mathcal A}(p)$ which were obtained in Bhowmik and Sen (2023, Monatshefte für Mathematik, 201, 359–373) and conjecture on the exact values of such constants.

1 Introduction

Let ${\mathbb D}$ be the unit disk, let $\partial {\mathbb D}$ be the unit circle, and let ${\mathcal F}$ be the set of all holomorphic functions from ${\mathbb D}$ to the complex plane ${\mathbb C}$ with $f'(0)=1$ . Given a function $f\in {\mathcal F}$ , let $B_f$ be the radius of the largest univalent disk in $f({\mathbb D})$ , and let $L_f$ be the radius of the largest disk in $f({\mathbb D})$ . Here, by a univalent disk $\Delta $ in $f({\mathbb D})$ , we mean that there exists a domain $\Omega $ in ${\mathbb D}$ such that f maps $\Omega $ univalently onto $\Delta $ . In 1924, Andre Bloch—a French mathematician, proved a classical result which asserts that for $f\in {\mathcal F}$ , $B_f>0$ (see [3]). The infimum of $B_f$ , $f\in {\mathcal F}$ is called the Bloch constant which we denote by B; i.e.,

$$ \begin{align*} B:=\inf~\{B_f: f\in{\mathcal F}\}. \end{align*} $$

This result is called as the Bloch’s theorem. In 1929, Landau (see [7]) first introduced the concept of Bloch constant. At the same time, he also introduced another constant, namely, the Landau constant for functions in the class ${\mathcal F}$ which is denoted by L and defined as follows:

$$ \begin{align*}L:=\inf~\{L_f: f\in{\mathcal F}\}. \end{align*} $$

At present, the best known upper and lower bounds for B are

$$ \begin{align*}\frac{\sqrt{3}}{4}+2\times 10^{-4}< B \leq \frac{1}{\sqrt{1+\sqrt{3}}}\frac{\Gamma(1/3)\Gamma(11/12)}{\Gamma(1/4)}\approx 0.4719. \end{align*} $$

The lower bound for the Bloch constant B was obtained by Chen and Gauthier (see [5]). The upper bound for the Bloch constant B was obtained by Ahlfors and Grunsky (see [1]); also, they conjectured that this upper bound is the precise value of the Bloch constant. We now present here a brief overview of the Landau constant. In 1943, Rademacher (compare [10]) and Yanagihara (in 1995, see [12]) proved that the upper and the lower bounds for the Landau constant are

$$ \begin{align*}\frac{1}{2}+10^{-335}<L\leq \frac{\Gamma(1/3)\Gamma(5/6)}{\Gamma(1/6)}\approx 0.5433. \end{align*} $$

Rademacher (compare [10]) also conjectured that this upper bound is the precise value of the Landau constant. It is worth to mention here that, after the proof of the famous Bieberbach conjecture by Louis de Branges in 1985, one of the most outstanding open problems in geometric function theory is to find the precise value of the Bloch and the Landau constants for functions in the class ${\mathcal F}$ . In the sequel, we also briefly discuss about the locally univalent and the univalent Bloch constants for holomorphic functions which are defined by

$$ \begin{align*} B_l&:=\inf~\{B_f:f\in{\mathcal F},\;\; f'(z)\ne 0,\; z\in{\mathbb D}\}\;\;\mbox{and}\\ B_u&:=\inf~\{B_f:f\in{\mathcal F},\;\;f\;\mbox{is univalent in}\;{\mathbb D}\}, \end{align*} $$

respectively. The relation between Bloch constant, Landau constant, locally univalent Bloch constant, and univalent Bloch constant is

$$ \begin{align*}B\leq B_l\leq L\leq B_u. \end{align*} $$

In 1995, Yanagihara (see [12]) proved that $B_l>1/2+ 10^{-335}$ . In 2009, Skinner (see [11]) proved that $B_u>0.5708858$ . These bounds are latest bounds and best known so far.

Many eminent Mathematicians studied the Bloch constant for meromorphic functions considering the spherical metric, which was a natural choice for them, as meromorphic functions take values in ${\widehat {\mathbb C}}\,$ —the extended complex plane. We present here a short description of the results known so far in this direction. In [9], Minda proved that the precise value of the Bloch constant for the family of locally univalent meromorphic functions on ${\mathbb C}$ is $\pi /2$ . In the same article, he showed that the Bloch constant for the family of all nonconstant meromorphic functions on ${\mathbb C}$ lies between $\pi /3$ and $2\arctan (1/\sqrt {2})$ , which is improved by Bonk and Eremenko in the year $2000$ (see [4]) and they obtained the precise value of this constant as $\arctan \sqrt {8}$ .

In the article [2], we considered the analogous problem of estimating the Landau and the Bloch constants for a class of meromorphic functions in the Euclidean metric. Precisely, let $\mathcal {A}(p)$ consisting of all functions f that are holomorphic in ${\mathbb D}\backslash \{p\}$ with a simple pole at $z=p\in (0,1)$ and $f'(0)\neq 0$ . For $f\in \mathcal {A}(p)$ , let $B_f(p)$ be the radius of the largest univalent disk that lies in $f({\mathbb D})$ and let $L_f(p)$ be the radius of the largest disk that lies in $f({\mathbb D})$ . The Bloch and the Landau constants for $f\in \mathcal {A}(p)$ are defined by

$$ \begin{align*}B(p):=\inf\,\{B_f(p) : f\in\mathcal{A}(p)\} \;\mbox{and}\; L(p):=\inf\,\{{ L}_f(p) : f\in\mathcal{A}(p)\}, \end{align*} $$

respectively. In [2], we proved that

$$ \begin{align*}B(p)\geq (8-\sqrt{63})^2p^2|f'(0)|\;\;\mbox{and} \;\; L(p)\geq \frac{(9-4\sqrt{5})p^2|f'(0)|}{8}. \end{align*} $$

In this article, we significantly improve the lower bounds of $B(p)$ and $L(p)$ and conjecture on the exact values of these constants.

2 Main result

Theorem 2.1 If B and L be the Bloch and the Landau constants for the class ${\mathcal F}$ , then

$$ \begin{align*}B(p)\geq\frac{4p|f'(0)|B}{(1+p)^2}\;\;\mbox{and}\;\;L(p)\geq\frac{4p|f'(0)|L}{(1+p)^2}. \end{align*} $$

Proof Let $\Omega _p$ be the domain obtained from the unit disk ${\mathbb D}$ by deleting the line segment $[p,\;1)$ , i.e.,

$$ \begin{align*}\Omega_p:={\mathbb D}\backslash [p,1),\;\;\mbox{where}\;\; 0<p<1. \end{align*} $$

Clearly, $\Omega _p$ is a simply connected domain. This domain $\Omega _p$ can be mapped conformally onto ${\mathbb D}$ by a function $\kappa $ with the following Taylor expansion in the disk $\{z\in {\mathbb C}~:~|z|<p\}$ about the origin

$$ \begin{align*}\kappa(z)=\frac{(1+p)^2}{4p}z + \sum_{n=2}^{\infty} c_n z^n, \end{align*} $$

where $c_n>0$ for all $n\geq 2$ (see, for instance, [6, 8]). Now, for $f\in {\mathcal A}(p)$ , let $f_1\equiv f|_{\Omega _p}$ . Now, for each such $f_1$ , there exists a function $g\in {\mathcal F}$ with g having a simple pole at $e^{i\theta }\kappa (p)\in \partial {\mathbb D}$ , $\theta =\arg (f'(0))$ such that

$$ \begin{align*}f_1(z)=\frac{4p|f'(0)|}{(1+p)^2}(g\circ(e^{i\theta} \kappa))(z),\;z\in\Omega_p. \end{align*} $$

We note here that $f_1$ is a holomorphic function in $\Omega _p$ with $f_1'(0)=f'(0)$ and

$$ \begin{align*}f_1(\Omega_p)=\frac{4p|f'(0)|}{(1+p)^2}g({\mathbb D}). \end{align*} $$

Since $g\in {\mathcal F}$ , then $g({\mathbb D})$ contains a univalent disk of radius at least B and a disk of radius at least L. This implies that $f_1(\Omega _p)$ contains a univalent disk of radius at least $4p|f'(0)|B/(1+p)^2$ and a disk of radius at least $4p|f'(0)|L/(1+p)^2$ . As $f_1(\Omega _p)\subset f({\mathbb D})$ , therefore $f({\mathbb D})$ contains a univalent disk of radius at least $4p|f'(0)|B/(1+p)^2$ and a disk of radius at least $4p|f'(0)|L/(1+p)^2$ . This completes the proof.

Remarks (i) Since the best known lower bound of the Bloch constant B for ${\mathcal F}$ is $\sqrt {3}/4+2\times 10^{-4}$ (compare [5]), then form Theorem 2.1, we have

$$ \begin{align*}B(p)>\frac{(\sqrt{3}+8\times 10^{-4})|f'(0)|p}{(1+p)^2}. \end{align*} $$

This lower bound improves the lower bound proved in [2, Theorem 2]. Also, since the best known lower bound of the Landau constant for holomorphic function is $1/2+10^{-335}$ (compare [12]), then from the Theorem 2.1, we get

$$ \begin{align*}L(p)>\frac{(2+4\times 10^{-335})|f'(0)|p}{(1+p)^2}. \end{align*} $$

This lower bound of the Landau constant $L(p)$ for the class of functions ${\mathcal A}(p)$ improves the bound given in [2, Theorem 1].

(ii) In [2], we have considered a subclass ${\mathcal A}_1(p)$ of ${\mathcal A}(p)$ and improved the lower bounds of the Bloch and the Landau constants for function in ${\mathcal A}_1(p)$ as $p^2|f'(0)|/27$ and $(9-4\sqrt {5})p^2|f'(0)|/(1+\sqrt {2})$ , respectively (see [2, Theorems 3 and 4]). We note here that the lower bounds presented in the Remark (i) also improve the lower bounds proved in [2, Theorems 3 and 4] for this particular subclass of ${\mathcal A}(p)$ .

(iii) Let

$$ \begin{align*} &B_l(p):=\inf~\{B_f(p)\,:\,f\in{\mathcal A}(p),\;f'(z)\ne0,\;z\in{\mathbb D}\backslash\{p\}\}\;\;\mbox{and}\\ &B_u(p):=\inf~\{B_f(p)\,:\,f\in{\mathcal A}(p),\;f\;\mbox{is univalent in}\;{\mathbb D}\backslash\{p\}\}. \end{align*} $$

Then from Theorem 2.1, we get

$$ \begin{align*}B_l(p)>\frac{(2+4\times 10^{-335})|f'(0)|p}{(1+p)^2}\;\;\mbox{and}\;\;B_u(p)>\frac{2.2835432|f'(0)|p}{(1+p)^2}; \end{align*} $$

since the lower bounds of the locally univalent and univalent Bloch constants for holomorphic functions are $1/2+ 10^{-335}$ and $0.5708858$ , respectively.

(iv) We comment here that Theorem 2.1 can be generalized for functions holomorphic in ${\mathbb D}\backslash [p,1)$ , $p\in (0,1)$ having singular points lying in the line segment $[p,1)$ . The method of proof will remain the same which we adopted in the Theorem 2.1.

From Theorem 2.1, we only get information about the lower bounds of the Bloch and the Landau constants for the class ${\mathcal A}(p)$ , but we have no information about the upper bounds and the exact values of such constants. It will be an interesting problem to find upper bounds and the precise values of such constants. In Theorem 2.1, if we allow $p\rightarrow 1-$ , then

$$ \begin{align*}B(1)=\lim_{p\rightarrow 1-} B(p)\geq B|f'(0)|,\;\;\mbox{and}\;\;L(1)=\lim_{p\rightarrow 1-} L(p)\geq L|f'(0)|. \end{align*} $$

If $f'(0)=1$ , and $B(1)$ and $L(1)$ denote the Bloch and the Landau constants for functions in the class ${\mathcal F}$ , with a simple pole at $z=1$ , then we know that one can easily show $B(1)=B$ and $L(1)=L$ . Thus, equalities hold in the above inequalities. This observation motivates us to conjecture on the precise values of the Bloch and the Landau constants for functions in the class ${\mathcal A}(p)$ as follows:

Conjecture 1 If B and L are the exact values of the Bloch and the Landau constants, respectively, for functions in the class ${\mathcal F}$ , then

$$ \begin{align*}B(p)=\frac{4p|f'(0)|B}{(1+p)^2}~ \mbox {and}\,~ L(p)=\frac{4p|f'(0)|L}{(1+p)^2}. \end{align*} $$