 $2$ Simple Groups of Lie Type
$2$ Simple Groups of Lie Type
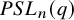 $PSL_n(q)$
and
$PSL_n(q)$
and
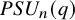 $PSU_n(q)$
by their
$PSU_n(q)$
by their
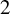 $2$
-fusion systems, q odd
$2$
-fusion systems, q odd