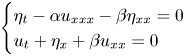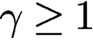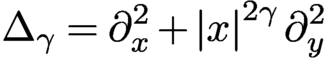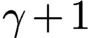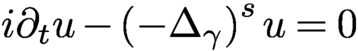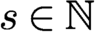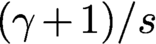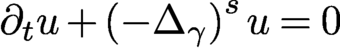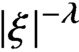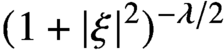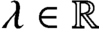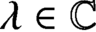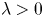9 results
Spectral theory of the invariant Laplacian on the disk and the sphere – a complex analysis approach
- Part of
-
- Journal:
- Canadian Journal of Mathematics , First View
- Published online by Cambridge University Press:
- 01 March 2024, pp. 1-35
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
On the linearized Whitham–Broer–Kaup system on bounded domains
- Part of
-
- Journal:
- Proceedings of the Royal Society of Edinburgh. Section A: Mathematics , First View
- Published online by Cambridge University Press:
- 07 September 2023, pp. 1-20
-
- Article
- Export citation
The Lp convergence of Fourier series on triangular domains
- Part of
-
- Journal:
- Proceedings of the Edinburgh Mathematical Society / Volume 66 / Issue 2 / May 2023
- Published online by Cambridge University Press:
- 19 June 2023, pp. 453-474
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
OBSERVABILITY OF BAOUENDI–GRUSHIN-TYPE EQUATIONS THROUGH RESOLVENT ESTIMATES
- Part of
-
- Journal:
- Journal of the Institute of Mathematics of Jussieu / Volume 22 / Issue 2 / March 2023
- Published online by Cambridge University Press:
- 14 June 2021, pp. 541-579
- Print publication:
- March 2023
-
- Article
- Export citation
Strichartz estimates for orthonormal families of initial data and weighted oscillatory integral estimates
- Part of
-
- Journal:
- Forum of Mathematics, Sigma / Volume 9 / 2021
- Published online by Cambridge University Press:
- 11 January 2021, e1
-
- Article
-
- You have access
- Open access
- Export citation
Stability of periodic waves for the fractional KdV and NLS equations
- Part of
-
- Journal:
- Proceedings of the Royal Society of Edinburgh. Section A: Mathematics / Volume 151 / Issue 4 / August 2021
- Published online by Cambridge University Press:
- 12 August 2020, pp. 1171-1203
- Print publication:
- August 2021
-
- Article
- Export citation
EXPONENTIAL STABILISATION OF A TREE-SHAPED NETWORK OF STRINGS WITH VARIABLE COEFFICIENTS*
-
- Journal:
- Glasgow Mathematical Journal / Volume 53 / Issue 3 / September 2011
- Published online by Cambridge University Press:
- 10 March 2011, pp. 481-499
- Print publication:
- September 2011
-
- Article
-
- You have access
- Export citation
Solvability conditions for some non-Fredholm operators
- Part of
-
- Journal:
- Proceedings of the Edinburgh Mathematical Society / Volume 54 / Issue 1 / February 2011
- Published online by Cambridge University Press:
- 30 November 2010, pp. 249-271
-
- Article
-
- You have access
- Export citation
Symmetric Green's Function for a Class of CIV Boundary Value Problems
-
- Journal:
- Canadian Mathematical Bulletin / Volume 31 / Issue 3 / 01 September 1988
- Published online by Cambridge University Press:
- 20 November 2018, pp. 272-279
- Print publication:
- 01 September 1988
-
- Article
-
- You have access
- Export citation

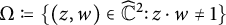 of the “complexified unit circle”
of the “complexified unit circle”