Modal structuralism promises an interpretation of set theory that avoids commitment to abstracta. This article investigates its underlying assumptions. In the first part, I start by highlighting some shortcomings of the standard axiomatisation of modal structuralism, and propose a new axiomatisation I call MSST (for Modal Structural Set Theory). The main theorem is that MSST interprets exactly Zermelo set theory plus the claim that every set is in some inaccessible rank of the cumulative hierarchy. In the second part of the article, I look at the prospects for supplementing MSST with a modal structural reflection principle, as suggested in Hellman (2015). I show that Hellman’s principle is inconsistent (Theorem 5.32), and argue that modal structural reflection principles in general are either incompatible with modal structuralism or extremely weak.

Let T be a second-order arithmetical theory, Λ a well-order, λ < Λ and X ⊆ ℕ. We use 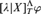$[\lambda |X]_T^{\rm{\Lambda }}\varphi$ as a formalization of “φ is provable from T and an oracle for the set X, using ω-rules of nesting depth at most λ”.

For a set of formulas Γ, define predicative oracle reflection for T over Γ (Pred–O–RFNΓ(T)) to be the schema that asserts that, if X ⊆ ℕ, Λ is a well-order and φ ∈ Γ, then

$$\forall \,\lambda < {\rm{\Lambda }}\,([\lambda |X]_T^{\rm{\Lambda }}\varphi \to \varphi ).$$

In particular, define predicative oracle consistency (Pred–O–Cons(T)) as Pred–O–RFN{0=1}(T).

Our main result is as follows. Let ATR0 be the second-order theory of Arithmetical Transfinite Recursion, 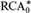${\rm{RCA}}_0^{\rm{*}}$ be Weakened Recursive Comprehension and ACA be Arithmetical Comprehension with Full Induction. Then,

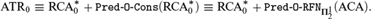$${\rm{ATR}}_0 \equiv {\rm{RCA}}_0^{\rm{*}} + {\rm{Pred - O - Cons\ }}\left( {{\rm{RCA}}_0^{\rm{*}} } \right) \equiv {\rm{RCA}}_0^{\rm{*}} + \,{\rm{Pred - O - Cons\ }}\left( {{\rm{RCA}}_0^{\rm{*}} } \right) \equiv {\rm{RCA}}_0^{\rm{*}} + \,{\rm{Pred - O - RFN}}\,_{{\bf{\Pi }}_2^1 } \left( {{\rm{ACA}}} \right).$$

We may even replace 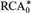${\rm{RCA}}_0^{\rm{*}}$ by the weaker ECA0, the second-order analogue of Elementary Arithmetic.

Thus we characterize ATR0, a theory often considered to embody Predicative Reductionism, in terms of strong reflection and consistency principles.