1 results
Hyperplanes of the Form f1(x, y)z1 + · · · + fk(x, y)zk + g(x, y) Are Variables
-
- Journal:
- Canadian Mathematical Bulletin / Volume 48 / Issue 4 / 01 December 2005
- Published online by Cambridge University Press:
- 20 November 2018, pp. 622-635
- Print publication:
- 01 December 2005
-
- Article
-
- You have access
- Export citation
-
The Abhyankar–Sathaye Embedded Hyperplane Problem asks whether any hypersurface of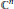
${{\mathbb{C}}^{n}}$ isomorphic to 
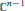${{\mathbb{C}}^{n-1}}$ is rectifiable, i.e., equivalent to a linear hyperplane up to an automorphism of 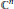${{\mathbb{C}}^{n}}$. Generalizing the approach adopted by Kaliman, Vénéreau, and Zaidenberg, which consists in using almost nothing but the acyclicity of 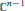${{\mathbb{C}}^{n-1}}$, we solve this problem for hypersurfaces given by polynomials of 
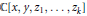$\mathbb{C}\left[ x,y,{{z}_{1}},...,{{z}_{k}} \right]$ as in the title.
