1 results
Limits of geodesic push-forwards of horocycle invariant measures
- Part of
-
- Journal:
- Ergodic Theory and Dynamical Systems / Volume 41 / Issue 9 / September 2021
- Published online by Cambridge University Press:
- 23 October 2020, pp. 2782-2804
- Print publication:
- September 2021
-
- Article
- Export citation
-
We prove several general conditional convergence results on ergodic averages for horocycle and geodesic subgroups of any continuous
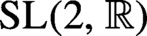$\operatorname {SL}(2, \mathbb {R})$
-action on a locally compact space. These results are motivated by theorems of Eskin, Mirzakhani and Mohammadi on the
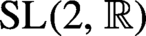$\operatorname {SL}(2, \mathbb {R})$
-action on the moduli space of Abelian differentials. By our argument we can derive from these theorems an improved version of the ‘weak convergence’ of push-forwards of horocycle measures under the geodesic flow and a short proof of weaker versions of theorems of Chaika and Eskin on Birkhoff genericity and Oseledets regularity in almost all directions for the Teichmüller geodesic flow.
