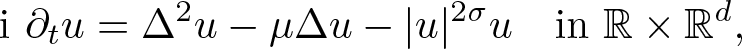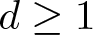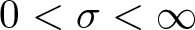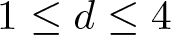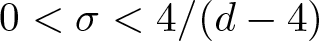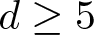6 results
Blowup of cylindrically symmetric solutions for biharmonic NLS
- Part of
-
- Journal:
- Proceedings of the Edinburgh Mathematical Society / Volume 67 / Issue 4 / November 2024
- Published online by Cambridge University Press:
- 03 October 2024, pp. 1085-1098
-
- Article
-
- You have access
- HTML
- Export citation
Blowup of Volterra Integro-Differential Equations and Applications to Semi-Linear Volterra Diffusion Equations
- Part of
-
- Journal:
- Numerical Mathematics: Theory, Methods and Applications / Volume 10 / Issue 4 / November 2017
- Published online by Cambridge University Press:
- 12 September 2017, pp. 737-759
- Print publication:
- November 2017
-
- Article
- Export citation
Some sharp results about the global existence and blowup of solutions to a class of pseudo-parabolic equations
- Part of
-
- Journal:
- Proceedings of the Royal Society of Edinburgh. Section A: Mathematics / Volume 147 / Issue 6 / December 2017
- Published online by Cambridge University Press:
- 14 August 2017, pp. 1311-1331
- Print publication:
- December 2017
-
- Article
- Export citation
(In-)stability of singular equivariant solutions to the Landau–Lifshitz–Gilbert equation
-
- Journal:
- European Journal of Applied Mathematics / Volume 24 / Issue 6 / December 2013
- Published online by Cambridge University Press:
- 09 August 2013, pp. 921-948
-
- Article
- Export citation
A Numerical Study of Blowup in the Harmonic Map Heat Flow Using the MMPDE Moving Mesh Method
-
- Journal:
- Numerical Mathematics: Theory, Methods and Applications / Volume 6 / Issue 2 / May 2013
- Published online by Cambridge University Press:
- 28 May 2015, pp. 364-383
- Print publication:
- May 2013
-
- Article
- Export citation
Gromov–Witten Invariants of Blow-ups Along Surfaces
-
- Journal:
- Compositio Mathematica / Volume 125 / Issue 3 / February 2001
- Published online by Cambridge University Press:
- 04 December 2007, pp. 345-352
- Print publication:
- February 2001
-
- Article
-
- You have access
- Export citation