1 results
Distributed lag regression by an almost periodic design matrix
-
- Journal:
- Journal of Applied Probability / Volume 15 / Issue 4 / December 1978
- Published online by Cambridge University Press:
- 14 July 2016, pp. 759-773
- Print publication:
- December 1978
-
- Article
- Export citation
-
Frequency domain estimation and tests of hypotheses are considered for a general multivariate distributed lag regression model. The usual vector of regression functions is replaced by a matrix of almost periodic functions, a case for which the terms appearing in the frequency domain estimators have well-defined limits. Asymptotic distributions and consistency are established for the regression coefficients and error spectra. A test statistic is proposed for the no regression hypothesis which is asymptotically central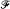
under the null hypothesis and converges to infinity under the alternative. In the multivariate case, a product of beta-distributed variables is obtained.
under the null hypothesis and converges to infinity under the alternative. In the multivariate case, a product of beta-distributed variables is obtained.