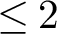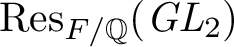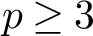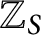5 results
Control theorems for Hilbert modular varieties
- Part of
-
- Journal:
- Canadian Mathematical Bulletin , First View
- Published online by Cambridge University Press:
- 14 January 2025, pp. 1-20
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
ON A PROPERNESS OF THE HILBERT EIGENVARIETY AT INTEGRAL WEIGHTS: THE CASE OF QUADRATIC RESIDUE FIELDS
- Part of
-
- Journal:
- Journal of the Institute of Mathematics of Jussieu / Volume 22 / Issue 6 / November 2023
- Published online by Cambridge University Press:
- 08 April 2022, pp. 2645-2716
- Print publication:
- November 2023
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
Finite descent obstruction for Hilbert modular varieties
- Part of
-
- Journal:
- Canadian Mathematical Bulletin / Volume 64 / Issue 2 / June 2021
- Published online by Cambridge University Press:
- 22 July 2020, pp. 452-473
- Print publication:
- June 2021
-
- Article
- Export citation
Analytic continuation of overconvergent Hilbert eigenforms in the totally split case
- Part of
-
- Journal:
- Compositio Mathematica / Volume 146 / Issue 3 / May 2010
- Published online by Cambridge University Press:
- 02 February 2010, pp. 541-560
- Print publication:
- May 2010
-
- Article
-
- You have access
- Export citation
On Ihara’s lemma for Hilbert modular varieties
- Part of
-
- Journal:
- Compositio Mathematica / Volume 145 / Issue 5 / September 2009
- Published online by Cambridge University Press:
- 09 September 2009, pp. 1114-1146
- Print publication:
- September 2009
-
- Article
-
- You have access
- Export citation