A group is $\frac 32$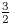 -generated if every non-trivial element is part of a generating pair. In 2019, Donoven and Harper showed that many Thompson groups are $\frac 32$
-generated if every non-trivial element is part of a generating pair. In 2019, Donoven and Harper showed that many Thompson groups are $\frac 32$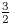 -generated and posed five questions. The first of these is whether there exists a 2-generated group with every proper quotient cyclic that is not $\frac 32$
-generated and posed five questions. The first of these is whether there exists a 2-generated group with every proper quotient cyclic that is not $\frac 32$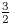 -generated. This is a natural question given the significant work in proving that no finite group has this property, but we show that there is such an infinite group. The groups we consider are a family of finite index subgroups $G_1,\, G_2,\, \ldots$
-generated. This is a natural question given the significant work in proving that no finite group has this property, but we show that there is such an infinite group. The groups we consider are a family of finite index subgroups $G_1,\, G_2,\, \ldots$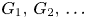 of the Houghton group $\operatorname {FSym}(\mathbb {Z})\rtimes \mathbb {Z}$
of the Houghton group $\operatorname {FSym}(\mathbb {Z})\rtimes \mathbb {Z}$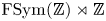 . We then show that $G_1$
. We then show that $G_1$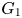 and $G_2$
and $G_2$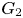 are $\frac 32$
are $\frac 32$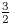 -generated and investigate the related notion of spread for these groups. We are able to show that they have finite spread at least 2. These are, therefore, the first infinite groups to be shown to have finite positive spread, and the first to be shown to have spread at least 2 (other than $\mathbb {Z}$
-generated and investigate the related notion of spread for these groups. We are able to show that they have finite spread at least 2. These are, therefore, the first infinite groups to be shown to have finite positive spread, and the first to be shown to have spread at least 2 (other than $\mathbb {Z}$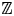 and the Tarski monsters, which have infinite spread). As a consequence, for each $k\in \{2,\, 3,\, \ldots \}$
and the Tarski monsters, which have infinite spread). As a consequence, for each $k\in \{2,\, 3,\, \ldots \}$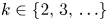 , we also have that $G_{2k}$
, we also have that $G_{2k}$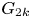 is index $k$
is index $k$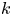 in $G_2$
in $G_2$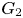 but $G_2$
but $G_2$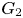 is $\frac 32$
is $\frac 32$ -generated whereas $G_{2k}$
-generated whereas $G_{2k}$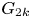 is not.
is not.