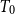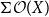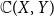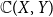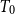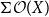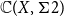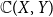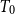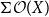In this paper, we mainly study the function spaces related to H-sober spaces. For an irreducible subset system H and $T_{0}$ spaces X and Y, it is proved that the following three conditions are equivalent: (1) the Scott space $\Sigma \mathcal O(X)$ of the lattice of all open sets of X is H-sober; (2) for every H-sober space Y, the function space 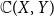$\mathbb{C}(X, Y)$ of all continuous mappings from X to Y equipped with the Isbell topology is H-sober; (3) for every H-sober space Y, the Isbell topology on $\mathbb{C}(X, Y)$ has property S with respect to H. 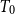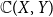One immediate corollary is that for a $T_{0}$ space X, Y is a d-space (resp., well-filtered space) iff the function space $\mathbb{C}(X, Y)$ equipped with the Isbell topology is a d-space (resp., well-filtered space). 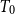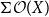It is shown that for any $T_0$ space X for which the Scott space $\Sigma \mathcal O(X)$ is non-sober, the function space 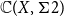$\mathbb{C}(X, \Sigma 2)$ equipped with the Isbell topology is not sober. 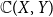The function spaces $\mathbb{C}(X, Y)$ equipped with the Scott topology, the compact-open topology and the pointwise convergence topology are also discussed. Our study also leads to a number of questions, whose answers will deepen our understanding of the function spaces related to H-sober spaces.