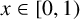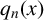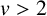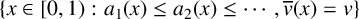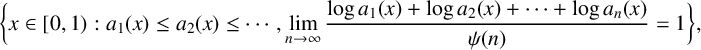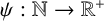THE MULTIFRACTAL SPECTRUM OF CONTINUED FRACTIONS WITH NONDECREASING PARTIAL QUOTIENTS
- Part of
-
- Journal:
- Bulletin of the Australian Mathematical Society , First View
- Published online by Cambridge University Press:
- 25 November 2024, pp. 1-13
-
- Article
- Export citation
-
Let [a_1(x),a_2(x),\ldots ,a_n(x),\ldots ] be the continued fraction expansion of 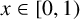x\in [0,1) and 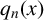q_n(x) be the denominator of its nth convergent. The irrationality exponent and Khintchine exponent of x are respectively defined by
\begin{align*} \overline{v}(x)=2+\limsup_{n\to\infty}\frac{\log a_{n+1}(x)}{\log q_n(x)} \quad \text{and}\quad \gamma(x)=\lim_{n\to\infty}\frac{1}{n}\sum_{i=1}^{n}\log a_i(x). \end{align*}We study the multifractal spectrum of the irrationality exponent and the Khintchine exponent for continued fractions with nondecreasing partial quotients. For any 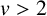v>2, we completely determine the Hausdorff dimensions of the sets 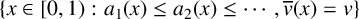\{x\in [0,1): a_1(x)\leq a_2(x)\leq \cdots , \overline {v}(x)=v\} and
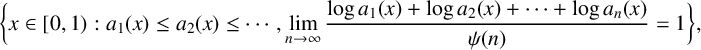\begin{align*}\bigg\{x\in[0,1): a_1(x)\leq a_2(x)\leq\cdots, \lim\limits_{n\to\infty}\frac{\log a_1(x)+\log a_2(x)+\cdots+\log a_n(x)}{\psi(n)}=1\bigg\},\end{align*}where 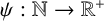\psi :\mathbb {N}\rightarrow \mathbb {R}^+ is a function satisfying \psi (n)\to \infty as n\to \infty .
We use cookies to distinguish you from other users and to provide you with a better experience on our websites. Close this message to accept cookies or find out how to manage your cookie settings.
