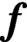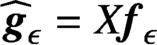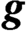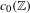Refine search
Actions for selected content:
6 results
12 - Completeness of the Space of Operators
-
- Book:
- Functional Analysis Revisited
- Published online:
- 31 October 2024
- Print publication:
- 04 July 2024, pp 127-143
-
- Chapter
- Export citation
3 - Observables and Operators
-
- Book:
- Quantum Mechanics in Nanoscience and Engineering
- Published online:
- 11 May 2023
- Print publication:
- 01 June 2023, pp 10-15
-
- Chapter
- Export citation
AN OPTIMAL LINEAR FILTER FOR ESTIMATION OF RANDOM FUNCTIONS IN HILBERT SPACE
- Part of
-
- Journal:
- The ANZIAM Journal / Volume 62 / Issue 3 / July 2020
- Published online by Cambridge University Press:
- 07 January 2021, pp. 274-301
-
- Article
- Export citation
Shadowing and structural stability for operators
- Part of
-
- Journal:
- Ergodic Theory and Dynamical Systems / Volume 41 / Issue 4 / April 2021
- Published online by Cambridge University Press:
- 10 January 2020, pp. 961-980
- Print publication:
- April 2021
-
- Article
- Export citation
Normalization of cell responses in cat striate cortex
-
- Journal:
- Visual Neuroscience / Volume 9 / Issue 2 / August 1992
- Published online by Cambridge University Press:
- 02 June 2009, pp. 181-197
-
- Article
- Export citation
Toward a constructive theory of unbounded linear operators
-
- Journal:
- The Journal of Symbolic Logic / Volume 65 / Issue 1 / March 2000
- Published online by Cambridge University Press:
- 12 March 2014, pp. 357-370
- Print publication:
- March 2000
-
- Article
- Export citation