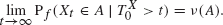Let (Xt) be a one-dimensional Ornstein-Uhlenbeck process with initial density function f : ℝ+ → ℝ+, which is a regularly varying function with exponent -(1 + η), η ∊ (0,1). We prove the existence of a probability measure ν with a Lebesgue density, depending on η, such that for every A ∊ B(R+):