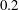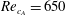In this article, a series of simulations of a rotating disk system with air cooling is introduced. These simulations are performed with the realizable k-ε eddy-viscosity model. Computational results illustrate the effect of the cooling air and disk rotation on the temperature of the disk surface. The maximum rotating disk speed reaches about 78300 rpm and the range of Reynolds numbers based on rotation speed is from about 0.7 × 106 to 3 × 106. The present work shows that the flow structure in the gap, which is on the opposite side of the cooling air, is rather similar to different cooling air flows. The temperature goes up as the rotating speed increases. The temperature in this gap will first decrease when the cooling air increases under lower mass flow rate. But when the cooling air continues to increase, the temperature will rise up indicating the existence of an optimum value of the cooling flow rate.

In recent days, nanofluids have derived the attention of researchers, scientists and engineers due to their abundant applications in Engineering and technology and specific applications such as Electronics cooling, vehicle cooling, medical applications including cancer therapy and so on. Motivated by these applications of nanofluids, this work is mainly concerned with the convective heat transfer of nanofluids. MHD slip flow of nanofluids with heat absorption over a rotating disk subjected to suction has been analyzed. Two types of nanofluids such as copper-water nanofluid and silver-water nanofluid are considered for the present study. The system of axisymmetric nonlinear partial differential equations governing the hydromagnetic steady flow and heat transfer are reduced to nonlinear ordinary differential equations by introducing suitable similarity transformations. The resulting non-linear ordinary differential equations are solved numerically by most efficient Nachtsheim-Swigert shooting iteration technique for satisfaction of asymptotic boundary conditions along with Runge – Kutta Fehlberg Method. The flow field is affected by the presence of physical parameters, such as magnetic interaction parameter, suction parameter, slip parameter and solid volume fraction, whereas the temperature field is addionally affected by magnetic interaction parameter, suction parameter, internal heat absorption parameter and solid volume fraction. With the amplifying effect in magnetic interaction parameter, suction parameter, slip parameter and solid volume fraction, the radial and tangential velocities decline. Axial velocity gets decelerated for increasing magnetic interaction parameter and slip parameter whereas it gets accelerated for growing effect of suction parameter and solid volume fraction. The temperature of the fluid within the boundary layer enhances with the increasing effect of magnetic interaction parameter and solid volume fraction while it reduces for increasing values of the suction parameter and internal heat absorption parameter. Also the values of radial and tangential skin friction coefficients and Nusselt number are obtained numerically and are tabulated.

This research investigates the effect of uniform suction on the absolute instability of Type I cross-flow modes in the boundary layer on a rotating disk. Specifically, it is designed to investigate whether wall suction would transform the absolute instability into a global mode, as first postulated in the numerical simulations of Davies & Carpenter (J. Fluid Mech., vol. 486, 2003, pp. 287–329). The disk is designed so that with a suction parameter of 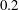$0.2$, the radial location of the absolute instability critical Reynolds number, 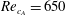$Re_{c_{A}}=650$, occurs on the disk. Wall suction is applied from $Re=317$ to 696.5. The design for wall suction follows that of Gregory & Walker (J. Fluid Mech., 1960, pp. 225–234) where an array of holes through the disk communicate between the measurement side of the disk and the underside of the disk which is inside of an enclosure that is maintained at a slight vacuum. The enclosure pressure is adjustable so that a range of suction or blowing parameters can be investigated. The holes in the measurement surface are covered by a compressed wire porous mesh to aid in uniformizing the suction on the measurement surface of the disk. The mesh is covered by a thin porous high-density polyethylene sheet featuring a $20~{\rm\mu}\text{m}$ pore size which provides a smooth finely porous surface. A companion numerical simulation is performed to investigate the effect that the size and vacuum pressure of the underside enclosure have on the uniformity of the measurement surface suction. Temporal disturbances are introduced using the method of Othman & Corke (J. Fluid Mech., 2006, pp. 63–94). The results document the evolution of disturbance wavepackets in space and time. These show a temporal growth of the wavepackets as the location of the absolute instability is approached which is in strong contrast to the temporal evolution without suction observed by Othman and Corke. The results appear to support the effect of wall suction on the absolute instability postulated by Thomas (PhD thesis, 2007, Cardiff University, UK) and Thomas & Davies (J. Fluid Mech., vol. 663, 2010, pp. 401–433).

In this paper, a thermoelastic analysis of rotating disks with different thickness profiles made of functionally graded materials (FGMs) subjected to internal pressure is presented. Material properties (except Poisson’s ratio) and disk thickness profile are described by means of two functions namely power and exponential function. A comparative study of thermoelastic analysis is given for material properties and disk thickness profiles. The results of are compared with those obtained by finite element method (FEM) that shows good agreement. The effect of thickness profiles, gradient parameters and angular velocity on the thermoelastic performance of the disk have been studied.

The present paper studies the three-dimensional, off centered stagnation flow of a Jeffrey fluid over a rotating disk. The governing non-linear equations and their associated boundary conditions are transformed into coupled ordinary differential equations by utilizing an appropriate similarity transformation. Homotopy analysis method is utilized to evaluate the analytical solution in the form of infinite series. Also, the convergence region of the obtained solution is determined and plotted. The effects of pertaining parameters on radial, azimuthal and induced velocities of the fluid flow are presented graphically and discussed. Moreover comparisons have also been made with the previous results as a special case.

In this work a numerical investigation has been conducted to study the unsteady oscillatory flow of a viscous fluid induced by a swirling disk. The disk stretches radially with the time-based sinusoidal oscillations. The governing equations for the three-dimensional boundary layer-flow are normalized using a suitable set of similarity transformations. The normalized partial differential equations are then solved numerically using a finite difference scheme by altering the semi-infinite domain to a finite domain. The effects of various imperative parameters on the oscillatory flow are discussed with graphs and tables.

La séparation de la phase huileuse à partir d'effluents huile-eau comportant des émulsions secondaires est très difficile par les procédés traditionnels et nécessite l'emploi de l'ultrafiltration. Des émulsions huile-eau contenant 2 et 4 % d'huile ont été ultrafiltrées à l'aide d'un prototype de filtration dynamique comportant un disque tournant à grande vitesse près d'une membrane de 50 kDa de 190 cm2 de surface. La turbidité de la solution initiale à 4 % (21 900 NTU) chute à 2 NTU dans le perméat après 15 min de filtration, ce qui représente une rétention de 99,5 % de l'huile. Les flux de perméat augmentent avec la vitesse de rotation du disque pour atteindre environ 90 l.h-1.m-2 à 2000 tr.min-1 à 24 °C. L'ajout d'ailettes de 6 mm de hauteur permet de doubler le flux de perméat à la même vitesse. Les microémulsions sont constituées de 4 à 20 % de cyclohexane, 0,5 à 16 % de 2-butanol et de 0,25 à 8 % de dodecyl sulfate de sodium (SDS) dans de l'eau pure. Les flux de perméat, très élevés (200 l.h-1.m-2) à 2000 tr.min-1 avec un disque lisse pour la solution la plus diluée chutent à 15 l.h-1.m-2 pour la plus concentrée. L'ajout d'ailettes permet de doubler ces flux.