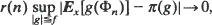Collective synchrony refers to the simultaneous occurrence of behavior, cognition, emotion, and/or physiology within teams of three or more persons. It has been suggested that collective synchrony may emanate from the copresence of team members, from their engagement in a shared task, and from coordination enacted in pursuit of a collective goal. In this paper, a regime-switching dynamic factor analytical approach is used to examine interindividual similarities in a particular behavioral measure (i.e., speed) in a collegiate soccer team. First, the analytical approach is presented didactically, including the state space modeling framework in general, followed by the regime-switching dynamic factor model in particular. Next, an empirical application of the approach is presented. Speed similarity (covariation in speed, operationalized in two ways: running cadence and distance covered) during competitive women’s soccer games is examined. A key methodological aspect of the approach is that the collective is the unit of analysis, and individuals vary about collective dynamics and their evolution. Reporting on the results of this study, we show how features of substantive interest, such as the magnitude and prevalence of behavioral similarity, can be parameterized, interpreted, and aggregated. Finally, we highlight several key findings, as well as opportunities for future research, in terms of methodological and substantive aims for advancing the study of collective synchrony.