The object of this paper is to study the stochastic asymptotic exponential stability of a stochastic integral equation of the form

A random solution of the stochastic integral equation is considered to be a second order stochastic process satisfying the equation almost surely. The random solution, y(t, ω) is said to be. stochastically asymptotically exponentially stable if there exist some β > 0 and a γ > 0 such that 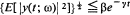 for t∈ R+.
for t∈ R+.
The results of the paper extend the results of Tsokos' generalization of the classical stability theorem of Poincaré-Lyapunov.


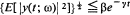 for
for 