1 results
Local Rankin–Selberg integrals for Speh representations
- Part of
-
- Journal:
- Compositio Mathematica / Volume 156 / Issue 5 / May 2020
- Published online by Cambridge University Press:
- 13 April 2020, pp. 908-945
- Print publication:
- May 2020
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
-
We construct analogues of Rankin–Selberg integrals for Speh representations of the general linear group over a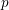
$p$-adic field. The integrals are in terms of the (extended) Shalika model and are expected to be the local counterparts of (suitably regularized) global integrals involving square-integrable automorphic forms and Eisenstein series on the general linear group over a global field. We relate the local integrals to the classical ones studied by Jacquet, Piatetski-Shapiro and Shalika. We also introduce a unitary structure for Speh representation on the Shalika model, as well as various other models including Zelevinsky’s degenerate Whittaker model.
