We introduce a new affinely invariant structure on smooth surfaces in ℝ3 by defining a family of reflections in all points of the surface. We show that the bifurcation set of this family has a special structure at ‘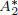 points’, which are not detected by the flat geometry of the surface. These
points’, which are not detected by the flat geometry of the surface. These 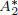 points (without an associated structure on the surface) have also arisen in the study of the centre symmetry set; using our technique we are able to explain how the points are created and annihilated in a generic family of surfaces. We also present the bifurcation set in a global setting.
points (without an associated structure on the surface) have also arisen in the study of the centre symmetry set; using our technique we are able to explain how the points are created and annihilated in a generic family of surfaces. We also present the bifurcation set in a global setting.