1 results
DISCRETENESS CRITERIA FOR ISOMETRIC GROUPS ACTING ON COMPLEX HYPERBOLIC SPACES
- Part of
-
- Journal:
- Bulletin of the Australian Mathematical Society / Volume 81 / Issue 3 / June 2010
- Published online by Cambridge University Press:
- 17 March 2010, pp. 481-487
- Print publication:
- June 2010
-
- Article
-
- You have access
- Export citation
-
In this paper, four new discreteness criteria for isometric groups on complex hyperbolic spaces are proved, one of which shows that the Condition C hypothesis in Cao [‘Discrete and dense subgroups acting on complex hyperbolic space’, Bull. Aust. Math. Soc.78 (2008), 211–224, Theorem 1.4] is removable; another shows that the parabolic condition hypothesis in Li and Wang [‘Discreteness criteria for Möbius groups acting on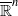
II’, Bull. Aust. Math. Soc.80 (2009), 275–290, Theorem 3.1] is not necessary.
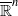II’, 