In this paper we introduce new local symbols, which we call 4-function local symbols. We formulate reciprocity laws for them. These reciprocity laws are proven using a new method - multidimensional iterated integrals. Besides providing reciprocity laws for the new 4-function local symbols, the same method works for proving reciprocity laws for the Parshin symbol. Both the new 4-function local symbols and the Parshin symbol can be expressed as a finite product of newly defined bi-local symbols, each of which satisfies a reciprocity law. The K-theoretic variant of the first 4-function local symbol is defined in the Appendix. It differs by a sign from the one defined via iterated integrals. Both the sign and the K-theoretic variant of the 4-function local symbol satisfy reciprocity laws, whose proof is based on Milnor K-theory (see the Appendix). The relation of the 4-function local symbols to the double free loop space of the surface is given by iterated integrals over membranes.

We show that higher order invariants of smooth functions can be written as linear combinations of full invariants times iterated integrals. The non-uniqueness of such a presentation is captured in the kernel of the ensuing map from the tensor product. This kernel is computed explicitly. As a consequence, higher order invariants form a free module of the algebra of full invariants.

We will introduce a regularization for $p$-adic multiple zeta values and show that the generalized double shuffle relations hold. This settles a question raised by Deligne, given as a project in the Arizona Winter School 2002. Our approach is to use the theory of Coleman functions on the moduli space of genus zero curves with marked points and its compactification. The main ingredients are the analytic continuation of Coleman functions to the normal bundle of divisors at infinity and definition of a special tangential base point on the moduli space.

In this note we are studying the Lie algebras associated to non-abelian unipotent periods on $P^1_{\mathbb{Q}(\mu_n)}\setminus\{0,\mu_n,\infty\}$. Let $n$ be a prime number. We assume that for any $m\geq 1$ the numbers $Li_{m+1}(\xi_n^k)$ for $1\leq k\leq (n-1)/2$ are linearly independent over $\mathbb{Q}$ in $\mathbb{C}/(2\pi\ri)^{m+1}\mathbb{Q}$. Let $S=\{k_1,\cdots,k_q\}$ be a subset of $\{1,\dots,p-1\}$ such that if $k\in S$, then $p-k\in S$ and $(S+S)\cap S=\emptyset$ (the sum of two elements of $S$ is calculated $\mathrm{Mod}p$). Then we show that in the Lie algebra associated to non-abelian unipotent periods on $P^1_{\mathbb{Q}(\mu_n)}\setminus \{0,\mu_n,\infty\}$ there are derivations $D^{k_1}_{m+1},\dots,D^{k_q}_{m+1}$ in each degree $m+1$ and these derivations are free generators of a free Lie subalgebra of this Lie algebra.

AMS 2000 Mathematics subject classification: Primary 11G55

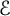
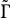
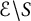
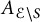
The operator Im is defined as m-fold indefinite integration with zero constants of integration. The zero distribution of Im(p) for polynomials p is studied in general, and for two special classes of polynomials in detail. The main results are: (i) The zeros of In(Pn), where Pn(𝑧) is the n-th Legendre polynomial, converge to a certain algebraic curve; (ii) the zeros of an integer) converge to pieces of a circle and of two "Szegö curves".