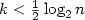Communication complexity of two-party (multiparty)protocols has established itself as a successful method for proving lower bounds on the complexity of concrete problems for numerous computing models. While the relations between communication complexity and oblivious, semilective computations are usually transparent and the main difficulty is reduced to proving nontrivial lower bounds on the communication complexity of given computing problems, the situation essentially changes, if one considers non-oblivious or multilective computations. The known lower bound proofs for such computations are far from being transparent and the crucial ideas of these proofs are often hidden behind some nontrivial combinatorial analysis. The aim of this paper is to create a general framework for the use of two-party communication protocols for lower bound proofs on multilective computations. The result of this creation is not only a transparent presentation of some known lower bounds on the complexity of multilective computations on distinct computing models, but also the derivation of new nontrivial lower bounds on multilective VLSI circuits and multilective planar Boolean circuits.In the case of VLSI circuits we obtain a generalization ofThompson's lower bounds on AT 2 complexity for multilective circuits.The Ω(n 2) lower bound on the number of gates of any k-multilectiveplanar Boolean circuit computing a specific Boolean function of n variablesis established for k<\frac{1}{2} \log_2 n 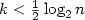 . Another advantage of this framework is that it provides lower bounds for a lot of concrete functions. This contrasts to the typical papersdevoted to lower bound proofs, where one establishes a lower bound for one or a few specific functions.
. Another advantage of this framework is that it provides lower bounds for a lot of concrete functions. This contrasts to the typical papersdevoted to lower bound proofs, where one establishes a lower bound for one or a few specific functions.