1 results
Simplicity of Partial Skew Group Rings of Abelian Groups
-
- Journal:
- Canadian Mathematical Bulletin / Volume 57 / Issue 3 / 01 September 2014
- Published online by Cambridge University Press:
- 20 November 2018, pp. 511-519
- Print publication:
- 01 September 2014
-
- Article
-
- You have access
- Export citation
-
Let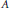
$A$ be a ring with local units, 
$E$ a set of local units for 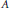$A$, 
$G$ an abelian group, and 
$\alpha$ a partial action of $G$ by ideals of 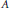$A$ that contain local units. We show that 
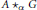$\text{A}\,{{\star }_{\alpha }}\,G$ is simple if and only if 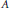$A$ is $G$-simple and the center of the corner 
$e{{\delta }_{0}}\left( \text{A}\,{{\star }_{\alpha }}\,G \right)e{{\delta }_{0}}$ is a field for all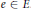 
$e\,\in \,E$. We apply the result to characterize simplicity of partial skew group rings in two cases, namely for partial skew group rings arising from partial actions by clopen subsets of a compact set and partial actions on the set level.
