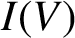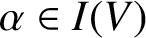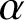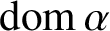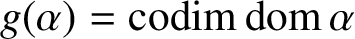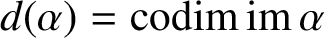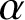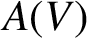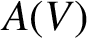Let V be an infinite-dimensional vector space over a field F and let
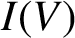 $I(V)$
be the inverse semigroup of all injective partial linear transformations on V. Given
$I(V)$
be the inverse semigroup of all injective partial linear transformations on V. Given
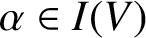 $\alpha \in I(V)$
, we denote the domain and the range of
$\alpha \in I(V)$
, we denote the domain and the range of
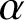 $\alpha $
by
$\alpha $
by
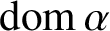 ${\mathop {\textrm {dom}}}\,\alpha $
and
${\mathop {\textrm {dom}}}\,\alpha $
and
 ${\mathop {\textrm {im}}}\,\alpha $
, and we call the cardinals
${\mathop {\textrm {im}}}\,\alpha $
, and we call the cardinals
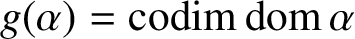 $g(\alpha )={\mathop {\textrm {codim}}}\,{\mathop {\textrm {dom}}}\,\alpha $
and
$g(\alpha )={\mathop {\textrm {codim}}}\,{\mathop {\textrm {dom}}}\,\alpha $
and
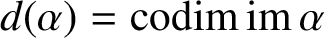 $d(\alpha )={\mathop {\textrm {codim}}}\,{\mathop {\textrm {im}}}\,\alpha $
the ‘gap’ and the ‘defect’ of
$d(\alpha )={\mathop {\textrm {codim}}}\,{\mathop {\textrm {im}}}\,\alpha $
the ‘gap’ and the ‘defect’ of
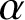 $\alpha $
. We study the semigroup
$\alpha $
. We study the semigroup
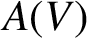 $A(V)$
of all injective partial linear transformations with equal gap and defect and characterise Green’s relations and ideals in
$A(V)$
of all injective partial linear transformations with equal gap and defect and characterise Green’s relations and ideals in
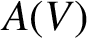 $A(V)$
. This is analogous to work by Sanwong and Sullivan [‘Injective transformations with equal gap and defect’, Bull. Aust. Math. Soc. 79 (2009), 327–336] on a similarly defined semigroup for the set case, but we show that these semigroups are never isomorphic.
$A(V)$
. This is analogous to work by Sanwong and Sullivan [‘Injective transformations with equal gap and defect’, Bull. Aust. Math. Soc. 79 (2009), 327–336] on a similarly defined semigroup for the set case, but we show that these semigroups are never isomorphic.