This paper discusses the stochastic monotonicity property of the conditional order statistics from independent multiple-outlier scale variables in terms of the likelihood ratio order. Let X1, …, Xn be a set of non-negative independent random variables with Xi, i=1, …, p, having common distribution function F(λ1x), and Xj, j=p+1, …, n, having common distribution function F(λ2x), where F(·) denotes the baseline distribution. Let Xi:n(p, q) be the ith smallest order statistics from this sample. Denote by 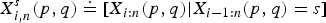 X_{i,n}^{s}(p,q)\doteq [X_{i:n}(p,q)|X_{i-1:n}(p,q)=s]. Under the assumptions that xf′(x)/f(x) is decreasing in x∈ℛ+, λ1≤λ2 and s1≤s2, it is shown that
X_{i,n}^{s}(p,q)\doteq [X_{i:n}(p,q)|X_{i-1:n}(p,q)=s]. Under the assumptions that xf′(x)/f(x) is decreasing in x∈ℛ+, λ1≤λ2 and s1≤s2, it is shown that 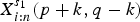 X_{i:n}^{s_{1}}(p+k,q-k) is larger than
X_{i:n}^{s_{1}}(p+k,q-k) is larger than 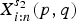 X_{i:n}^{s_{2}}(p,q) according to the likelihood ratio order for any 2≤i≤n and k=1, 2, …, q. Some parametric families of distributions are also provided to illustrate the theoretical results.
X_{i:n}^{s_{2}}(p,q) according to the likelihood ratio order for any 2≤i≤n and k=1, 2, …, q. Some parametric families of distributions are also provided to illustrate the theoretical results.