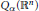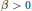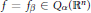A borderline case function $f$ for ${{Q}_{\alpha }}\left( {{\mathbb{R}}^{n}} \right)$ spaces is defined as a Haar wavelet decomposition, with the coefficients depending on a fixed parameter $\beta \,>\,0$. On its support ${{I}_{0}}\,=\,{{\left[ 0,\,1 \right]}^{n}},\,f\left( x \right)$ can be expressed by the binary expansions of the coordinates of $x$. In particular, $f\,=\,{{f}_{\beta }}\,\in \,{{Q}_{\alpha }}\left( {{\mathbb{R}}^{n}} \right)$ if and only if 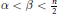$\alpha \,<\,\beta \,<\frac{n}{2}$ , while for 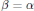$\beta \,=\,\alpha $, it was shown by Yue and Dafni that $f$ satisfies a John–Nirenberg inequality for ${{Q}_{\alpha }}\left( {{\mathbb{R}}^{n}} \right)$. When $\beta \,\ne \,1$, $f$ is a self-affine function. It is continuous almost everywhere and discontinuous at all dyadic points inside 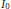${{I}_{0}}$. In addition, it is not monotone along any coordinate direction in any small cube. When the parameter $\beta \,\in \,\left( 0,\,1 \right)$, $f$ is onto from 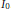${{I}_{0}}$ to 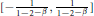$\left[ -\frac{1}{1-{{2}^{-\beta }}},\,\frac{1}{1-{{2}^{-\beta }}} \right]$ , and the graph of $f$ has a non-integer fractal dimension 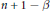$n\,+\,1\,-\beta$.